Mercury is poured into a U-tube in which the cross-sectional area of the left-hand limb is three times smaller than that of the right one. The level of the mercury in the narrow limb is a distandce l=30cm from the upper end of the tube. How much will the mercury level rise in the right-hand limb if the left one is filled to the top with water?
The correct Answer is:C
To solve the problem, we need to analyze the situation in the U-tube when the left limb is filled with water and the mercury levels adjust accordingly.
1. Understanding the U-tube Setup:
- Let the cross-sectional area of the left limb (A1) be A.
- The cross-sectional area of the right limb (A2) is 3A (since it is three times larger).
- The height of mercury in the left limb is given as l=30cm.
2. Filling the Left Limb with Water:
- When the left limb is filled to the top with water, the pressure exerted by the water column must be balanced by the pressure exerted by the mercury in the right limb.
3. Pressure Balance:
- The pressure exerted by the water column (height h) in the left limb can be expressed as:
Pwater=ρwatergh
- The pressure exerted by the mercury column in the right limb (height hm) is:
Pmercury=ρmercuryghm
- At equilibrium, the pressures must be equal:
ρwatergh=ρmercuryghm
4. Relating Heights and Areas:
- Since the area of the left limb is A and the area of the right limb is 3A, the volume of water added to the left limb will cause a rise in mercury in the right limb.
- The volume of water added (height h in left limb) will equal the volume of mercury displaced in the right limb:
Ah=3Ahm
- This simplifies to:
h=3hm⇒hm=h3
5. Finding the Height of Water:
- The height of water h in the left limb can be expressed in terms of the height of mercury hm:
h=30+3hm
- Substituting hm=h3 into the equation gives:
h=30+3(h3)⇒h=30+h
- This leads to a contradiction unless we consider the initial height of mercury in the left limb.
6. Final Calculation:
- To find the rise in the right limb, we need to solve for hm using the pressure balance:
ρwatergh=ρmercuryghm
- Rearranging gives:
hm=ρwaterρmercuryh
- Given that ρwater≈1000kg/m3 and ρmercury≈13600kg/m3:
hm=100013600⋅30≈0.0221m=2.21cm
Conclusion:
The mercury level will rise approximately 2.21 cm in the right-hand limb.
- Let the cross-sectional area of the left limb (A1) be A.
- The cross-sectional area of the right limb (A2) is 3A (since it is three times larger).
- The height of mercury in the left limb is given as l=30cm.
- When the left limb is filled to the top with water, the pressure exerted by the water column must be balanced by the pressure exerted by the mercury in the right limb.
- The pressure exerted by the water column (height h) in the left limb can be expressed as:
Pwater=ρwatergh
- The pressure exerted by the mercury column in the right limb (height hm) is:
Pmercury=ρmercuryghm
- At equilibrium, the pressures must be equal:
ρwatergh=ρmercuryghm
- Since the area of the left limb is A and the area of the right limb is 3A, the volume of water added to the left limb will cause a rise in mercury in the right limb.
- The volume of water added (height h in left limb) will equal the volume of mercury displaced in the right limb:
Ah=3Ahm
- This simplifies to:
h=3hm⇒hm=h3
- The height of water h in the left limb can be expressed in terms of the height of mercury hm:
h=30+3hm
- Substituting hm=h3 into the equation gives:
h=30+3(h3)⇒h=30+h
- This leads to a contradiction unless we consider the initial height of mercury in the left limb.
- To find the rise in the right limb, we need to solve for hm using the pressure balance:
ρwatergh=ρmercuryghm
- Rearranging gives:
hm=ρwaterρmercuryh
- Given that ρwater≈1000kg/m3 and ρmercury≈13600kg/m3:
hm=100013600⋅30≈0.0221m=2.21cm
The mercury level will rise approximately 2.21 cm in the right-hand limb.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question2videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Single Correct36videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct49videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
A U-tube in which the cross-sectional area of the limb on the left is one-third of the limb on the right contains mercury (density 13.6g/cm3). The level of mercury in the narrow limb extends to a distance of 30cm from the upper end of the tube. What will be the rise in the level of mercury in the right limb, if the left limb is filled to the top with water (neglect surface tension effects)?
 View Solution
View SolutionA U-shaped tube open to the air at both ends contains some mercury. A quantity of water is carefully poured into the left arm of the U-shaped tube until the vertical height of the water column is 15.0cm.
(a) What is the gauge pressure at the water mercury interface ?
(b) Calculate the vertical distance h from the top of the mercury in the right hand arm of the tube to the top of the water in the left-hand arm.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
A U -tube in which the cross - sectional area of the limb on the left is one quarter, the limb on the right contains mercury (density13.6g/cm3). The level of mercury in the narrow limb is at a distance of 36 cm from the upper end of the tube. What will be the rise in the level of mercury in the right limb if the left limb is filled to the top with water ?
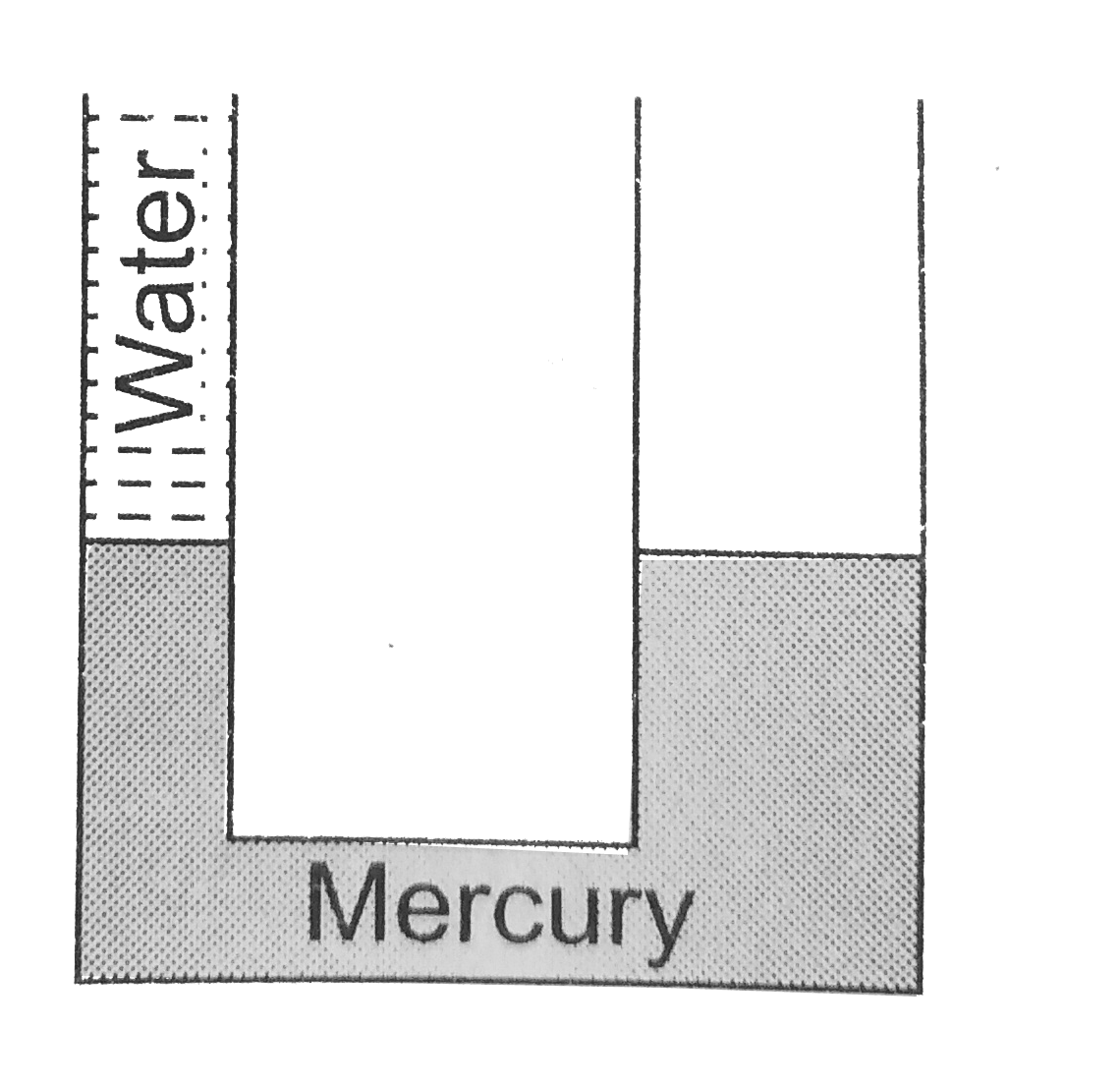 A1.2 cmB2.35 cmC0.56 cmD0.8 cm
A1.2 cmB2.35 cmC0.56 cmD0.8 cm - Question 2 - Select One
An open U-tube contains mercury. When 11.2 cm of water is poured into one of the arms of the tube, how high does the mercury rise in the other arm from its initial level ?
A0.82 cmB1.35 cmC0.41 cmD2.32 cm - Question 3 - Select One
Arrange the following steps in proper sequence to determine the pressure exerted by a gas enclosed in a container using a manometer.
(a)Connect one end of the manometer to the container filled with gas.
(b)Both the limbs of the manometer are exposed to air, mercury level in both the limbs are equal due to atmospheric pressure.
(c )Determine the difference in level of the mercury in both the limbs of the manometer (l).
(d) If the level of the mercury in the limb connected to the container is above the mercury level in the limb exposed to air, the pressure of the gas in the container is determined as atmospheric pressure -l.
(e ) If the level of the mercury in the limb connected to the container is below the mercury level in the limb exposed to air, the pressure of the gas in the container is determined as atmospheric pressure +l.
(f) Note the level of the mercury in the limbs of the manometer.Aa b c e f eBe b a d c fCb a c f d eDb c d a f e
Two communicating cylindrical tubes contain mercury. The diameter of one vessel is four times large than the diameter of the outer. A column of water of height 70cm is poured into the narrow vessel. How much will the mercury level rise in the other vessel and how much will it sink in the narrow one? How much will the mercury level rise in the narrow vessel, if a column of water of the same height is poured into the broad vessel?
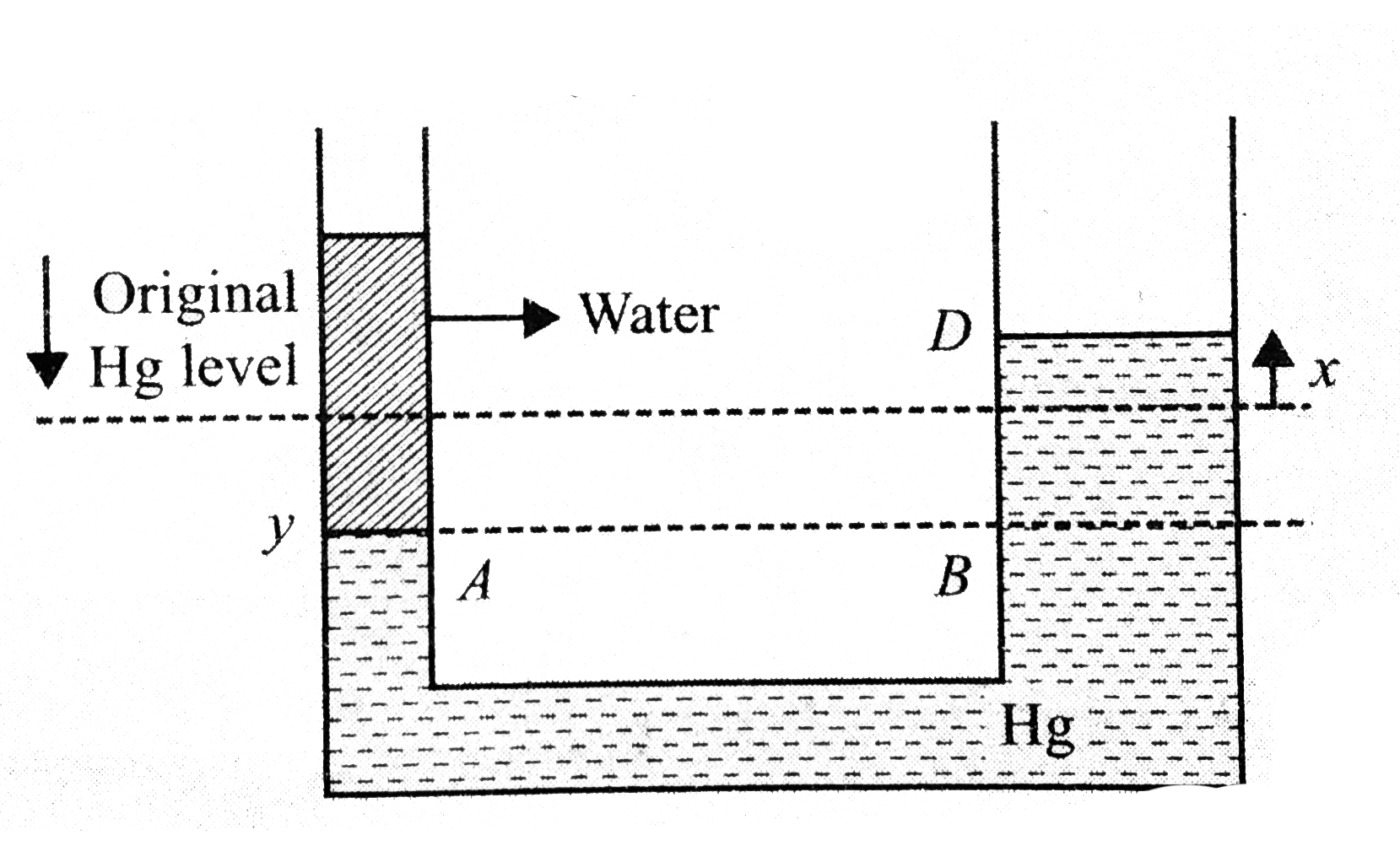 View Solution
View SolutionWhen one limb of a manometer is connected to a container filled with a gas, the level of the mercury in the other limb rises by 'h' cm. Then the pressure of the gas in the container is 76+h cm of Hg.
View SolutionBoth limbs of a 'U' tube are of equal length. One of the limbs is sealed and contains a column of 28 cm of air at atmospheric pressure. The air is separated from the atmosphere by mercury. What will be the height of air in the sealed limb, if the other limb is now filled to the top with mercury ? Atmospheric pressure is 76 cm of mercury.
View SolutionArrange the following steps in proper sequence to determine the pressure exerted by a gas enclosed in a container using a manometer.
(A) Connect one end of the manometer to the container filled with gas.
(B) Both the limbs of the manometer are exposed to air, mercury level in both the limbs are equal due to atmospheric pressure
(C) Determine the difference in level of the mercury in both the limbs of the manometer (t).
(D) If the level of the mercury in the limb connected to the container is above the mercury level in the limb exposed to air, the pressure of the gas in the container is determined as atmospheric pressure - l
(E) If the level of the mercury in the limb connected to the container is above the mercury level in the limb exposed to air, the pressure of the gas in the container is determined as atmospheric pressure +l
(F) Note the level of the mercury in the limbs of the monometer.View SolutionTwo communicating vessels contain mercury. The diameter of one vessel in n times larger then the diameter of the other. A column of water of height h is poured into the left vessel. The mercury level will rise in the right hand vessel (s = relative density of mercury and ρ = density of water) by
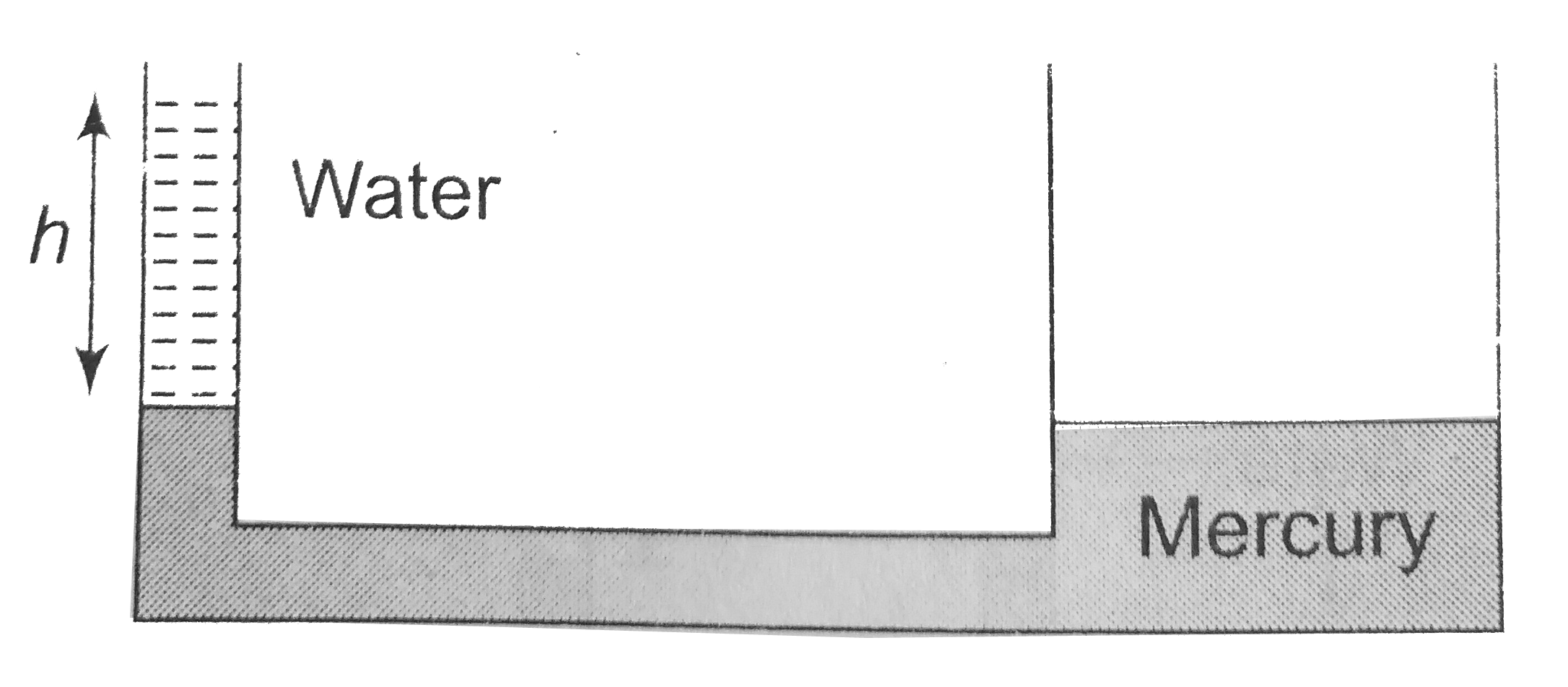 View Solution
View Solution

