A uniform rod AB, 4m long and weighing 12 kg, is supported at end A, with a 6 kg lead weight at B. The rod floats as shown in figure with one-half of its length submerged. The buoyant force on the lead mass is negligible as it is of negligible volume. Find the tension in the cord and the total volume of the rod
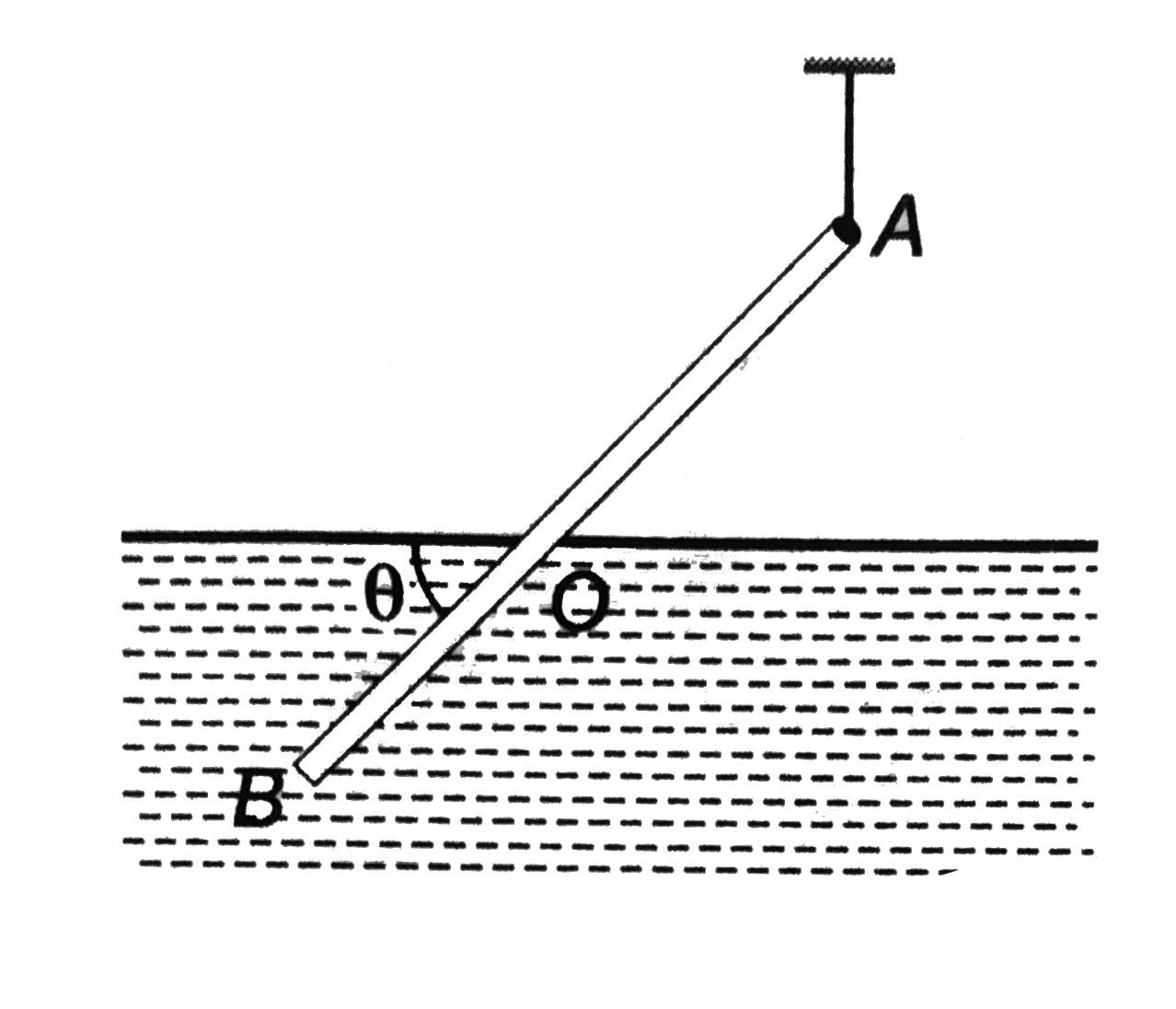
The correct Answer is:A, B, C
T+U=60+w…(i)
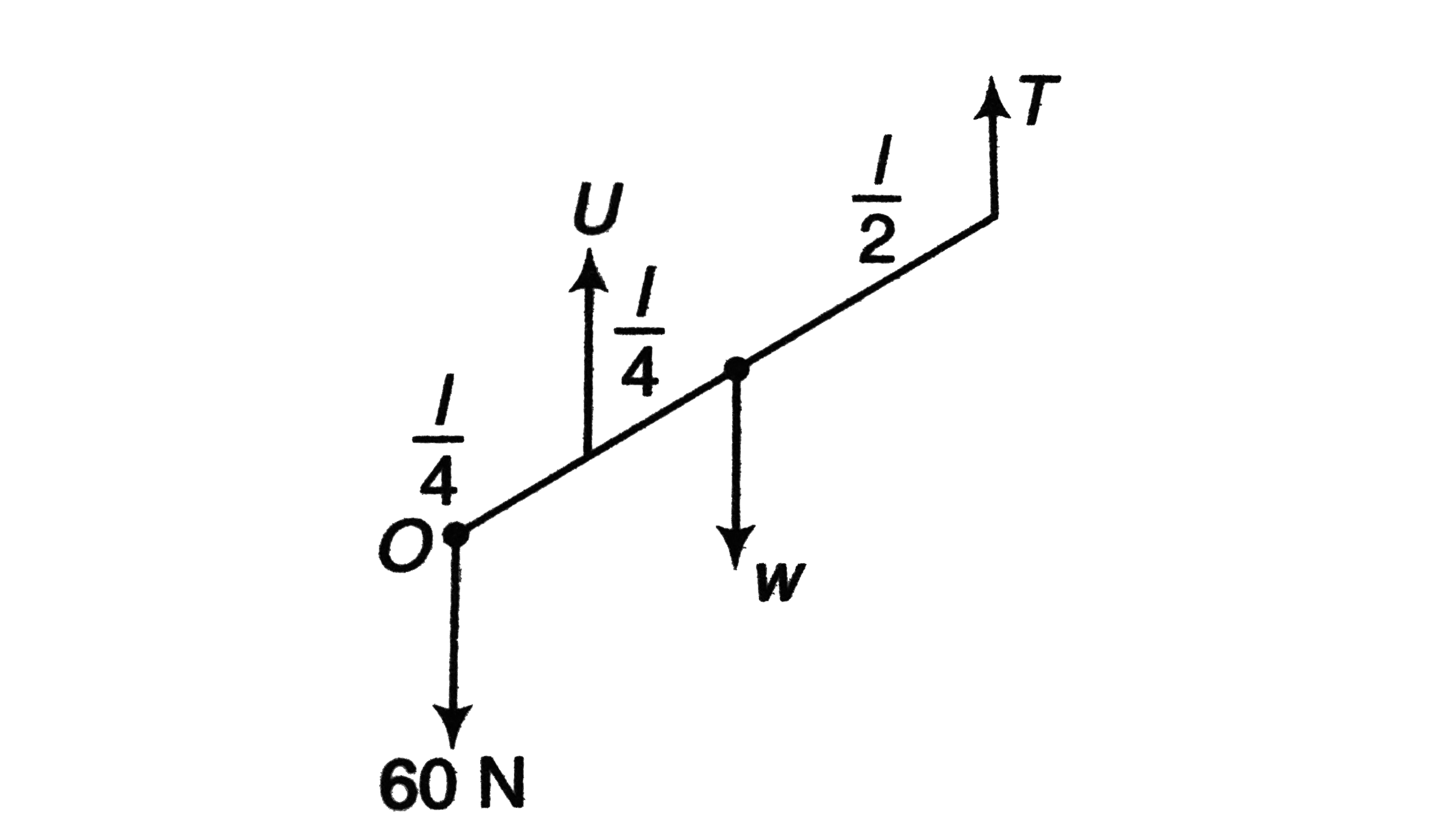
Σ (Moments) about =O
∴=U(l4)+T(l)=W(l2)
or U+4T=2W …(ii)
w=120N….(iii)
Solving these three equations we get T=20n and U=160N
Now, 160(V2)ρwg=(V2)(1000)(10)
∴V=32×10−3m3.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Subjective Question2videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 2 Single Correct36videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - FLUID MECHANICSEXERCISE - Level 1 Single Correct49videos
FLUID MECHANICS
- BOOK - DC PANDEYCHAPTER - EXPERIMENTSEXERCISE - Subjective15videos
EXPERIMENTS
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
Similar Questions
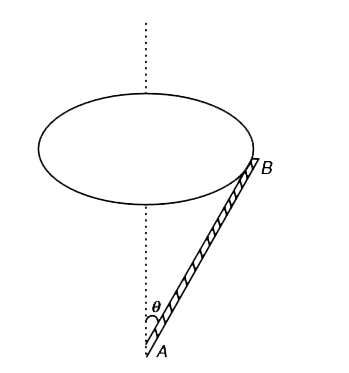
A uniform rod of mass m and length L is fixed to an axis, making an angle θ with it as shown in the figure. The rod is rotated about this axis so that the free end of the rod moves with a uniform speed ‘v’. Find the angular momentum of the rod about the axis. Is the angular momentum of the rod about point A constant?
 View Solution
View SolutionA uniform rod of weight Fg and length L is supported at its ends by a frictionless through as shown in figure. (a) Show that the centre of gravity of the rod must be vertically over point O when the rod is in equilibrium. (b) Determine the equilibrium value of the angle θ.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A uniform rod is 4m long and weights 10kg. If it is supported on a kinetic edge at one meter from the end, what weight placed at that end keeps the rod horizontal.
A8kgB10kgC12kgD15kg - Question 2 - Select One
A uniform rod of length L and weight WR is suspended as shown in the accompanying figure. A weight W is added to the end of the rod. The rod rests in horizontal position and is hinged at O. the support wire is at an angle θ to the rod. What is the tension T in the wire ?
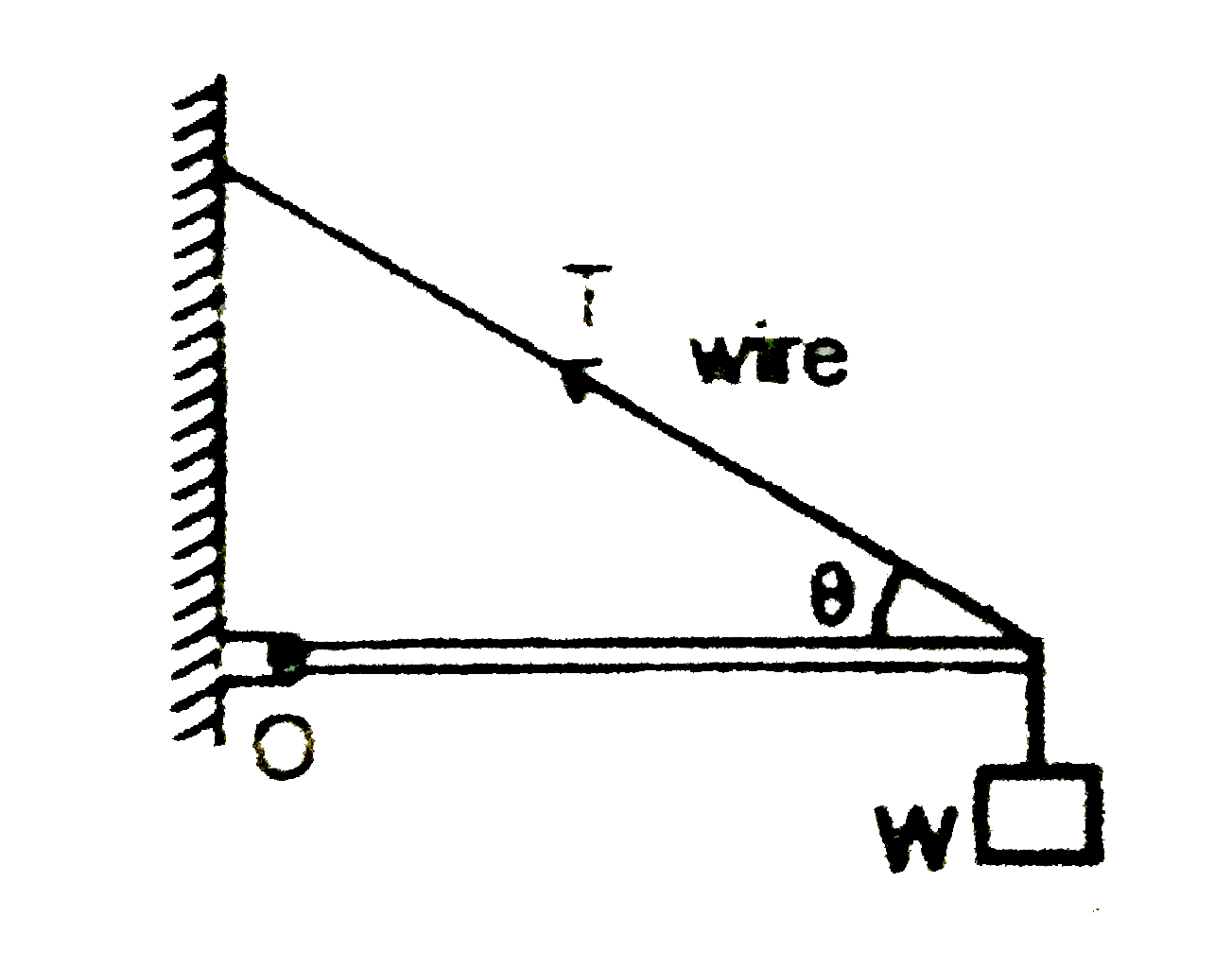 AT=WsinθBT=W+WRCT=W+1/2WRDT=W+1/2WRsinθ
AT=WsinθBT=W+WRCT=W+1/2WRDT=W+1/2WRsinθ - Question 3 - Select One

A uniform rod AB of mass m and length L rotates about a fixed vertical axis making a constant angle θ with it as shown in figure. The rod is rotated about this axis, so that point B the free end of the rod moves with a uniform speed V in the horizontal plane then the angular momentum of the rod about the axis is:A12mVLcosθB13mVLsinθCmVLsincosθDzero
A ligh rod of length 1 m is pivoted at its centre and two masses of 5 kg and 2 kg are hung from the ends as shown in the figure. Find the initial angular acceleration of the rod assuming that it was horizontal in the beginning.
 View Solution
View SolutionA uniform rod of length 4 m and mass 20 kg is lying horizontal on the ground. The work done in keeping it vertical with one of its ends touching the ground, will be -
View SolutionSuppose the rod in the previous problem has a mass of 1 kg distributed uniformly over its length.
a. find the initial angular acceleration of the rod.
b. Find the tension in the supports to the blocks of mass 2 kg and 5kg.View SolutionA uniform rod of mass 20 Kg and length 1.6 m is piovted at its end and swings freely in the vertical plane. Angular acceleration of the rod just after the rod is relased from rest in the horizontal position is
 View Solution
View SolutionA uniform rod of length L pivoted at the bottom of a pond of depth L/2 stays in stable equilibrium as shown in the figure.

Find the force acting at the pivoted end of the rod in terms of mass m of the rod.View Solution

