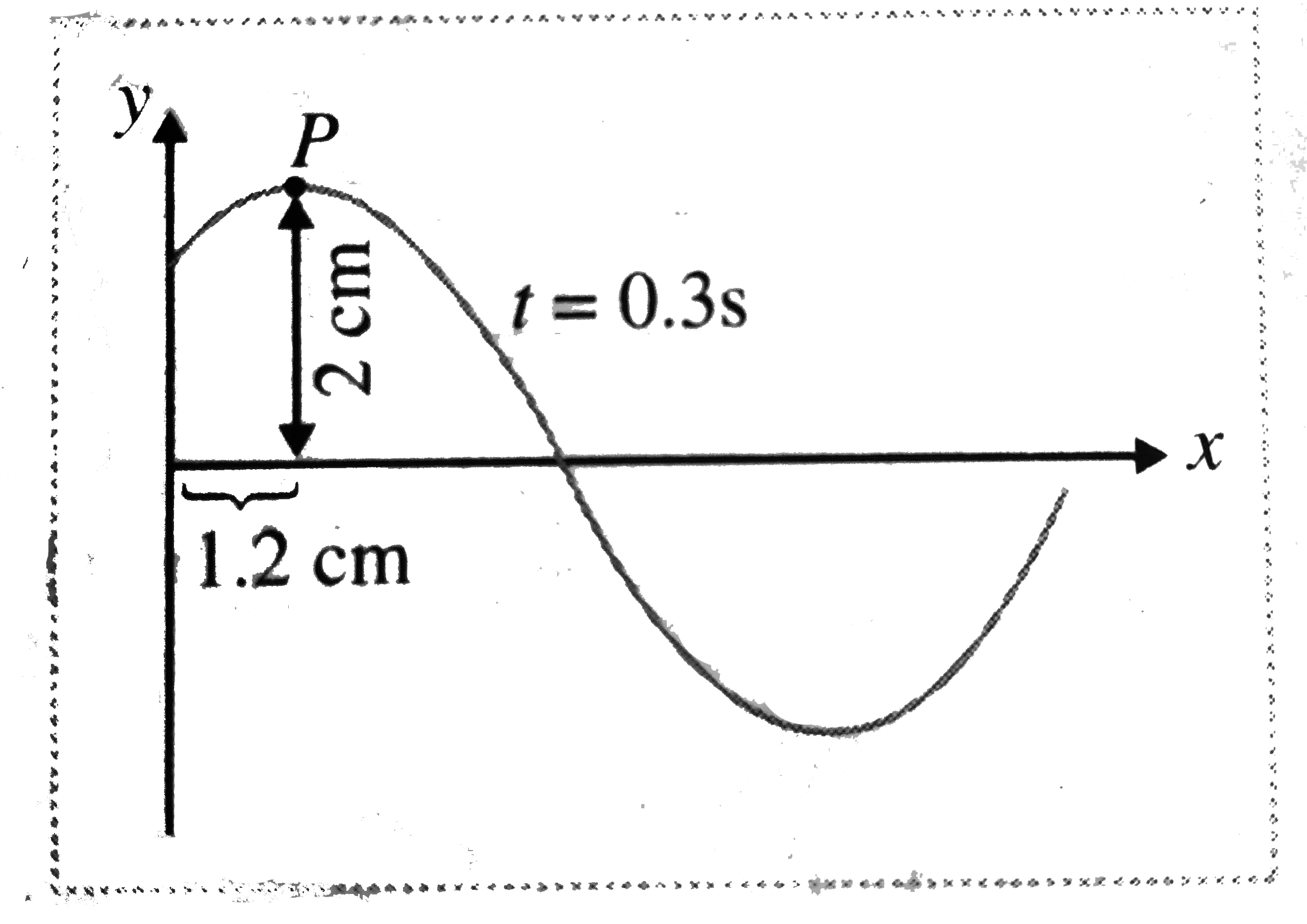
Figure shows a snapshot of a sinusoidal travelling wave taken at t=0.3s.The wavelength is 7.5cm and the amplitude is 2cm. If the crest P was at x=0at t=0, write the equation of travelling wave. ,

The correct Answer is:A, B, C, D
Given, A=2cm,λ=7.5cm
∴k=2πλ=0.84cm−1
The wave has travelled a distance of 1.2cm in 0.3s. Hence, speed of the wave,
v=1.20.3=4cm/s
∴ Angular frequency ω=vk=3.36rad/s
Since the wave is travelling along positive x-direction and crest (maximum displacement) is at x=0 at t=0 , we can write the wave equation as
y(x,t)=Acos(kx−ωt)
or y(x,t)=Acos(ωt−kx) as cos(−θ)=cosθ
Therefore, the desired equation is
y(x,t)=(2cm)cos[(0.84cm−1x−(3.36rad/s)t]cm
Topper's Solved these Questions
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Example Type 5
1 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Miscellaneous Examples
9 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Example Type 4
1 Videos
- VECTORSBOOK - DC PANDEYCHAPTER - VECTORSEXERCISE - Medical enrances gallery
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
Similar Questions
Knowledge Check
A transverse sinusoidal wave moves along a string in the positive x-direction at a speed of 10cm/s. The wavelength of ythe wave is 0.5m and its amplitude is 10cm. At a particular time t, the snap-shot of the wave is shown in figure. The velocity of point P when its displacement is 5cm is -
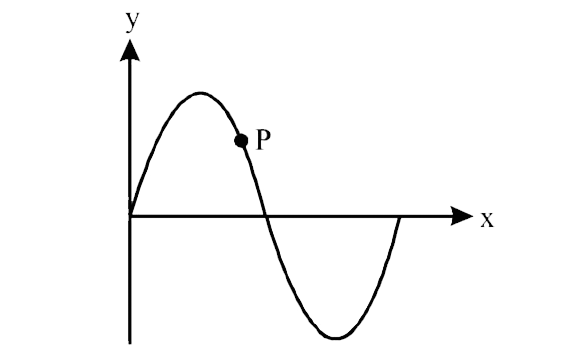 A(a) √350ˆJm/sB(b) −√350ˆJm/sC( c ) √350ˆim/sD(d) −√350ˆim/s
A(a) √350ˆJm/sB(b) −√350ˆJm/sC( c ) √350ˆim/sD(d) −√350ˆim/sA transverse wave, whose amplitude is 0.5 m wavelength is 1 m and frequency is 2Hz. is travelling along positive x direction. The equation of this wave will be-
Ay=0.5cos(2πx−4πt)By=0.5cos(2πx−4πt)Cy=0.5sin(πx−2πt)Dy=0.5cos(2πx−2πt)A progressive wave is travelling along the positive direction of the x-axis. For this wave, amplitude = 5 cm, period = 0.2 s and wavelength = 30 cm
The equation of the wave using SI units is given byAy=0.05sin2π(t0.2−x0.3)By=0.5sin2π(t0.2−x30)Cy=5sin2π(t0.2+x3)Dy=0.05sin2π(x0.3+t0.2)
A snapshot is taken of a periodic wave travelling on a string as shown below. What is the amplitude and wavelength of the wave?
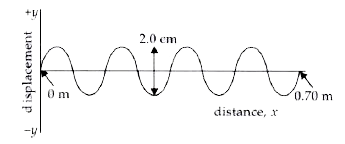
View SolutionTwo sine waves of same frequency and amplitude, travel on a stretched string in opposite directions. Speed of each wave is 10 cm/s. These two waves superimpose to form a standing wave pattern on the string. The maximum amplitude in the standing wave pattern is 0.5 mm. The figure shows the snapshot of the string at t = 0.Write the equation of the two travelling waves.

View SolutionA transverse sinusoidal wave moves along a string in the positive x-direction at a speed of 10 cm/s. The wavelength of the wave is 0.5 m and its ampli- tude is 10 cm. At a particular time t, the snap-shot of the wave is shown in figure. The velocity of point P when its displacement is 5 cm is Figure :
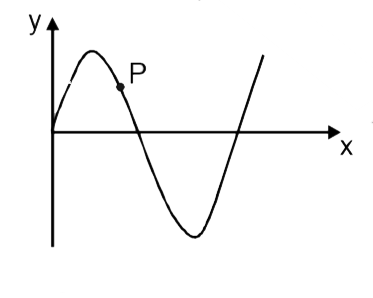
View SolutionA transverse sinusoidal wave moves along a string in the positive x-direction at a speed of 10cm/s. The wavelength of the wave is 0.5 m and its amplitude is 10cm. At a particular time t,the snapshot of the wave is shown in figure. The velocity of point P when its displacement is 5 cm is

(a) √3π50ˆjm/s
(b)−√3π50ˆjm/s
(c)√3π50ˆim/s
(d) −√3π50ˆim/s
View SolutionFigure shows a plot of the transverse displacements of the particles of a string at t = 0 through which a travelling wave is passing in the positive x-direction. The wave speed is 20cms−1. Find (a) the amplitude, (b) the wavelength, (c) the wave number and (d) the frequency of the wave.
 .
.
View Solution

_PHY_E06_334_Q01.png)