For a wave described by $y = A sin (ω t - k x)$ , consider the following or not and in what direction and describe whether the particle is speeding up, slowing sown or instantanteously not accelerating?

Video Solution

Text Solution

Verified by Experts

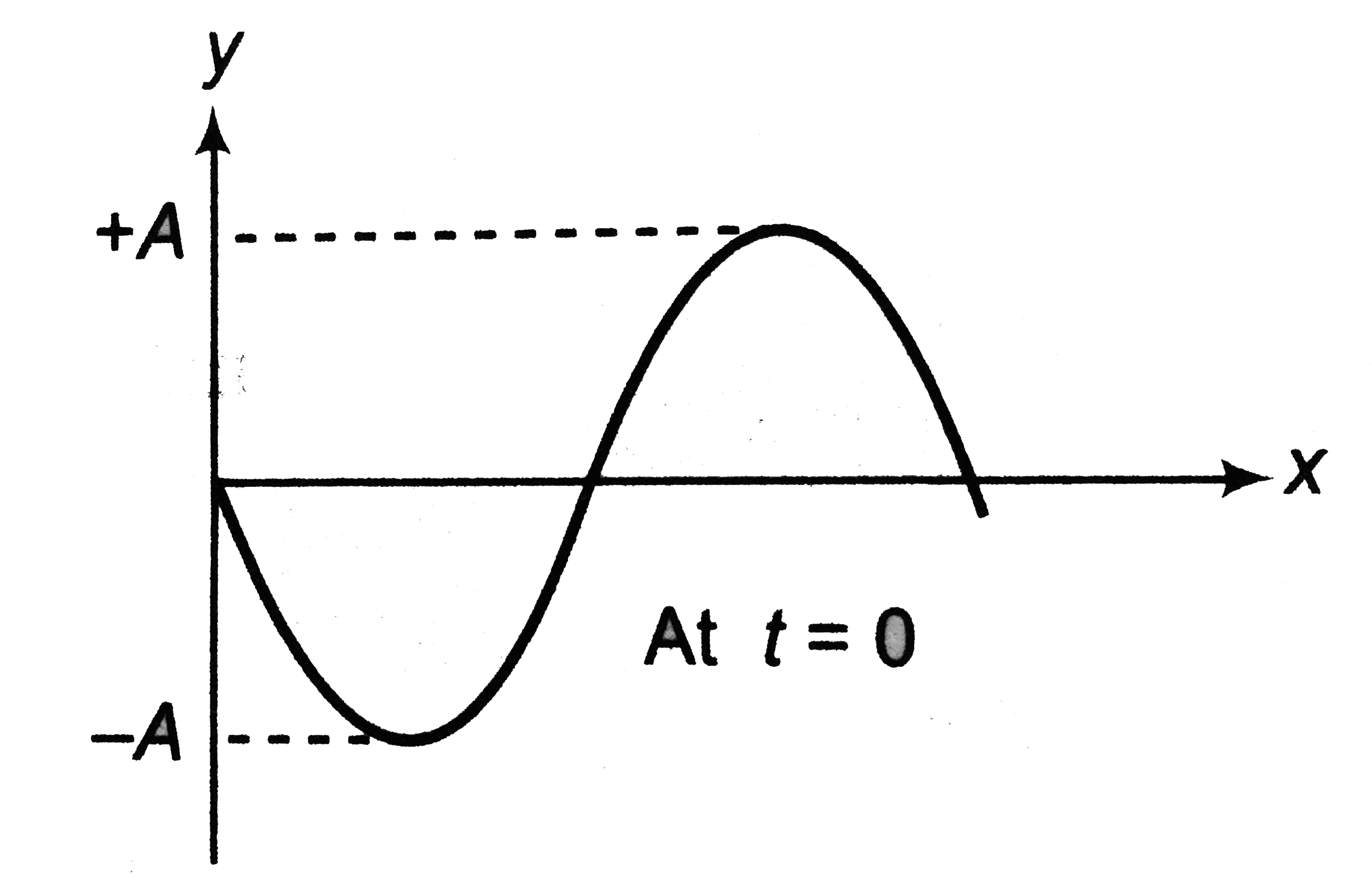
$y = A sin (ω t - k x)$
Particle velocity $v_{p} (x, t) = \frac{\partial y}{\partial t} = ω A cos (ω t - k x)$ and particle acceleration
$a_{p} (x, t) = \frac{\partial^{2} y}{\partial t} = - ω^{2} A sin (ω t - k x)$
(a) $t = 0, x = 0 : v_{p} = + ω A$ and $a_{p} = 0$
i.e. particle is moving upward but its acceleration is zero.
(b) $t = 0$ , $x = \frac{π}{4 k}$ $x = \frac{π}{4 k}$
$∴ k x = \frac{π}{4}$
$v_{p} = ω A cos (- \frac{π}{4}) = + \frac{ω A}{\sqrt{2}}$
and $a_{p} = - ω^{2} A sin (- \frac{π}{4}) = + \frac{ω^{2} A}{\sqrt{2}}$
Velocity of particle is positive, i.e. the particle is moving upward (along positive y- direction). Further $(v_{P})$ and $(a_{p}) a r e \in t h e s a m e d i r e c t i o n (⊥ h a r e p o s i t i v e) . H e n c e, t h e p a r t i c \leq i s s p e e d \in g u p . (C)$ t =0 , x =(pi)/(2k) , x=(pi)/(2k) :. kx = (pi)/(2) $$ v_(p) =omegaA cos(-pi//2) = 0 $$ a_(p) = -omega^(2) A sin (-pi//2) =omega^(2) A $ i . e . p a r t i c \leq i s s t a t i o n a r y or a t i t s e x t e r m e p o s i t i o n$ (y = - A) $. S o, i t i s s p e e d \in g u p a t t h i s \in s tan t . (d)$ t =0 , x= (3pi)/(4k) , x = (3pi)/(4k) :. kx = (3pi)/(4) $$ :. v_(p) = omegaA cos (-(3pi)/(4)) = -(omegaA)/(sqrt(2)) $$ a_(p) = -omega^(2)A sin(-(3pi)/(4)) = + -(0megaA)/sqrt(2) $$ a_(p) = - omega^(2) A sin (-(3pi)/(4))= + (omega^(2)A )/sqrt(2) $ V e l o c i t y o f p a r t i c \leq i s \neg a t i v e, i . e . t h e p a r t i c \leq i s m o v \in g d o w n w a r d s . F u r t h e r$ v_(p) $and$ (a_(P)) are in opposite direction , i.e. the particle is slowing down.

Updated on:21/07/2023