Four positive charges (2√2−1)Q are arranged at the four corners of a square. Another charge q is placed at the center of the square. Resulting force acting on each corner charge is zero if q is
−7Q4
−4Q7
−Q
−(√2+1)Q
The correct Answer is:A
..
Q1=(2√2−1)Q
F1 = force between Q1andQ1 at distance a
F2=ForcebetweenQ_1 and Q_1atdistancesqrt2a<br>F_3=ForcebetweenQ_1andqatbetweena/sqrt2<br>forF_3→be∈theshowndirection,qandQ_1shod––haveoppositesigns.For≠tchar≥→bezeroonQ_1placedatP.<br>|F_3|=Restant–––––ofF_1, F_2 and F_1<br>:. (kQ_1q)/(a//sqrt2)^2=sqrt2F_1+F_2<br>=sqrt2[(kQ_1Q_2)/a^2]+(kQ_1Q_1)/(sqrt2a)^2<br>:. |q|=Q_1/2 [sqrt2+1/2]=((2sqrt2+1)/4)Q_1<br>=((2sqrt2+1)/4)(2sqrt2-1)Q<br>=7/4 Qorq=-7/4Q ltbr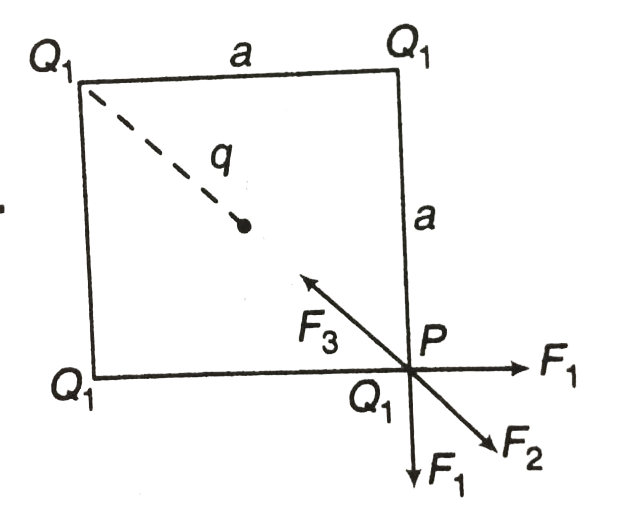 
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Subjective15videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - SUBJECTIVE_TYPE6videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Level 1 Assertion And Reason19videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions120videos
GRAVITATION
Similar Questions
Four identical charges, Q each, are fixed at the vertices of a square. A free charge q is placed at the centre of the square. Investigate the nature of equilibrium of charge q if it is to be displaced slightly along any of the two diagonals of the square.
View SolutionFour positive point charges Q are placed at the corners of a square of side a. Calculate the charge that should be placed at the centre so that system remains in equilibrium.
View Solution
Knowledge Check
- Question 1 - Select One
Four charges are arranged at the corners of a square ABCD, as shown. The force on a positive charge kept at the centre of the square is
AzeroBalong diagonal ACCalong diagonal BDDperpendicular to the side AB - Question 2 - Select One
Four charges are arranged at the corners of a square ABCD, as shown. The force on a =ve charge kept at the centre of the square is -
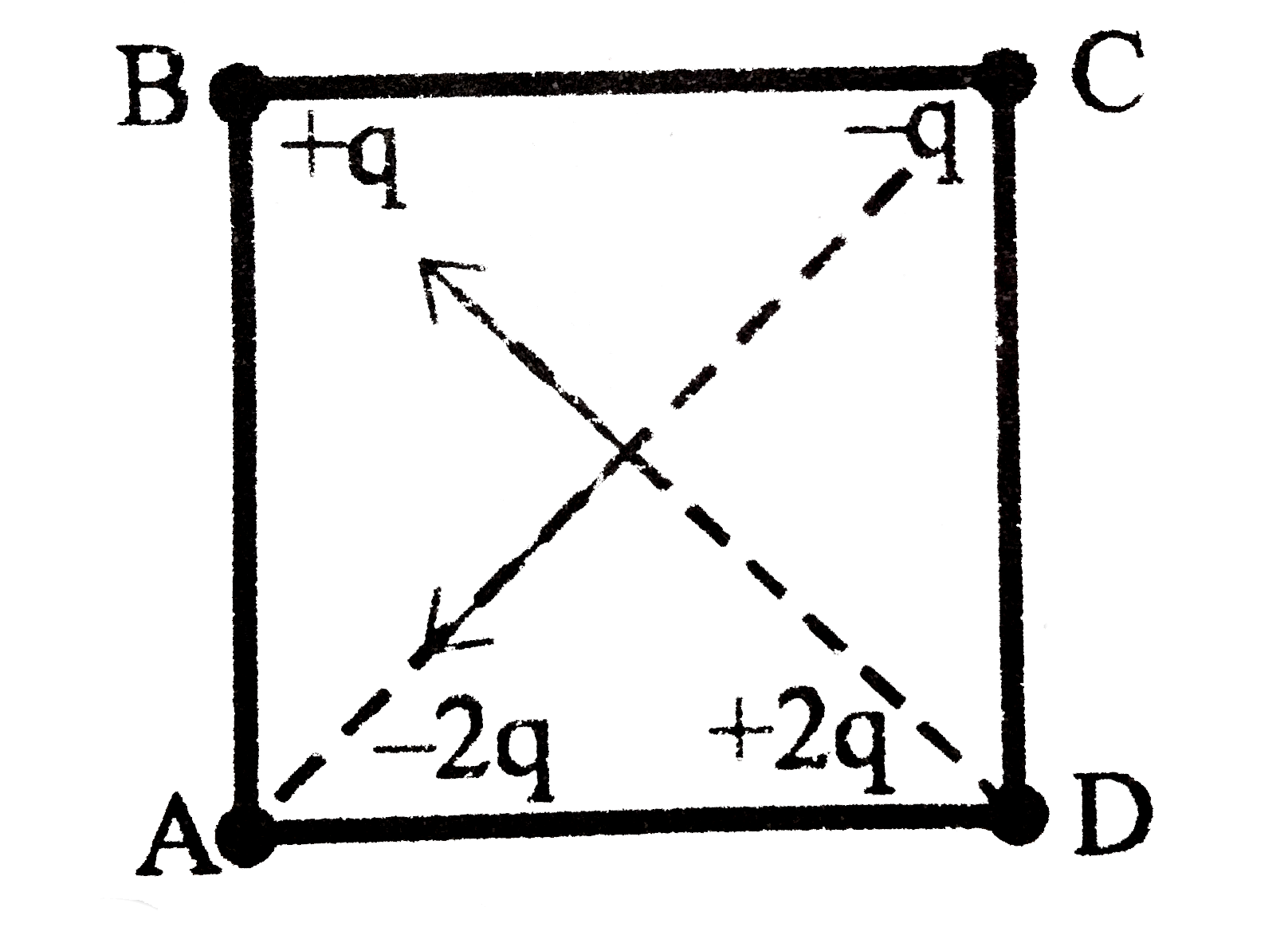AZeroBalong diagonal ACCalong diagonal BDDperpendicular to the side AB - Question 3 - Select One
A charges Q is placed at each of the two opposite corners of a square. A charge q is placed to each of the other two corners. If the resultant force on each charge q is zero, then
Aq=√2QBq=−√2QCq=2√2QDq=−2√2Q
Four point charges of +1μc each are placed on the four corners of a square of side 1 m. Calculate the electric potential at the centre of the square.
View SolutionFour charges +Q,−Q,+Q,−Q are placed at the corners of a square taken in order. At the centre of the square
View SolutionFour charges equal to −Q are placed at the four corners of a square and a charge q is at its centre. If the system is in equilibrium the value of q is
View SolutionFour charges equal to −Q are placed at the four corners of a square and a charge q is at its centre, If the system is in equilibrium the value of q si
View SolutionFour point charge are placed at the corners of a square with diagonal 2a as shown. What is the total electric field at the center of the squre?
View Solution