Two identical conducting spheres, fixed in space, attract each other with an electrostatic force of 0.108N when separated by 50.0cm, centre-to-centre. A thin conducting wire then connects the spheres. When the wire is removed, the spheres repel each other with an electrostatic force of 0.0360N. What were the initial charges on the spheres?
The correct Answer is:A, C
To solve the problem, we will follow these steps:
Step 1: Understand the initial conditions
We have two identical conducting spheres with charges Q1 and Q2. They attract each other with a force of 0.108N when separated by a distance of 50.0cm (or 0.5m).
Step 2: Use Coulomb's Law for attraction
According to Coulomb's Law, the force between two charges is given by:
F=k|Q1Q2|r2
where k=8.99×109N m2/C2 and r=0.5m.
Substituting the values, we have:
0.108=8.99×109|Q1Q2|(0.5)2
0.108=8.99×109|Q1Q2|0.25
0.108=35.96×109|Q1Q2|
Step 3: Solve for |Q1Q2|
Rearranging gives:
|Q1Q2|=0.10835.96×109=3×10−12C2
This is our Equation 1.
Step 4: Connect the spheres with a wire
When a thin conducting wire connects the spheres, charges redistribute until both spheres have the same charge Q. The total charge is conserved:
Q1+Q2=2Q
Thus, we can express Q as:
Q=Q1+Q22
Step 5: Use Coulomb's Law for repulsion
After the wire is removed, the spheres repel each other with a force of 0.0360N. The repulsive force is given by:
Fr=kQ2r2
Substituting the values:
0.0360=8.99×109Q2(0.5)2
0.0360=35.96×109Q2
Step 6: Solve for Q2
Rearranging gives:
Q2=0.036035.96×109=1×10−12C2
This is our Equation 2.
Step 7: Relate Equations 1 and 2
From Equation 1:
|Q1Q2|=3×10−12
From Equation 2:
Q2=1×10−12
Since Q=Q1+Q22, we can express Q1+Q2 as:
Q1+Q2=2Q=2×√1×10−12=2×10−6C
Step 8: Set up the equations
Now we have two equations:
1. Q1+Q2=2×10−6
2. |Q1Q2|=3×10−12
Step 9: Substitute Q2 in terms of Q1
From the first equation:
Q2=2×10−6−Q1
Substituting into the second equation:
Q1(2×10−6−Q1)=3×10−12
Expanding gives:
2×10−6Q1−Q21=3×10−12
Rearranging gives a quadratic equation:
Q21−2×10−6Q1+3×10−12=0
Step 10: Solve the quadratic equation
Using the quadratic formula Q1=−b±√b2−4ac2a:
Q1=2×10−6±√(2×10−6)2−4×1×3×10−122
Calculating the discriminant:
=2×10−6±√4×10−12−12×10−122=2×10−6±√−8×10−122
This indicates two possible values for Q1.
Step 11: Find Q2
Using the values of Q1, we can find Q2 using:
Q2=2×10−6−Q1
Final Answer
The initial charges on the spheres are:
- Q1=3μC
- Q2=−1μC
We have two identical conducting spheres with charges Q1 and Q2. They attract each other with a force of 0.108N when separated by a distance of 50.0cm (or 0.5m).
According to Coulomb's Law, the force between two charges is given by:
F=k|Q1Q2|r2
where k=8.99×109N m2/C2 and r=0.5m.
0.108=8.99×109|Q1Q2|(0.5)2
0.108=8.99×109|Q1Q2|0.25
0.108=35.96×109|Q1Q2|
Rearranging gives:
|Q1Q2|=0.10835.96×109=3×10−12C2
This is our Equation 1.
When a thin conducting wire connects the spheres, charges redistribute until both spheres have the same charge Q. The total charge is conserved:
Q1+Q2=2Q
Thus, we can express Q as:
Q=Q1+Q22
After the wire is removed, the spheres repel each other with a force of 0.0360N. The repulsive force is given by:
Fr=kQ2r2
Substituting the values:
0.0360=8.99×109Q2(0.5)2
0.0360=35.96×109Q2
Rearranging gives:
Q2=0.036035.96×109=1×10−12C2
This is our Equation 2.
From Equation 1:
|Q1Q2|=3×10−12
From Equation 2:
Q2=1×10−12
Since Q=Q1+Q22, we can express Q1+Q2 as:
Q1+Q2=2Q=2×√1×10−12=2×10−6C
Now we have two equations:
1. Q1+Q2=2×10−6
2. |Q1Q2|=3×10−12
From the first equation:
Q2=2×10−6−Q1
Substituting into the second equation:
Q1(2×10−6−Q1)=3×10−12
Expanding gives:
2×10−6Q1−Q21=3×10−12
Rearranging gives a quadratic equation:
Q21−2×10−6Q1+3×10−12=0
Using the quadratic formula Q1=−b±√b2−4ac2a:
Q1=2×10−6±√(2×10−6)2−4×1×3×10−122
Calculating the discriminant:
=2×10−6±√4×10−12−12×10−122=2×10−6±√−8×10−122
This indicates two possible values for Q1.
Using the values of Q1, we can find Q2 using:
Q2=2×10−6−Q1
The initial charges on the spheres are:
- Q1=3μC
- Q2=−1μC
Topper's Solved these Questions
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Level 1 Subjective
Explore 15 Videos
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:SUBJECTIVE_TYPE
Explore 6 Videos
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Level 1 Assertion And Reason
Explore 19 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBook:DC PANDEYChapter:ELECTROSTATIC POTENTIAL AND CAPACITORSExercise:(C) Chapter exercises
Explore 50 Videos
- GRAVITATIONBook:DC PANDEYChapter:GRAVITATIONExercise:All Questions
Explore 120 Videos
Similar Questions
Two identical conducting spheres, fixed in place, attract each other with an electrostatic force of 0.108 N when their center-to-center separation is 50.0 cm. The spheres are then connected by a thin conducting wire. When the wire is removed, the spheres repel each other with an electrostatic force of 0.144 N. Of the initial charges on the spheres, with a positive net charge, what was (a) the negative charge on one of them and (b) the positive charge on the other ?
View SolutionTwo identical particles are charged and held at a distance of 1m form each pther. They are found to be attraction each other with a force of 0.027N . Now they are connected by a conducting wire so that charge folws between them. Whe the charge flow stop, they are found to be repelling each other with a force of 0.009N. Find the initial charge on each particle.
View Solution
Knowledge Check
Two identical conducting sphere carrying different charges attact each other with a force F when placed in air medium at a distance d apart. The spheres are brought into contact and then taken to their original positions. Now, the two sphere repel each other with a force whole magnitude is equal to the initial attractive force. The ratio between initial charges on the spheres is
A−(3+√8) onlyB−3+√8 onlyC−(3+√8) or (−3+√8)D+√3Two identical conducting sphere carrying different charges attract each other with a force F when placed in air medium at a distance d apart. The spheres are brought into contact and then taken to their original positions. Now, the two sphere repel each other with a force whole magnitude is equal to the initial attractive force. The ratio between initial charges on the spheres is
A−(3+√8) onlyB−3+√8 onlyC−(3+√8) or (−3+√8)D−√8The centres of two identical small conducting spheres are 1m apart. They carry charge of opposite kind and attract each other with a force F. When they are connected by a conducting thin wire they repel each other with a force F/3. What is the ratio of magnitude of charge carried by the sphere initially ?
A1:1B2:1C3:1D4:1
Two identical conducting spheres 1 and 2 carry equal amounts of charge and are ficed apart at a certain distance larger than their diameters. The spheres repel each other with an electrical force of 88mN. A thired identical sphere 3, having an insulating handle and initially uncharged, is touched first to sphere 1 and then to sphere 2 and finally removed. Find the force between spheres 1 and 2 as shown if Figure.
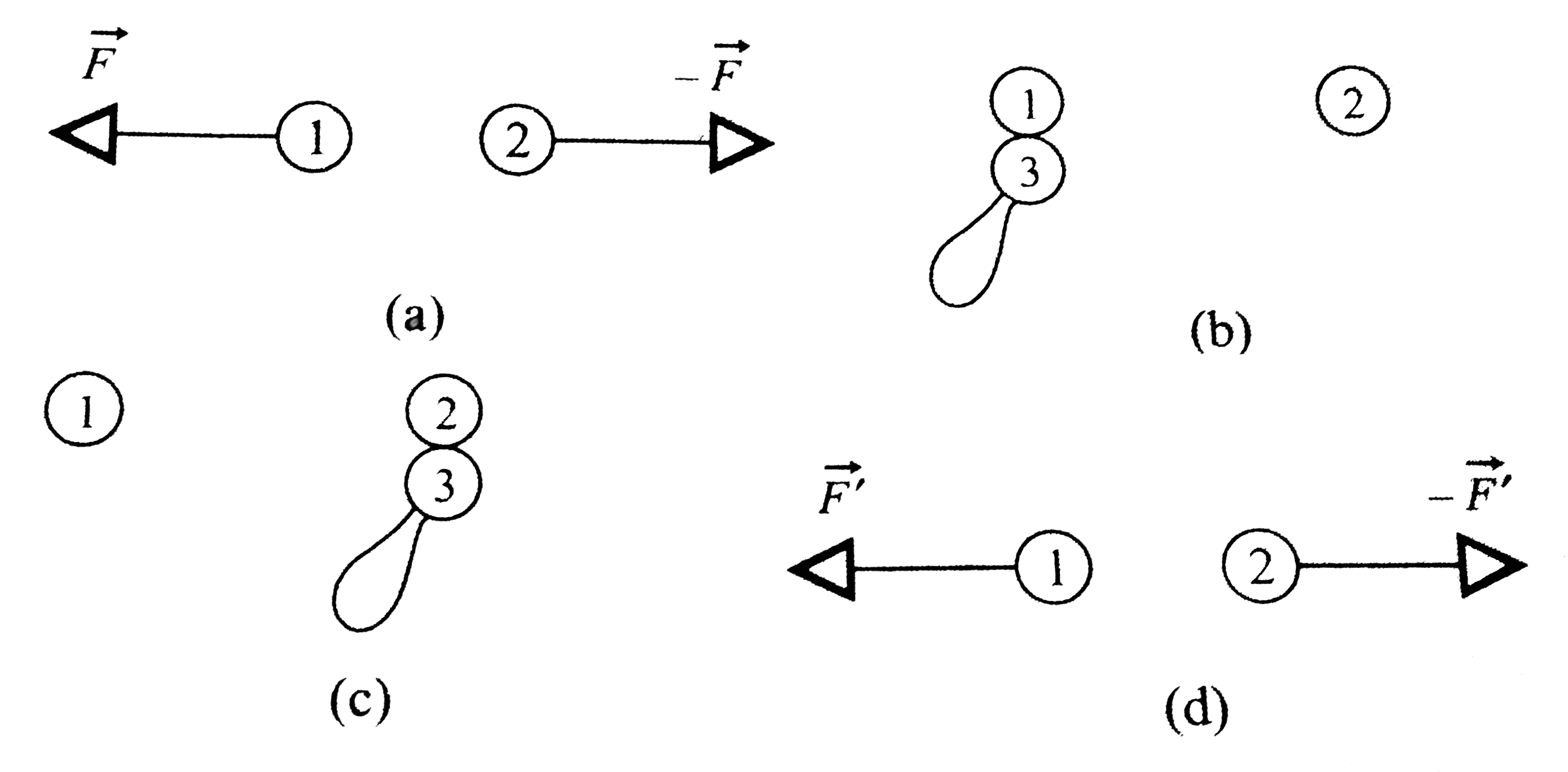 ,
,
View SolutionTwo small conducting spheres have charges 2.1nC and −0.1nCare touched to each other and then separated by a distance of 0.5 m. Find the force between them
View SolutionTwo identical metal spheres A and B have equal and similar charges. They repel each other with a force 103N, when they are placed 10cm apart in a medium of dielectric constant 7. Determine the charge on each sphere.
View SolutionThe centres of two identical small conducting sphere are 1 m apart. They carry charge of opposite kind and attract each other with a force F. when they connected by conducting thin wire they repel each other with a force F/3. The ratio of magnitude of charges carried by the spheres initially in n:1. Find value of n
View SolutionTwo metallic spheres of radii a and b are placed faraway from each other and are connected for a thin conducting wire. A charge Q is given to one of the spheres . Calculate the charge on each sphere at electrostatic equilibrium.
View Solution