A point charge –q revolves around a fixed charge +Q in elliptical orbit. The minimum ar.d maximum distance of q from Q are r1 and r2, respectively. The mass of revolving particle is Q>q and assume no gravitational effects. Find the velocity of q at positions when it is at r1 and r2 distance from Q.
The correct Answer is:A, B
From conservation of mechanical energy and conservation of angular mometum asbout point O, we have
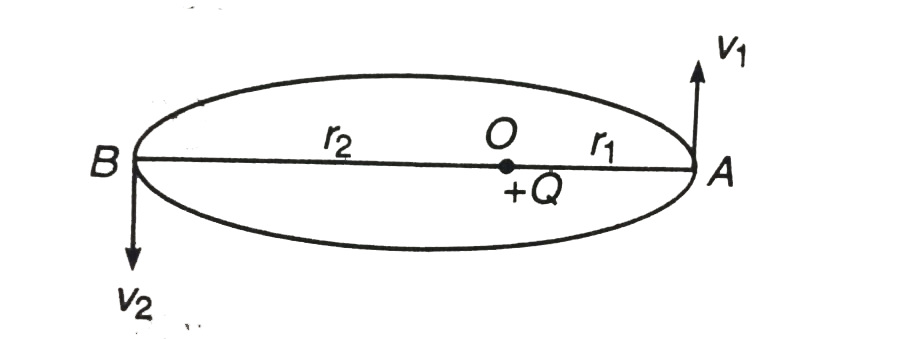
12mv21−14πε0.Qqr1=12mv22−14πε0.Q.qr2………i
and mv1r1sin90∘=mv2r2sin90∘
or v1r1=v2r2
Solving these two equations we have
v1=√Qqr22πε0mr1(r1+r2) and =√Qqr22πε0mr2(r1+r2)=v2
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.1
4 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.2
9 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 8
3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
A particle of mass m having negative charge q moves along an ellipse around a fixed positive charge Q so that its maximum and minimum distances from fixed charge are equal to r1 and r2 respectively. Calculate angular momentum L of this particle:
View Solution" Electric field intensity due to a point charge " "q" ' at a distance 'r' from it is "
View Solution
Knowledge Check
Two point charges q1 and q2 are released from rest in a gravity free hall when distance between them is a. Find the maximum speeds of charged particle. The mass of each charged particle is m.
Aq1q24πε0aB√(q1q2)4πε0ma)C√(2q1q24πε0ma)Dnone of theseCharge q2 of mass m revolves around a stationary charge q1 in a circulare orbit of radius r. The orbital periodic time of q2 would be
A[4π2mr3kq1q2]1/2B[kq1q24π2mr3]1/2C[4π2mr4kq1q2]1/2D[4π2mr2kq1q2]1/2Two particles p and q located at distances rp and rq respectively from the centre of a rotating disc such that rp>rq.
Aboth p and q have the same accelerationBboth p and q do not have any accelerationCp has greater acceleration then qDq has greater acceleration than p
A particle of mass m carrying charge '+q1' is revolving around a fixed charge '−q2' in a circular path of radius r. Calculate the period of revolution.
View SolutionFind out potential energy of the two point charge system having charges q1 and q2 separated by distance r.
View SolutionA particle of charge q1 and mass m is revolving around a fixed negative charge of magnitude q2 in a circular path of radius r. Find the time period of revolution.
View SolutionA particle of mass m carrying a charge −q1 starts moving around a fixed charge +q2 along a circulare path of radius r. Find the time period of revolution T of charge −q1.
View SolutionOn ortating a point charge having a charge q around a chrage Q in a circla of radius r. The work done will be
View Solution