One side of radius of curvature R2=120cm of a convexo-convex lens of material of refractive index μ=1.5 and focal length f1=40cm is slivered. It is placed on a horizontal surface with silvered surface in contact with it. Another convex lens of focal length f2=20cm is fixed coaxially d=10cm above the first lens. A luminous point object O on the axis gives rise to an image coincide with it. Find its height above the upper lens.
The correct Answer is:A, C
140=(1.5−1)(1120+1R1)
Solving we get, R2=24cm
Applying lens formula, for L2

1v1+1x=120……(i)
Using μ2v−μ1u=μ2−μ1R for unsilvered side of L1.
1.5−120−1.0v1−10=1.5−1.024...(ii)
Solving Eqs.(i) and (ii), we get x=10cm
Topper's Solved these Questions
Similar Questions
The radii of curvature of a lens are +20cm and +30cm .The material of the lens has a refractive index 1.6 .Then focal length of the lens (in cm) if it is placed in water of refractive index μ=(4)/(3) is
View SolutionA convex lens of focal length 40cm is held at a distance 12cm coaxially above a concave mirror of focale length 18cm.
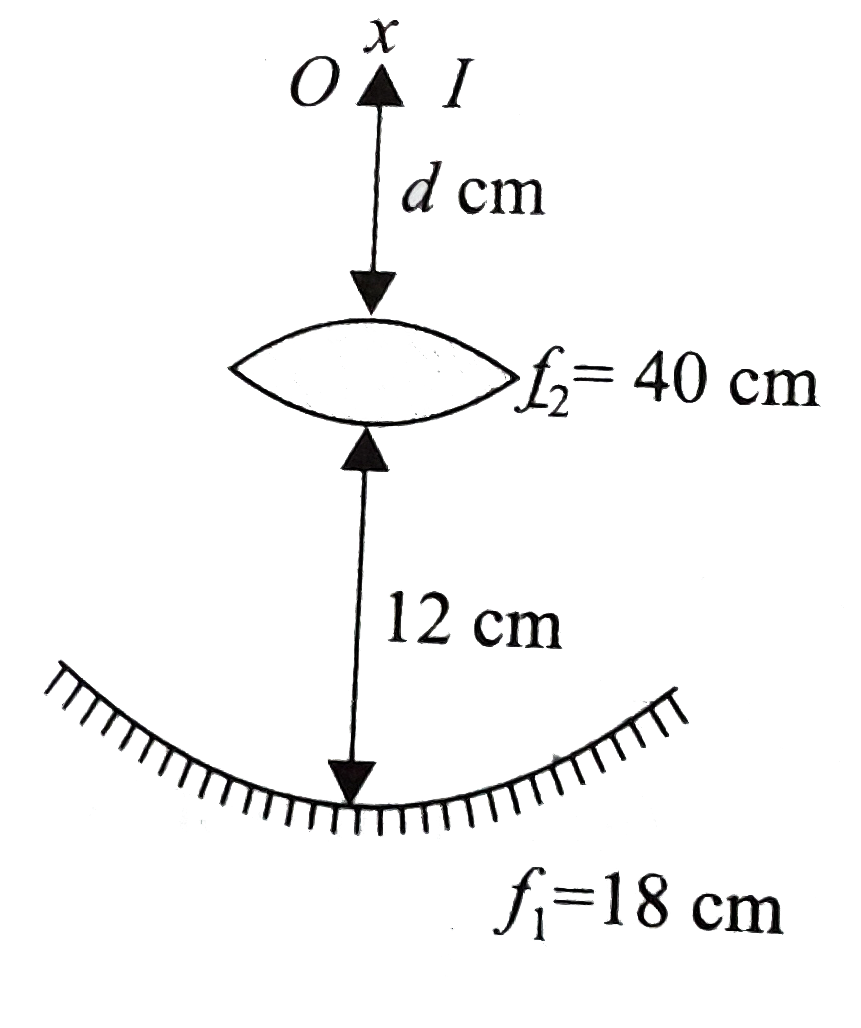
Q. A luminous point object placed d cm above the lens on its axis gives rise to an imag coincident iwth itself, then the value of d is equal to
View Solution
Knowledge Check
The radius of curvature for a convex lens is 40 cm , for each surface. Its refractive index is 1.5. The focal length will be
A40 cmB20 cmC80 cmD30 cmThe radius of curvature of each surface of a lens is 40 cm. Its refractive index is 1.5. The focal length of lens will be
A40 cmB20 cmC80 cmD30 cmAn eye specialist prescribes spectacles having combination of convex lens of focal length 40 cm in contact with a concave lens of focal length 25 cm. The power of this lens combination in diopters is
A+1.5DB−1.5DC+6.67DD−6.67D
The radius of curvature of curved surface of a thin plano-convex lens is 10cm and the refractive index is 1.5. If the plano surface is silvered, then the focal length will be.
View SolutionA planocovex lens is made of a material of refractive in μ=1.5 The radius of curvature of curved surface of the lens is 20.cm. If its plane surface3 is silvered, the focal length of the silvered lens will be
View SolutionFind the focal length of plano-convex lens of material having refractive index of 1.5. Radius of curvature of convex surface is 10 cm.

View SolutionThe radius of curvature of the face of plano-convex lens is 12cm and its refractive index is 1.5. If the plane surface of the lens is now silvered, then find the focal length of the lens.
View SolutionA convexo-concave convergent lens is made of glass of refractive index 1.5 and focal length 24cm. Radius of curvature for one surface is double than that of the other. Then,radii of curvature for the two surfaces are (in cm)
View Solution
