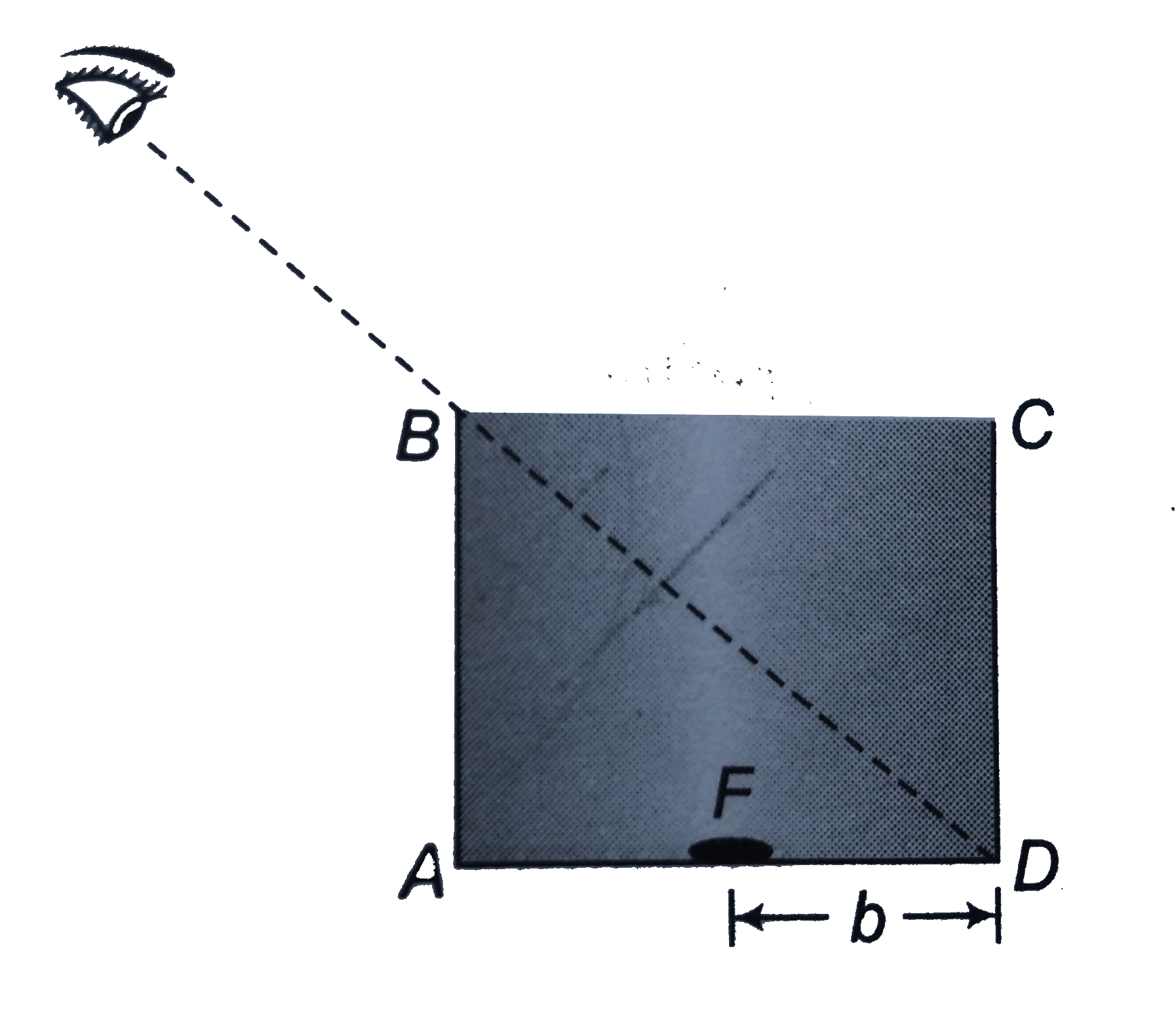
A cubical vessel with non-transparent walls is so located that the eye of an observer does not see its bottom but sees all of the wall CD.
To what height should water be poured into the vessel for the observer to see an object F arranged at a distance of b=10cm from corner D? The face of the vessel is a=40cm. Refractive index of water is 43.
The correct Answer is:B, C
Since, the vessel is cubical, ∠GDE=45∘
and GE=ED=h(say)
then EF=ED−FD=(h−10)
Further, 43=sin45∘sinr
∴r=32∘
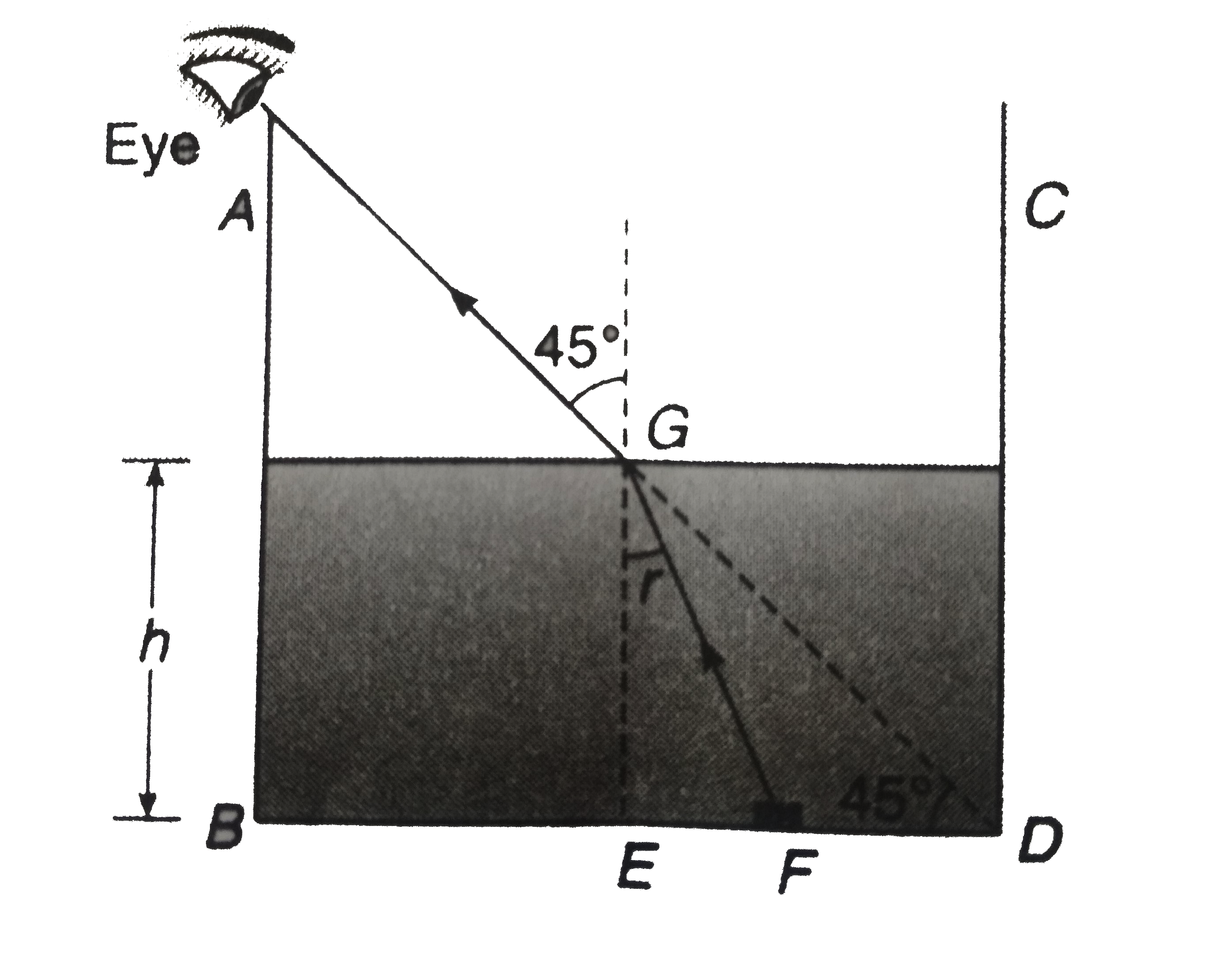
Now, EFGE=tanr=tan32∘
∴h−10h=0.62
Solving this, we get
h=26.65cm
Topper's Solved these Questions
Similar Questions
Knowledge Check
Ability of the eye to see objects at all distances is called
ABinocular visionBMyopiaCHypermetropiaDAccommodationA cubical vessel has opaque walls. An observer (dark circle in figure below) is located such that she can see only the wall CD but not the bottom. Nearly to what height should water be poured so that she can see an object placed at the bottom at a distance of 10 cm from the corner C ? Refractive index of water is 1.33.
 A10 cmB16 cmC27 cmD45 cm
A10 cmB16 cmC27 cmD45 cmTo what height h should a cylindrical vessel of diameter d be filled with a liquid so that the total force on the vertical surface of the vessel be equal to the force on the bottom-
Ah=dBh=2dCh=3dDh=d/2
If a person can sees clearly at a distance of 100 cm, then find the power of leris used to see object at 40 cm.
View SolutionThe depth of water in a bucket is 40 cm but its bottom appears to be raised by 10 cm. what is the refractive index of water ?
View SolutionA cubical vessel of height 1 m is full of water. What is the workdone in pumping water out of the vessel?
View SolutionA vessel whose bottom has round holes with diameter of 1 mm is filled with water Assuming that surface tension acts only at holes, then the maximum height to which the water can be filled in vessel without leakage is (given surface tension of water is 75×10−3N/m) and g=10m/s2
View SolutionA large vessel having a very small circular hole of diameter 1 mm at the bottom is filled with water. Up to what maximum height (in cm) water can be filled in the vessel so that there is no leakage? [surface tension of water 7.5×10−2Nm−1andg=10ms−2]
View Solution

