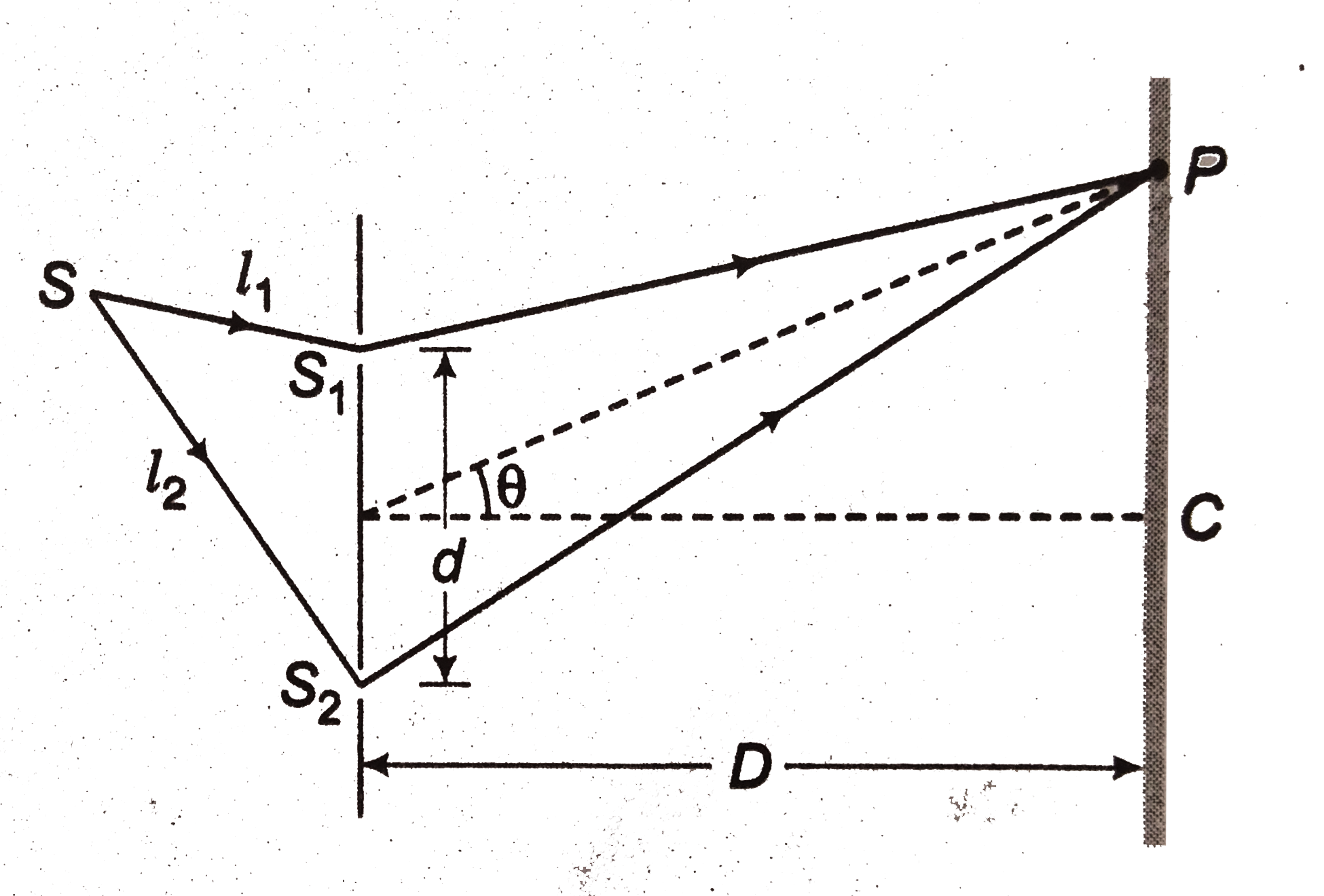
In a Young's double slit experiment, the light sources is at distance l1=20μm and l2=40μm form the main slit. The light of wavelength lambda = 500nm is incident on slits separated at a distance 10μm. A screen is placed at a distance D = 2 away from the slits as shown in figure. Find
(a) the values of θ relative to the central line where maxima appear on the screen?
(b) how many maxima will appear on the screen?
what should be the minimum thickness of a slab of refractive index 1.5 placed on the path of one of the ray so that minima occurs at C ?
 .
.
(a) The optical path difference between the beams arriving at P,
Δx=(l2−l1)+dsinθ
The condition for maximum intensity is [n=0,±1,±2………….]
Δx=nλ
Thus, sinθ=1d[Δx−(l2−l1)]=1d[nλ−(l2−l1)]
=110×(10−6)[n×500×10−9×20×10−6]
=2[(n40)−1]
Hence, θ=sin−1[2((n40)−1)]
(b)|sinθ|≤1
∴(−1≤2[(n40)−1]≤1)
or −20≤(n−40)≤20
or 20≤n≤60
Hence, number of maximas = 60-20 = 40
(c) At C, phase difference ϕ=2πλ(l2−l1)=(2π500×10−9)(20×10−6)
=80π
Hence, maximum intensity will appear at C. For minimum intensity at C,
(μ−1)t=λ2
or l t=λ2(μ−1)=500×10−92×0.5=500nm .
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.13videos
INTERFERENCE AND DIFFRACTION OF LIGHT
- BOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Exercise 32.26videos
INTERFERENCE AND DIFFRACTION OF LIGHT
- BOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Example Type 63videos
INTERFERENCE AND DIFFRACTION OF LIGHT
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions120videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery59videos
MAGNETIC FIELD AND FORCES
Similar Questions
In Young's double slit experiment the slits are separated by 0.24 mm. The screen is 2 m away from the slits . The fringe width is 0.3 cm. Calculate the wavelength of the light used in the experiment.
View SolutionIn Young's double-slit experiment, the slit separation is 0.5 mm and the screen is 0.5 m away from the slit. For a monochromatic light of wavelength 500 nm, the distance of 3rd maxima from the 2nd minima on the other side of central maxima is
View Solution
Knowledge Check
- Question 1 - Select One
In a Young's double slit experiment, the slit separation is 1mm and the screen is 1m from the slit. For a monochromatic light of wavelength 500nm, the distance of 3rd minima from the central maxima is
A(a) 0.50mmB(b) 1.25mmC(c) 1.50mmD(d) 1.75mm - Question 2 - Select One
In Young's double slit experiment, two slits are made 5 mm apart and the screen is palced 2m away . What is the fringe separation when light of wavelength 500 nm is used ?
A0.002 m mB0..02 m mC0 . 2 m mD2 m m - Question 3 - Select One
In a Young's double slit experiment two slits are separated by 2 mm and the screen is placed one meter away. When a light of wavelength 500 nm is used, the fringe separation will be:
A0.25 mmB0.50 mmC0.75 mmD1 mm
In a Young’s double slit experiment, the slit separation is 0.2cm, the distance between the screen and slit is 1 m . Wavelength of the light used is 5000Å. The distance between two consecutive dark fringes (in mm ) is
View SolutionIn the Young's double slit experiment, the spacing between two slits is 0.1mm. If the screen is kept at a distance of 1.0m from the slits and the wavelength of ligth is 5000Å, then the fringe width is
View SolutionIn a Young's double slit experiment, the fringe width will remain same, if ( D = distance between screen and plane of slits, d = separation between two slits and λ = wavelength of light used)
View SolutionLight of wavelength 5000 Å is incident on a double with and the interference fringes are formed on a screen plated at a distance of 1m from the slits. If the distance between the two consecutive dark bands is 1 mm, then the distance between the two slits is
View SolutionIn a Young's double-slit experiment, the slit separation is 0.2 mm and the distance between the screen and double-slit is 1.0 m. wavelength of light used is 5000 Å. The distance between two consecutive dark fringes is
View Solution

