A beam of light parallel to central line AB is incident on the plane of slits. The number of minimum, obtained on the large screen is . Now if the beam is tilted by some angle as shown in figure, then the number of minima obtained is . Then, 
will be zero.
The correct Answer is:A
Only fringe pattern will shift. Number of fringes
on screen will remain unchanged.
Topper's Solved these Questions
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 1Subjective
22 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Subjective Questions
5 Videos
- INTERFERENCE AND DIFFRACTION OF LIGHTBOOK - DC PANDEYCHAPTER - INTERFERENCE AND DIFFRACTION OF LIGHTEXERCISE - Level 1 Objective
11 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
Similar Questions
In the figure shown if a parallel beam of white light is incident on the plane of the slits then the distance of the white spot on the screen from O is [Assume <<D,λ<<d]
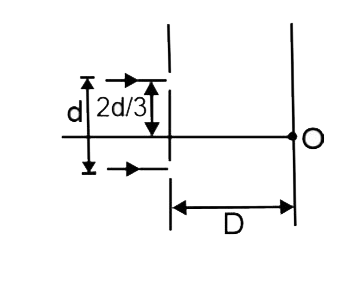
View SolutionIn figure, if a parallel beam of white light is incident on the plane of the slit, then the distance of the nearest white spot on the screen form O is [assume d<<D,λ<<D]
View Solution
Knowledge Check
In figure, if a parallel beam of white light is incident on the plane of the slit, then the distance of the nearest white spot on the screen form O is [assume d<<D,λ<<D]
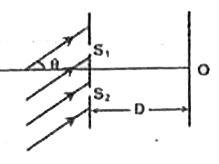
A0Bd/2Cd/3Dd/6In Young's double slit experiment parallel monochromatic light is incident on both the slits at an angle as shown in the figure. To obtain the central maxima at on the screen we can
Aplace a thin glass sheet of appropriate thickness in front of slit S1.Bplace a thin glass sheet of appropriate thickness in front of slits S2Csubmerge the whole experimental setup in appropriate liquid.Drotate the plane of slits by angle 6 anticlockwise
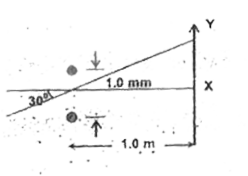
A coherent parallel beam of microwaves of wavelength A = 0.5 mm fails on a Young's double slit apparatus. The separation between maxima is measured on a screen placed paraiiei to the plane, of the slits at a distance of 1.0 m from it as shown in figtird. The separation between the slits is 2d - 1mm.
(a) If the incident beam fails normally on the double slit apparatus, find the y-coordinates of all the interface minima of the screen.
(b) If the incident beam makes an angle of 30° with the x-axis (as shown in fig. find the y-coordinates of the first minima on either side of the central maximum.
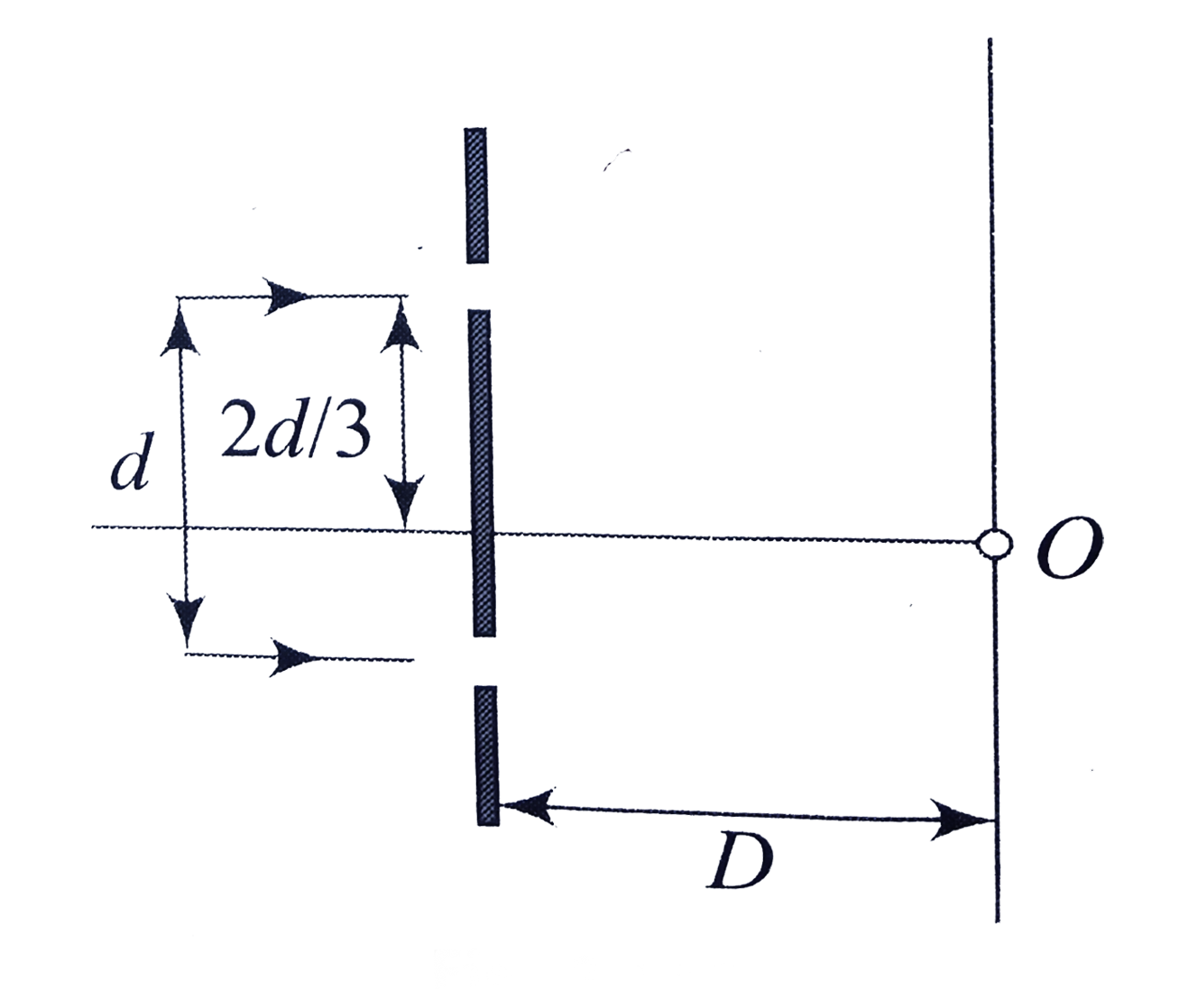
View SolutionIn the figure , if a parallel beam of white light is incident on the plane of the slits S1andS2 then the distance of the nearest white spot on the screen from O is [ Assume D>>d,d>>λ ]
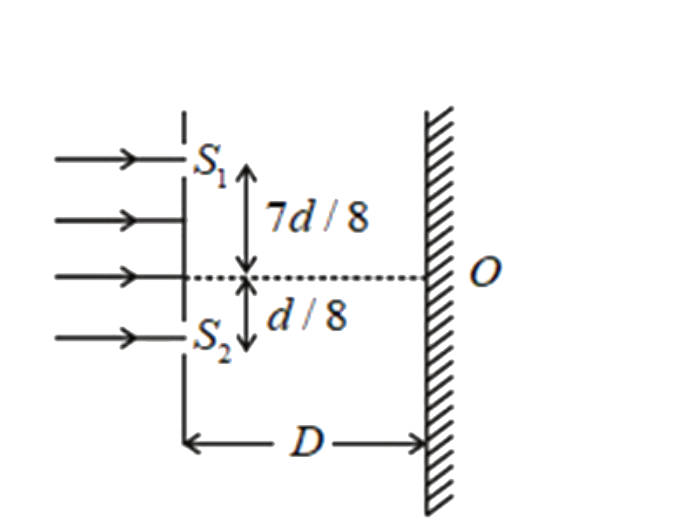
View SolutionIn the figure , if a parallel beam of white light is incident on the plane of the slits S1andS2 then the distance of the nearest white spot on the screen from O is [ Assume D>>d,d>>λ ]
View SolutionIn Young's double slit experiment parallel monochromatic light is incident on both the slits at an angle as shown in the figure. To obtain the central maxima at on the screen we can
View SolutionA coherent parallel beam of microwaves of wavelength λ=0.5mm falls on a Young's double- slit apparatus. The separation between the slits is 1.0 mm. The intensity of microwaves is measured on a screen placed parallel to the plane of the slits at a distance of 1.0 m from it as shown in Fig. 2.42.
If the incident beam makes an angle or 30∘ with the x-axis (as in the dotted arrow shown in the figure), find the y-coordinates of the first minima on either side of the central maximum.
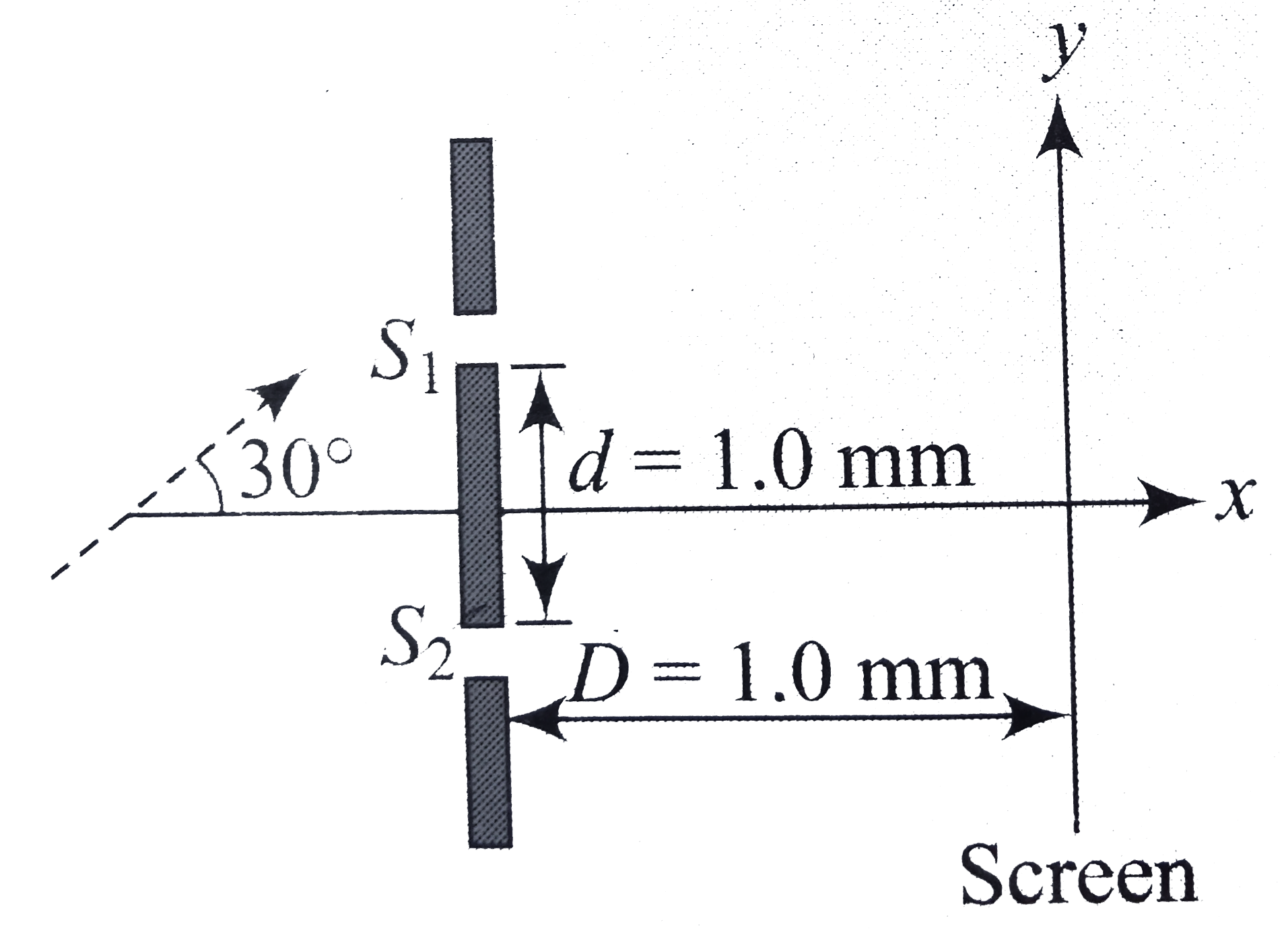
View SolutionA coherent parallel beam of microwaves of wavelength λ=0.5mm falls on aYoung's double- slit apparatus. The separation between the slits is 1.0 mm. The intensity of microwaves is measured on a screen placed parallel to the plane of the slits at a distance of 1.0 m from it as shown in Fig. 2.42.
If the incident beam makes an angle or 30∘ with the x-axis (as in the dotted arrow shown in the figure), find the y-coordinates of the first minima on either side of the central maximum.
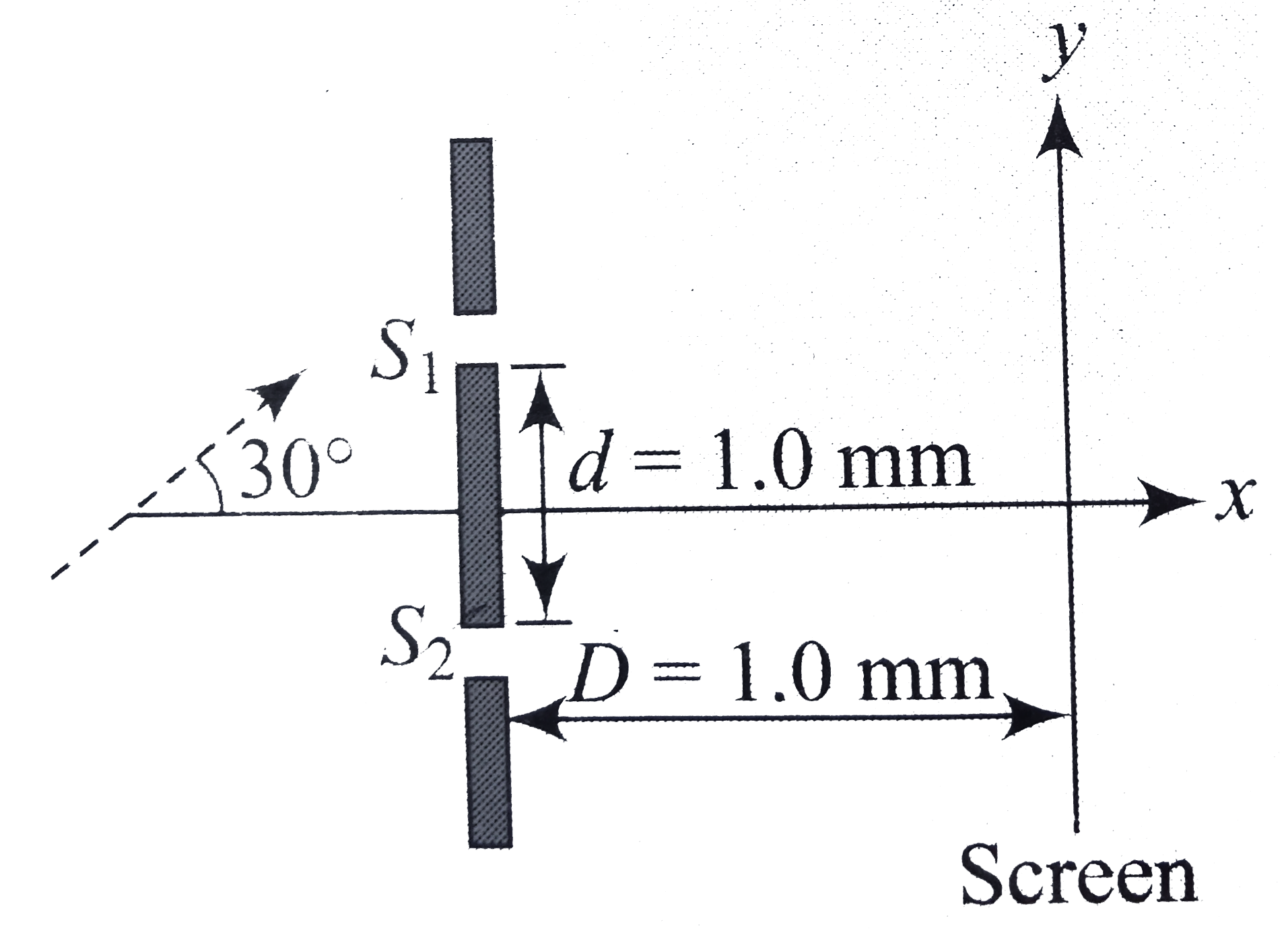
View Solution