If OABC is a tetrahedron such thatOA2+BC2=OB2+CA2=OC2+AB2 then
OA⊥BC
OB⊥AC
OC⊥AB
AB⊥AC
Let −−→OA=→a,−−→OB=→b,−−→OC=→c
Then from the given conditions.
→a.→a+(→b−→c).(→b−→c)=→b.→b+(→c−→a).(→c−→a)
⇒−2→b.→c=−2→c.→a
⇒→c.(→b−→a)=0
⇒ar(△PQR)=12×4×4=8
Similar Questions
यदि OABC एक चतुष्फलक इस प्रकार है कि OA2+BC2=OB2+CA2=OC2+AB2, तब
View SolutionO is any point in the exterior of a ΔABC. Prove that 2(OA+OB+OC)>(AB+BC+CA)
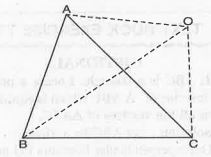 .
.
View SolutionIf 0 is a point in the exterior of an ABC, then show that OA+OB +OC gt (1)/2 (AB + BC + CA)
View SolutionThe point O is outside the ∠ABC. Show that 2(OA+OB+OC)>AB+BC+CA.
View SolutionA point O lies inside a ΔABC. Show that OA+OB+OC>12(AB+BC+CA).
View SolutionIn a tetrahedron OABC, the edges are of lengths, |OA|=|BC|=a,|OB|=|AC|=b,|OC|=|AB|=c. Let G1andG2 be the centroids of the triangle ABC and AOC such that OG1⊥BG2, then the value of a2+c2b2 is
View SolutionLet O be any point in the interior of ΔABC, prove that :
AB+BC+CA<2(OA+OB+OC)
View SolutionIn a tetrahedron OABC, the edges are of lengths, |OA|=|BC|=a,|OB|=|AC|=b,|OC|=|AB|=c. Let G_1 and G_2 be the centroids of the triangle ABC and AOC such that OG_1 _|_ BG_2, then the value of (a^2+c^2)/b^2 is
View Solution
