In the figure, seg DE∣∣ side AB. DC=2BD, A(ΔCDE)=20cm2. Find A(□ABDE).
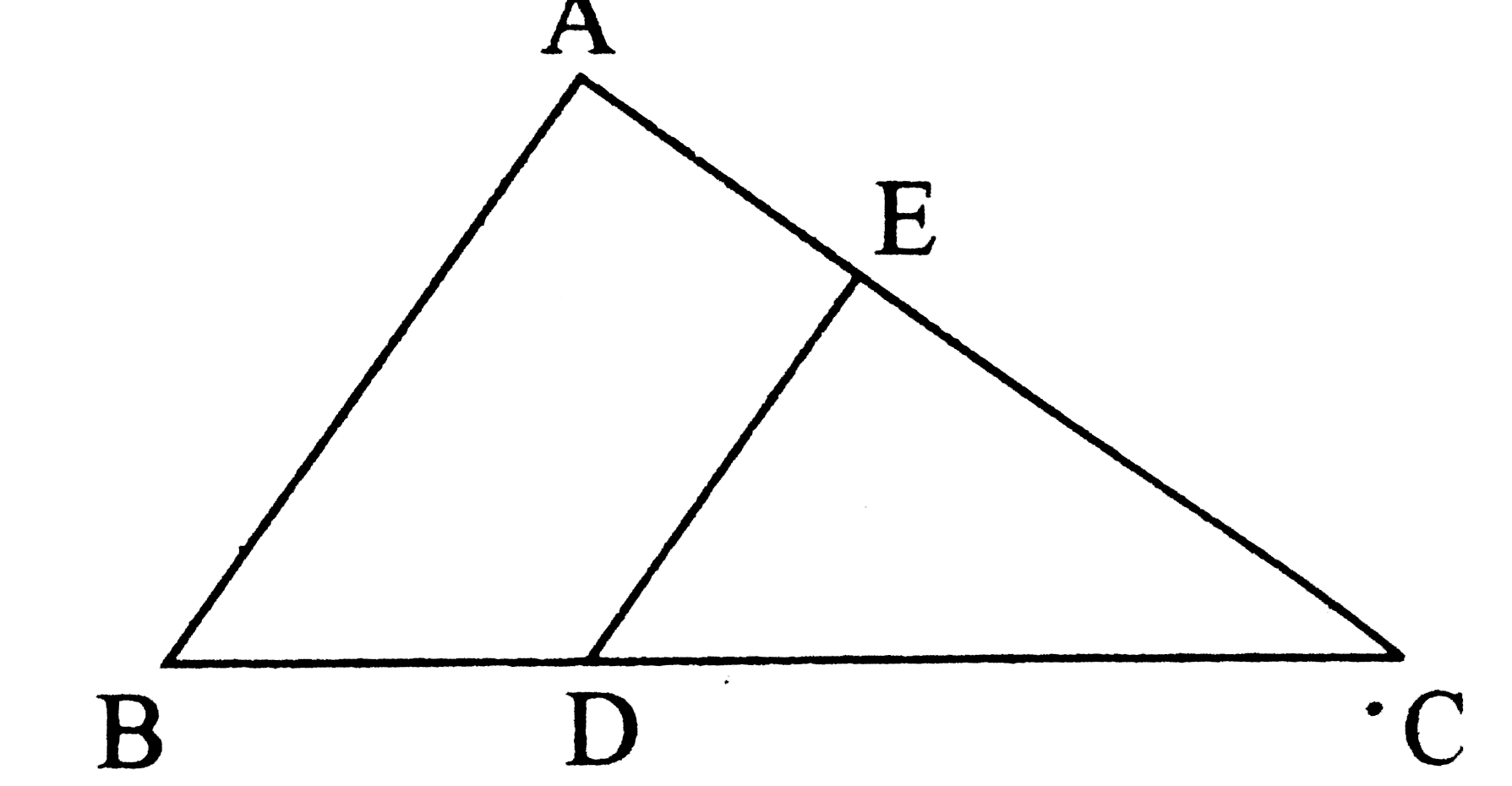
DC=2BD……(given)
Let BD be x. Then DC=2x
BC=BD+DC=x+2x=3x
Seg DE∣∣ side AB
CA is the transversal
∴∠CED≅∠CAB …..(Corresponding angles)
In ΔCED and ΔCAB,
∠ECD≅∠ACB……(Common angle)
∴ΔCED~ΔCAB......("AA" test for similarity)
By the theorem on areas of similar triangles,
A(ΔCED)A(ΔCAB)=CD2CB2=(CDCB)2=(2x3x)2=(23)2=49
∴20A(ΔCAB)=49 ∵A(ΔCAB)=20×94=45cm2
A(ΔCAB)=A(ΔCED)+A(□ABDE)........(Area addition postulate)
∴45=20+A(□ABDE)
∴A(□ABDE)=45−20
∴A(□ABDE)=25
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - EXAMPLE TYPE50videos
SIMILARITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - CHALLENGING QUESTIONS8videos
SIMILARITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - 2.4 (3 mark each)8videos
SIMILARITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATIONS EXERCISE - ASSIGNEMENT 2.48videos
QUADRATIC EQUATIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - EXAMPLES FOR PRACTICE (MCQs)35videos
STATISTICS
Similar Questions
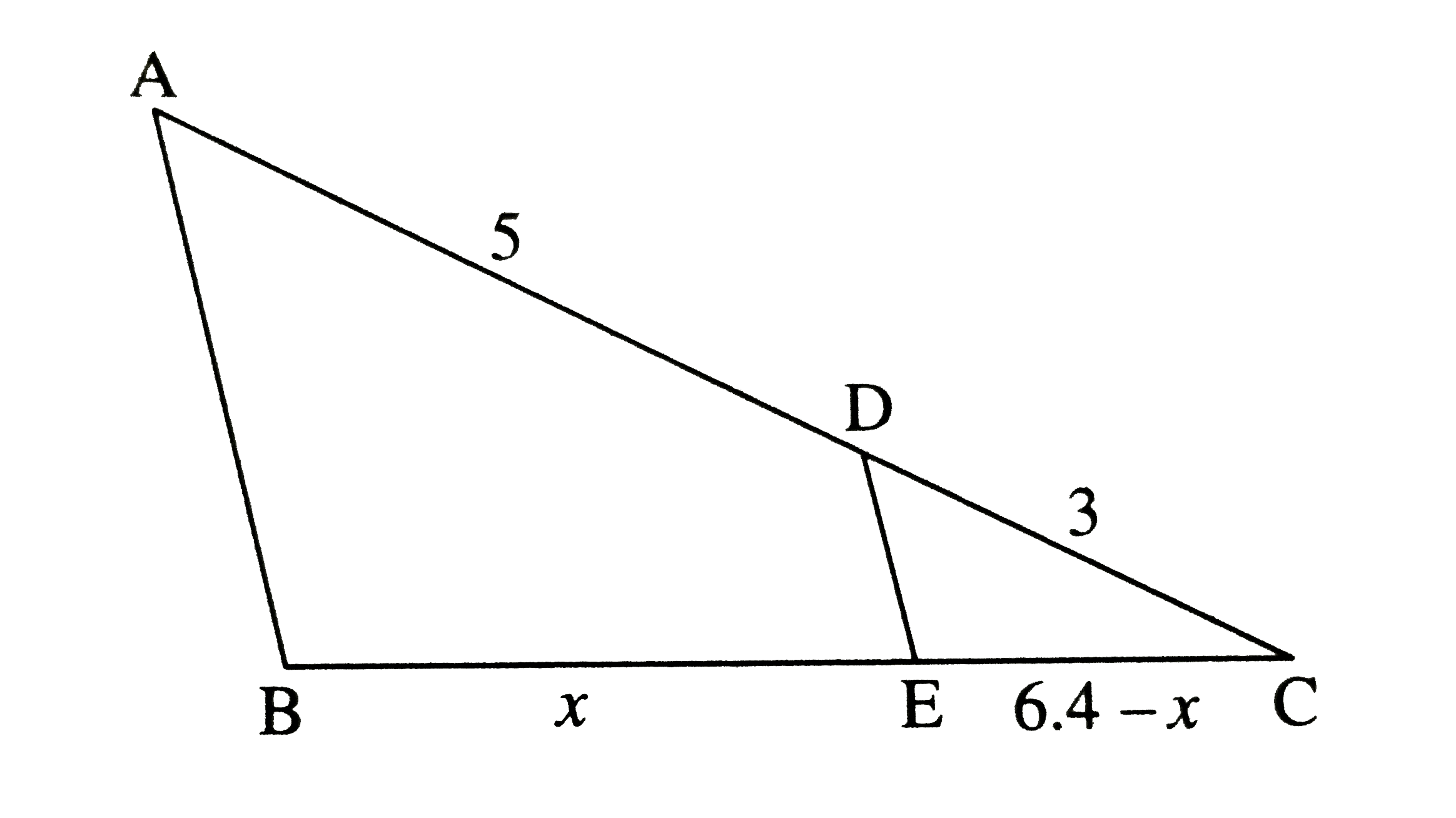
In the figure A−D−C and B−E−C seg DE∣∣ side AB. If AD=5,DC=3,BC=6.4 then find BE.
 View Solution
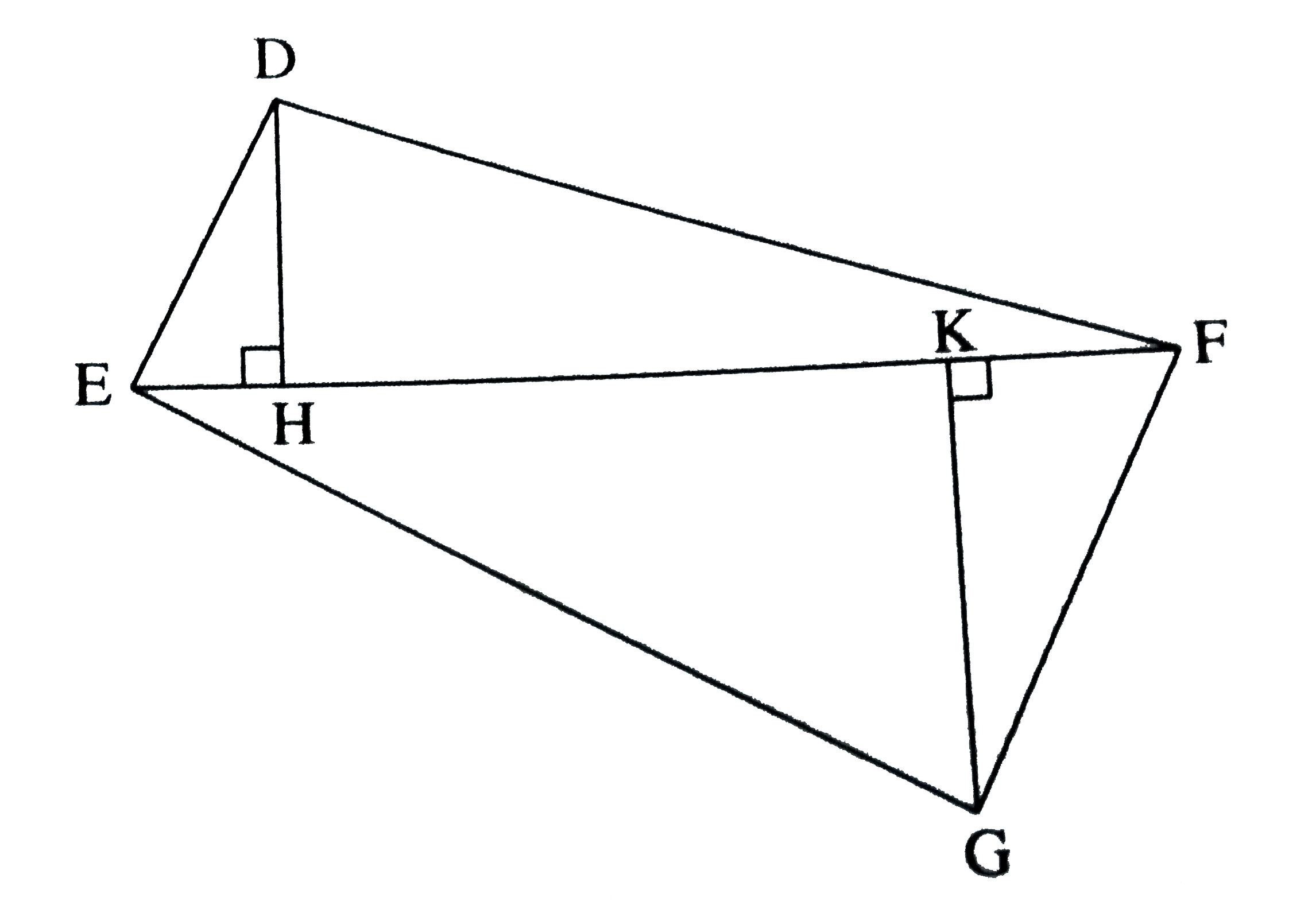
View SolutionIn the figure, set DH⊥ side EF and seg GK⊥ side EF. If DH=12cm,GK=20 and A(ΔDEF)=300cm2.
Find (i) EF(ii) A(ΔGEF) (iii) A(□DEGF).
 View Solution
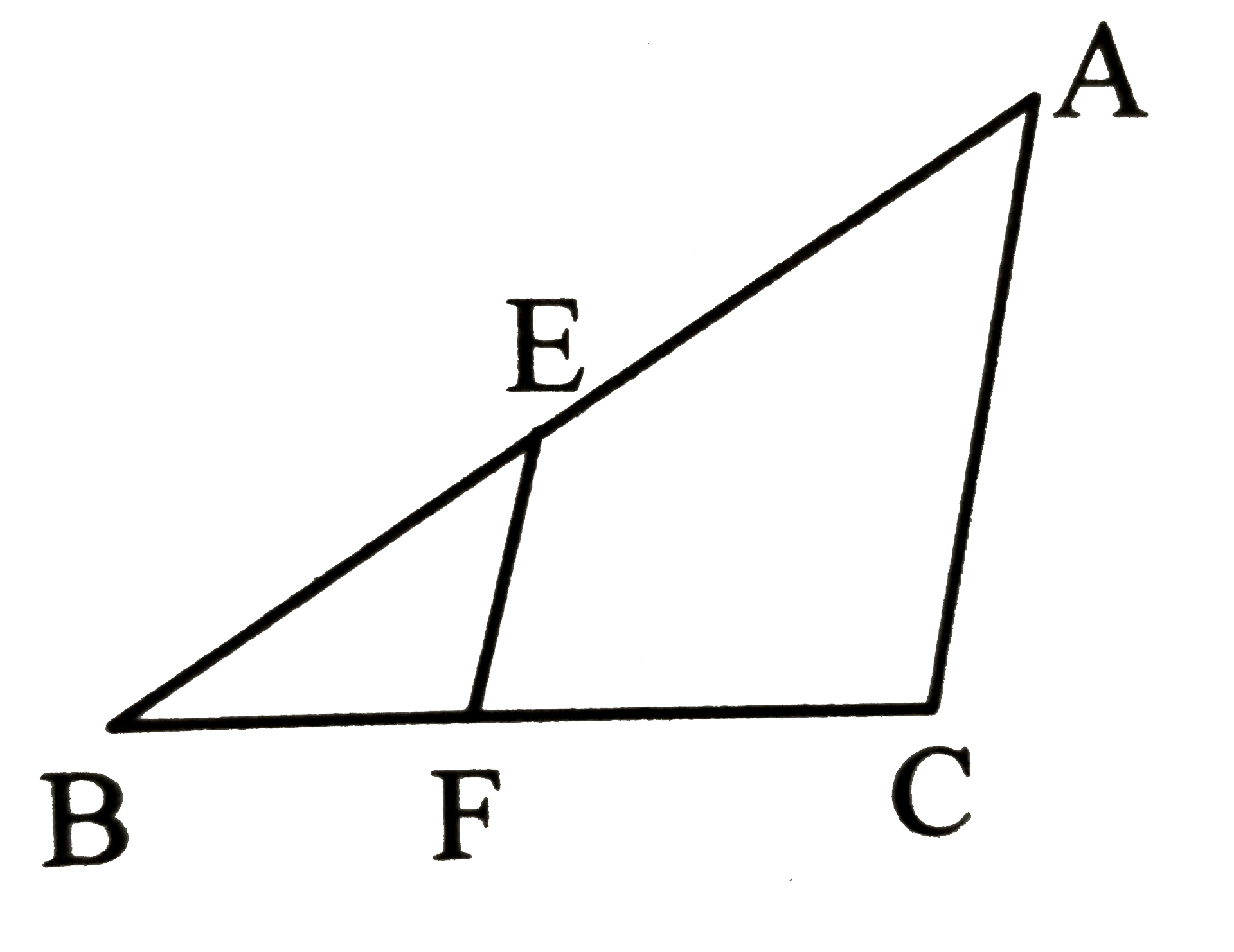
View SolutionIn the adjoining figure, seg EF|| side AC, AB = 18, AE = 10, BF = 4. Find BC.
 View Solution
View SolutionIn the adjoining figure, seg PQ||seg DE, A(ΔPQF)=20 sq. uints, PF = 2 DP, then find A (□DPQE) by completing the following activity.
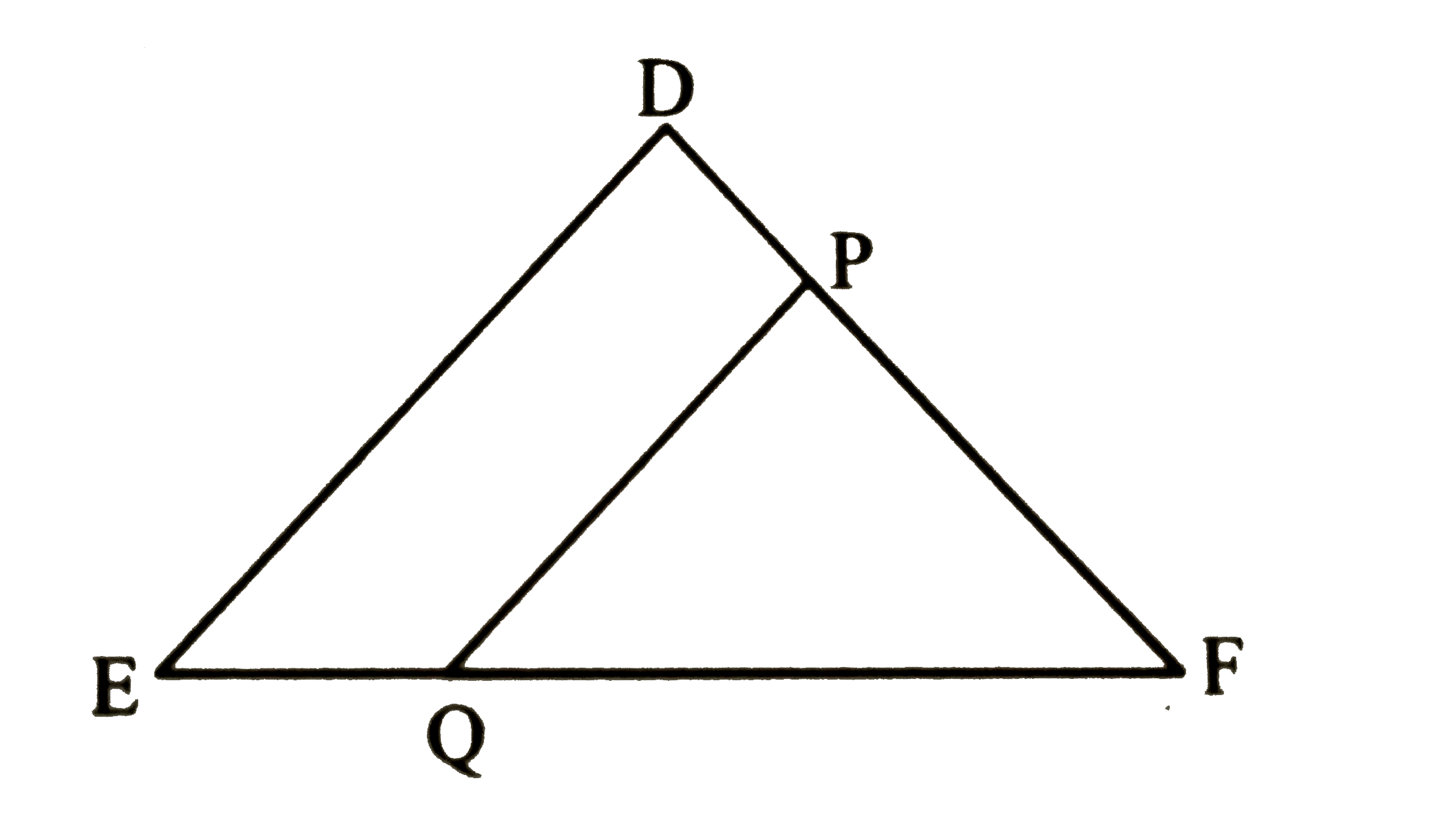 View Solution
View SolutionIn the figure, seg AB∣∣ seg CD∣∣ seg EF AC=1.5, BD=10, DF=6 then AE=?
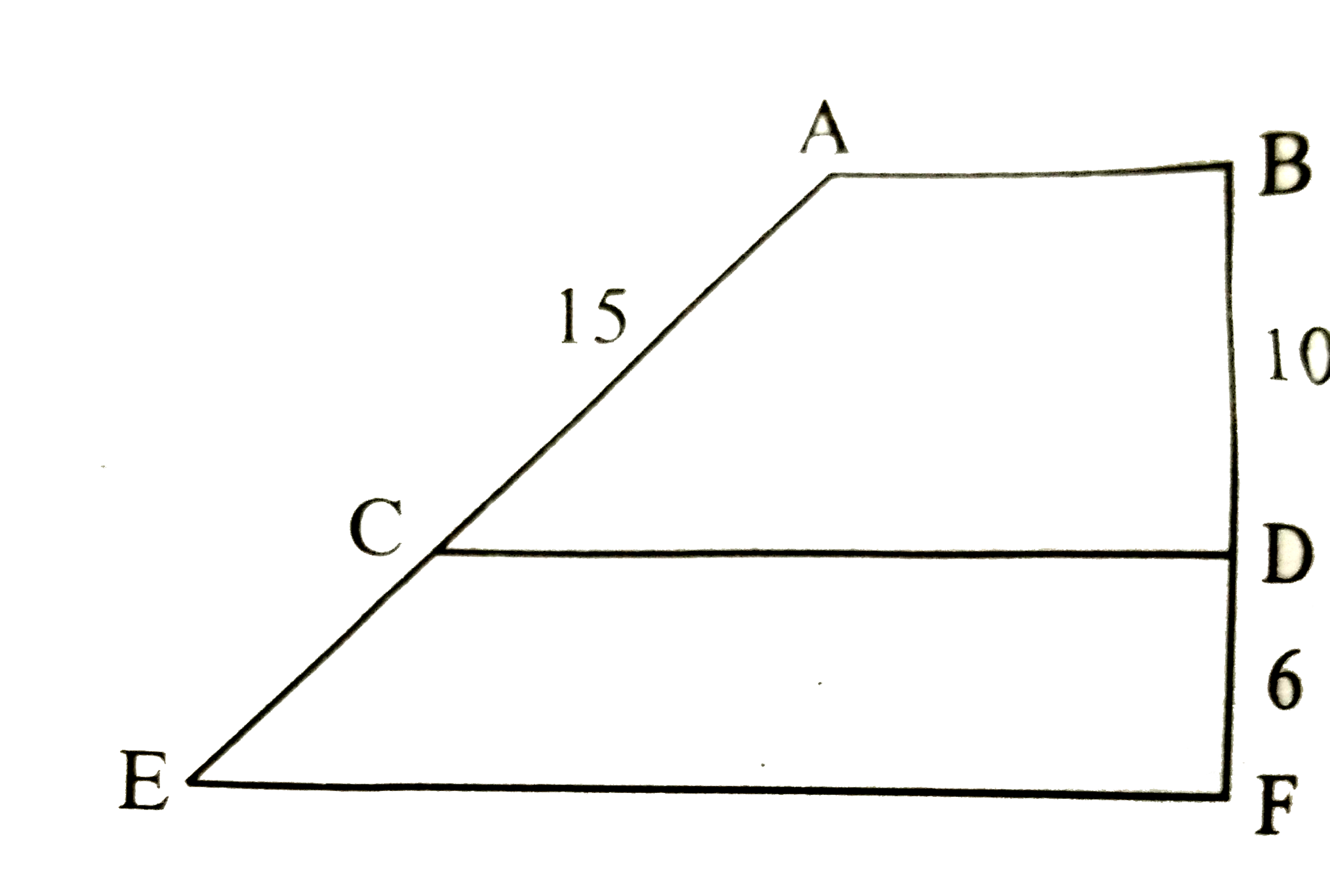 View Solution
View SolutionIn ΔABC, seg DE∣∣ side BC. If 2Ar(ΔADE)=Ar(□DBCE), find AB:AD and show BC=√3×DE.
View SolutionIn the given figure, DE|∣|BC,AD=2cm,BD=2.5cm,AE=3.2cmandDE=4cm. Find AC and BC.
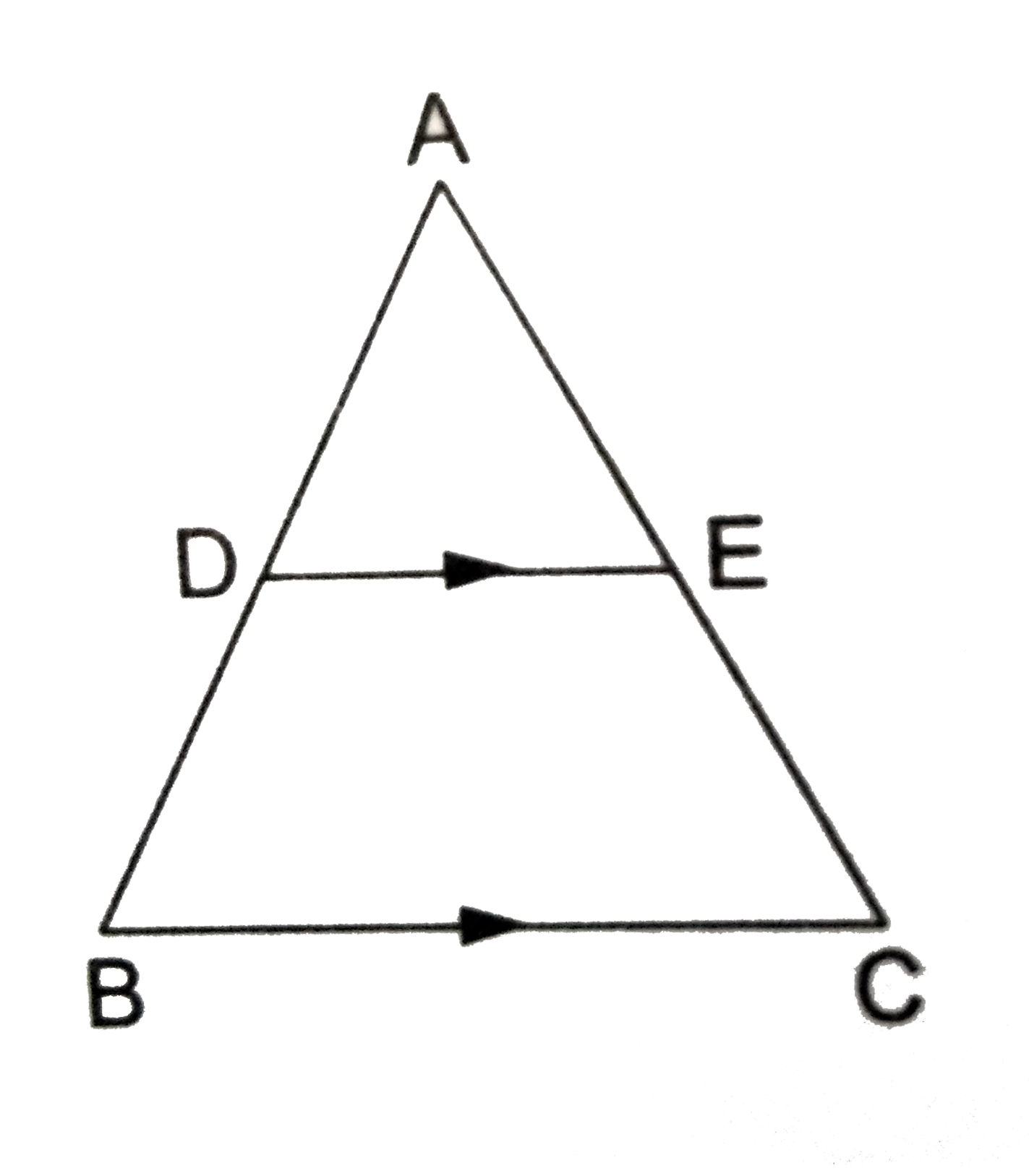 View Solution
View SolutionIn the figure, seg AD⊥ side BC and B−D−C, then prove AB2−BD2=AC2−CD2.
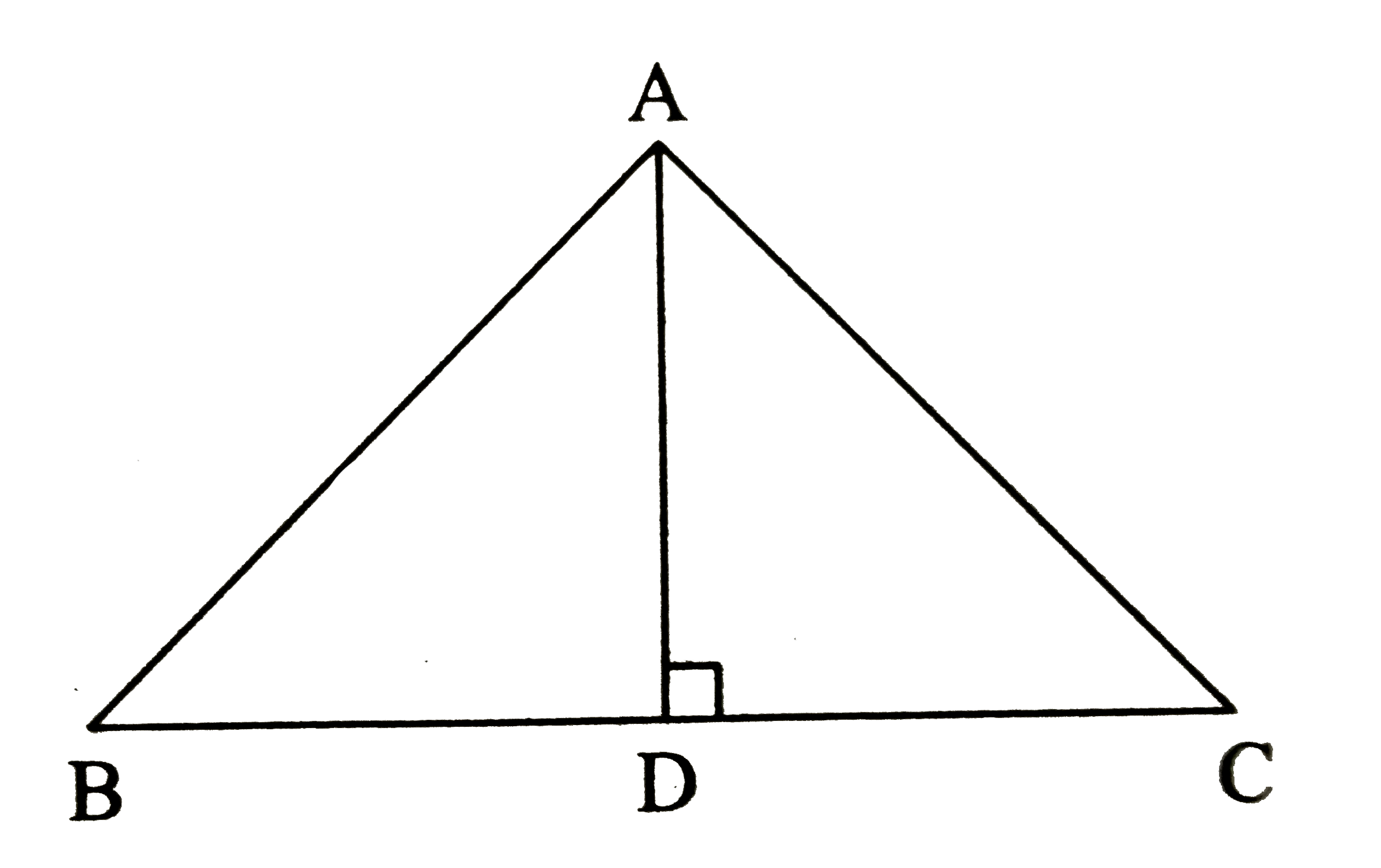 View Solution
View SolutionIn the adjoining figure,seg DE||side AC and seg DC ||side AP.
Prove that BEEC=BCCP.
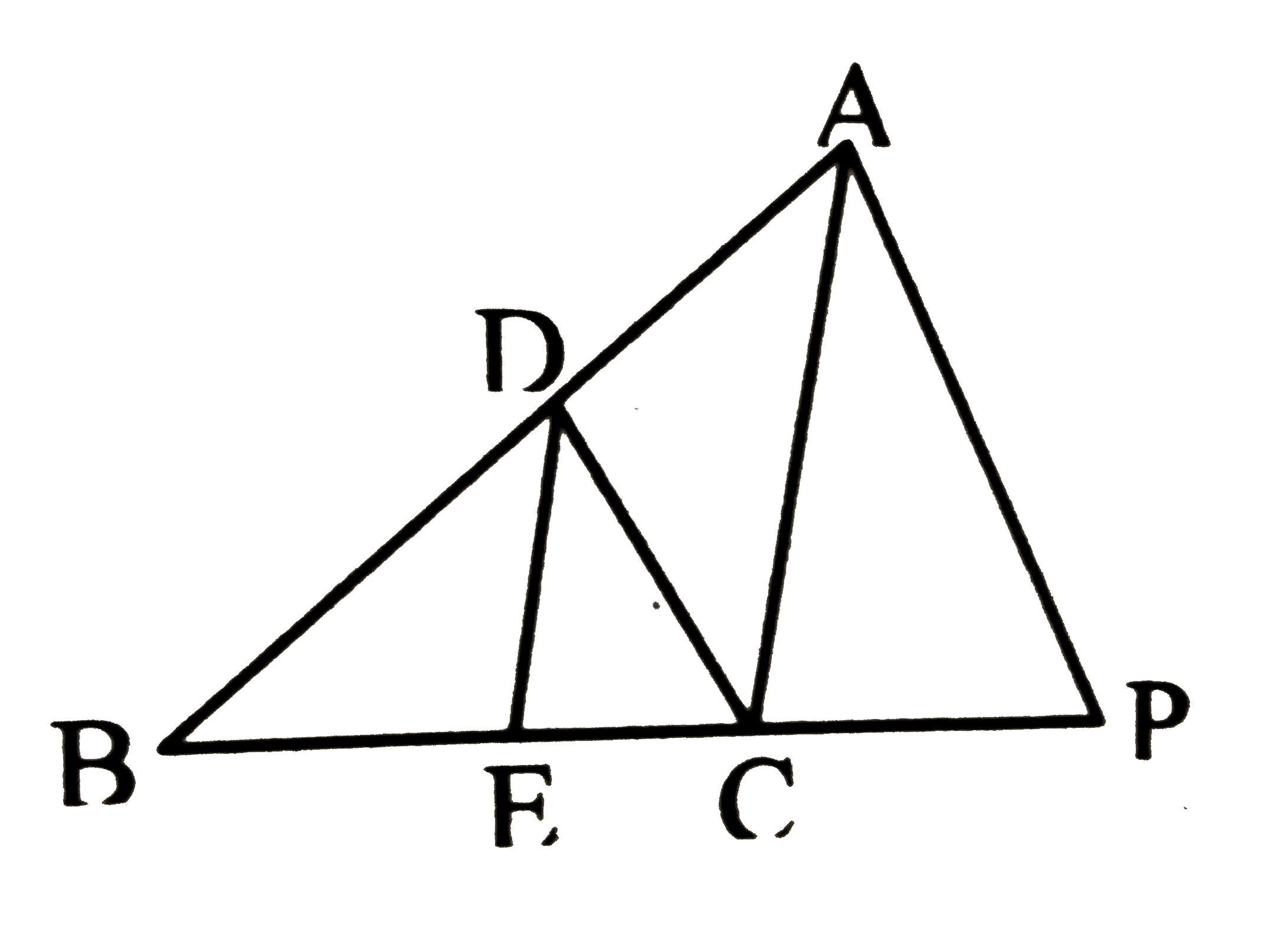 View Solution
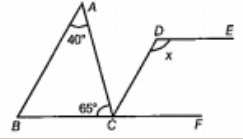
View SolutionIn the figure AB||DC and DE||BF. Find the value of x.
 View Solution
View Solution

