Two persons on the same side of a tall building notice the angle of elevation of the top of the building to be $30^{\circ}$ and $60^{\circ}$ respectively. If the height of the building is $72 m$ , find the distance between the two persons to the nearest metre. $(\sqrt{3} = 1.73$ )

Video Solution

Text Solution

Verified by Experts

In the figure,
AB is the building of height $72 m$ .
C and D are the positions of two persons $B - C - D$
Let $B C = x m$ and $C D = y m$
The angle of elevation from C is $60^{\circ}$
In right angled $Δ A B C, tan ∠ A C B = {tan 60}^{\circ} = \frac{A B}{B C} = \frac{72}{x}$
$∴ \sqrt{3} = \frac{72}{x}$
$∴ x = \frac{72}{\sqrt{3}} = \frac{72}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{72 \sqrt{3}}{3} ∴ x = 24 \sqrt{3} m$ .......1

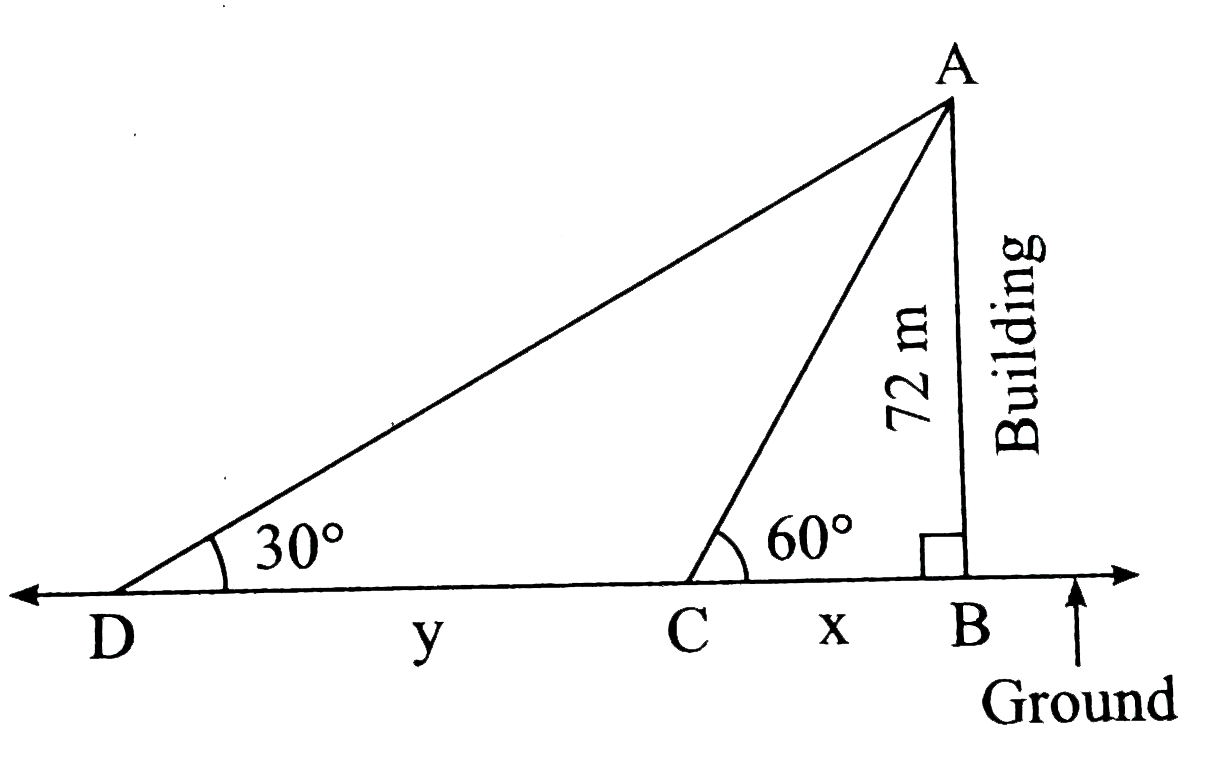
The anglee of elevation from D is $30^{\circ}$ .
In right angled $Δ A B D, tan ∠ A D B = {tan 30}^{\circ} = \frac{A B}{B D} = \frac{72}{x + y}$
$∴ \frac{1}{\sqrt{3}} = \frac{72}{24 \sqrt{3 + y}}$ [From 1]
$: 24 \sqrt{3} + y = 72 \sqrt{3}$
$∴ y = 72 \sqrt{3} - 24 \sqrt{3} = 48 \sqrt{3}$
$∴ y = 48 \times 1.73 ∴ y = 83.04 m \approx 83 m$
The distance between the two persons is $83 m$ .

Updated on:21/07/2023

From two points A and B on the same side of a building the angles of elevation of the top of the building are $30^{\circ} and 60^{\circ}$ respectively. if the height of the building is 10 m find the distances between A and B correct to two decimal places
A10 . 66 m
B13 . 43 m
C11 . 55 m
D12 . 26 m
From the top of a cliff 90 m high, the angles of depression of the top and bottom of a tower are observed to be $30^{\circ}$ and $60^{\circ}$ respectively. The height of the tower is :
A60 m
B75 m
C30 m
D45 m
From the top of a building, the angle of elevation and depression of top and bottom of a tower are $60^{\circ} and 30^{\circ}$ respectively. If the height of the building is $5$ m, then find the height of the tower.
A $10 \sqrt{3}$ m
B15 m
C $15 \sqrt{3}$ m
D20 m