O is any point in the interior of ΔABC. Bisectors of ∠AOB,BOC and AOC intersect sideAB, side BC, side AC in F,D and E respectively.
Prove that BF×AE×CD=AF×CE×BD.
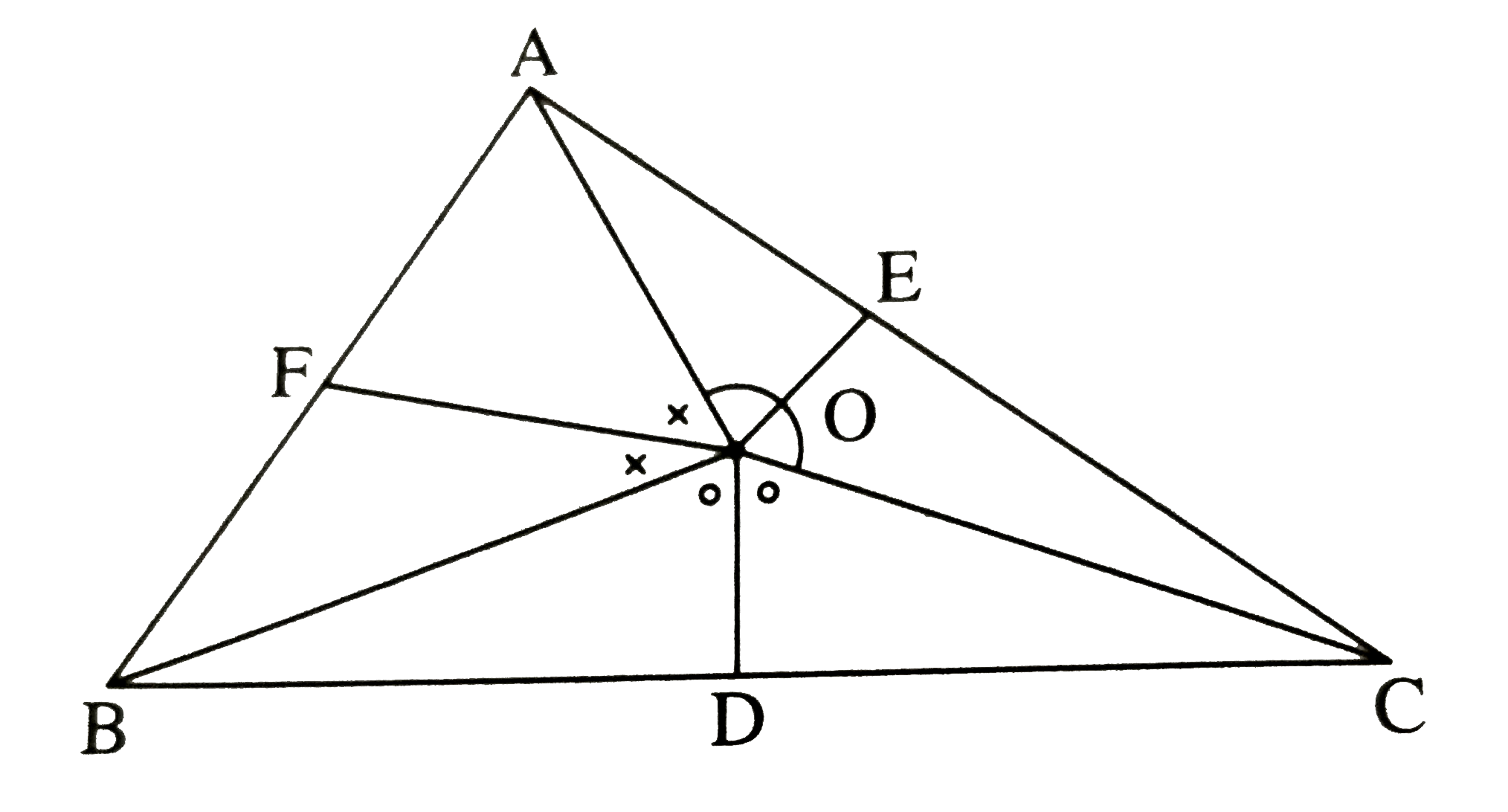
In ΔAOB,
ray OF bisects ∠AOB ...(Given)
AOOB=AFFB …(By theorem of angle bisector of trinagle ) … (1)
In ΔBOC
ray OD bisects ∠BOC … (Given)
OBOC=BDDC ...(By theorem of angle bisector of Delta ) ...(2)
In ΔAOC,
ray OE bisects ∠AOC ... (Given)
OCOA=CEAE ...(By theorem of angle bisector of Delta ) ...(3)
Multiplying (1),(2),(3) , we get
AOOB×OBOC×OCOA=AFFB×BDDC×CEAE
∴1=AFFB×BDDC×CEAE
∴AF×BD×CE=BF×AE×CD
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE )7videos
CHALLENGING QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Choose the correct alternative :3videos
CHALLENGING QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONS EXERCISE - EXAMPLE FOR PRACTICE22videos
CHALLENGING QUESTIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - Challenging Questions10videos
CIRCLE
Similar Questions
Knowledge Check
- Question 1 - Select One
From a point O in the interior of a ΔABC perpendiculars OD, OE and OF are drawn to the sides BC, CA and AB respectively, then which one of the following is true ?
AAF2+BD2+CE2=AE2+CD2+BF2BAB2+BC2=AC2CAF2+BD2+CE2=OA2+OB2+OC2DAF2+BD2+CE2=OD2+OE2+OF2 - Question 2 - Select One
D is any point on side AC of ΔABC. If P,Q, X, Y are the mid points of AB,BC,ADandDCrespectively, then the ratio of PXandQYis
A1:2B1:1C2:1D2:3
Let O be any point in the interior of ΔABC, prove that :
AB+BC+CA<2(OA+OB+OC)View SolutionThe incircle of an isosceles triangle ABC, in which AB = AC, touches the sides BC, CA and AB at D, E and F respectively . Prove that BD = DC.
 View Solution
View SolutionThe incircle of an isosceles triangle ABC, with AB=AC, touches the sides AB, BC, CA at D,E and F respectively. Prove that E bisects BC.
View SolutionThe incircle of an isoceles triangle ABC, with AB=AC, touches the sides AB,BC and CA at D,E and F respecrively. Prove that E bisects BC.
View SolutionIf a line intersects sides AB and AC of a ΔABCat D and E respectively and is parallel to BC, prove that ADAB=AEAC
View SolutionA circle with centre P is inscribed in the ΔABC Side AB, side BC and side A C touch the circle at points L,M and N respectively . Prove that :
A(ΔABC)=12(AB+BC+AC)×r
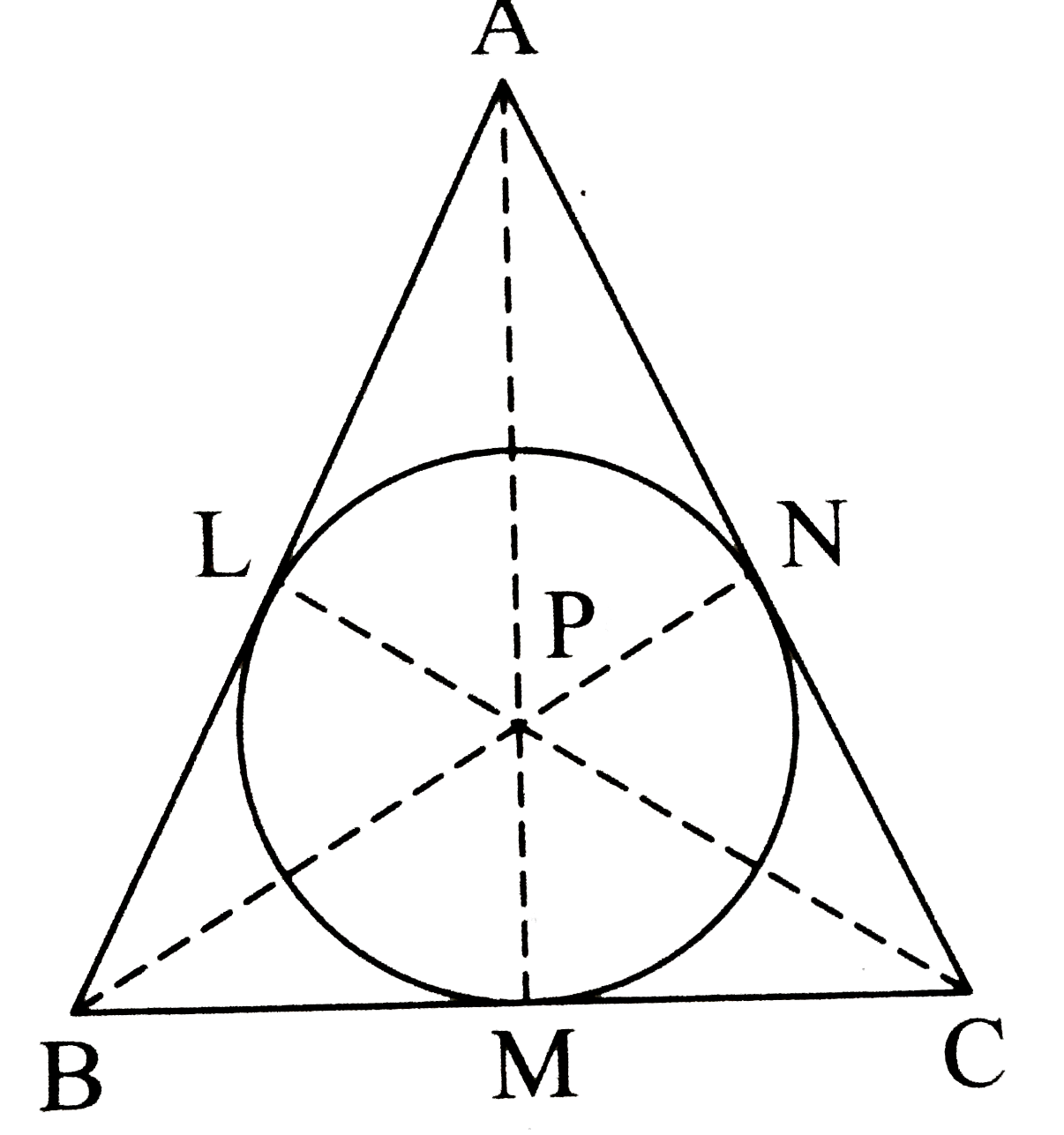 View Solution
View Solution

