In the figure, O is the centre of the circle. Seg AC is the diameter ∠ABC is inscribed in arc ABC and intercepts arc AMC then prove ∠ABC=90∘

m (arc AMC) =180∘ …. (Measure of a semicircle is 180∘)
∠ABC=12m (arc AMC) …. (Inscribed angle theorem)
∴∠ABC=12×180∘
∴∠ABC=90∘
Topper's Solved these Questions
- MODEL QUESTION PAPERBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPEREXERCISE - COMPLETE ANY ONE OUT OF TWO ACTIVITES:
2 Videos
- MODEL QUESTION PAPERBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPEREXERCISE - SOLVE ANY TWO OF THE FOLLOWING SUBQUESTIONS:
4 Videos
- MODEL QUESTION PAPERBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MODEL QUESTION PAPEREXERCISE - COMPLETE ANY TWO OUT OF THREE ACTIVITIES :
3 Videos
- MENSURATIONBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MENSURATIONEXERCISE - Assignment 8.5
8 Videos
- PRACTICE QUESTION BASEDBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PRACTICE QUESTION BASEDEXERCISE - STATISTICS
13 Videos
Similar Questions
In Figure, AB=CB and O is the centre of the circle. Prove that BO bisects ∠ABC.
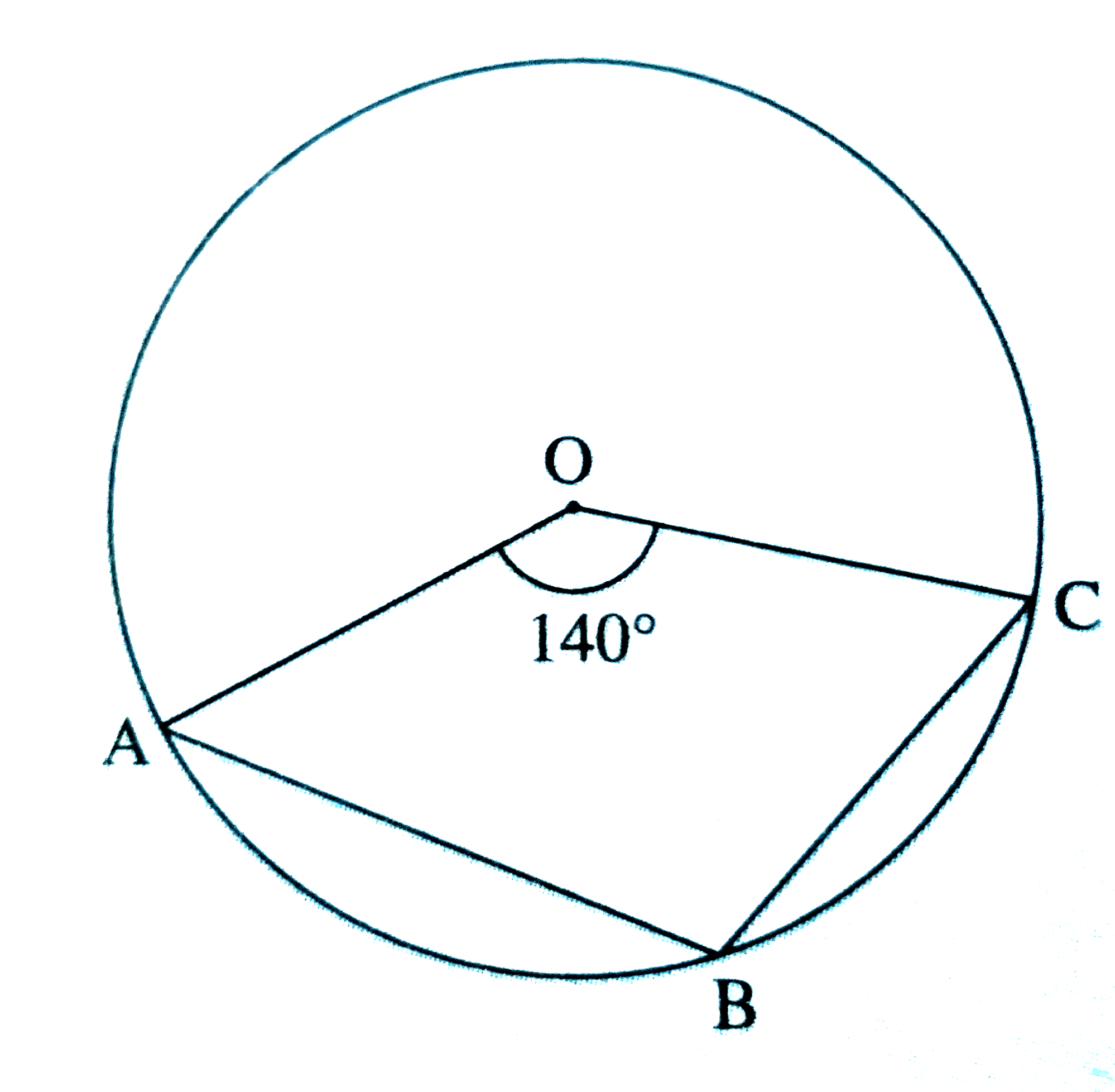
View SolutionIn the figure, O is the centre of the circle such that ∠AOC−140∘, then ∠ABC=…….
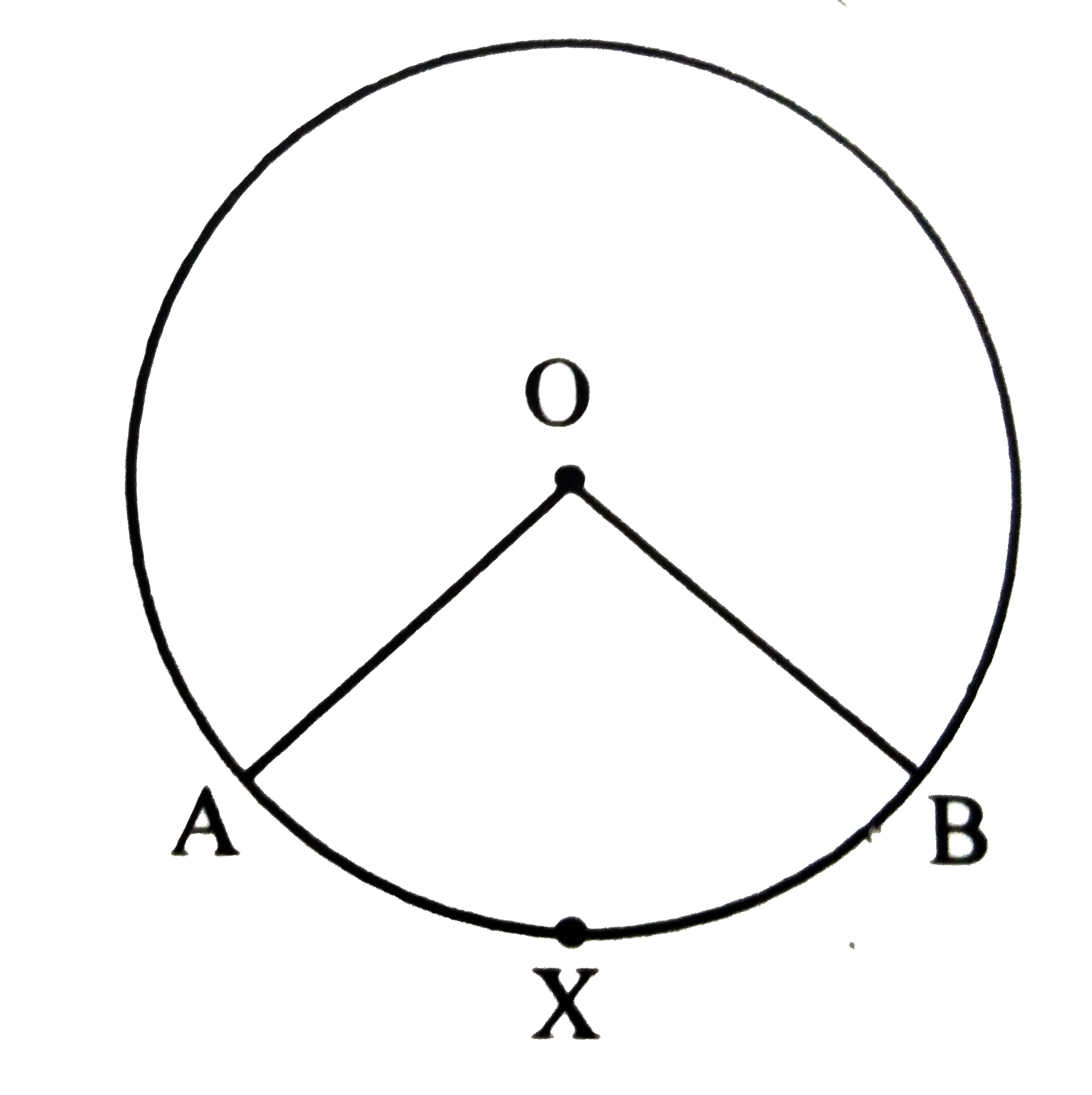
View SolutionIn the figures, ∠ABC is inscribed in arc ABC and intercepts arc AXC and ∠BCD is inscribed in arc BCD and intercepts arc BYD. If ∠ABC=30∘ and m ( arc BYD ) =80∘ then the m ( arc AXC ) = ….... and measure of ∠BCD=….........

View SolutionIn the given figure , O is the centre of the circle. If m(arc AXB)=80∘, then m∠AOB=
View SolutionIn the figure, O is the centre of the circle . Seg AB is the diameter at the point C on the circle the tangent CD is drawn. Line BD is a tangent to the circle at point B. Show that seg OD ∣∣ chord AC.
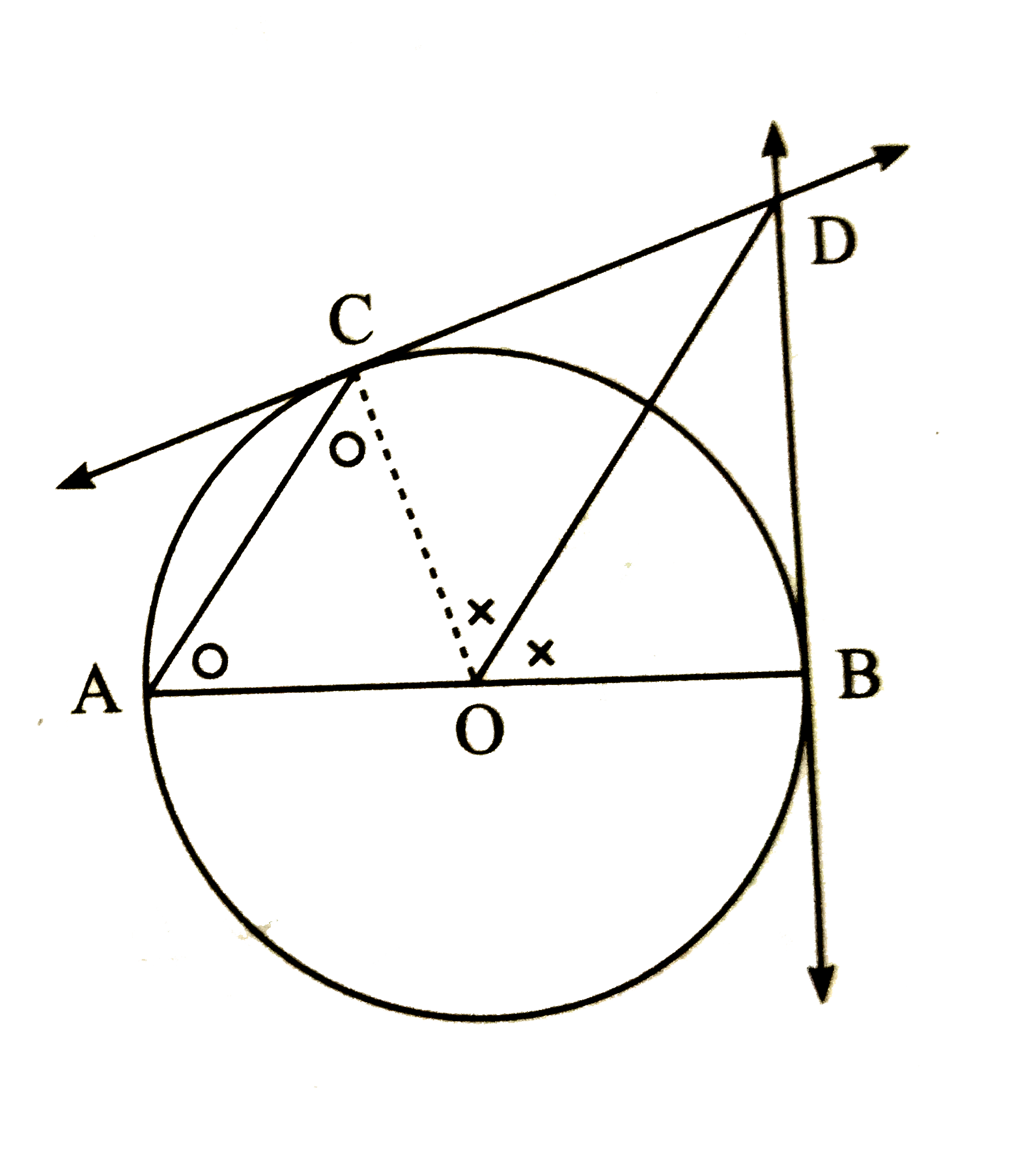
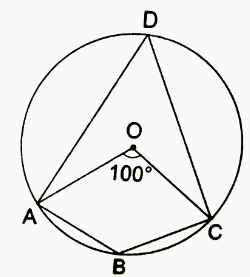
View SolutionIn Figure, O is the center of the circle and the measure of arc ABC is 100∘ Determine ∠ADCand∠ABC
View SolutionIn the given figure, O is the centre of the circle and BA = AC. If ∠ABC=50∘, find ∠BOC and ∠BDC.
View Solution,'O' is the centre of the circle, segment AB is diameter of the circle. If ∠ABC=34∘ find m(arcAXC)
View SolutionCorollaries of inscribed angle theorem :
Angle inscribed in the same arc arc contruent
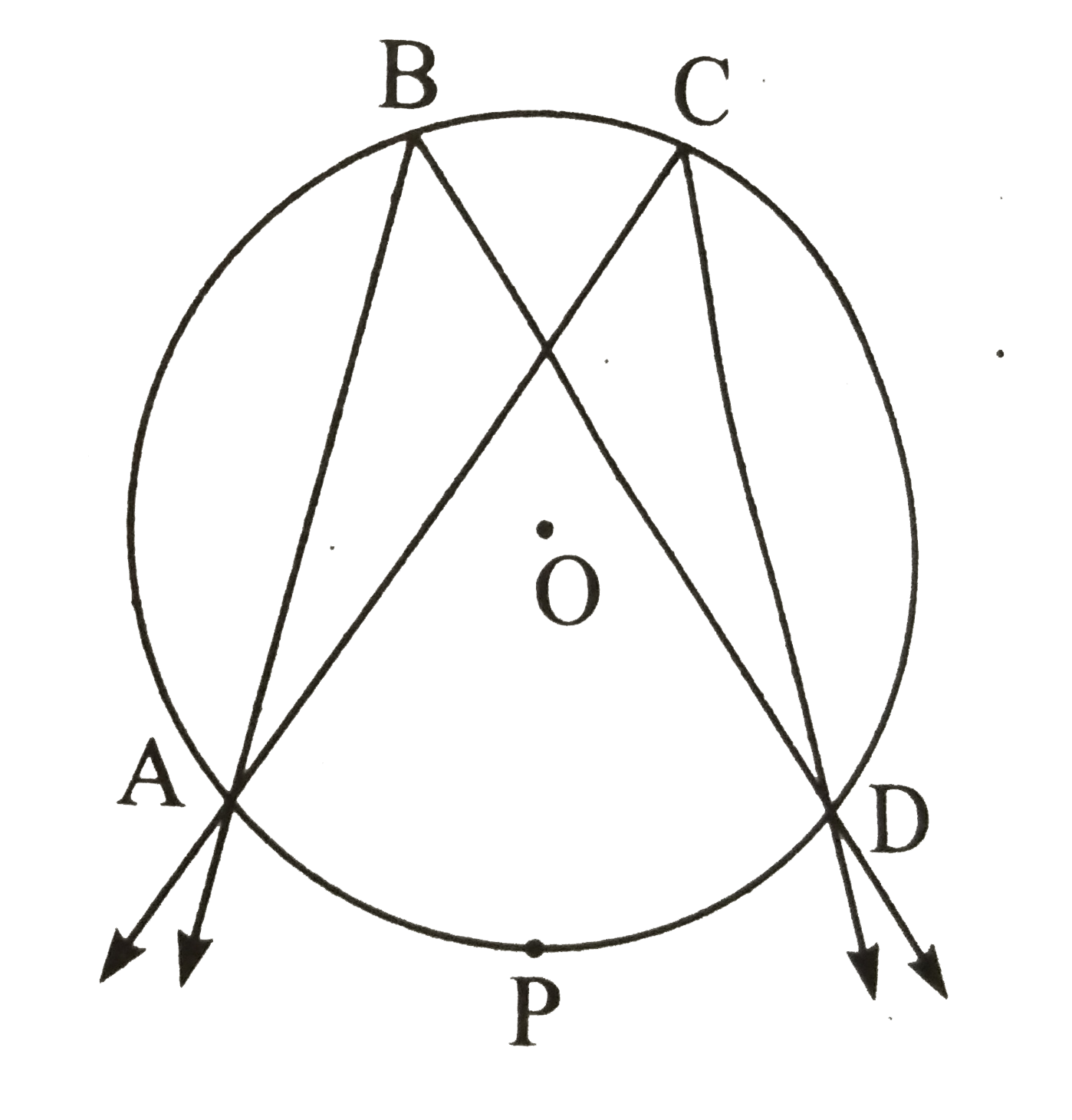
Given : (1) A circle with centre O
(2) ∠ABD and ∠ACD are inscribed in arc ABC and intercepts arc APD.
To prove : ∠ABD≅∠ACD
View SolutionIn the following figure, O is the centre of the circle. ∠ABC is inscribed in arc ABC and ∠ABC=65∘. Complete the following activity to find the measure of ∠AOC
Activity :
∠ABC=12m□ …..(Inscribed angle theorem)
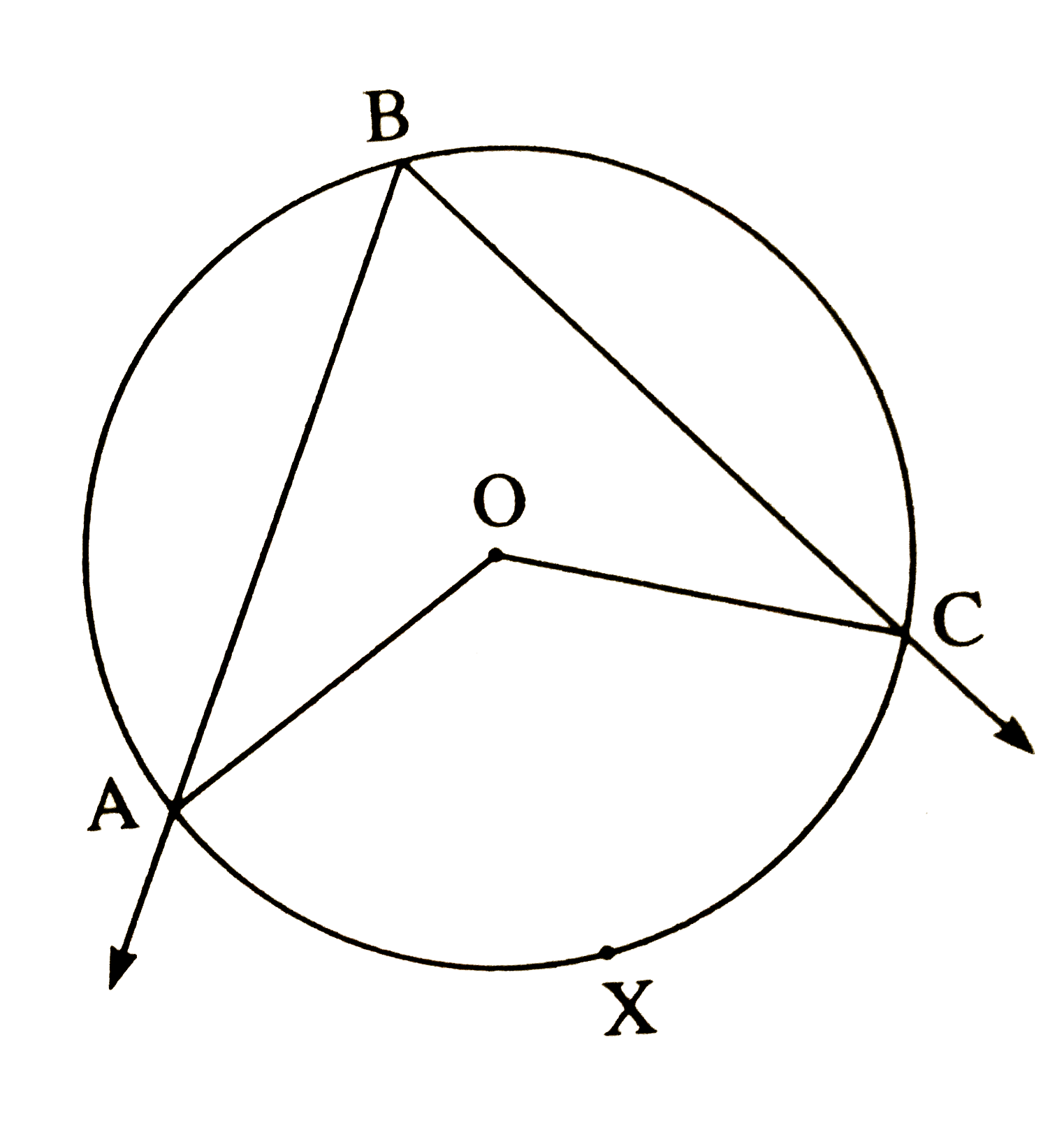
∴□×2m (arc AXC)
∴m (arc AXC = □
∠AOC=m (arc AXC)... (Definition of measure of minor arc)
∴∠AOC=□
View Solution
