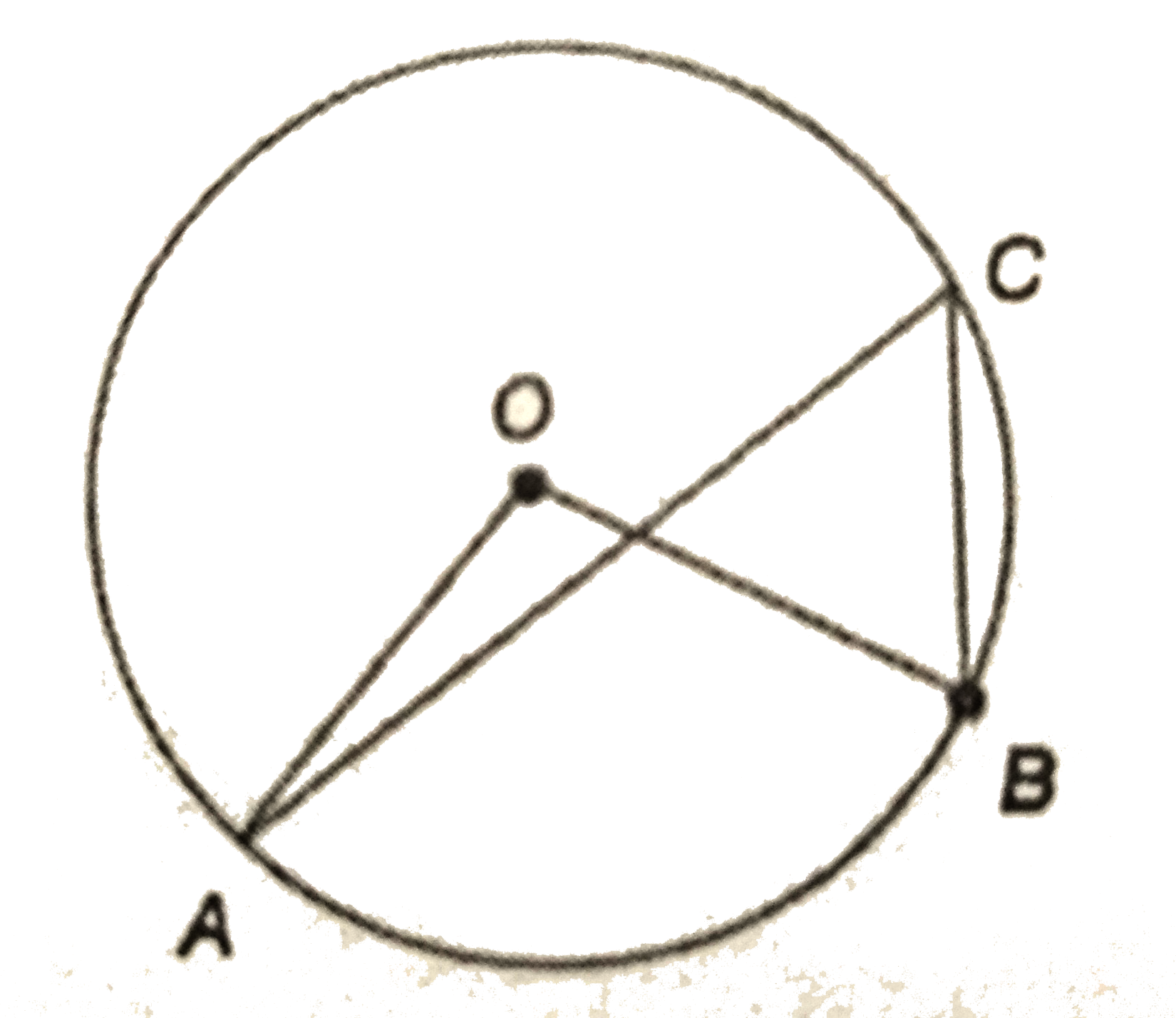,'O' is the centre of the circle, segment AB is diameter of the circle. If ∠ABC=34∘ find m(arcAXC)
Step by step video & image solution for ,'O' is the centre of the circle, segment AB is diameter of the circle. If angle ABC=34 ^@ find m(arc AXC) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
In a circle with the centre is O and AB is the diameter of the circle and CD is the chord of the circle. If angle ABC is 54∘ and the length of CD and BC is equal, then find the ∠DBC.
A1. 36∘B2. 24∘C3. 48∘D4. 12∘PQ is tangent at a point R of the circle with centre O. If ST is a diameter of the circle and ∠TRQ=30∘ find ∠PRS
A30∘B60∘C45∘D120∘
In the adjoining figure, O is the centre of the circle. AB is an arc of the circle and ∠AOB=80∘. Find ∠ACB.

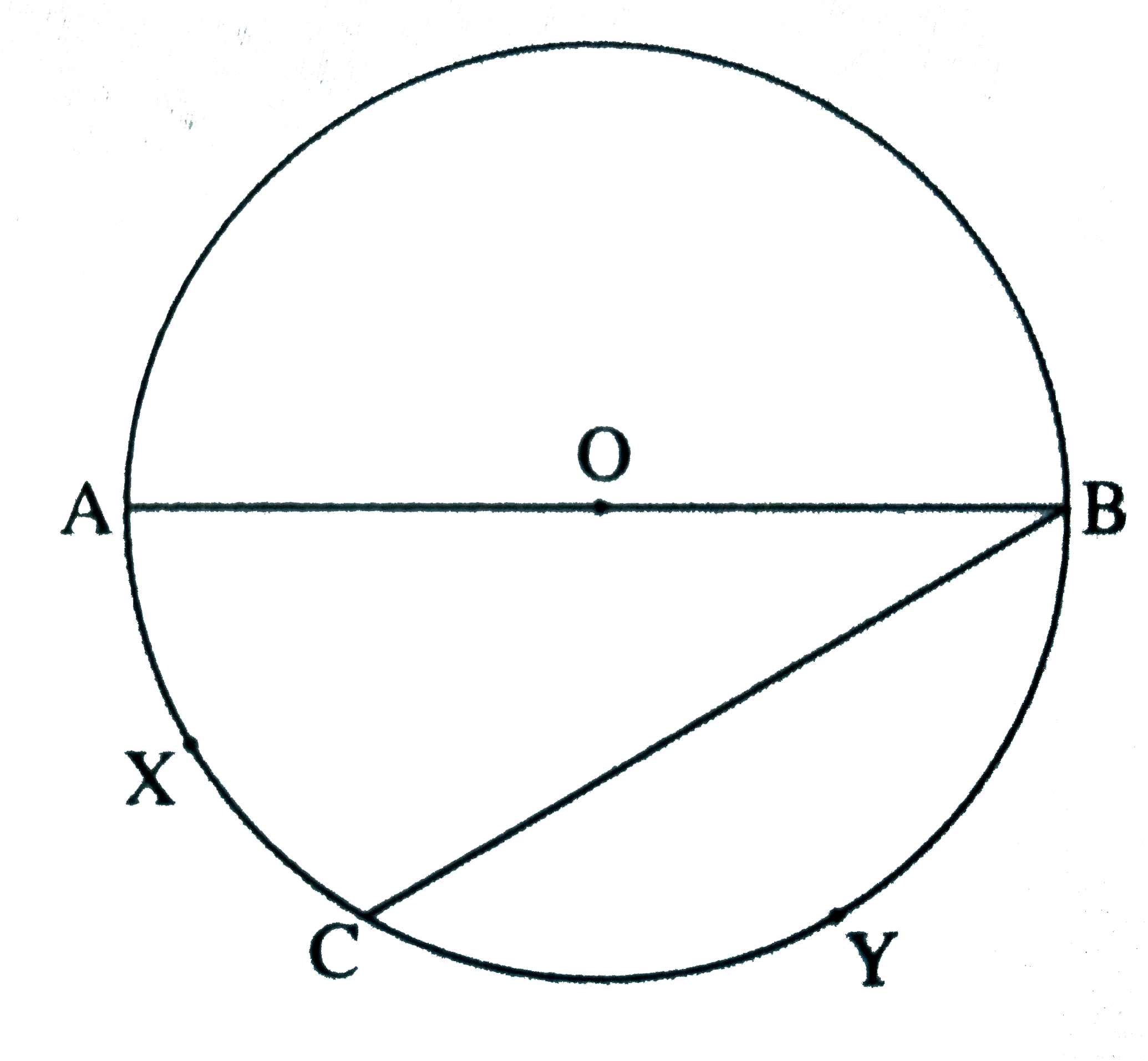
View SolutionIn the figure, seg AB is the diameter of the circle, ∠ABC=30∘, Find (1) m(arc AXC)
(2) m(arc BYC)
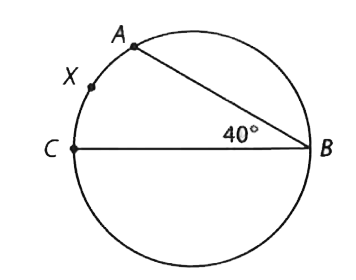
View SolutionAngle ABC is 40∘ (see figure) and the area of the circle is 81π. If CB is a diameter of the circle, how long is arc AXC?
View SolutionIn the figure, O is the centre of the circle. Seg AC is the diameter ∠ABC is inscribed in arc ABC and intercepts arc AMC then prove ∠ABC=90∘

View SolutionIn the given 'O' is the centre of the circle, Arc AB=ArcBC=Cd.If∠OAB=48∘, find:
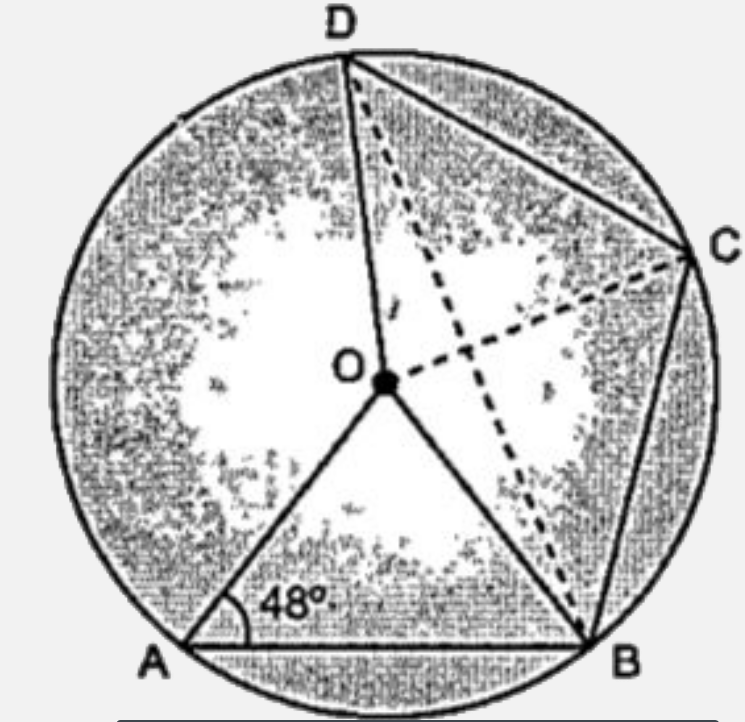
∠OBD
View SolutionIn the given figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC.
if ∠AOB=100∘ find ∠BOC
View Solution