Question

Define practical simple pendulum. Show that motion of bob of pendulum with small amplitude is linear S.H.M. Hence obtain an expression for its period. What are the factors on which its period depends ?
The total free surface energy of a liquid drop is $\pi \sqrt{2}$ times the surface tensin of the liquid. Calculate the diameter of the drop in S.I unit.

Explanation

Consider a simple pendulum of length L, suspended from a rigid support O. When displaced from its initial position of rest through a small angle `theta` in a vertical plane and released, it performs oscillations between two extremes, B and C as shown in the figure. At B, teh forces on the bob are its weight `mvecg` and the tension `vecF_(1)` in the string. Resolve `mvecg` into two components : mg cos `theta` in the direction opposite to that of the tension and mg sin `theta` perpendicular to the string. mg cos `theta` is balanced by the tension in the string. mg sin `theta` tends to restore the bob to the equilibrium position.

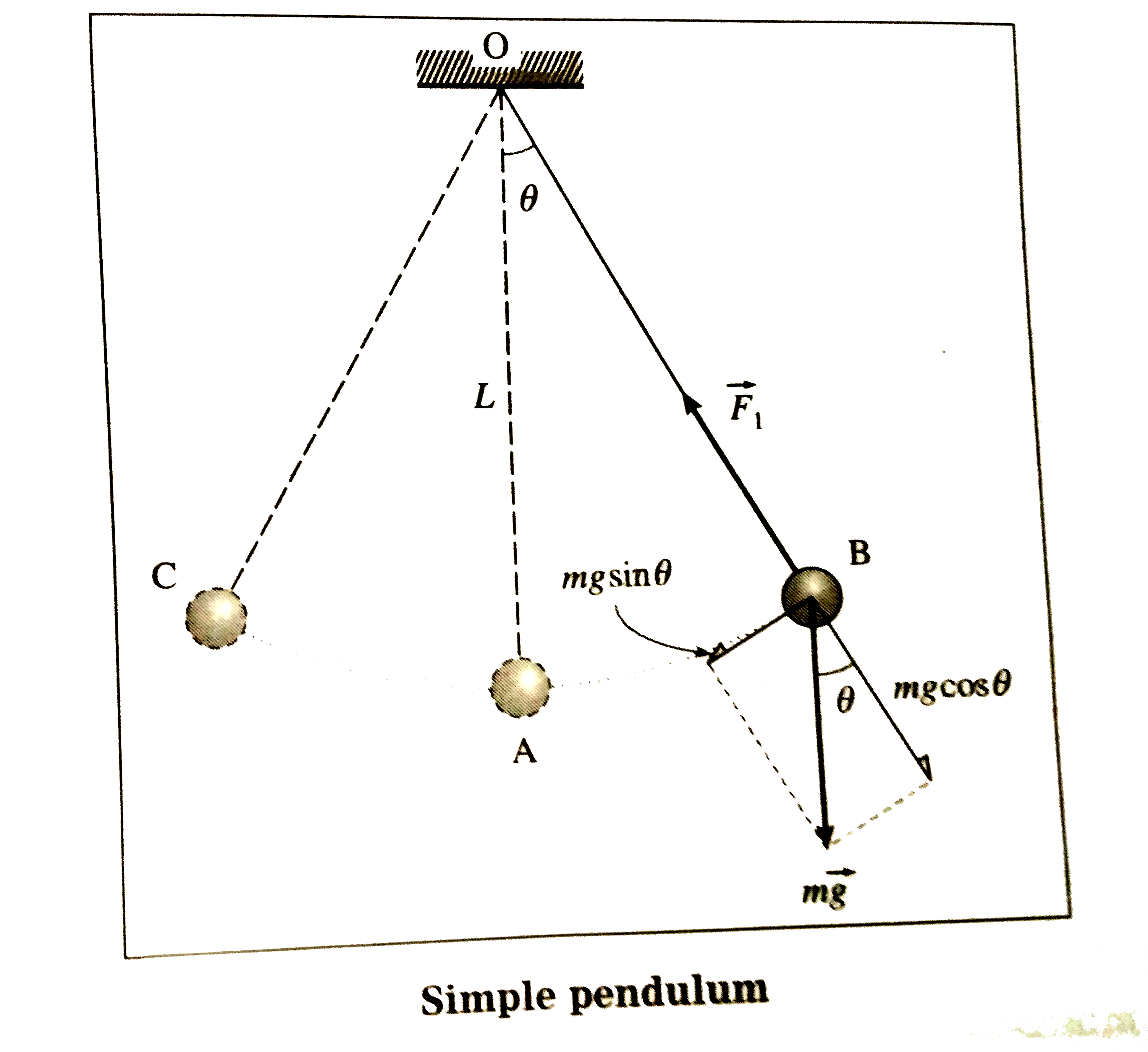
Restoring force, `F=-mg sin theta" "`.....(1)
It `theta` is small and expressed in radian,
`sin theta~~theta=(arc)/(radius)=(AB)/(OB)=(x)/(L)`
`:. F=-mgtheta=-mg(x)/(L)" "`.......(2)
Since m,g and L are constant, `" "F pop(-x)" "`.....(3)
Thus, the magnitude of the restoring force si proportional to the magnitude of the displacement and the force is in the direction opposite to that of displacement x of the bob from its mean position as indicated by the minus sign. Hence, it follows that the motion of a simple pendulum is linear SHM.
Now, `F=ma=-mg=-mg(x)/(L)" " a=-g(x)/(L)" "`.....(4)
From Eq. (4), acceleration per unit displacement `=|(a)/(x)|=(g)/(L)`
Period of SHM,
`T=(2pi)/(omega)=(2pi)/(sqrt("acceleration per unit displacement"))=(2pi)/(sqrt(g//L))`
`:. T=2pisqrt((L)/(g))`
This expression shows that the period of a simple pendulum depends upon the length of the pendulum and the acceleration due to gravity at the palce.