A particle of charge q and mass m is projected with a velocity $v_{0}$ toward a circular region having uniform magnetic field B perpendicular and into the plane of paper from point P as shown in Fig. 1.136. R is the radius and O is the center of the circular region. If the line OP makes an angle $θ$ with the direction of $v_{0}$ then the value of $v_{0}$ so that particle passes through O is

A

$\frac{q B R}{m sin θ}$

B

$\frac{q B R}{2 m sin θ}$

C

$\frac{2 q B R}{m sin θ}$

D

$\frac{3 q B R}{2 m sin θ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let r be the radius of circular path. If the particle passes through O, then from the given figure.
$sin θ = \frac{R / 2}{r} = \frac{R}{2 r}$ ………..(i)
As $r = \frac{m v_{0}}{q B}$
$∴ v_{0} = \frac{q B r}{m} = \frac{q B R}{2 m sin θ}$ (Using (i))

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

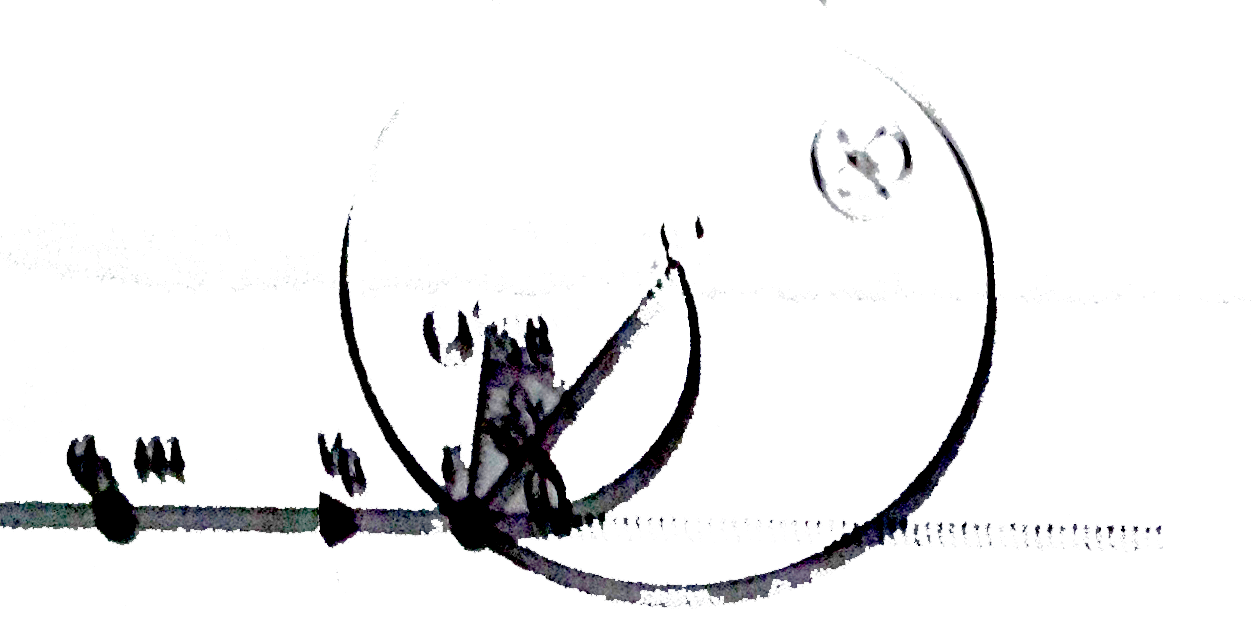
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A charged particle of charge $Q$ and mass $m$ moves with velocity $v$ in a circular path due to transverse magnetic field, B, then its frequency is
A $\frac{Q B}{2 π m}$
B $\frac{Q v B}{2 π m}$
C $\frac{Q m v B}{2 π}$
D $\frac{v b}{2 π Q m}$
Question 2 - Select One
A charge particle of charge q and mass m is moving with velocity v as shown in fig In a uniform magnetic field B along -ve z-direction.Select the correct alternative (s).

AVelocity of the particle when it comes out form the magnetic field is $\to v - v {cos 60}^{\circ} ˆ i + v {sin 60}^{\circ} ˆ j$
BTime for which the particle was in magnetic field is $\frac{π m}{3 q B}$
CDistance travelled in magnetic field is $\frac{π m v}{3 q B}$
DNone of these
Question 3 - Select One
A particle of charge $q$ and mass $m$ moving with a velocity $v$ along the x-axis enters the region $x > 0$ with uniform magnetic field $B$ along the $ˆ k$ direction. The particle will penetrate in this region in the $x$ -direction upto a distance $d$ equal to
AZero
B $\frac{m v}{q B}$
C $\frac{2 m v}{q B}$
Dinfinity

NCERT FINGERTIPS-MOVING CHARGES AND MAGNETISM -Higher Order Thinking Skills

Home
Ask Doubt
Profile