Manas has drawn a circle with centre O of which AB is a chord. A tangent is drawn at the point B which intersects extended AO at the point. T. If ∠BAT=21∘, find the value of ∠BTA. Solution in Bengali
Given that ∠BAT=21∘
∴∠OBA=21∘[∵OA=OB, radii of same circle]
Again, BT is a tangent at B of the cirxcle with centre O and OB is a radius passing through B.
∴OB⊥BT,∴∠OBT=90∘
Now ∠ABT=∠ABO+∠OBT
=21∘+90∘[∠OBA=21∘and∠OBT=90∘]=111∘
Then ∠BAT=180∘−(∠BAT+∠ABT+∠ABT)[∵ sum of three angles of a triangle is 180∘]
=180∘−(21∘+111∘)=180∘−132∘=48∘
Hence ∠BAT=48∘.

Topper's Solved these Questions
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.120videos
THEOREMS RELATED TO TANGENT IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.223videos
THEOREMS RELATED TO TANGENT IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXERCISE 2.3 (Long Answer Type Questions)14videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - TRIGONOMETRIC RATIOS AND TRIGONOMETRIC IDENTITIES EXERCISE - EXERCISE66videos
TRIGONOMETRIC RATIOS AND TRIGONOMETRIC IDENTITIES
Similar Questions
PR and PS are two tangents drawn from a extental point P of the Circle with centre at O. If PR=8cmand∠RPO=60∘, then the length of PS=
01:00View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
06:15View SolutionProve that angle in the segment of a circle which is greater than a semi-circle is an acute angle.
08:30View SolutionIf tanθ=pq, what is the value of psecθ−q cosecθpsecθ+q cosecθ=
09:02View SolutionIf a chord AB subtends and angle of 60∘ at the centre of a circle, then the angle between the tangents to the circle drawn from A and B is
03:53View SolutionTwo radii OA and OB of a circle with centre O are perpendicular to each other. If two tangents are drawn at the point A and B intersect each other at the point T, prove that AB=OT and they bisect each other at a ridght angle.
07:17View SolutionP is any point on diameter of a circle with centre O.A perpendicular drawn on diameter at the point O intersects the point O intersects the circle at the point Q. Extended QP intersects the circle at the point R.A tangent drawn at the point R intersects extended OP at the point S. Prove theat SP=SR.[GP−X]
02:16View SolutionTwo chords AC and BD of a circle intersect each other at the point O. If two tangents drawn at the points A and B intersect each other at the point P and two tangents drawn at the points C and D intersect at the point Q, prove that ∠P+∠Q=2∠BOC.[GP−X]
03:39View SolutionIn the figure, O is the centre and BOA is a diameter of the circle. A tangent drawn to the circels at the point P intersects the extended BA at the point T. If ∠PBO=30∘, find the value of ∠PAT.
02:55View SolutionTwo tangents are drawn from an external point A of the circle with centre at O which touches the circle at the points B and C. Prove that AO is the perpendicular bisector of BC.
View SolutionIn Fig O is the centre of a circle and AT is a tangent at point A . what is the measure of ∠BAT ?
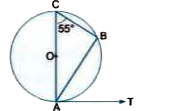 04:49View Solution
04:49View SolutionMasum O draws a central circle whose AB is a chord. Draw a tangent at point B which intersects the extended AO at point T. angleBAT=21^@ If, angleBTA Calculate the value of.
04:01View SolutionIf a chord AB subtends and angle of 60∘ at the centre of a circle, then the angle between the tangents to the circle drawn from A and B is
03:32View SolutionA tangent line from point A is drawn on a circle of unit radius. Line segment AB is drawn whose length is equal to the length of arc AC. A straight line BC is drawn. Which intersects the diameter of the extended circle at the end point P of AO. If the tangent at C intersects at point O of increased PA then DeltaCPQ Is the area of
03:25View Solutionयदि जीवा AB वृत्त के केन्द्र पर 60∘ का कोण अंतरित करता है तो A और B बिन्दुओं पर खीची गई स्पर्शरेखाओं के बीच का कोण होगा
03:23View Solution

