Two radii OA and OB of a circle with centre O are perpendicular to each other. If two tangents are drawn at the point A and B intersect each other at the point T, prove that AB=OT and they bisect each other at a ridght angle. Solution in Bengali
Two radii OA and OB of a circle with centre at O are prependicular to ecah other. Two tangents ST and RT at the points A and B respectively intersect each other at T.
Let us join the points A, B and O, T. Let AB and OT intersect eacth other at C.
To prove : AB=OT and AB and OT bisect each other at a right angle.
Proof : In the quadrilateral OATB,OA⊥OB (given),∴∠AOB=90∘,OA⊥TS,∠OAT=90∘andOB⊥TR∴∠OBT=90∘,i.e., three of the angles of OATB are right-angles. So, the fourth angle is also a right angle.
∴ each of the angles of OATB is a right angle.
Again, OA=OB[∵ radii of same circle]
∴OA=OB=BT=TA.
therefoer each of the side of the quadrillateral OATB are eqial and each of the angles is a right angle.

∴OATB is a square and AB and OT are two of its diagonals.
We know that diagonals of a square are equal and the diagonals bisect each other at right-angles.
∴AB=OT and AB and OT bisect each other at a right angle. [Proved]
Topper's Solved these Questions
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.120videos
THEOREMS RELATED TO TANGENT IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.223videos
THEOREMS RELATED TO TANGENT IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXERCISE 2.3 (Long Answer Type Questions)14videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - TRIGONOMETRIC RATIOS AND TRIGONOMETRIC IDENTITIES EXERCISE - EXERCISE66videos
TRIGONOMETRIC RATIOS AND TRIGONOMETRIC IDENTITIES
Similar Questions
PR and PS are two tangents drawn from a extental point P of the Circle with centre at O. If PR=8cmand∠RPO=60∘, then the length of PS=
02:07View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
03:52View SolutionProve that angle in the segment of a circle which is greater than a semi-circle is an acute angle.
05:52View SolutionIf tanθ=pq, what is the value of psecθ−q cosecθpsecθ+q cosecθ=
05:38View SolutionThe radius of a circle with centre O is 7 cm. Two radii OA and OB are drawn at right angles to each other. Find the areas of minor and major segments.
07:33View Solutionचित्र में O एवं O' केन्द्र के दो वृत्त एक-दूसरे का बाह्यतः बिन्दु C पर स्पर्श करते है। इन वृत्तों की एक उभयनिष्ठ स्पर्श रेखा AB खींची गई है। सिद्ध कीजिए कि ∠ACB=90∘
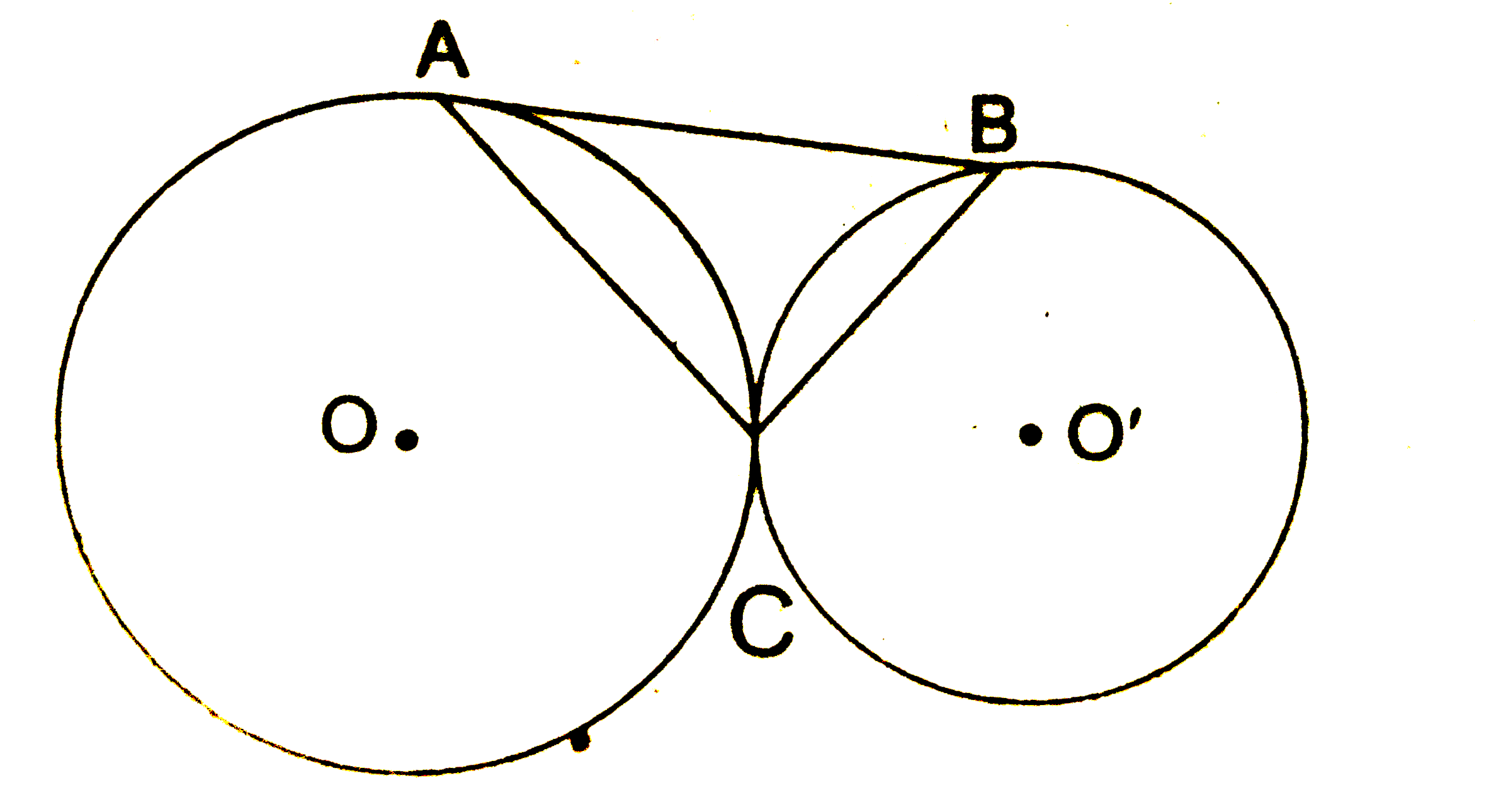 09:02View Solution
09:02View Solutionदो वृत्तों के केन्द्र O और O ' है, जो एक-दूसरे को बाहृतः बिन्दु P पर स्पर्श करते है। इन वृत्तों की एक उभयनिष्ट अनुस्पर्श रेखा AB खींची जाती है। सिद्ध कीजिए कि ∠APB=90∘.
02:06View Solutionदो वृत्त एक-दूसरे को दो बिन्दुओं पर प्रतिच्छेद करते हैं | सिद्ध कीजिए कि इनके केन्द्रों को मिलाने वाली रेखा, उभयनिष्ठ जीवा को लम्ब समद्विभाजित करती है |
06:23View SolutionTwo chords AB and CD of a circle with centre O intersect each other at the point P. prove that AOD+∠BOC=2∠PBC. If ∠BOC are supplementary to each other, then prove that the two chords are perpendicular to each other.
03:05View SolutionTwo chords AC and BD of a circle intersect each other at the point O. If two tangents drawn at the points A and B intersect each other at the point P and two tangents drawn at the points C and D intersect at the point Q, prove that ∠P+∠Q=2∠BOC.[GP−X]
03:35View SolutionTwo tangents drawn at the point A and B on a circle intersect each other at the point P. If ∠APB=60∘, then ∠PAB=
07:50View Solutionदी आकृति में, PO⊥QO है। O केन्द्र के वृत्त में, बिन्दु P और Q पर खींची स्पर्श रेखायें बिन्दु T पर कटती है। सिद्ध कीजिए कि PQ और OT एक-दूसरे को समकोण पर समद्विभाजित करती है।
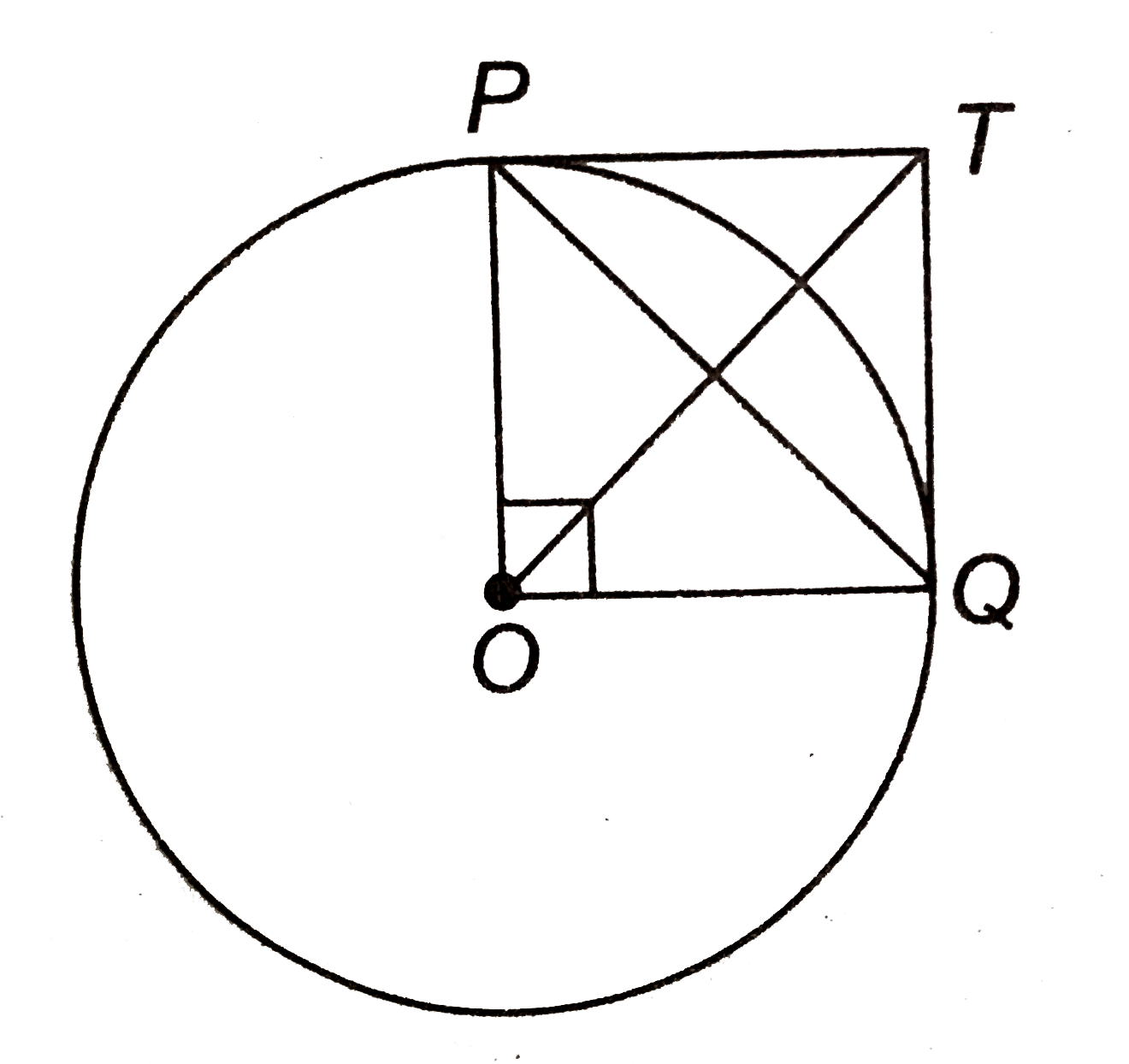 06:00View Solution
06:00View SolutionTwo simple lines PQ and RS intersect each other at O point. If the line OA, anglePOR Co and line OB, angleQOS If bisects, prove that OA and OB will be located on the same straight line.
01:45View SolutionTwo tangents AB and AC are drawn from an external point A to a circle with centre O . If they are inclined to each other at an angle of 100∘ then what is the value of ∠BOC.
06:02View SolutionO The two chords AB and CD of a central circle intersect at the point P. Prove that angleAOD+angleBOC=2angleBPC . If angleAOD Oh angleBOC If they complement each other, then we prove that the two chords are perpendicular to each other.
02:24View Solution

