A boat is moving with a velocity vbw=5km/hr relative to water. At time t=0.the boat passes through a piece of cork floating in water while moving down stream.If it turns back at time t1=30min.
a) when the boat meet the cork again?
b) The distance travelled by the boat during this time.
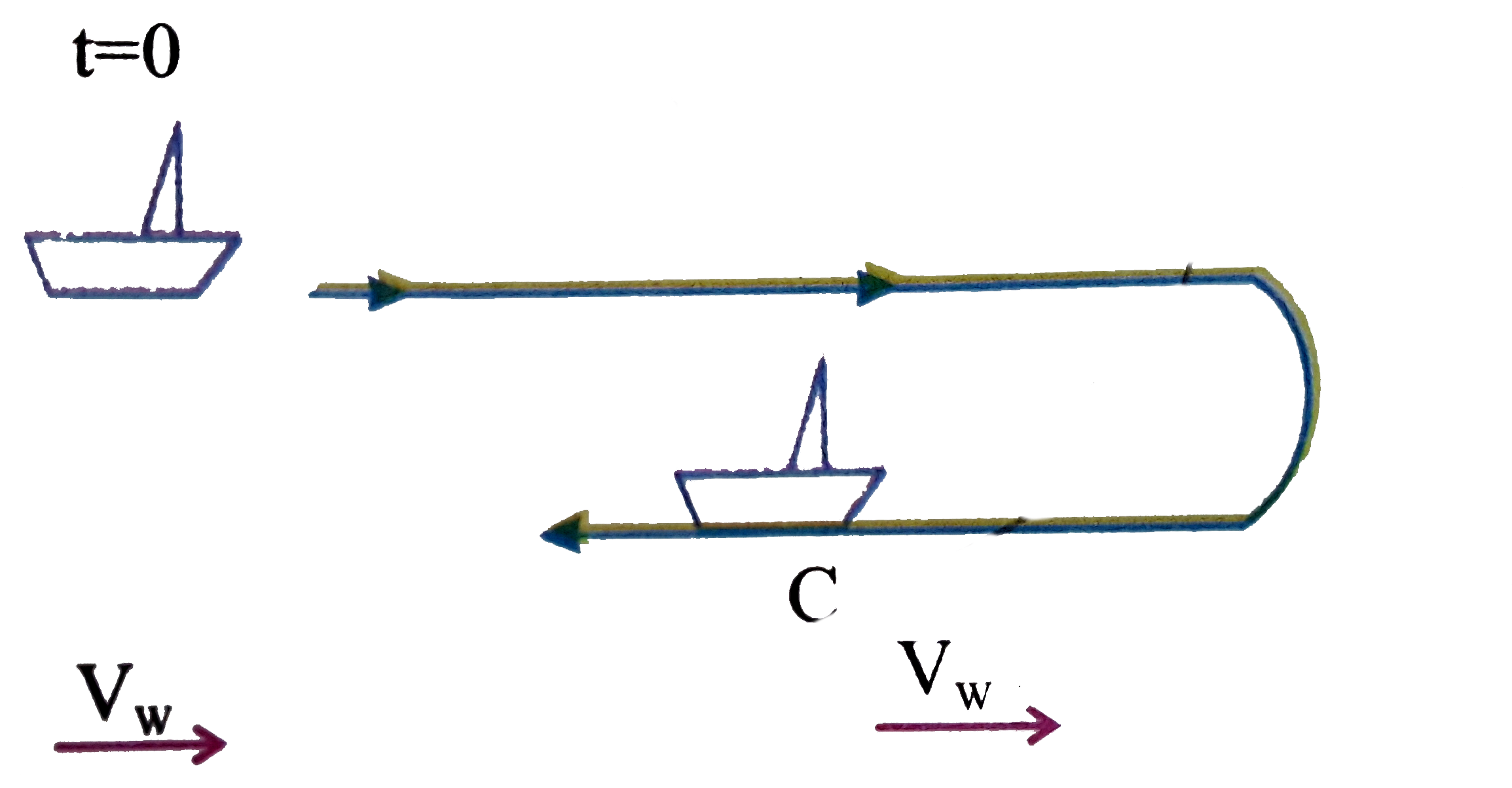
Time of travelling of both from A to B ,(T1) and B to C , (T'1=time of moving the cork from A to C.
 .
.
Velocity of boat from A to B
→vb,w+vedcvw=(5+u)kmh−1
And velocity of boat from B to C
→vb,w+vedcvw=(5+u)kmh−1
Distance moved by boat in time t'1
AB=(5+4)t1
And disannce moved by boat in timet'1=BC=(5−u)t'1
Distance moved by cord during this time
AC=u(t1+t'1)
But AB=AC+BC
(5+u)t1=u(t1+t'1)+(5−u)t'1
5t1=5t'1⇒t'1=t1=30min
Hence, the cord meets the boat again aftre 1h.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Solved Examples
9 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.1
17 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
If the ratio of the speed of the boat in downstream and speed of the stream is 7 ∶ 2 and the speed of the boat in still water is 35 km/hr. What would be the distance travelled in upstream by boat in 5 hours?
View SolutionA boat of 90kg is floating in still water. A boy of mass 30 kg walks from the stern to the bow. The length of the boat is 3 m. Calculate the distance through which the boat will move.
View Solution
Knowledge Check
A boat takes 4hrs to travel certain distance in a river in down stream and it takes 6hrs to travel the same distance in upstream. Then the time taken by the boat to travel the same distance in still water is
A4.8hrsB9.8hrsC24hrsD10hrsA boat can be rowed in still water at a speed u. The boat is moving downstream in a river in which water flows at a speed v. There is raft floating in water and therefore moving along with water at speed v. Let the boat overtakes the raft at the moment t = 0.
The distance between the boat and raft at a later instant of time t isA(u+v)tButCvtD(u−v)tA boat can travel with a speed of 30 km/hr in still water. If the speed of the stream is 6 km/hr, find the time taken by the boat to go 108 km downstream.
A2 hrsB5 hrsC3 hrsD4 hrs
Speed of a motorboat in still water is 45 km./hr. If it takes 1 hour 20 min. to travel 80 km. downstream, how much time will it take to cover the same distance the stream ?
View SolutionA boat can be rowed in still water at a speed u. The boat is moving downstream in a river in which water flows at a speed v. There is raft floating in water and therefore moving along with water at speed v. Let the boat overtakes the raft at the moment t = 0.
Let the boat turns back at time t=t0 and starts moving towards the raft. The separation between the raft and boat after a time interval t' measured from the moment of turning back is
View SolutionA boat can be rowed in still water at a speed u. The boat is moving downstream in a river in which water flows at a speed v. There is raft floating in water and therefore moving along with water at speed v. Let the boat overtakes the raft at the moment t = 0.
If the raft has covered a distance x during the interval in which boat overtakes the raft, turns back and crosses it again the speed of stream is
View SolutionA boat moves relative to water with a velocity v and river is flowing with 2v. At what angle the boat shall move with the stream to have minimum drift?
View SolutionA boat moves relative to water with a velocity which is n=2.0 times less than the river flow velocity. At what angle to the stream direction must the boat move to minimize drifting?
View Solution
