A 2−m wide truck is moving wigh a uniform speed v0=8ms−1 along a straight horizontal road. A pedestrian starts to cross the road with a uniform speed v when the truck is 4m away from him, The minimum value of v so that he can cross the road safely is .
2.62ms−1
4.6ms−1
3.57ms−1
1.414ms−1
The correct Answer is:C
Let the man start crossing the roat at an angle θ with the roadside. For safe crossing, the condition is that the man must cross the road by the time the truck describes the destance 4+2cotθ
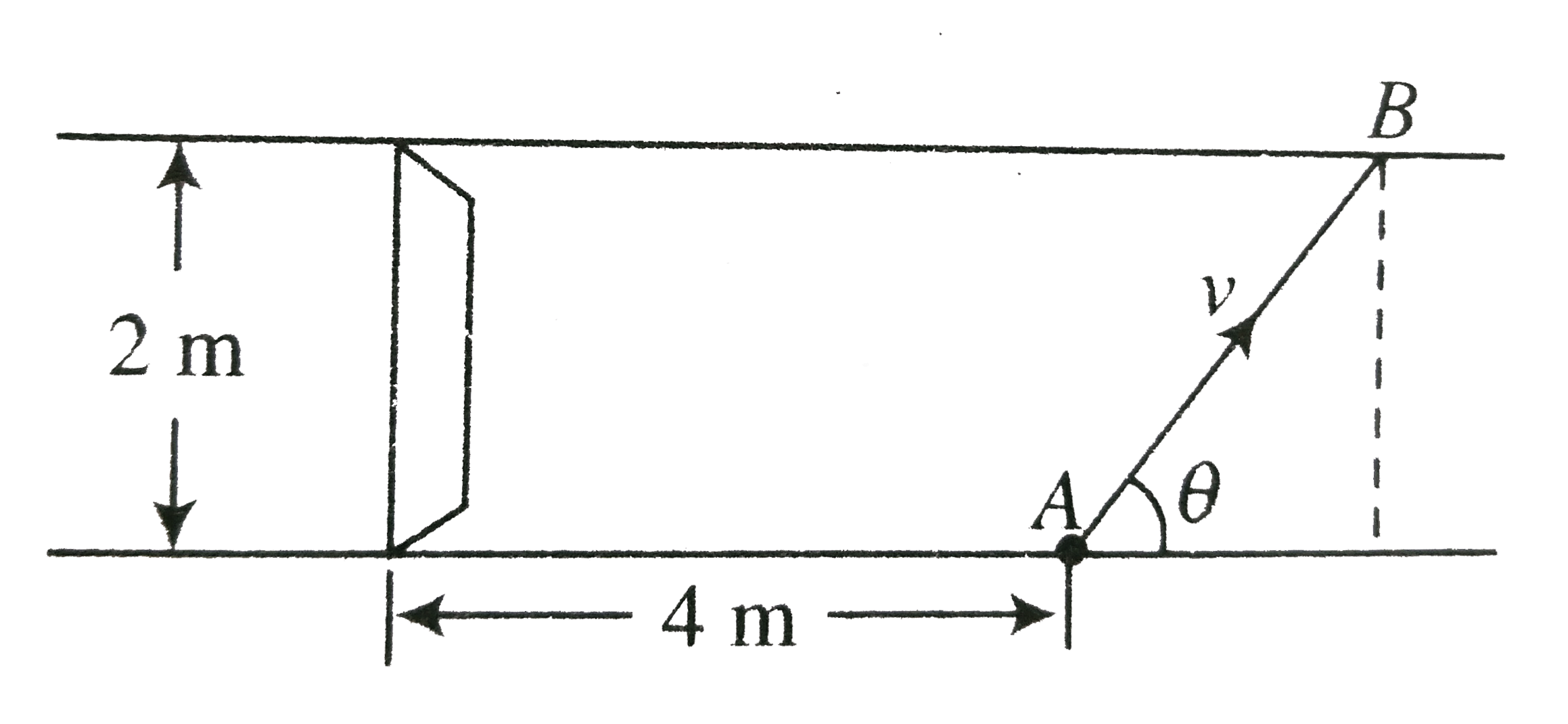 .
.
So, 4+2cotθ8=2/sinθv or v=8(2sinθ+cosθ)
For minimum v,vddθ=0
or (−82cosθ−sinθ((2sinθ+cosθ))2=0 or 2cosθ−sinθ=0
or tanθ=2, so sinθ=2√5,cosθ=1√5
vmin=82(2√50+1√5)=8√5=3.57ms−1.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Graphical Concept17videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Graphical cancept1videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Subjective29videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer9videos
KINEMATICS-2
Similar Questions
A boy is moving with constant velocity v0on a straight road. When he is at a distance d behind the bus, the bus starts from rest and moves with a constant acceleration α. Find the minimum value of v0 so that the boy catches the bus.
View SolutionA truck is moving at a constant speed of 50km/h on a straight road which terminates on a wall. A fly starts moving with a constant speed of 100km/h from the wall towards the truck when the truck is at a distance 25km from the wall. Fly reaches the truck and then turns back towards the wall and then turns back towards the wall and then on reaching the wall it again turns towards the truck and so on. it makes several trips between the truck and the wall, before the truck just reaches the wall.
(a) What is the total distance travelled by the fly during this period?
(b) how many trips the fly makes between the truck and the wall?View Solution
Knowledge Check
- Question 1 - Select One
A 2−m wide truck is moving with a uniform speed v0=8ms−1 along a straight horizontal road. A pedestrian starts to cross the road with a uniform speed v when the truck is 4m away from him, The minimum value of v so that he can cross the road safely is .
A2.62 m/sB4.6 m/sC3.57 m/sD1.414 m/s - Question 2 - Select One
A particle is moving at uniform speed 2ms−1 along a circle of radius 0.5m.The centripetal acceleration of particle is
A1ms−2B2ms−2C4ms−2D8ms−2 - Question 3 - Select One
A car is moving along a straight horizontal road with a speed v0 . If the coefficient of friction between the tyre and the road is μ, the shortest distance in which the car can be stopped is.
Av202μgBv0μgC(v0μg)2Dv0μ
A boy is standing on a truck moving with a constant velocity of 15m/s on the horizontal road. The boy throws a ball in such a way that it returns to the truck after the truck has moved 60m. Find the speed and the angle of projection (a) as seen by the truck and (b) as seen from the road.
View SolutionA car is moving along a straight horizontal road with a speed v0 . If the coefficient of friction between the tyres and the road is μ , the shortest distance in which the car can be stopped is
View SolutionA car starts from rest on a 1600m long bridge. The coefficient of friction between the tyre and road is 0.8. The minimum time taken by the car to cross the bridge is
View SolutionFigure shows a body of mass m moving with a uniform speed v along a circle of radius r . The change in velocity in going from A to B is
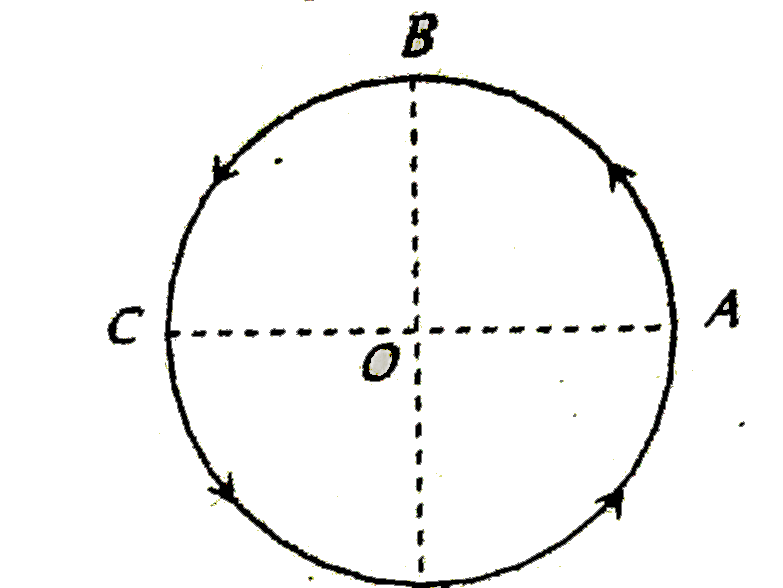 View Solution
View SolutionTwo cars are moving with speed v1,v2 towards a crossing along two roads. If their distance from the crossing be 40 metres and 50 metres at an instant of time then they do not collide if their speed are such that
View Solution

