A particle is thrown with velocity u at an angle ∝ from the horizontal. Another particle is thrown with the same velocity at an angle ∝ from the verticle. What will be the ratio of times of flight of two particles ?
To solve the problem of finding the ratio of the times of flight of two particles thrown with the same velocity u, one at an angle α from the horizontal and the other at an angle α from the vertical, we can follow these steps:
Step 1: Determine the time of flight for the first particle
The time of flight T1 for a projectile thrown at an angle α from the horizontal is given by the formula:
T1=2usinαg
where:
- u is the initial velocity,
- g is the acceleration due to gravity.
Step 2: Determine the angle for the second particle
For the second particle, which is thrown at the same velocity u but at an angle α from the vertical, we need to find the equivalent angle from the horizontal. The angle from the horizontal is:
θ=90∘−α
Step 3: Determine the time of flight for the second particle
Now, we can calculate the time of flight T2 for the second particle using the angle θ:
T2=2usin(90∘−α)g
Using the trigonometric identity sin(90∘−α)=cosα, we can rewrite this as:
T2=2ucosαg
Step 4: Calculate the ratio of times of flight
Now, we can find the ratio of the times of flight T1T2:
T1T2=2usinαg2ucosαg
The 2u and g cancel out:
T1T2=sinαcosα=tanα
Final Answer
Thus, the ratio of the times of flight of the two particles is:
T1T2=tanα
---
The time of flight T1 for a projectile thrown at an angle α from the horizontal is given by the formula:
- u is the initial velocity,
- g is the acceleration due to gravity.
For the second particle, which is thrown at the same velocity u but at an angle α from the vertical, we need to find the equivalent angle from the horizontal. The angle from the horizontal is:
Now, we can calculate the time of flight T2 for the second particle using the angle θ:
Now, we can find the ratio of the times of flight T1T2:
Thus, the ratio of the times of flight of the two particles is:
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.225videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.312videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Solved Examples7videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer9videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression2videos
KINETIC THEORY OF GASES
Similar Questions
A particle P is projected with velocity u1 at an angle of 30∘ with the horizontal. Another particle Q is thrown vertically upwards with velocity u2 from a point vertically below the highest point of path of P. Determine the necessary condition for the two particles to collide at the highest point.
 .View Solution
.View SolutionA particle is thrown with speed u of an angle theta with the horizontal when the particle makes an angle phi with the horizontal its speed changes to v
View Solution
Knowledge Check
- Question 1 - Select One
A particle is thrown up with a ccrtain velocity and at an angle 0 with the horizontal. The variation of kinetic energy with time is given by :
A B
B C
C D
D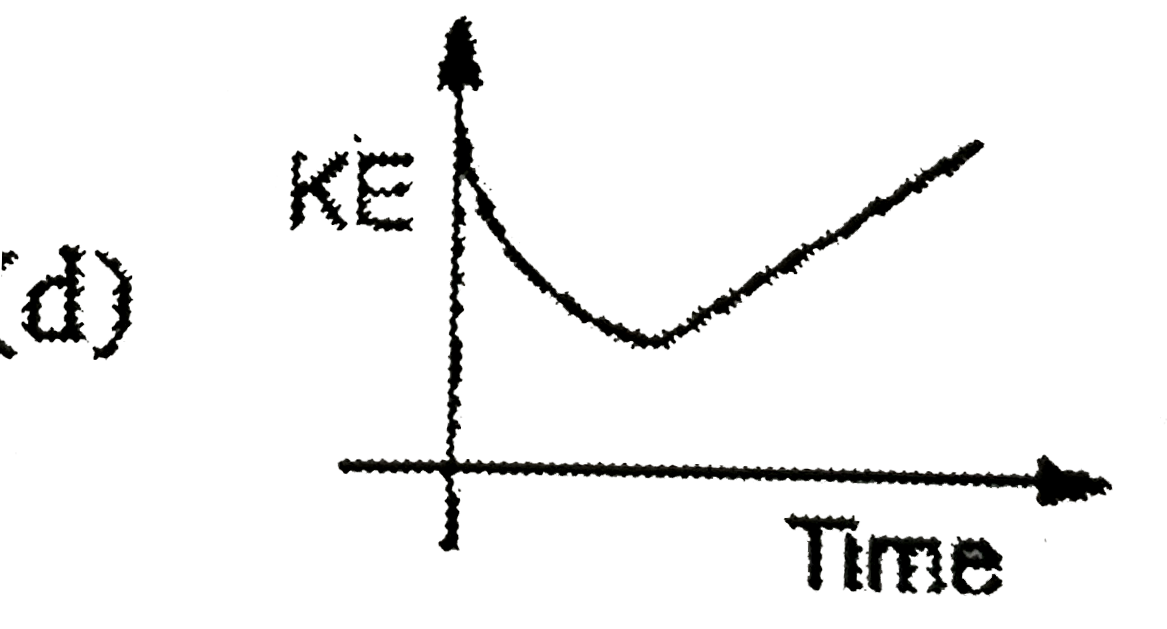
- Question 2 - Select One
A particle is thrown with a speed u at an angle θ with the horizontal. When the particle makes an angle ϕ with the horizontal. Its speed changes to v :
Av=ucosθBv=ucosθ.cosϕCv=ucosθ.secϕDv=usecθ.cosϕ - Question 3 - Select One
If a body A of mass M is thrown with velocity V at an angle of 30∘ to the horizontal and another body B of the same mass is thrown with the same speed at an angle of 60∘ to the horizontal. The ratio of horizontal range of A to B will be
A1:3B1:1C1:√3D√3:1
A particle is projected form ground with velocity u ar angle θ from horizontal. Match the following two columns.
 View Solution
View SolutionA particle is thrown with speed u at an angle α with horizontal from the ground. After how much time, the velocity of particle will make an angle β with horizontal.
View SolutionA body A of mass m is thrown with velocity υ at an angle of 30∘ to the horizontal and another body B of the same mass is thrown with velocity υ at an angle of 60∘ to the horizontal, find the ratio of the horizontal range and maximum height of A and B.
View SolutionA particle is thrown with the speed u at an angle α with the horizontal. When the particle makes an angle β the horizontal , its speed will be
View SolutionAll the particles thrown with same initial velocity would strike the ground.
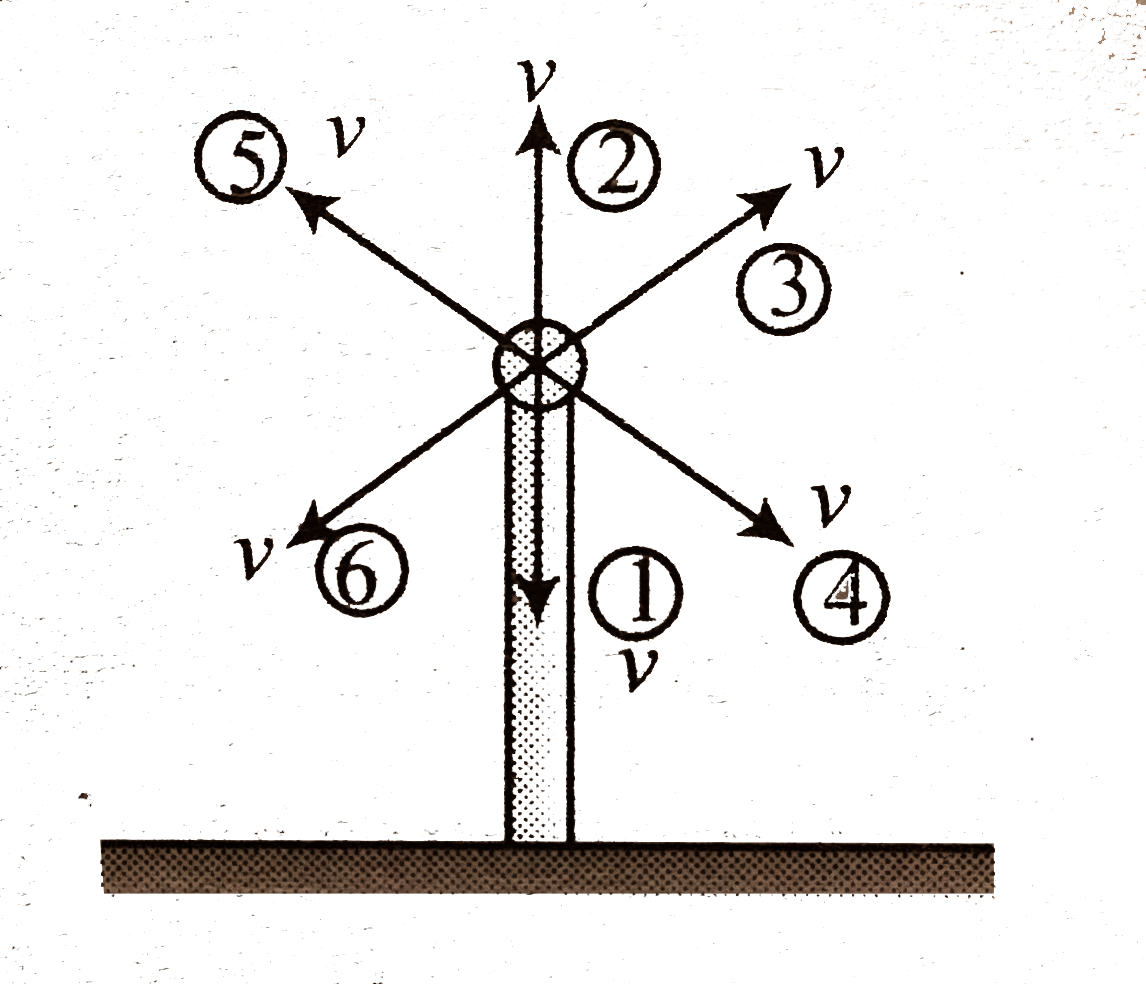 .View Solution
.View Solution

