A plank of mass m rests symmetrically on two wedges B and C of mass M. What is the acceleration of the plank? Neglect friction between all the contact surfaces.

When the plank is released, let it fall through a distance and let both the wedges move through a distance x.
(i)y=xtanθ...(i)
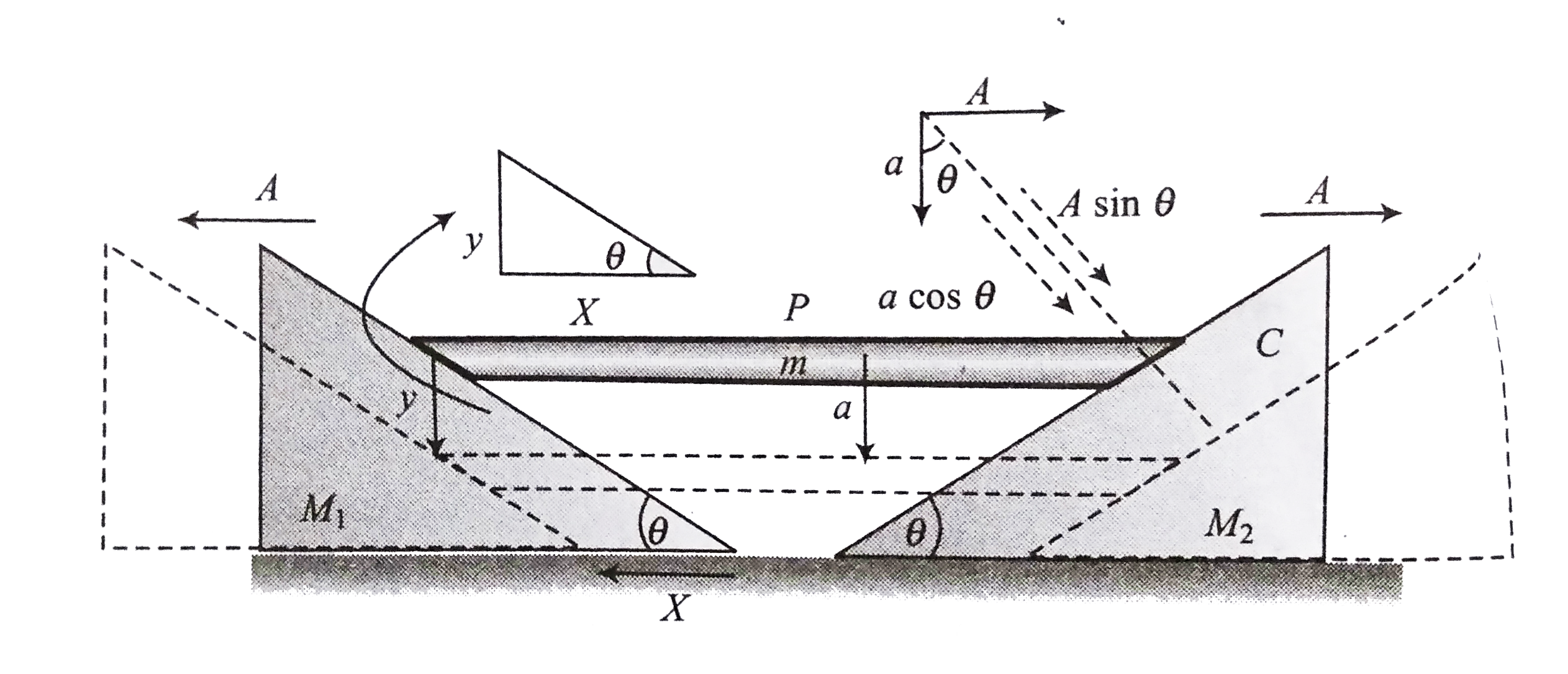
On differnetiating this expression twice, we obtain a=Atanθ FBD of rod and wedge
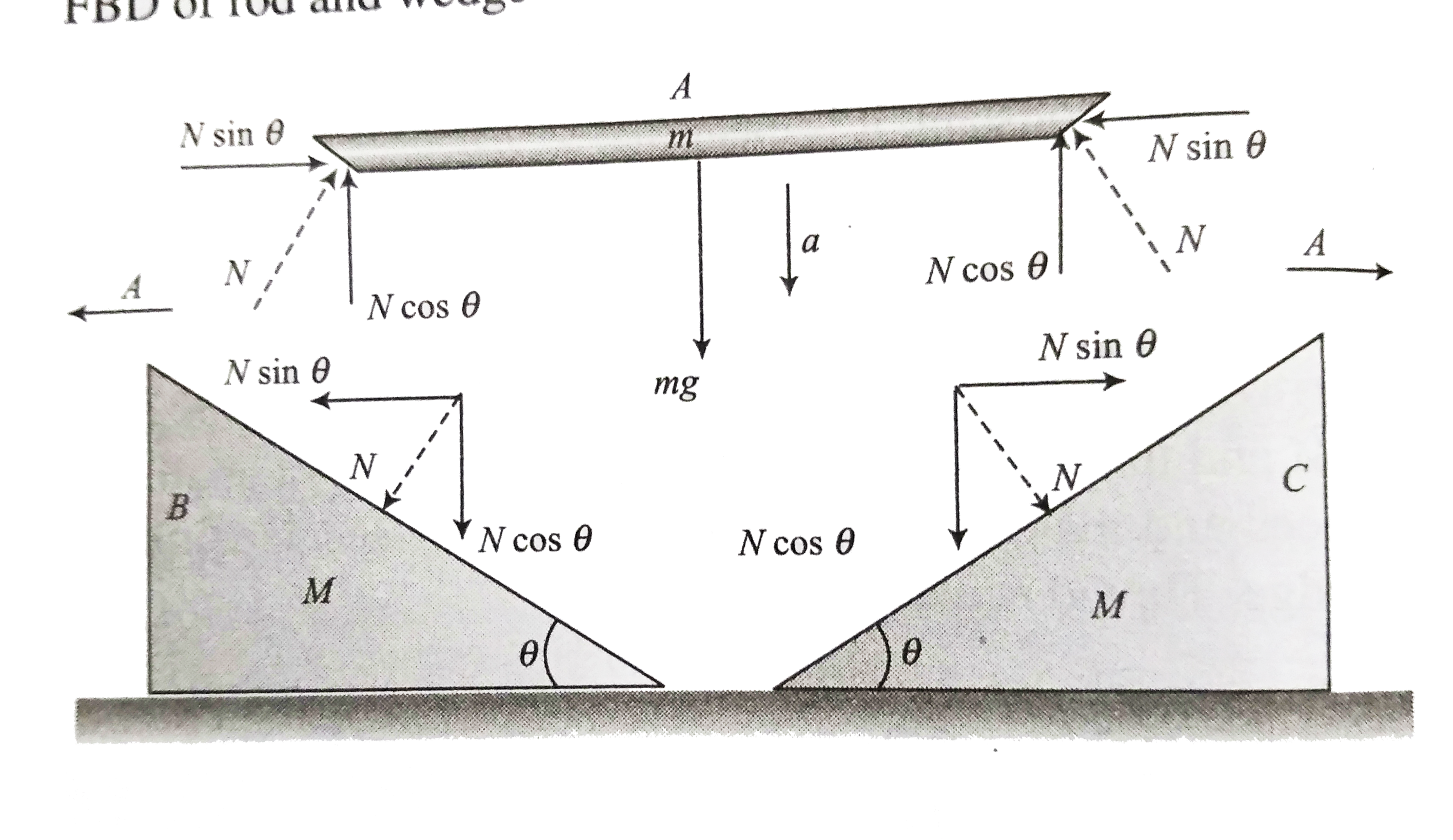
Equation of wedge
ΣFx=Nsinθ=MA ...(ii)
ΣFy=N'−Ncosθ−Mg=0 ...(iii)
Equations of plank:
ΣFx=Nsinθ−Nsinθ=0....(iv)
ΣFy=mg−2Ncosθ=ma ...(v)
From (ii), N=MAsinθ
On substituting expression for N and a in (v), we obtain
mg−2Macosθsinθ=mAsinθcosθ
⇒A=mgsinθcosθmsin2θ+2Mcos2θ.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Solved Examples11videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.111videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
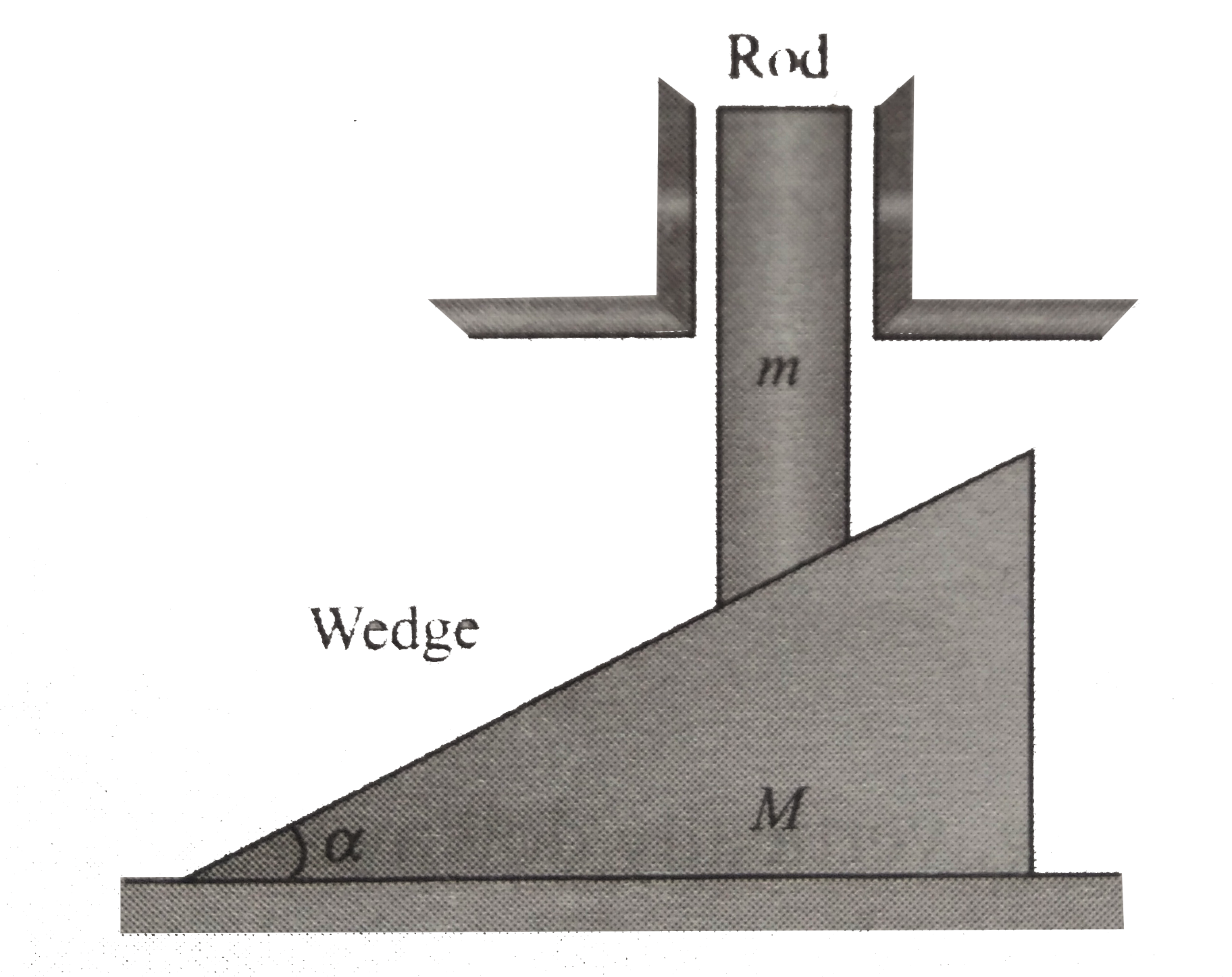
A rod of mass m is supported on a wedge of mass M shown in fig. Find the accelerations of rod a and wedge A in the arrangment. The friction between all contact surfaces in negligible.
 View Solution
View SolutionFind the acceleration of rod A and wedge B in the arrangement shown in figure if the mass of rod equal that of the wedge and the friction between all contact surfaces is negligible Take angle of wedge as 45∘&g=9.8m/s2
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A plank of length 20m and mass 1kg is kept on a horizontal smooth surface. A cylinder of mass 1lg is kept near one end of the plank. The coefficient of friction between the two surface is 0.5. The plank is suddenly given a velocity 20m/s towards left
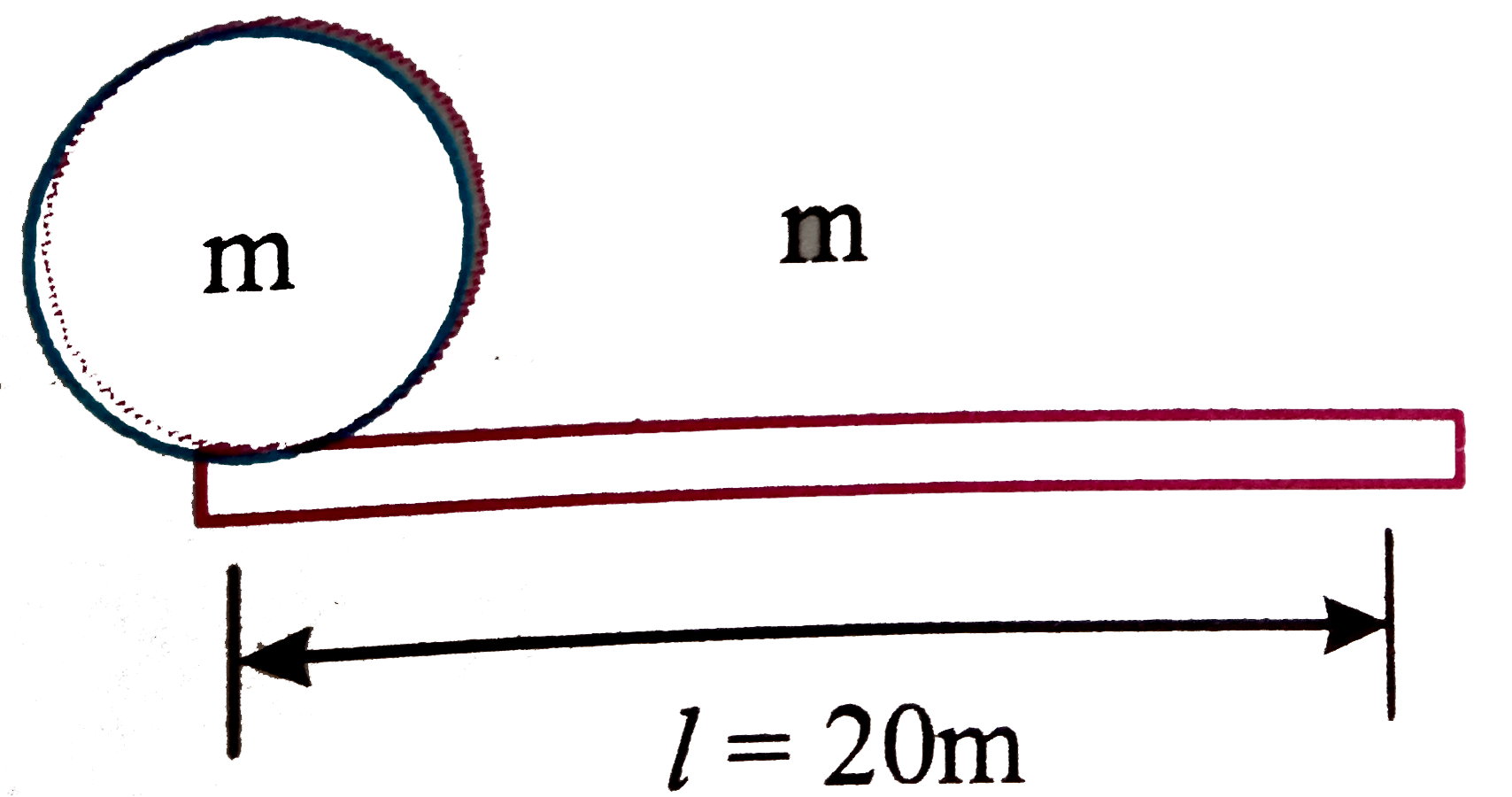
Which of the following statements is correct?Apure rolling of cylinder takes place immediatelyBinitially cylinder slips and then pure rolling beginsCpure rolling never beginsDthere is no lose in kinetic energy during its entire motion. - Question 2 - Select One
A plank of length 20m and mass 1kg is kept on a horizontal smooth surface. A cylinder of mass 1lg is kept near one end of the plank. The coefficient of friction between the two surface is 0.5. The plank is suddenly given a velocity 20m/s towards left
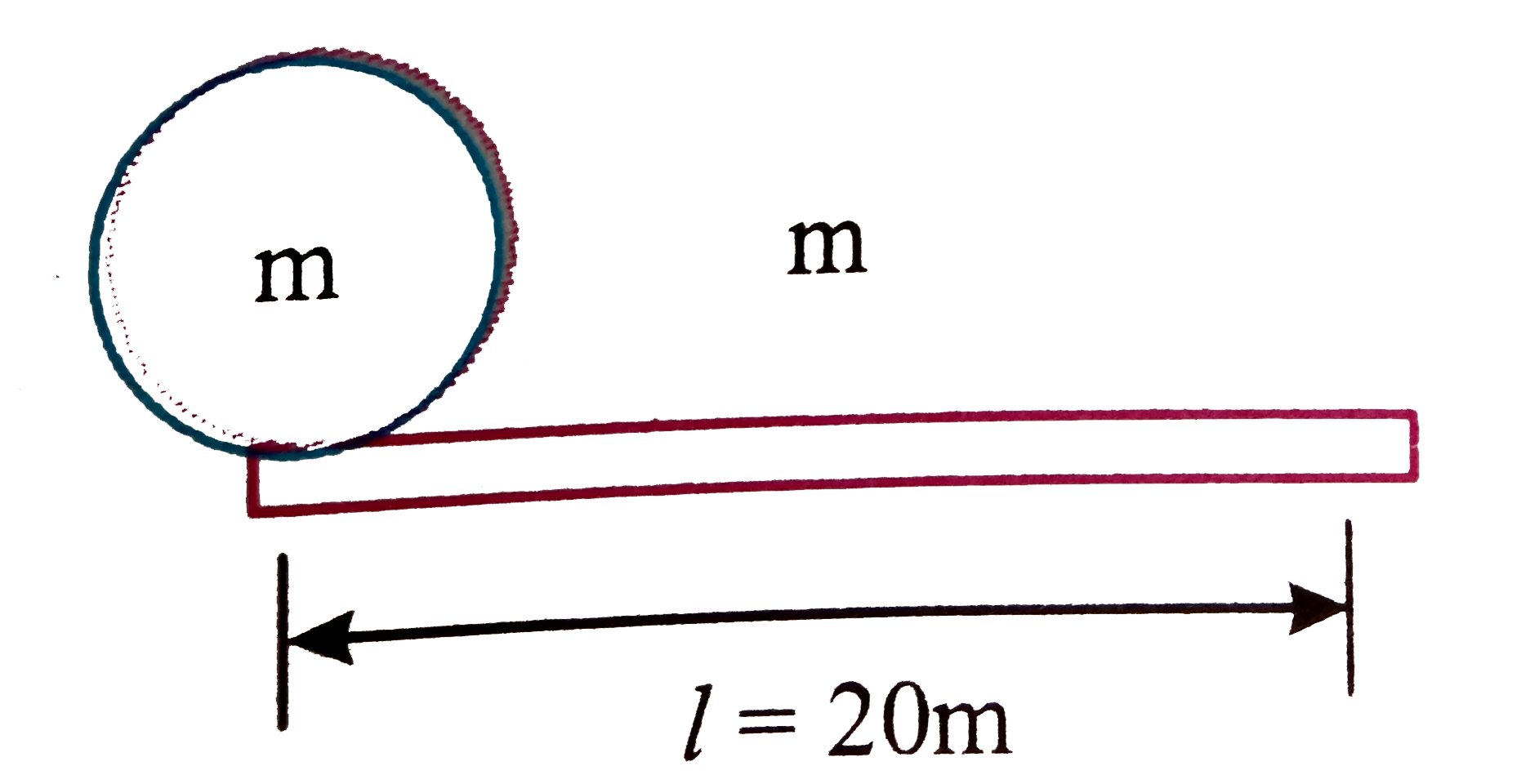
Which of the following statements is correct ?Ainitial acceleration of cylinder is 5m/s2 towards leftBinitial acceleration of cylinder is 5m/s2 towards rightCinitial acceleration of cylinder is 10m/s2 towards rightDinitial acceleration of cylinder is 10m/s2 - Question 3 - Select One
In fig. mass m is being pulled on the incline of a wedge of mass M. All the surfaces are smooth. Find the acceleration of the wedge.
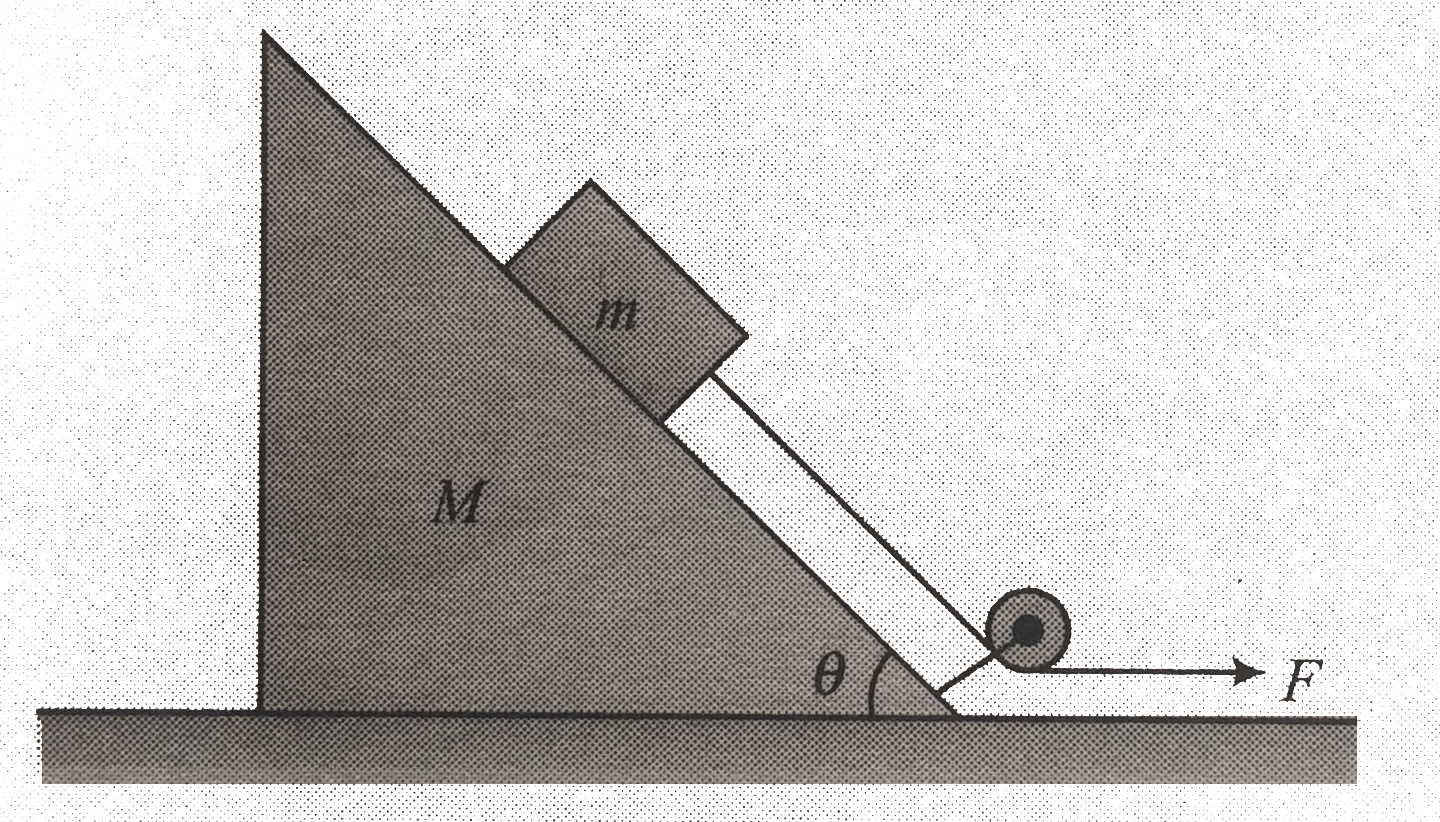 Aa=F(1−cosθ)mg2sinθcosθM+2msin2θBa=F(1+cosθ)+mgsinθcosθM+msin2θCa=F(1−cosθ)−mgsinθcosθM+msin2θDa=F(1+cosθ)−mgsinθcosθM−msin2θ
Aa=F(1−cosθ)mg2sinθcosθM+2msin2θBa=F(1+cosθ)+mgsinθcosθM+msin2θCa=F(1−cosθ)−mgsinθcosθM+msin2θDa=F(1+cosθ)−mgsinθcosθM−msin2θ
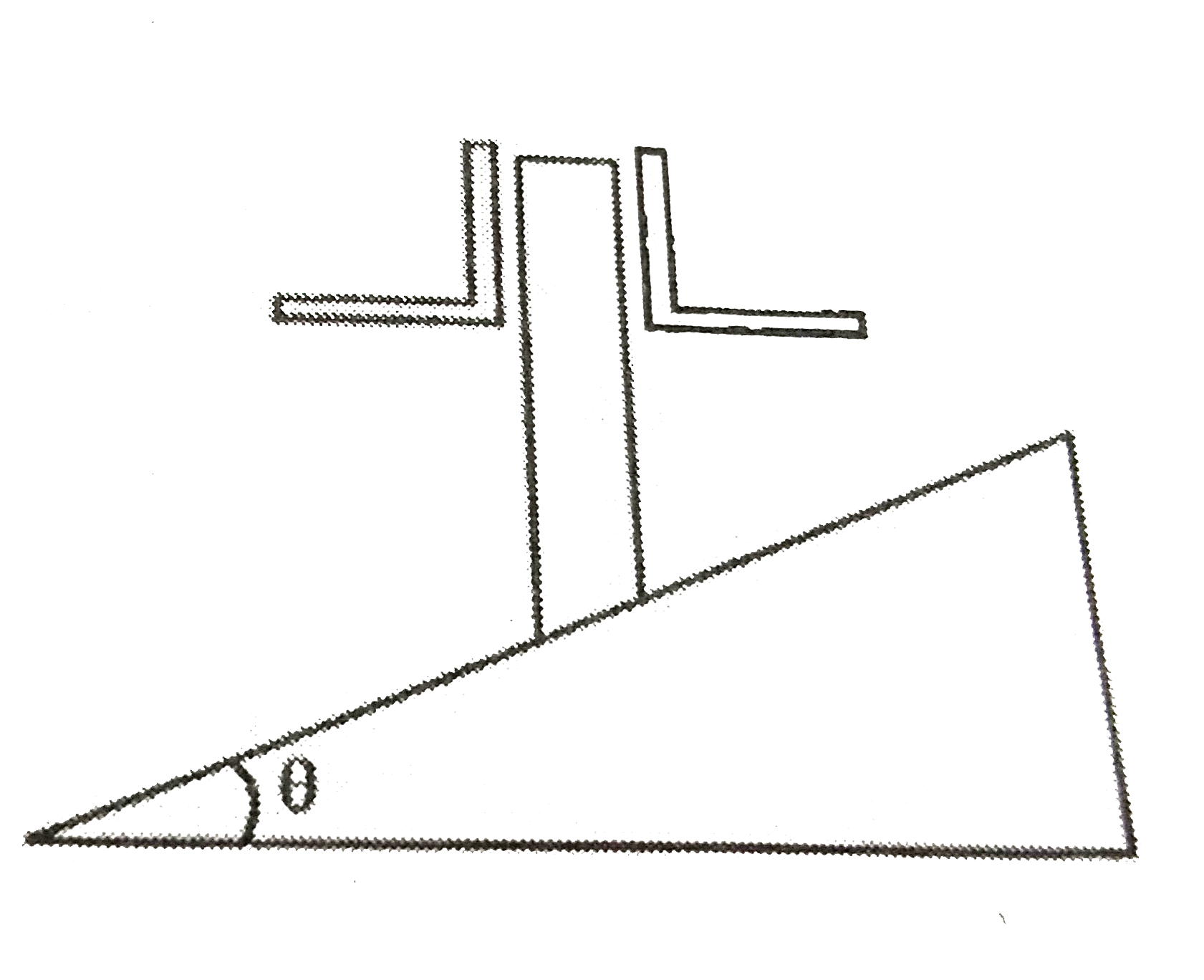
Find the accelerations of rod A and wedge B in the arrangement shown in figure if the ratio of the mass of the wedge to that of the rod equals η, and the friction between all contact surfaces is negligible.
 View Solution
View SolutionFind the acceleration of rod A and wedge B in the arrangement shown in fig if the ratio of the mass of wedge to that of the rod equals η and the friction between the contact surfaces are negligible.
 View Solution
View SolutionA rod 'A' constrained to move in vertical direction rests on a wedge B, as shown in the Fig. Find the accelerations of rod A and wedge B instantaneously after system is released from rest, neglecting friction at all the contact surfaces.
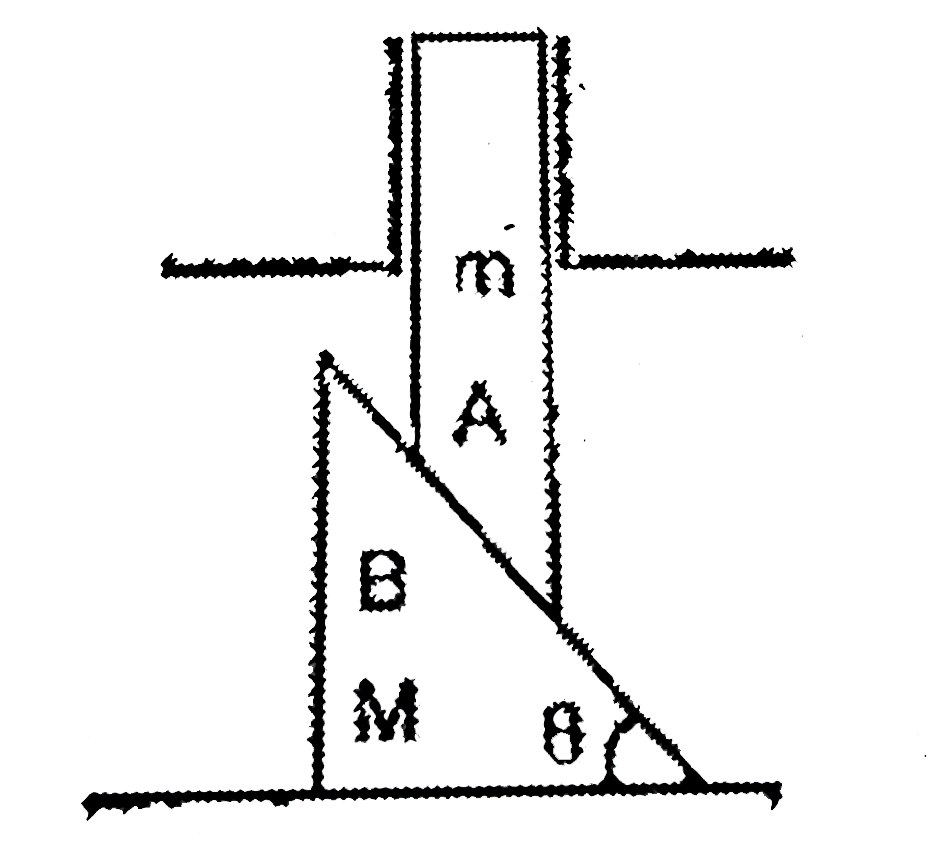 View Solution
View SolutionA bar of mass m1 is placed on a plank of mass m2 which rests on a smooth horizontal plane . The coefficient of friction between the surfaces of bar and plank is k . The plank is subjected to a horizontal force F depending on time t as F = at , where a is a constant . The moment of time t0 at which the plank starts sliding is :
 View Solution
View SolutionA plank of mass 3m is placed on a rough inclined plane and a man of mass m, the coefficient of friction between the board and inclined plane is μ=0.5, the minimum acceleration of man so that plank does not slide is :
 View Solution
View Solution

