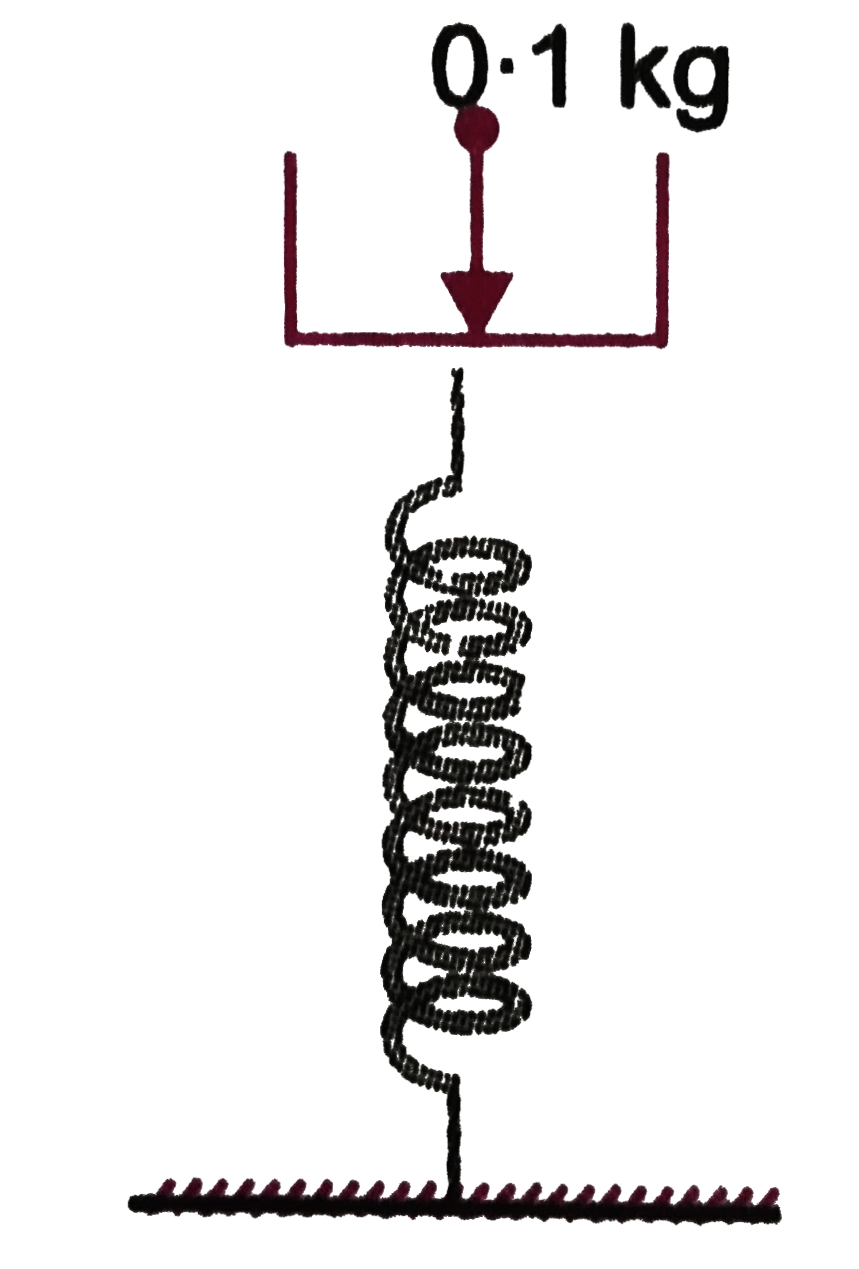
A massless platform is kept on a light elastic spring as shown in figure. When a small stone of mass 0.1 kg is dropped on the pan from a height of 0.24 m, the spring compresses by 0.01m. From what height should the stone be droppped to cause a compression of 0.04m in the spring ?
0.96m
2.96m
3.96m
0.48m
The correct Answer is:C
Let the particle be dropped from a height h and the spring be compressed by y. According to the conservation of mechanical energy, loss in PE of the particle=gain in elastic potential energy of the spring.
mg(h+y)=12ky2
Now, as the particle and spring are same for second case,
h1+y1h2+y2=(y1y2)2 or (0.24+0.01h2+0.04)=(0.010.04)2
Solving, we get h2=3.96m
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Multiple Correct25videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension55videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Subjective23videos
WORK, POWER & ENERGY
- BOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
Similar Questions
A platform having no mass is kept on a light elastic spring . When a small particle of 0.1 kg is dropped on this spring from a height of 0.50 m , the spring is compressed by 0.02 m . From which height the particle should be dropped to produce a compression of 0.05 m ?
View SolutionGiven figure shows a massless platform kept on a light elastic spring attached to ground from one end .
A small particle of mass 0.2 kg is dropped on the paltform from a height of 0.26 m , which causes a compression of 0.01 m in the spring . Calculate the height from which the particle must be dropped in order to cause a compressin of 0.05 m .View Solution
Knowledge Check
- Question 1 - Select One
A massless platform is kept on a light elastic spring, as shown in the figure. When particle of mass 0.1 kg is dropped on the pan from a height of 0.24 m, the particle strikes the pan, and the spring is compressed by 0.01 m. From what height should the particle be dropped to cause a compression of 0.04 m?
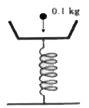 A0.96 mB2.96 mC3.96 mD0.48 m
A0.96 mB2.96 mC3.96 mD0.48 m - Question 2 - Select One
The two masses m1 and m2 are joined by a spring as shown. The system is dropped to the ground from a height. The spring will be
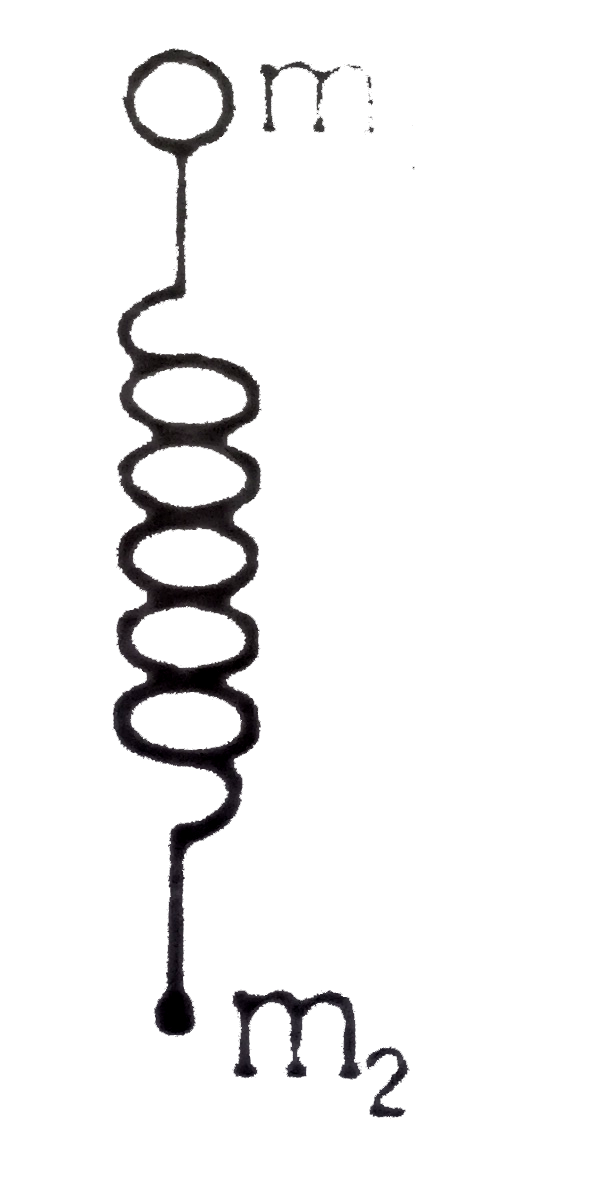 AStreched when m2>m1BCompressed when m2<m1Cneither compressed not stretched only when m1=m2Dneither compressed not stretched regardless of the values of m1 and m2.
AStreched when m2>m1BCompressed when m2<m1Cneither compressed not stretched only when m1=m2Dneither compressed not stretched regardless of the values of m1 and m2. - Question 3 - Select One
When a spring is compressed by 0.05 m a restoring force of 10 N is developed in it. Then the force constant of the spring is
A50 N/mB100 N/mC150 N/mD200 N/m
The block shown in figure is released from rest. Find out the speed of the block when the spring is compressed by 1 m
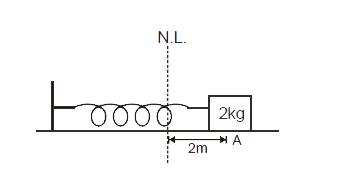 View Solution
View SolutionA spring gets compressed when a body of mass 5 g is dropped on it from a height of 2 m . Calculate the maximum distance s through whichthe spring is compressed. The force constant of spring is 100Nm−1 .
View SolutionA block of mass 180g is placed on a spring (spring constant k=120N/m) fixed from below. A ball of mass 20g is dropped from height 20m and the collision is completely inelastic. Find the maximum compression of the spring. Neglect the initial compression of the spring due to the block.
View SolutionA 1.0 kg block moving at 8.0 m/s strikes a spring fixed at one end to a wall and compresses the spring by 0.40 m, where its speed gets reduced to 2.0 m/s. After this event, the spring is mounted upright by fixing its bottom end to afloor, and a stone of mass 2.0 kg is placed on it, the spring is now compressed by 0.50 m from its rest length. The system is then released. How far above the rest-length point does the stone rise ?
View SolutionA block of 200 g mass is dropped from a height of 2 m on to a spring and compress the spring to a distance of 50 cm. The force constant of the spring is
View Solution

