One end of a spring of force constant is attached to the ceiling of an elevator. A block of mass is attached to the other end. Another spring of force constant is attached to the bottom of the mass and to the floor of the elevator as shown in figure. At equilibrium, the deformation in both the spring is equal and is . If the elevator moves with constant acceleration upward, the additional deformation in both the spring is . Find the elevator's accelerationn ( ).
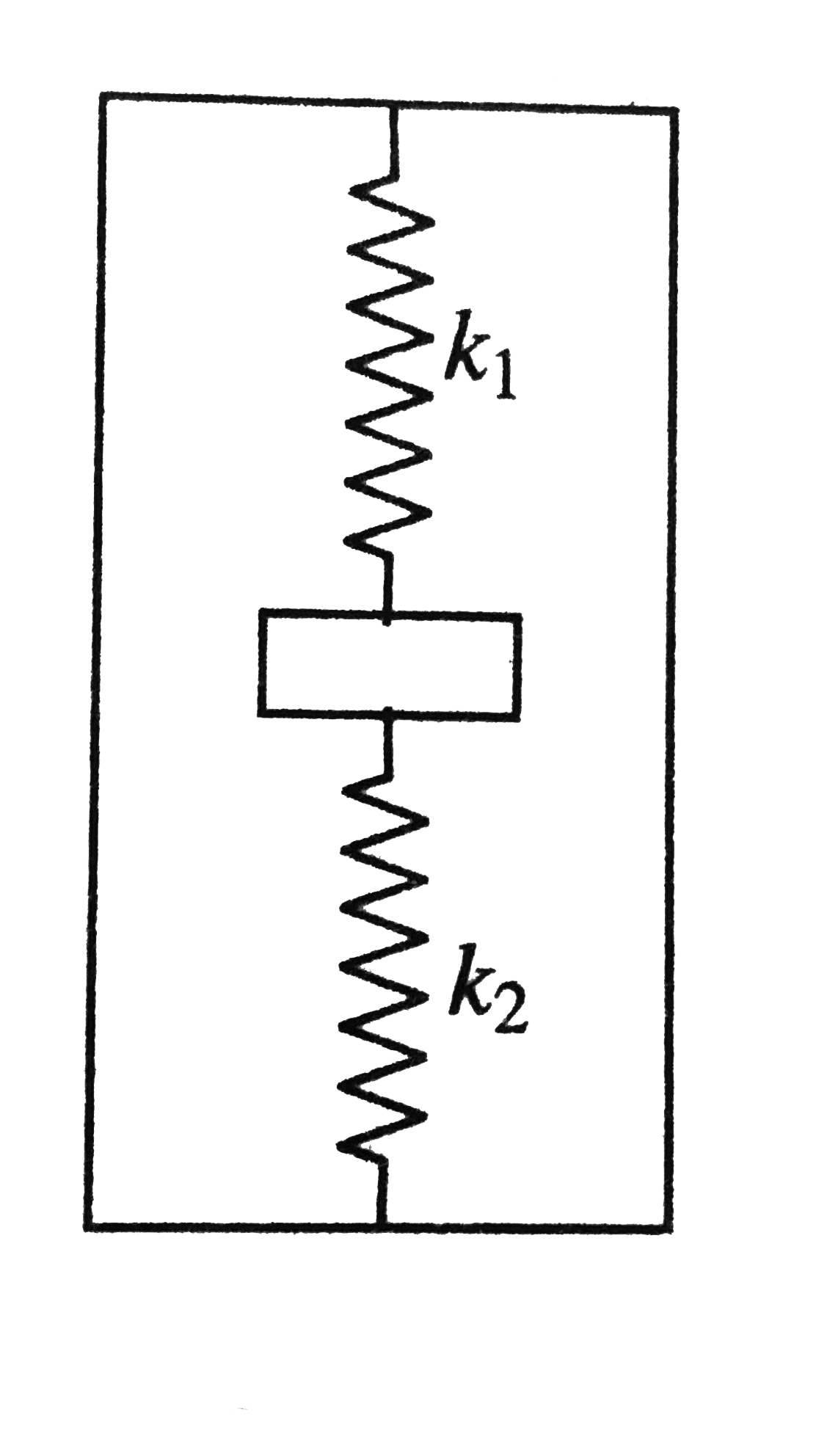
The correct Answer is:
At equilibrium,
(i)
In case of constant acceleration,
(ii)
From Eqs. (i) and (ii), we get
Topper's Solved these Questions
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Archives (sinble Correct )
Explore 19 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Archives(multiple Correct)
Explore 3 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICSChapter:WORK, POWER & ENERGYExercise:Linked Comprehension
Explore 55 Videos
- VECTORSBook:CENGAGE PHYSICSChapter:VECTORSExercise:Exercise Multiple Correct
Explore 5 Videos
Similar Questions
A sping of spring constant k is attached to the ceiling. A block of mass M is attached to its lower end and is released suddenly.
(ii) Find its speed at the instant it passes throught the equilibrium position.
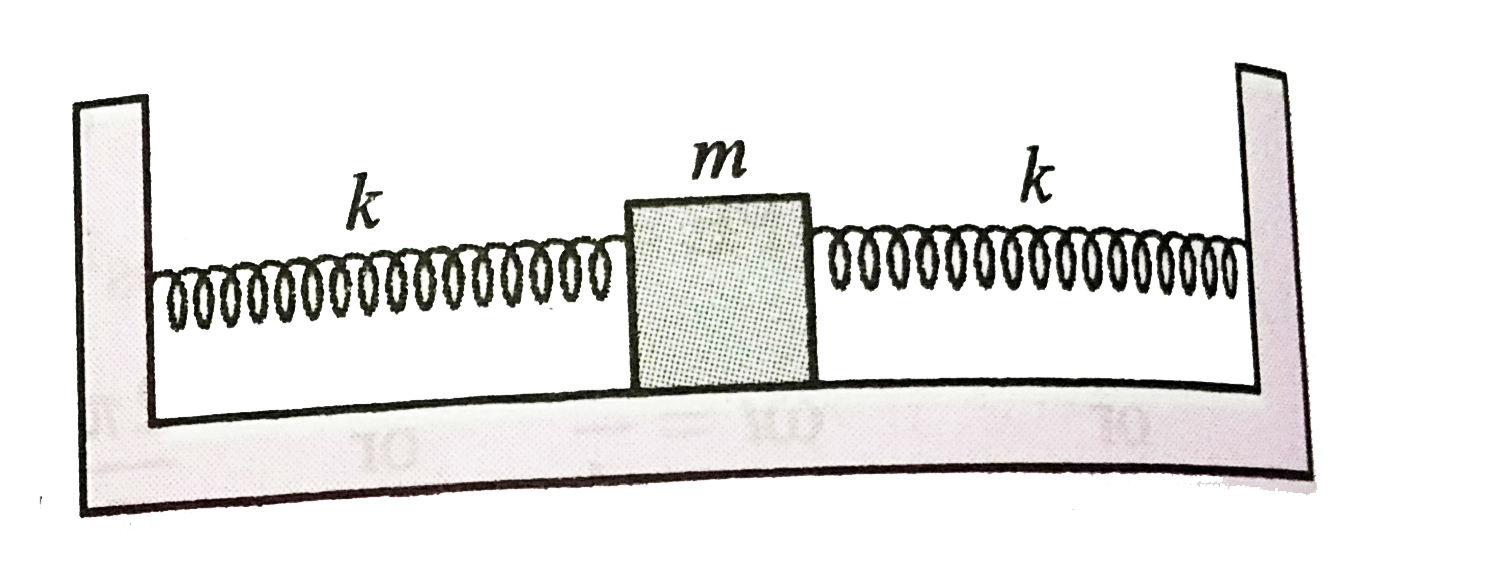
View SolutionTwo identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in the figure. The time period of oscillation is

View Solution
Knowledge Check
A block of mass m is attached to a massless spring of force constant k, the other end of which is fixed from the wall of a truck as shown in figure. The block is placed over a smooth surface and initially the spring is unstretched. Suddenly the truck starts moving towards right with a constant accleration a0. As seen from the truck
 AThe particle will execute SHMBThe time period of oscillations will be 2π√mk(Cthe amplitude of oscillations will be (m2a20)DThe energy of oscillatioins will be m2a20k
AThe particle will execute SHMBThe time period of oscillations will be 2π√mk(Cthe amplitude of oscillations will be (m2a20)DThe energy of oscillatioins will be m2a20kAn ideal spring with spring constant k is hung from the ceiling and a block of mass M is attached to its lower end. The mass is released with the spring initially unstretched. Then the maximum extension in the spring is
A(a) 4MgkB(b) 2MgkC(c) MgkD(d) Mg2kA block of mass m is attached to a massless spring of force constant k, the other end of which is fixed from the wall of a truck as shown in the figure. The block is placed over a smooth surface and initially the spring is unstretched. Suddenly the truj starts moving towards right with a constant acceleration a0. If the block is observed from the truck
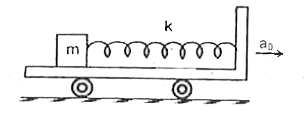 Athe block will execute SHMBthe time period of oscillations of block will be 2π√mkCthe amplitude of oscillation of block will be ma0kDthe energy of oscillations of block will be m2a20k
Athe block will execute SHMBthe time period of oscillations of block will be 2π√mkCthe amplitude of oscillation of block will be ma0kDthe energy of oscillations of block will be m2a20k
Two identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrium position by a distance x towards right, find the restoring force.
View SolutionA block of mass 1kg is kept on smooth floor of a truck. One end of a spring of force constant 100N/m is attached to the block and other end is attached to the body of truck as shown in the figure. At t=0, truck begins to move with constant acceleration 2m/s2. The amplitude of oscillation of block relative to the floor of truck is
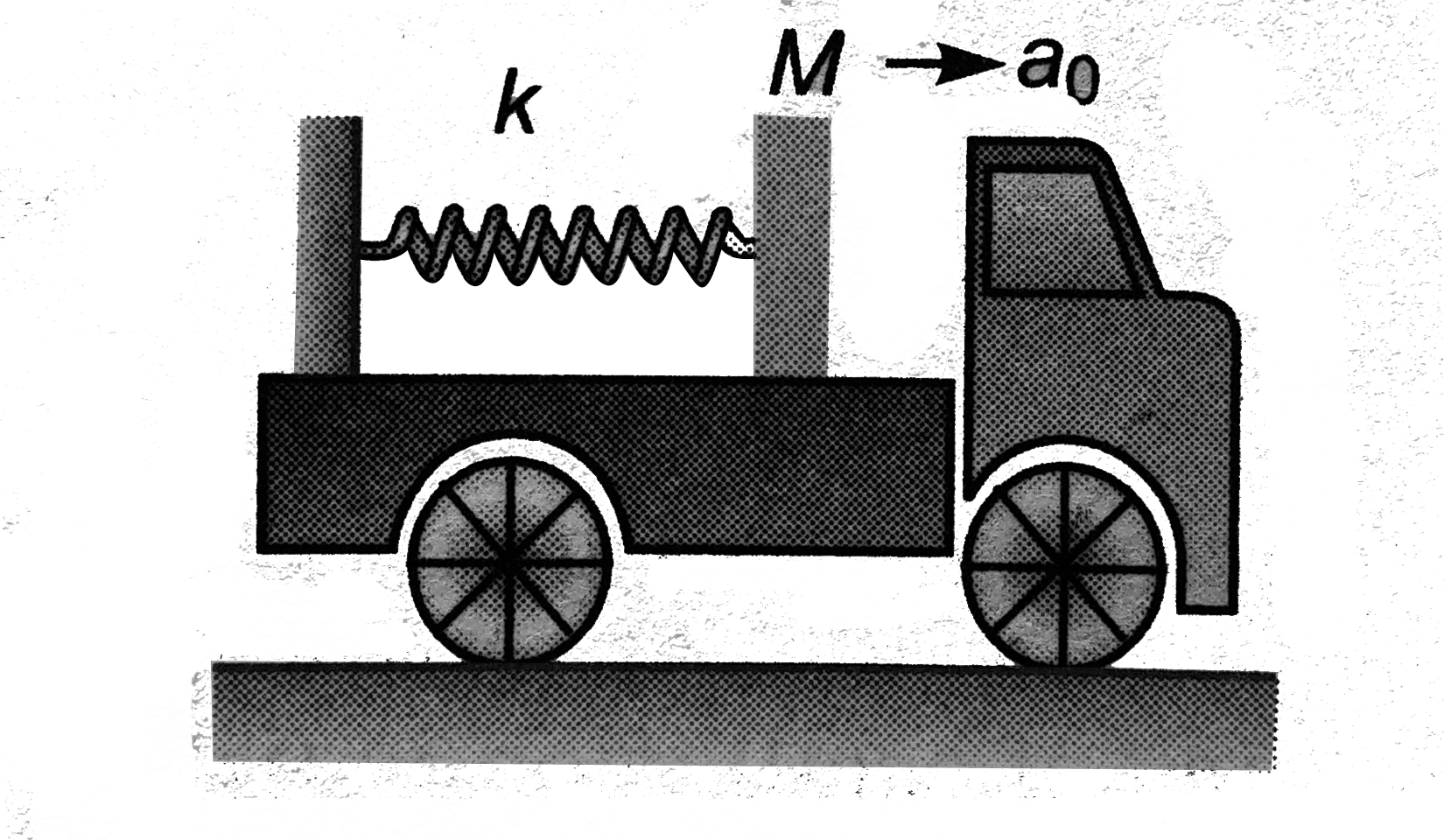
View SolutionAn ideal spring having force constant k is suspended from the rigid support and a block of mass M is attached to its lowe end. The mass is released from the natural length of the spring. Then the maximum exiension in the spring is
View SolutionA spring of force constant K rests on a smooth floor, with one end fixed to a wall. A block of mass m hits the free end of the spring with velocity v. The maximum force exerted by the spring on the wall is
View SolutionA spring of force constant 600Nm−1 is mounted on a horizontal table. A mass of 1.5 kg is attached to the free end of the spring,pulled sideways to a distance of 2 cm and released . The speed of the mass when the spring is compressed by 1 cm is
View Solution