Sound waves are passing through two routes-one in straight path and the other along a semicircular path of radius r and are again combined into one pipe and superposed as shown in the figure. If the velocity of sound waves in the pipe is v, then frequencies of resultant waves of maximum amplitude will be multiples integral of

vr(π−2)
vr(v−1)
2vr(π−1)
vr(π+1)
The correct Answer is:A
Path difference =(πr−2r)=(π−2)r=nλ ...(i)

where, v=f×λ where f= frequency
∴f=v/λ
⇒f=vn(π−2)r Putting λ from (i)
So, multiple integral =[v(π−2)r]
Topper's Solved these Questions
- WAVE MOTIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - WAVE MOTIONEXERCISE - Exercise 2
30 Videos
- WAVE MOTIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - WAVE MOTIONEXERCISE - MHT CET CORNER
23 Videos
- WAVE MOTIONBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - WAVE MOTIONEXERCISE - MHT CET CORNER
23 Videos
- SURFACE TENSION BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - SURFACE TENSION EXERCISE - MHT CET Corner
15 Videos
- WAVE THEORY OF LIGHT BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - WAVE THEORY OF LIGHT EXERCISE - MHT CET CORNER
4 Videos
Similar Questions
A tuning fork of frequency 250 Hz is vibrating at one end of a tube as shown in the figure. If maximum sound is heard at the other end, then the velocity of the waves will be
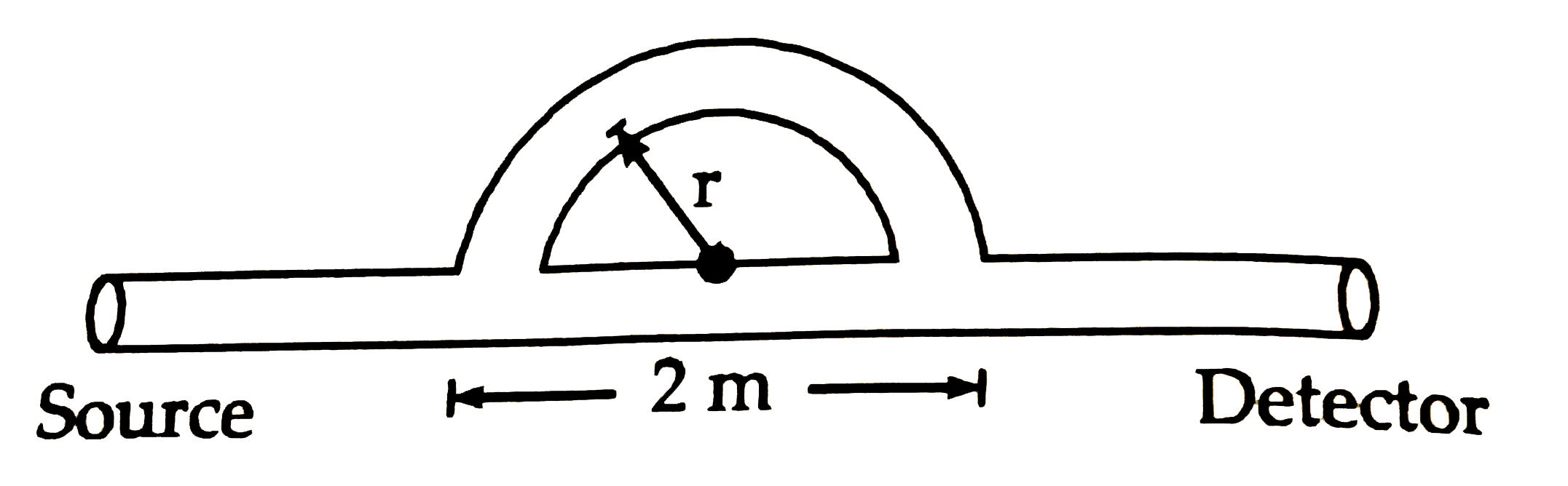
View SolutionWhen two sound waves with a phase difference of π/2, and each having amplitude A and frequency ω , are superimposed on each other, then the maximum amplitude and frequency of resultant wave is
View Solution
Knowledge Check
Sound waves are passing through two routes- one in straight path and the other along a semicircular path of radius r and are again combined into one pipe and superposed as shown in the figure. If the velocity of sound waves in the pipe is y, then frequencies of resultant waves of maximum amplitude will be integral multiples of
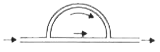 Avr(π−2)Bvr(π−1)C2vr(π−1)Dvr(π+1)
Avr(π−2)Bvr(π−1)C2vr(π−1)Dvr(π+1)When two sound waves with a phase difference of π/2, and each having amplitude A and frequency ω , are superimposed on each other, then the maximum amplitude and frequency of resultant wave is
AA√2:ω2BA√2:ωC√2A:ω2D√2A:ωA sound wave travelling in a medium is represented as shown in the figure.
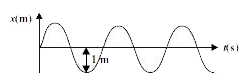
If vibrating source of sound makes 360 oscillations in 2 minutes, then the amplitude, wavelength and frequency of the sound wave are respectively (Take velocity of sound as 342 m s−1)A1 m, 114 m and 3 HzB2 m, 3 m and 14 HzC1 m, 5 m and 20 HzD1 m, 100 m and 10 Hz
The wavelength of a sound wave travelling in a solid becomes one third when it propagates through air. If the frequency of the wave remains constant, then find the decreases in the velocity of the wave
View SolutionThe wavelength of a sound wave travelling in a solid becomes one third when it propagates through air. If the frequency of the wave remains constant, then find the decreases in the velocity of the wave
View SolutionA sound consists of four frequencies : 300Hz,600Hz,1200Hzand2400Hz. A sound 'filter' is made by passing this sound through a bifurcate pipe as shown . The sound wave has to travel a distance of 50cm more in the right branch - pipe than in the straight pipe. The speed of sound in air is 300m/s. Then , which of the following frequencies will be almost completely muffled or 'silenced' at the outlet?

View SolutionTwo sound waves emerging from a source reach a point simultaneously along two paths. When the path difference is 12cm or 36cm, then there is a silence at that point. If the speed of sound in air be 330m/s, then calculate maximum possible frequency of the source.
View SolutionA sound consists of four frequencies : 300Hz,600Hz,1200Hzand2400Hz. A sound 'filter' is made by passing this sound through a bifurcate pipe as shown . The sound wave has to travel a distance of 50cm more in the right branch - pipe than in the straight pipe. The speed of sound in air is 300m/s. Then , which of the following frequencies will be almost completely muffled or 'silenced' at the outlet?

View Solution
