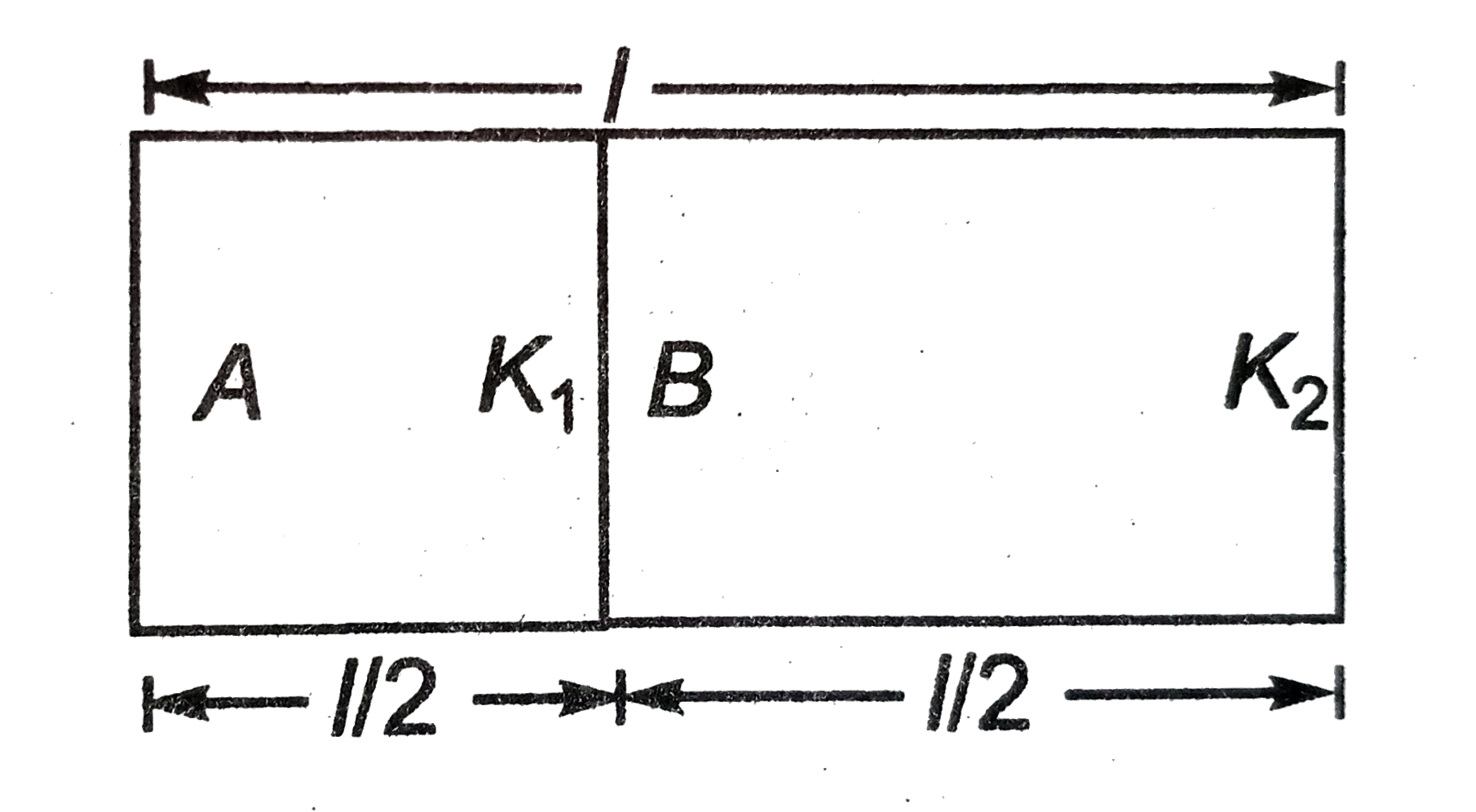
Two slabs A and B of different materials but with the same thickness are joined as Shown in the figure. The thermal conductivites of A and B are $k_{1}$ and $K_{2}$ respectively . The thermal conductivity of the composite slab will be

A

$\frac{1}{2} (K_{1} + K_{2}$

B

$\sqrt{K_{1} K_{2}}$

C

$(K_{1} + K_{2})$

D

$\frac{2 K_{1} K_{2}}{(K_{1} + K_{2})}$

Text Solution

Verified by Experts

The correct Answer is:D

The thermal resistance of a slab of length $l_{1}$ area of cross- section A and thermal conductivity K is given by
$R = \frac{l}{K A}$
for slab $A R_{1} = \frac{l / 2}{K_{1} A} \Rightarrow$ For slab $B R_{2} = \frac{l / 2}{K_{2} A}$
Since , the slabs are joined in series , the thermal resistance of the composite slab is
$R_{c} = R_{1} + R_{2} or \frac{l}{K_{c} A} = \frac{l / 2}{K_{1} A}$
$or \frac{1}{K_{c}} = \frac{1}{2} [\frac{1}{K_{1}} + \frac{1}{K_{2}}] \Rightarrow K_{c} = \frac{2 K_{1} K_{2}}{(K_{1} + K_{2})}$

|

Updated on:21/07/2023

Class 12 PHYSICS KINETIC THEORY OF GASES ANDRADIATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A composite slab consists of two slabs A and B of different materials but of the same thickness placed one on top of the other. The thermal conductivities of A and B are $k_{1}$ and $K_{2}$ respectively. A steady temperature difference of $12^{\circ} C$ is maintained across the composite slab. If $k_{1} = K_{2} / 2$ , the temperature difference across slab A will be:

A $4^{\circ} C$
B $8^{\circ} C$
C $12^{\circ} C$
D $16^{\circ} C$
Question 2 - Select One
Two slabs A and B of different materials but of the same thickness are joined end to end to form a composite slab. The thermal conductivities of A and B are $K_{1}$ and $K_{2}$ respectively. A steady temperature difference of $12^{\circ}$ C is maintained across the composite slab. If $K_{1} = \frac{K_{2}}{2}$ , the temperature difference across slab A is
A $4^{\circ}$ C
B $6^{\circ}$ C
C $8^{\circ}$ C
D $10^{\circ}$ C
Question 3 - Select One
Two rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are $K_{1}$ and $K_{2}$ . The thermal conductivity of the composite rod will be

A $\frac{K_{1} + K_{2}}{2}$
B $\frac{3 (K_{1} + K_{2})}{2}$
C $K_{1} + K_{2}$
D $2 (K_{1} + K_{2})$

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-KINETIC THEORY OF GASES ANDRADIATION-Exercise 2

Home
Ask Doubt
Profile