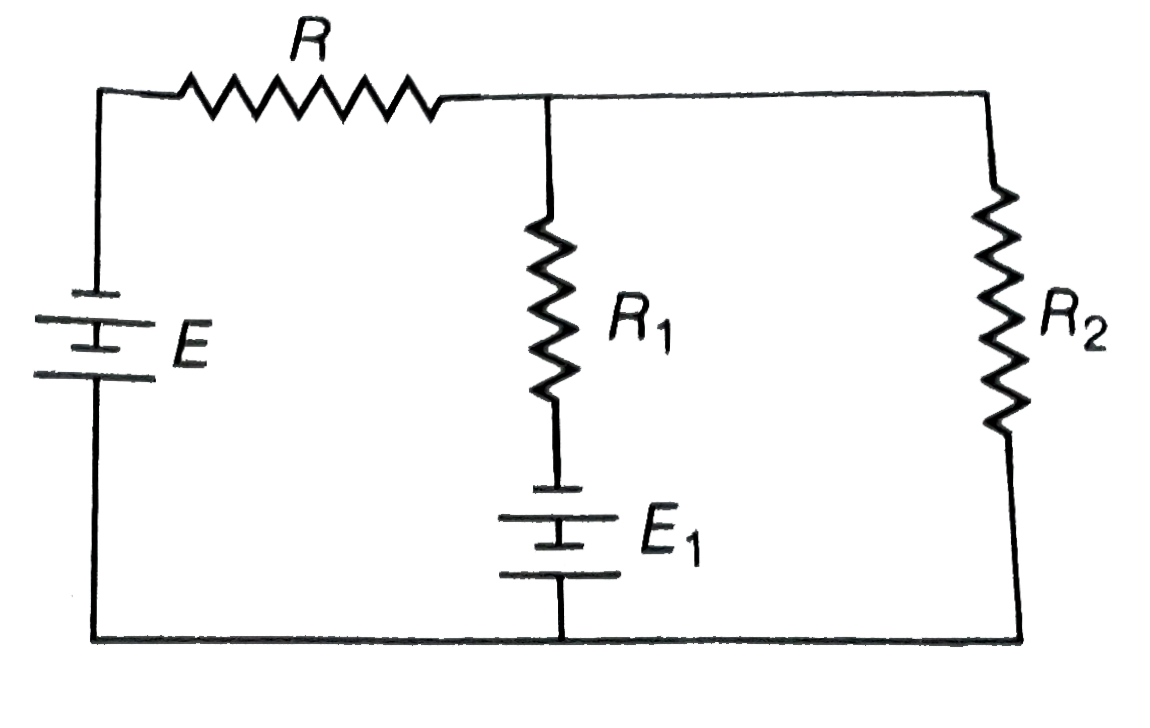
Figure shows a circuit with known resistances and . Neglect the internal resistance of the sources of current and resistance of the connecting wire . The magnitude of electromotive force E, such that the current through the resistance R is zero will be
A
B
C
D
Text Solution
The correct Answer is:C
Current through resistance R will be zero, if
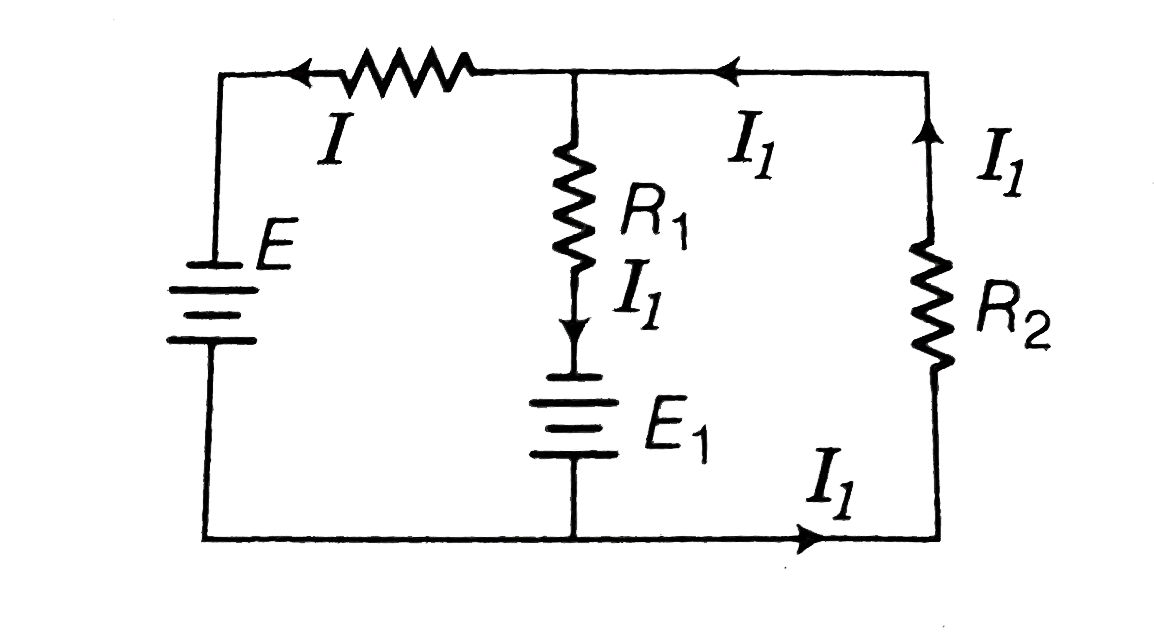
Since,
or
|
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE 2
26 Videos
- CURRENT ELECTRICITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CURRENT ELECTRICITYEXERCISE - MHT CET CORNER
32 Videos
- CURRENT ELECTRICITYBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - CURRENT ELECTRICITYEXERCISE - MHT CET CORNER
32 Videos
- COMMUNICATION SYSTEMBOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - COMMUNICATION SYSTEMEXERCISE - MHC CET Corner
6 Videos
- ELASTICITY BOOK - MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERSCHAPTER - ELASTICITY EXERCISE - MHT CET CORNER
16 Videos
Similar Questions
Knowledge Check
Shown a circuit with known resistance R,R1 and R2 neglect the resistance of the conducting wires and internal resistance of current source The magnitude of electrotive force E1 each that the currect l through the resistance R in zero will be
 AE(R2R1)BE(R1R2)CE(R1+R2R2)DE(R1R1+R2)
AE(R2R1)BE(R1R2)CE(R1+R2R2)DE(R1R1+R2)The current in the resistance R will be zero if
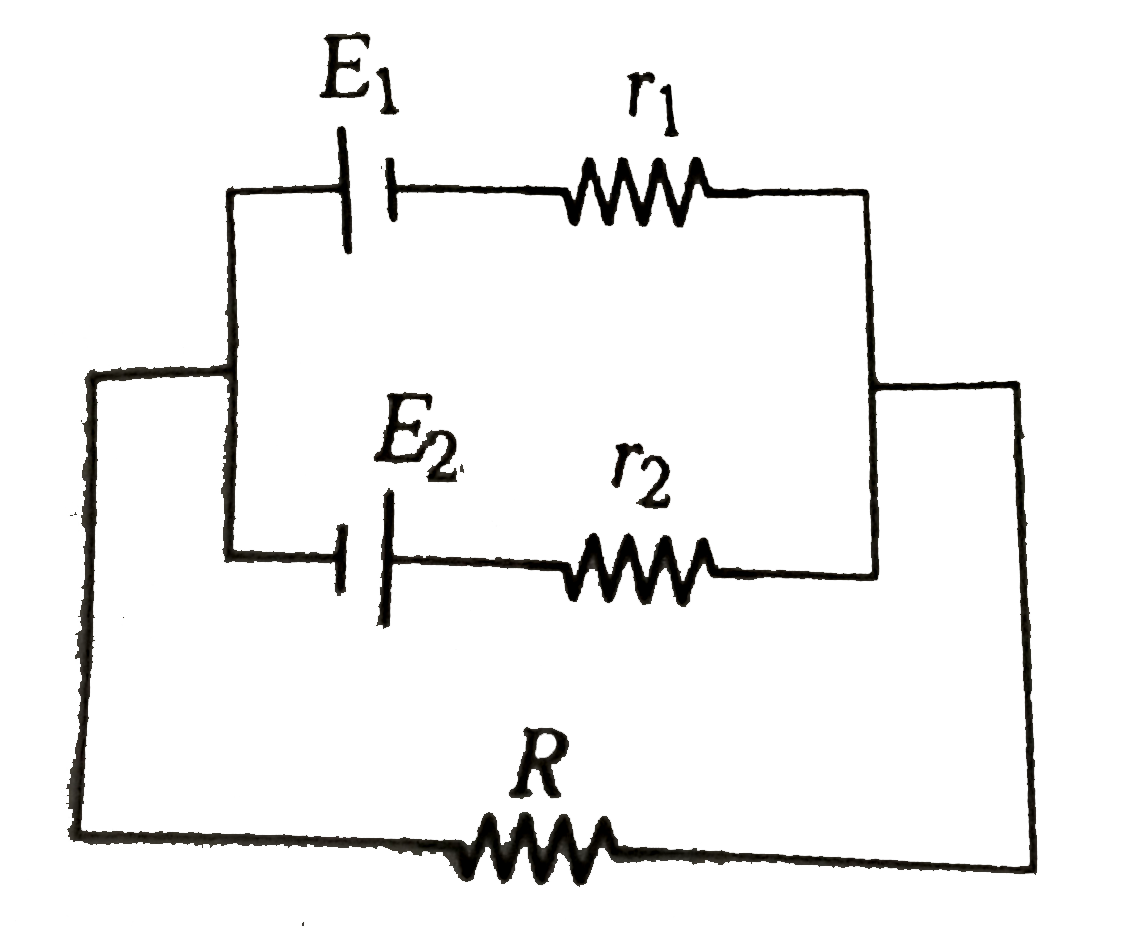 AE1r1=E2r2BE1r1=E2r2C(E1+E2)r1=E1r1D(E1−E2)r1=E2r1
AE1r1=E2r2BE1r1=E2r2C(E1+E2)r1=E1r1D(E1−E2)r1=E2r1An total resistance R is connected to a cell of internal resistance r the maximum current flows in the external resistance, when
AR=rBR<rCR>rDnone of the above
In the given circuit, the current through the resistance R is __________ A.
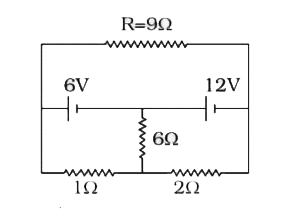
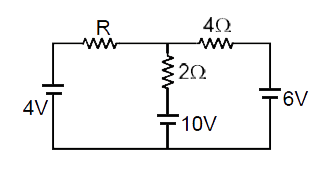
View SolutionFor what value of R in circuit , current through 4Ω resistance is zero.
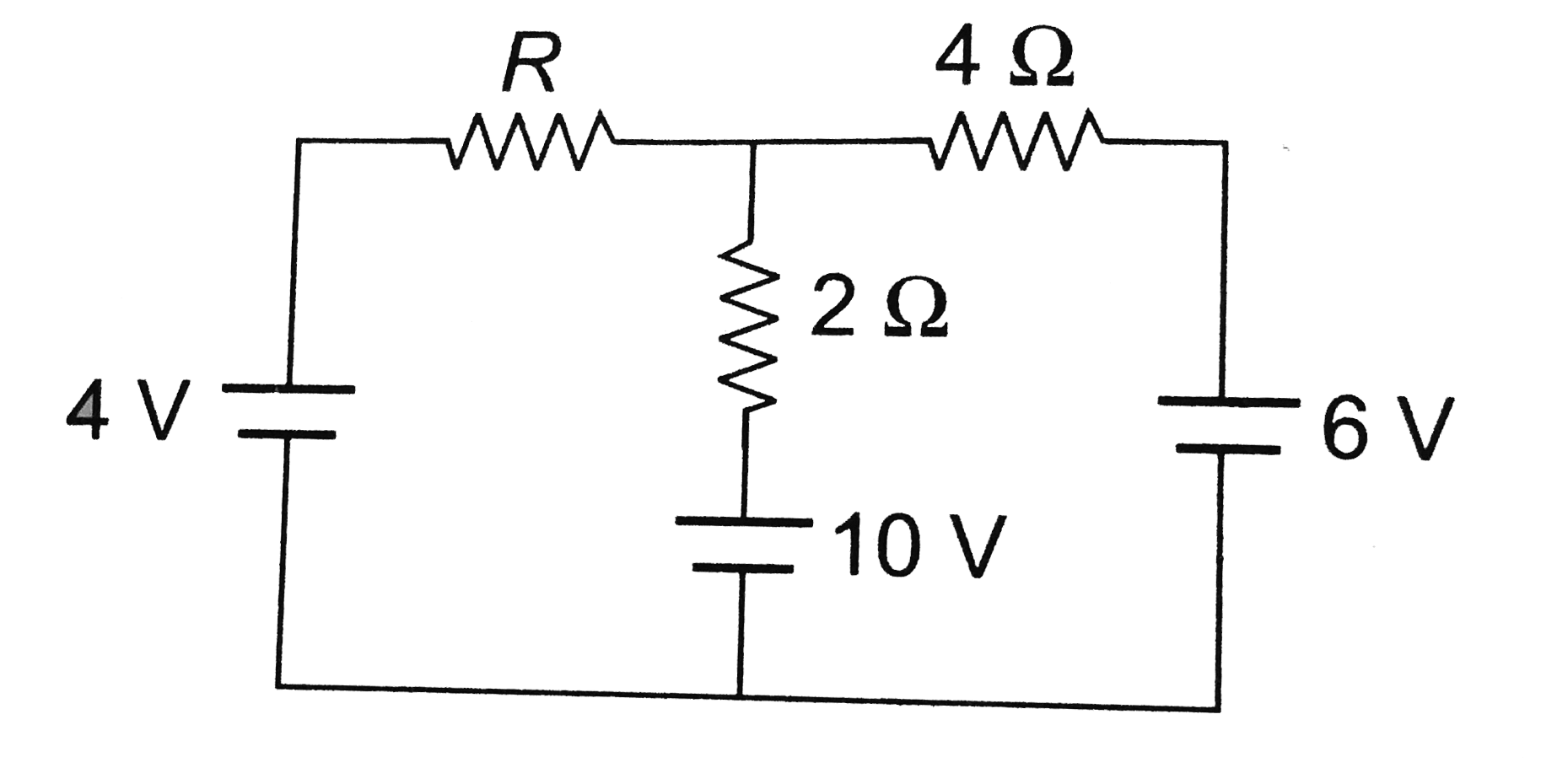
View SolutionFor what value of R (in Ω) in circuit, current through 4Ω resistance is zero ?
View SolutionIn a circuit a cell with internal resistance r is connected to an external resistance R. The condition for the maximum current that drawn from the cell is
View SolutionIn a circuit a cell with internal resistance r is connected to an external resistance R. The condition for the maximum current that drawn from the cell is
View Solution
