A large open top container of negligible mass and uniform cross sectional area A a has a small hole of cross sectional area A/100 in its side wall near the bottom. The container is kept on a smooth horizontal floor and contains a liquid of density ρ and mass M0. Assuming that the liquid starts flowing out horizontally through the hole at t=0, calculate a the acceleration of the container and its velocity when 75% of the liquid has drained out.
To solve the problem, we need to find the acceleration of the container and its velocity when 75% of the liquid has drained out. Here’s a step-by-step solution:
Step 1: Understand the System
We have a large open-top container with a small hole at the bottom. The container has a cross-sectional area A and the hole has a cross-sectional area A100. The liquid inside has a density ρ and an initial mass M0.
Step 2: Determine the Mass of Liquid Remaining
When 75% of the liquid has drained out, 25% remains. Thus, the mass of the liquid left in the container is:
M=M0−0.75M0=0.25M0
Step 3: Calculate the Thrust Force
The thrust force Ft exerted by the liquid flowing out can be calculated using the principle of momentum. The rate of mass flow dmdt through the hole can be expressed as:
dmdt=ρ⋅A100⋅v
where v is the velocity of the liquid flowing out of the hole.
The thrust force is given by:
Ft=v⋅dmdt=v⋅(ρ⋅A100⋅v)=ρAv2100
Step 4: Calculate the Acceleration of the Container
According to Newton's second law, the thrust force is also equal to the mass of the liquid remaining multiplied by the acceleration a of the container:
Ft=M⋅a=0.25M0⋅a
Setting the two expressions for thrust force equal gives:
ρAv2100=0.25M0⋅a
From this, we can express the acceleration a:
a=ρAv2100⋅0.25M0=4ρAv2100M0
Step 5: Calculate the Velocity of Efflux
Using Torricelli’s theorem, the velocity of efflux v can be calculated as:
v=√2gh
where h is the height of the liquid in the container. Since 75% of the liquid is drained, 25% remains:
h=0.25L
Thus,
v=√2g(0.25L)=√gL2
Step 6: Substitute v into the Acceleration Formula
Now we can substitute v back into the acceleration formula:
a=4ρA(√gL2)2100M0=4ρA⋅gL2100M0=2ρAgL100M0
This simplifies to:
a=ρAgL50M0
Step 7: Calculate the Velocity When 75% of the Liquid is Drained
Now substituting h back into the velocity of efflux:
v=√2g(0.25L)=√gL2
Final Results
1. The acceleration of the container when 75% of the liquid has drained out is:
a=ρAgL50M0
2. The velocity of the liquid when 75% has drained out is:
v=√gL2
We have a large open-top container with a small hole at the bottom. The container has a cross-sectional area A and the hole has a cross-sectional area A100. The liquid inside has a density ρ and an initial mass M0.
When 75% of the liquid has drained out, 25% remains. Thus, the mass of the liquid left in the container is:
M=M0−0.75M0=0.25M0
The thrust force Ft exerted by the liquid flowing out can be calculated using the principle of momentum. The rate of mass flow dmdt through the hole can be expressed as:
dmdt=ρ⋅A100⋅v
where v is the velocity of the liquid flowing out of the hole.
Ft=v⋅dmdt=v⋅(ρ⋅A100⋅v)=ρAv2100
According to Newton's second law, the thrust force is also equal to the mass of the liquid remaining multiplied by the acceleration a of the container:
Ft=M⋅a=0.25M0⋅a
Setting the two expressions for thrust force equal gives:
ρAv2100=0.25M0⋅a
From this, we can express the acceleration a:
a=ρAv2100⋅0.25M0=4ρAv2100M0
Using Torricelli’s theorem, the velocity of efflux v can be calculated as:
v=√2gh
where h is the height of the liquid in the container. Since 75% of the liquid is drained, 25% remains:
h=0.25L
Thus,
v=√2g(0.25L)=√gL2
Now we can substitute v back into the acceleration formula:
a=4ρA(√gL2)2100M0=4ρA⋅gL2100M0=2ρAgL100M0
This simplifies to:
a=ρAgL50M0
Now substituting h back into the velocity of efflux:
v=√2g(0.25L)=√gL2
1. The acceleration of the container when 75% of the liquid has drained out is:
a=ρAgL50M0
2. The velocity of the liquid when 75% has drained out is:
v=√gL2
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.1
11 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.2
11 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICSCHAPTER - FLUID MECHANICSEXERCISE - INTEGER_TYPE
1 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A large open top container of negligible mass and uniform cross-sectional area A has a small hole of croos-sectional area A100 in its side wall near the bottom.The container is kept on a smooth horizontal floor and contains a liquid of density ρ and mass m0. Assuming that the liquid starts flowing out horizontally through the hole at t=0, The acceleration of the container is x10m/s2 than x is -
View SolutionA large container contains a liquid of density p, mass m, and placed on a smooth horizontal floor. The mass of container is negligible and cross sectional area is A. Now at the bottom of the container, there is small hole of cross section area A/200 in the side wall through which the liquid starts flowing horizontally at t=0. What will be the velocity of efflux when half of the liquid is drained out. Also, find the acceleration of the liquid flowing out.
View Solution
Knowledge Check
A large open top container of negligible mass and uniform ross sectional area A has a small uniform cross sectional area a in its side wall near the bottom. The container is kept over a smooth horizontal floor and contains a liquid of density ρ and mass m0. Assuming that the liqudi starts flowing through the hole A the acceleration of the container will be
A2agABagAC2AgaDAgaA large container (with open top) of negligible mass and uniform cross-sectional area A has a small hole of cross-sectional area a in its side wall near the bottom. The container contains a liquid of density ρ and mass m. If the liquid starts flowing out of the hole at time t = 0, the velocity of efflux of the liquid when 75% of the liquid has drained out is:
A√3mg4AρB√2mgAρC2√mgAρD√mg2Aρ
A cylindrical massless container of cross sectional area A have a fluid filled up to height h and have a small orifice of area a in wall near its bottom. Find minimum coefficient between container and ground so that container does not move
View SolutionA vessel of 100cm2 cross sectional area contains water. The mass of water is 2 kg. Find the pressure acting on the bottom surface.
View SolutionA liquid is flowing through a pipe of non-uniform cross-section. At a point where area of cross-section of the pipe is less, match the following columns.

View SolutionA fixed container of height H with large cross-sectional area A is completely filled with water. Two small orifice of cross-sectional area a are made, one at the bottom and the other on the vertical side of the container at a distance H/2 from the top of the container find the time taken by the water level to reach a height of H/2 from the bottom of the container.
View SolutionA container of cross-section area A resting on a horizontal surface, holds two immiscible and incompressible liquid of densities ρand2ρ as shown in figure. The lower density liquid is open to the atmosphere having pressure P0. The pressure at the bottom of the container is
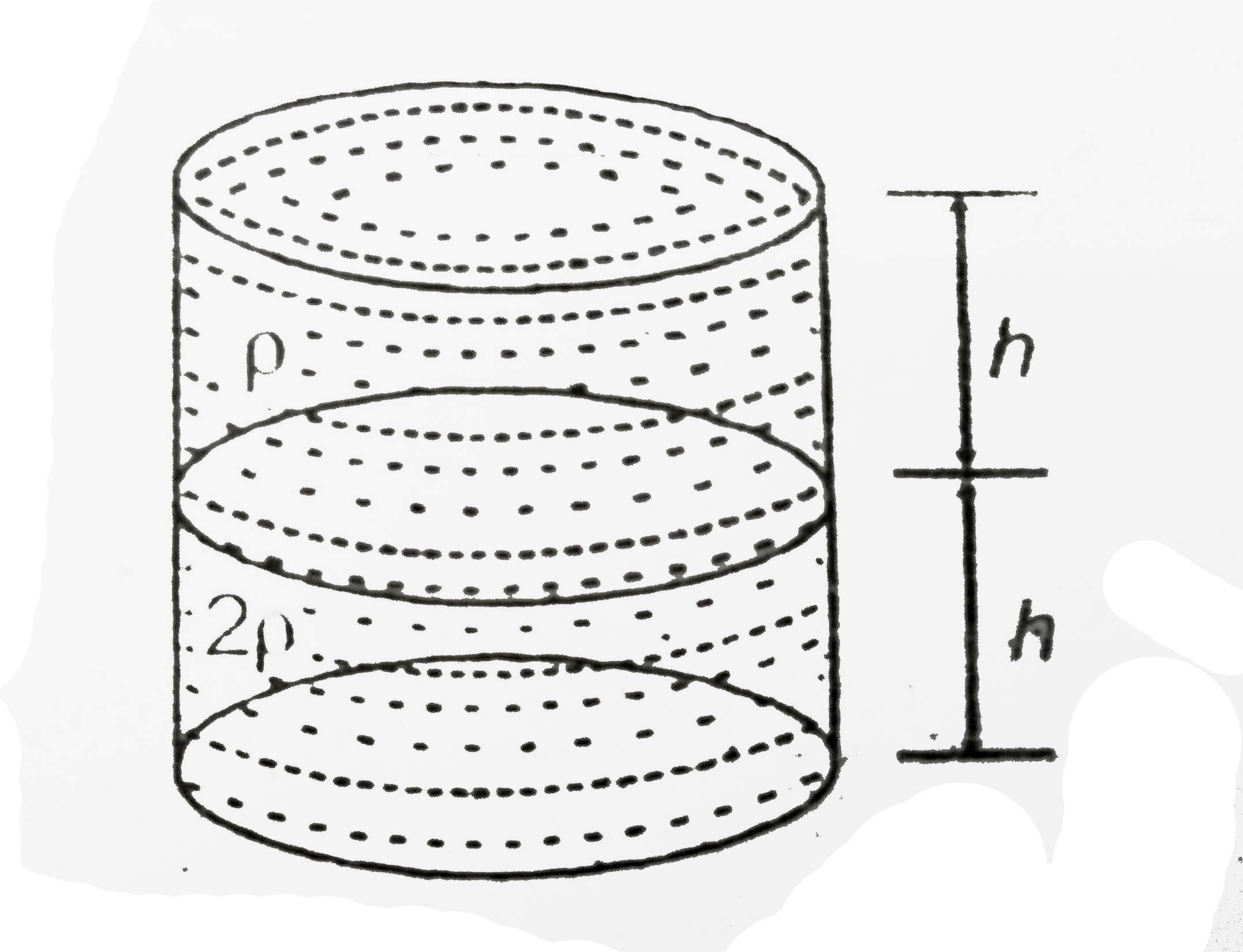
View SolutionThere are two holes, each of cross-sectional area a, on the opposite side of a wide rectangular tank containing a liquid of densityρ. When the liquid flows out of the holes, find the net force on the tank. Given h is the vertical distance between two holes.
View Solution
