A line charge λ per unit length is lodged uniformly onto the rim of a whell of mass M and radius R. The whell has light non-conducting spokes and is free to rotate without friction about its axis (figure). A uniform magnetic field extends over a circular region withing the rim. It is given by, B=B0k(r≤a,a<R)
What is the angular velocity of the whell after the field is suddenly switched off?

Given Linear charge density λ=Total chargelength=Q2πR.....(i)
Radius of rim=M, Magnetic field extends over a circular region
B=−B0ˆk(r≤a,a<R)=O(otherwise)
Let the angular velocity of the wheel be w, then induced emf e=−dϕdt
E exist along circumference of radius a due to change in magnetic flux.
e∫dI=−ddt(πa2B)⇒E×2πa=−πa2dBdt⇒E=a2.dBdt
Force on charge (F)=QE=(2πa×λ)(−a2.dBdt)=−πa2λdBdt
But force (F) =dPdt=d(MV)dt=M.dVdt⇒M.dVDT=−πa2λdBdt(∴V=RW)
MR(dW) =−πa2λdBdt⇒dW=−πa2λMR,dB,Integrating both sides ω=−πa2λBMR
As direction of angular velocity is along axis ω=λa2πMRBυ
Topper's Solved these Questions
- BOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTROMAGNETIC INDUCTION EXERCISE - Textual Exercise18videos
ELECTROMAGNETIC INDUCTION
- BOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - DAM SURE LAQ1videos
ELECTRIC CHARGES AND FIELDS
- BOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - ELECTROMAGNETIC WAVEEXERCISE - ADDITIONAL EXERCISES19videos
ELECTROMAGNETIC WAVE
Similar Questions
A line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig.). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=−B0k (r≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?
 View Solution
View SolutionA line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.22). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=B0k (ra≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
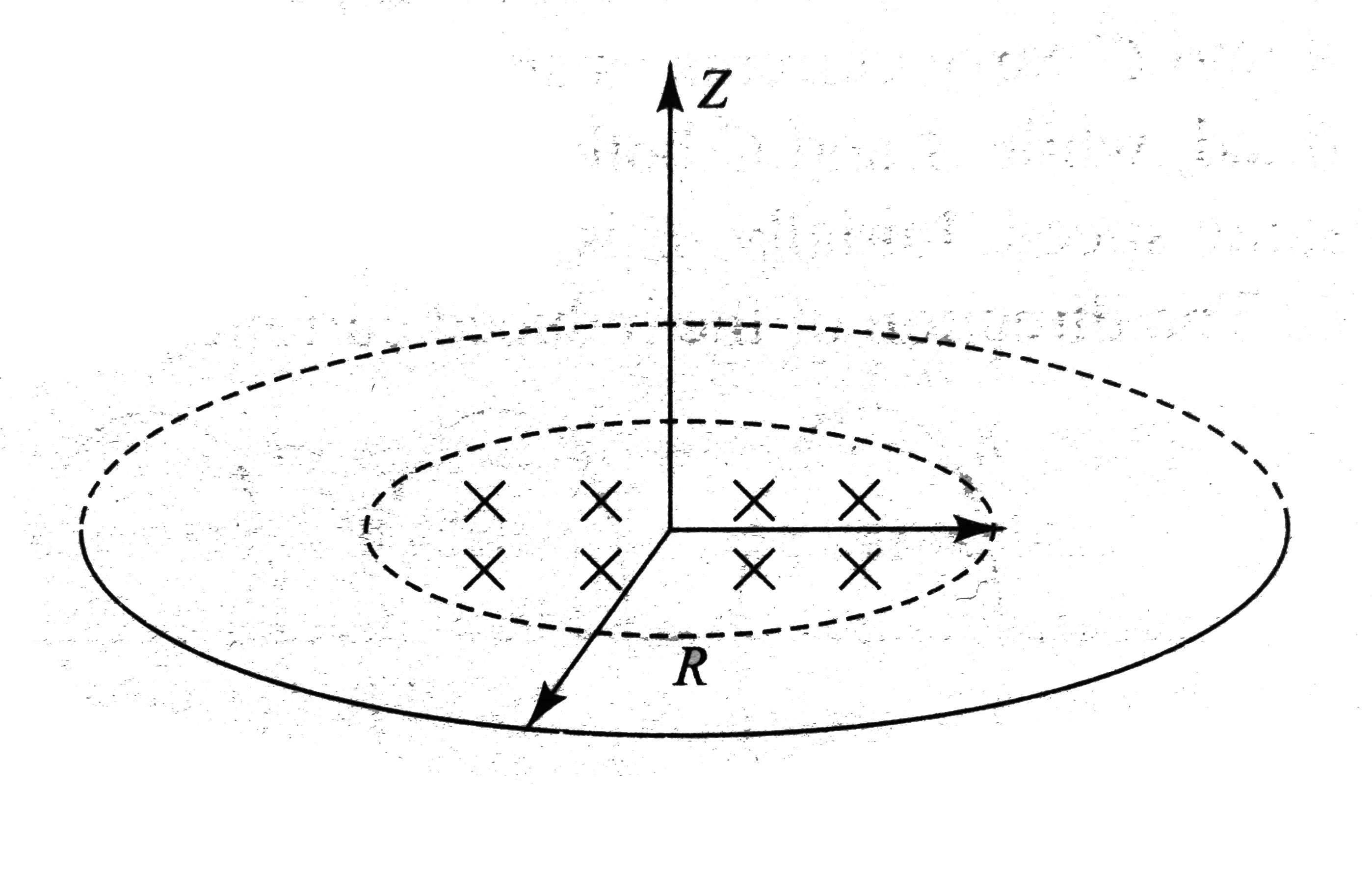 A(a) −2B0πa2rmRˆkB(b) −2B0πa2r3mRˆkC( c) B0πa2λmRˆkD( d) −B0πa2λmRˆk
A(a) −2B0πa2rmRˆkB(b) −2B0πa2r3mRˆkC( c) B0πa2λmRˆkD( d) −B0πa2λmRˆk - Question 2 - Select One
A non-conducting disk of radius R is rotating about its own axis with constant angular velocity ω in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
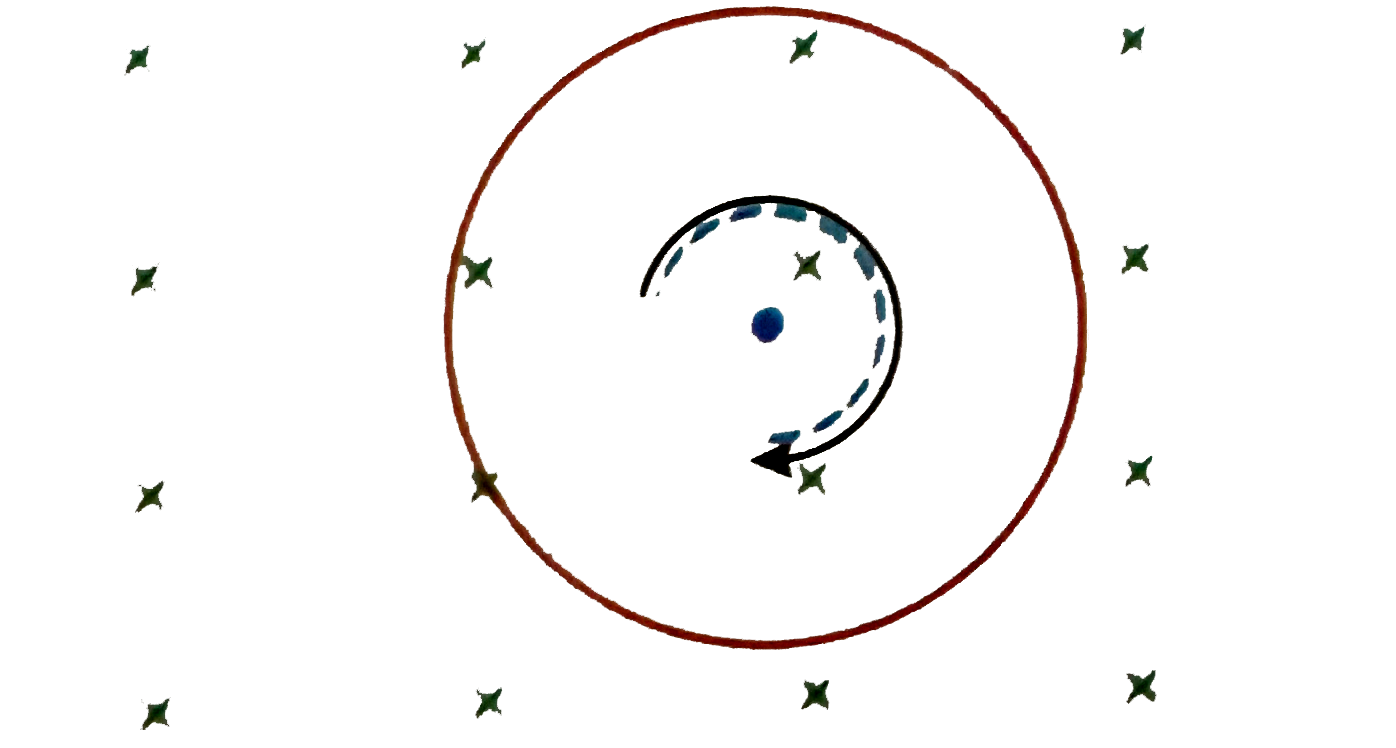 ABωR22BBωR2CZeroDBωR23
ABωR22BBωR2CZeroDBωR23
A line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.08). A uniform magnetic field extends over a circular region within the rim. It is given by,
→B=−B0ˆk(r≤a,aItR)
= 0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off ?
 View Solution
View SolutionA line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
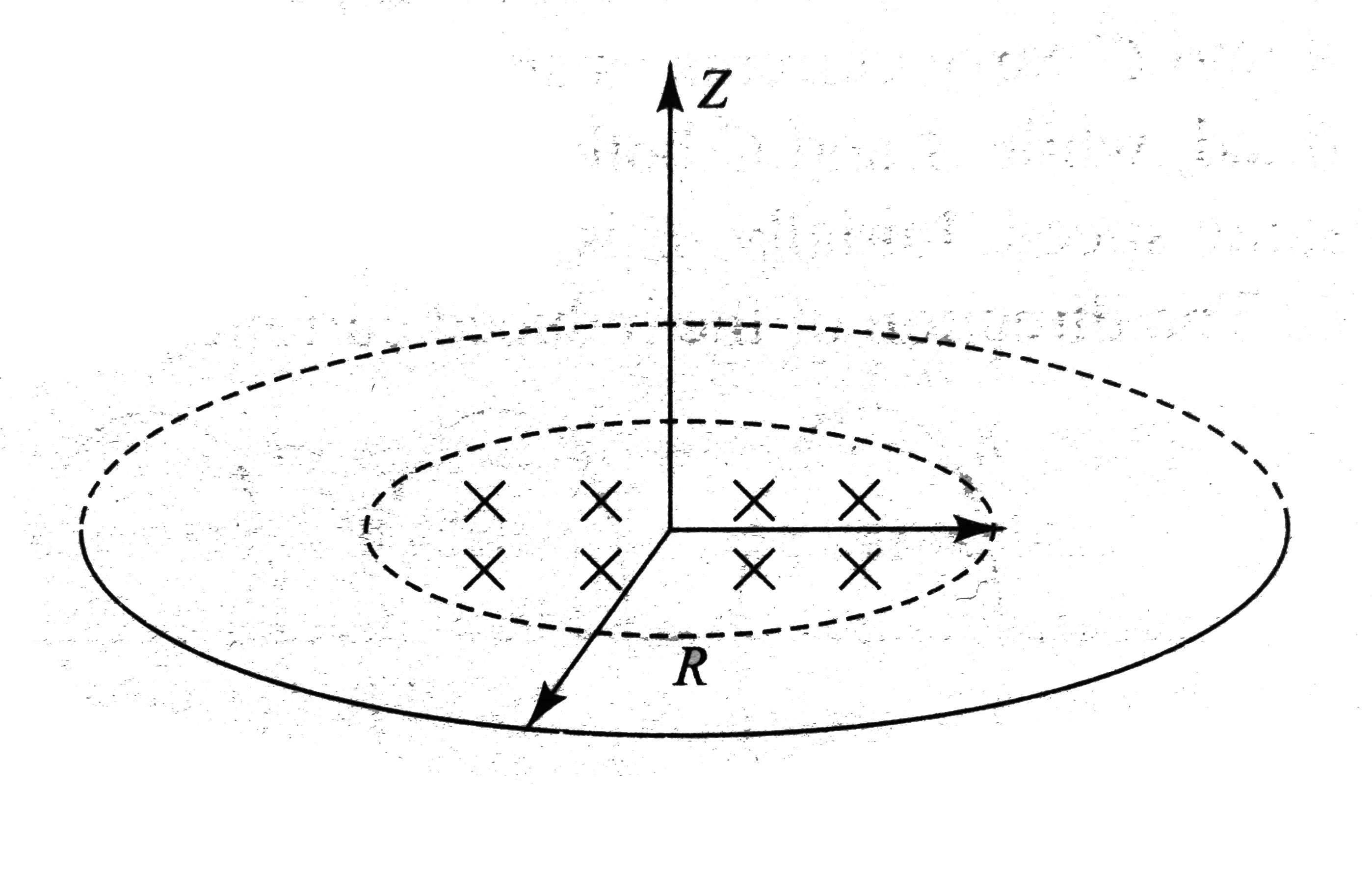 View Solution
View SolutionA conducting disc of radius r rotates with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View SolutionA conducting disc of radius r rotaes with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
View SolutionA charge Q is spread uniformly over an insulated ring of radius R.What is the magnetic moment of the ring if it is rotated with an angluar velocity ω about its axis?
View SolutionA ring of mass m, radius r having charge q uniformly distributed over it and free to rotate about its own axis is placed in a region having a magnetic field B parallel to its axis. If the magnetic field is suddenly switched off, the angular velocity acquired by the ring is:
View Solution

