A source contains two phosphorous radio nuclides .3215P(T1/2=14.3d) and .3315P(T1/2=25.3d). Initially, 10% of the decays come from .3315P. How long one must wait until 90% do so ?
Suppose initially the source has 90% .15Pt32 and 10% .15P321, say 9x gram of P2 and x gram of P1. After t days, suppose the source has 90% .15P332 and 10%.15P32t i.e., y gram of P2 and 9y gram of P1
we have to calculate :
from NN0=(12)n=(12)t/T=2−i/T
N=N02−t/T
y=9x2−t/14.3 for P2 and 9y=x2−t/25.3 for P1 Dividing we get
19=9×2(t/25.3−t/14.3)
or 181=2−11t/25.3×14.3
log 1 - log 81=−11t25.3×14.3 log 2
0−1−9085=−11t25.3×14.3×0.3010.
t=25.3×14.3×1.908511×0.3010=208.5 days.
Topper's Solved these Questions
- NUCLEIBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - NUCLEIEXERCISE - DAM SURE (VSAQ)
1 Videos
- NUCLEIBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - NUCLEIEXERCISE - TEXTUAL EXERCISES
32 Videos
- MOVING CHARGES AND MAGNETISHMBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - MOVING CHARGES AND MAGNETISHMEXERCISE - ADDITIONAL EXERCISES
26 Videos
- RAY OPTICAL AND INSTRUMENTSBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - RAY OPTICAL AND INSTRUMENTSEXERCISE - TEXTUAL EXERCISES
60 Videos
Similar Questions
A source contains two phosphorus radio nuclides 3215P(T12=14.3d) and 3315P(T12=25.3d). Initially, 10% of the decays come from 3315P . How long one must wait until 90% do so ?
View SolutionA source contains two phosphorus radionuclides .15P35(T1/2=14.3days) and .15P33(T1/2=25.3days). Initially, 10% of the decays come from .15P35. How long one must wait until 90% do so?
View Solution
Knowledge Check
For an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
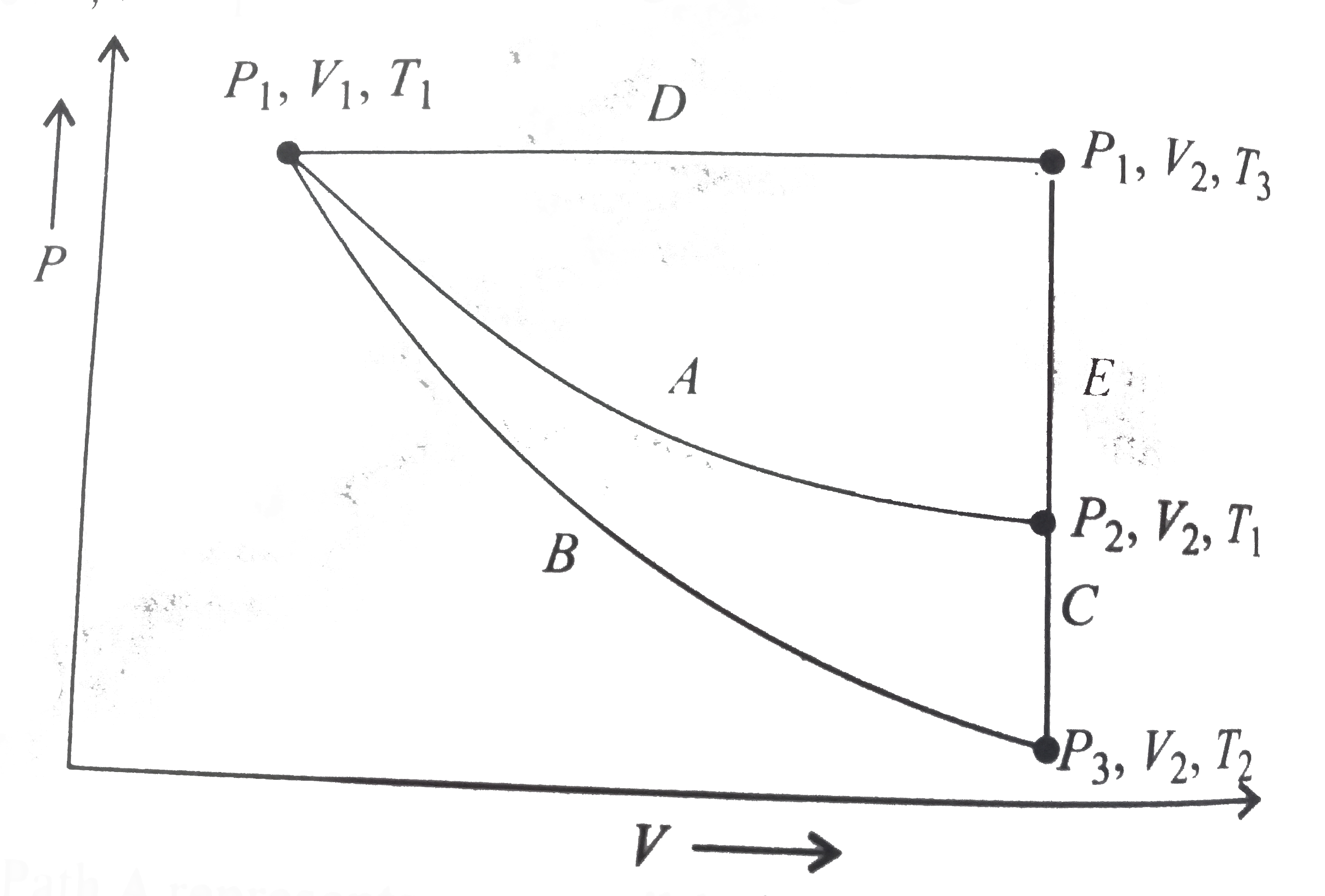
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is qrev, for path (D+E)?AP(V2−V1)B∫T1T3CV(T)TdTCnRInV2V1D∫T3T1CV(T)dTFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.
Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is ΔS for path (D+E)?A−P(V2−V1)B−nRInV2V1C+P(V2−V1)DnRInV2V1
A source contains two phosphorus radionuclides .15P35(T1/2=14.3days) and .15P33(T1/2=25.3days). Initially, 10% of the decays come form .15P35. How long one must wait until 90% do so?
View SolutionA radioactive sample contains two radionuclids A and B having décay constant λh−1 and 2λh−1. Initially, 25% of total decay comes from A. How long (in h ) will it take before 75% of total decay comes from A. (Take λ=ln3)
View Solution15Pr−1:15Pr−2=3:4, then r=
View SolutionA particle moves in a straight line with uniform acceleration and its distances from an origin O on the line (not necessarily the position at time t = 0) at times t1,t2,t3 are d1,d2,d3 respectively. Prove that if t1,t2,t3 form an A.P. whose common difference isd and d1,d2,d3 are in G.P. then the acceleration is(√d1−√d3)2d2
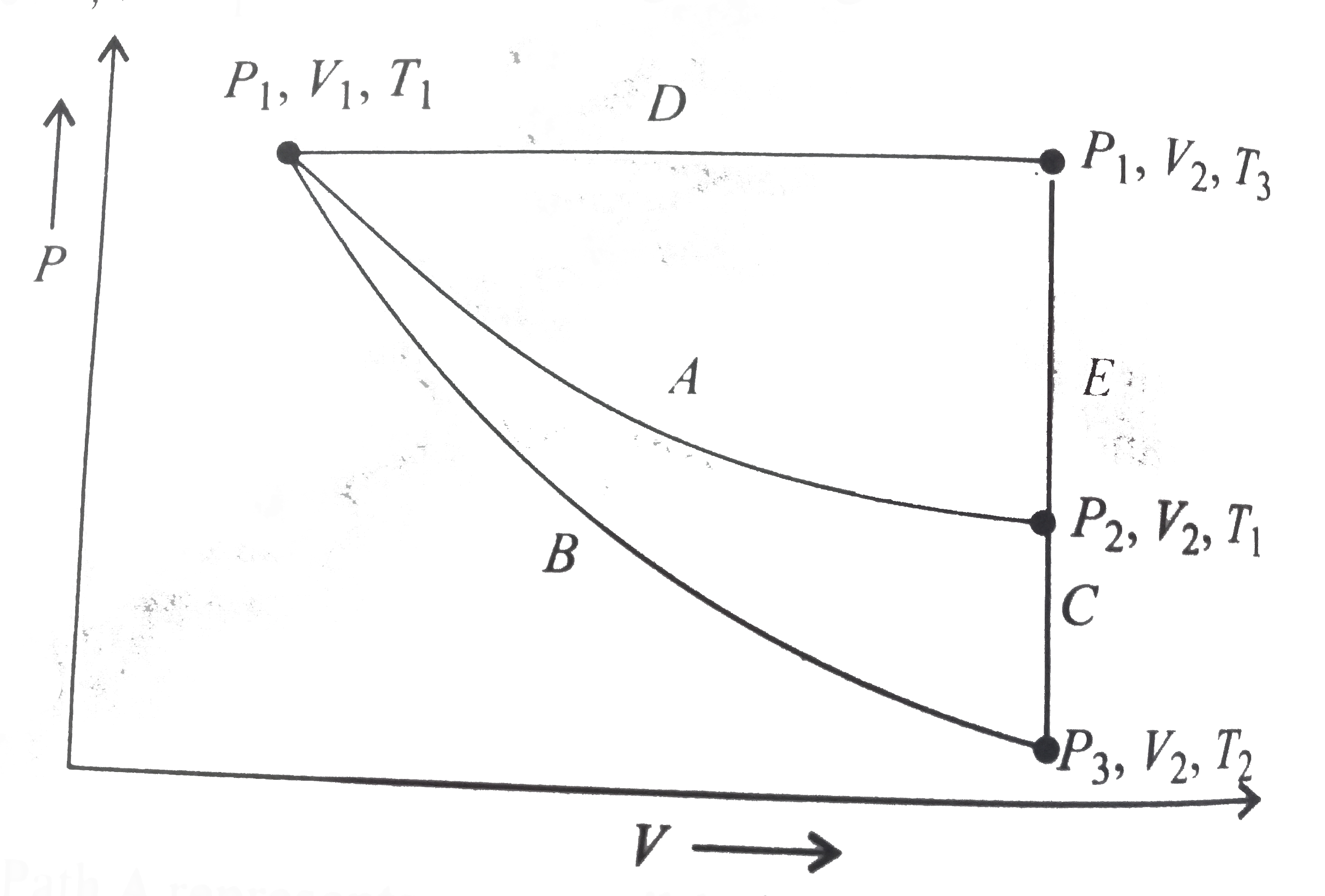
View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.

Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is qrev, for path (D+E)?
View SolutionFor an ideal gas, an illustratio of three different paths A(B+C) and (D+E) from an initial state P1,V1,T1 to a final state P2,V2,T1 is shown in the given figure.

Path Arepresents a reversible isothermal expansion form P1,V1 to P2,V2, Path (B+C) represents a reversible adiabatic expansion (B) from P1,V1,T1→P3,V2,T2 followed by reversible heating the gas at constant volume (C)from P3,V2,T2 to P2,V2,T1. Path (D+E) represents a reversible expansion at constant pressure P1(D) from P1,V1,T1 to P1,V2,T3 followed by a reversible cooling at constant volume V2(E) from P1,V2,T3→P2,V2,T1.
What is ΔS for path (D+E)?
View Solution
