The positions of two point charges $q_{1}$ and $q_{2}$ are ${\to r}_{1}$ and ${\to r}_{2}$ , respectively. Find the position of the point where the net field is zero due to thses charges.

A

$\frac{{\to r}_{1} \sqrt{q_{1}} + {\to r}_{2} {\sqrt{q}}_{2}}{{\sqrt{q}}_{1} + {\sqrt{q}}_{2}}$

B

$\frac{{\to r}_{1} \sqrt{q_{2}} + {\to r}_{2} {\sqrt{q}}_{1}}{{\sqrt{q}}_{1} + {\sqrt{q}}_{2}}$

C

$\frac{{\to r}_{1} \sqrt{q_{2}} + {\to r}_{2} {\sqrt{q}}_{1}}{\sqrt{q_{1} + q_{2}}}$

D

$\frac{{\to r}_{1} \sqrt{q_{1}} + {\to r}_{2} {\sqrt{q}}_{2}}{\sqrt{q_{1} + q_{2}}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

At P, Let the net field be zero,
${\to E}_{P} = {\to E}_{1} + {\to E}_{2} = 0$ …(i)

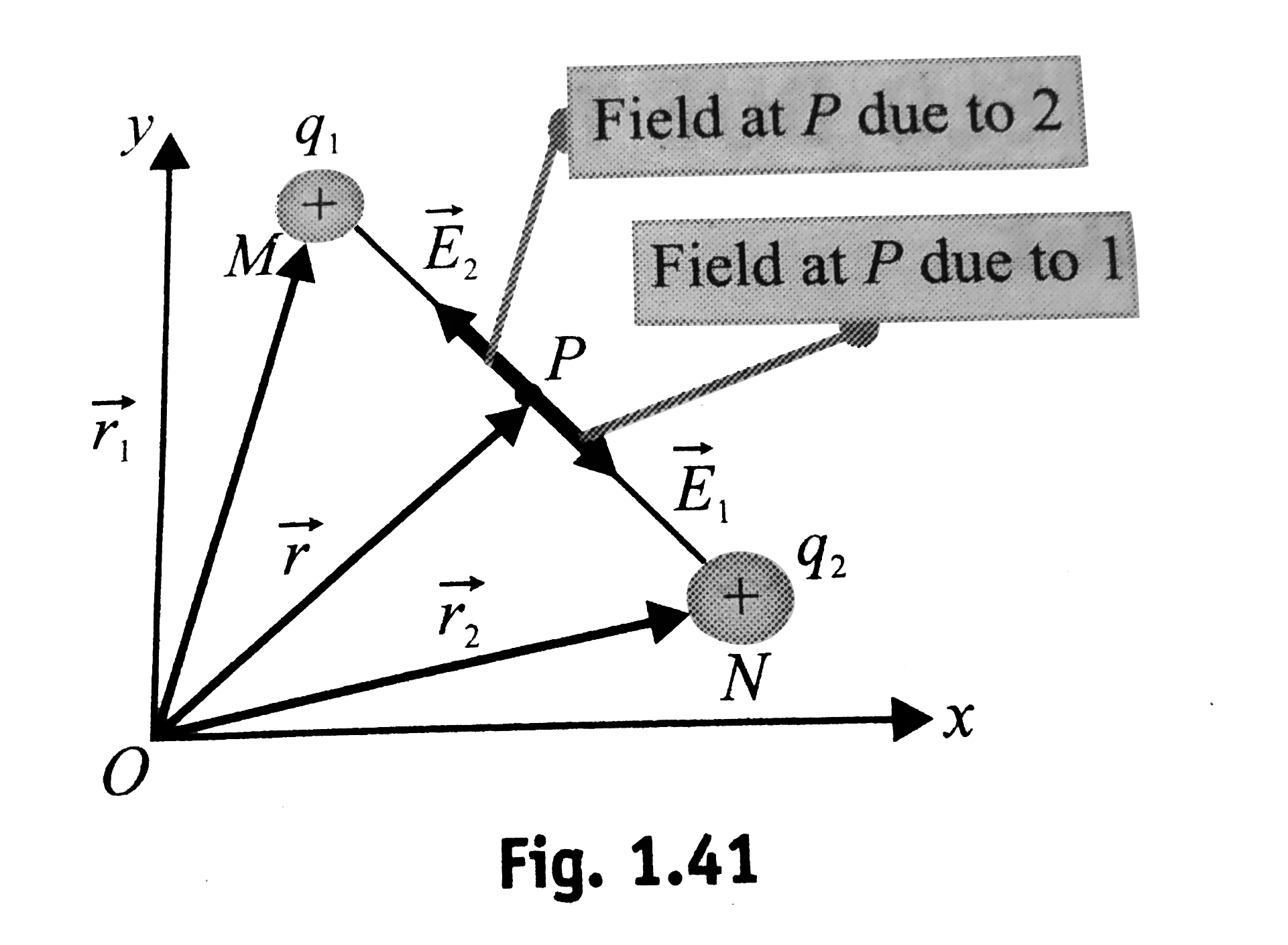
As we know,
${\to E}_{1} = \frac{q_{1} (\to r - {\to r}_{1})}{4 π ε_{0} ∣ \to r - {\to r}_{1}^{3}}$ …(ii)
and ${\to E}_{2} = \frac{q_{2} (\to r - {\to r}_{2})}{4 π ε_{0} ∣ \to r - {\to r}_{2}^{3}}$
Substituting ${\to E}_{1}$ and ${\to E}_{2}$ from eq.(ii) and (iii)in Eq.(i) we have
$\frac{q_{1} (\to r - \to r_{1})}{4 π ε_{0} {∣ ∣ \to r - \to r_{1} ∣ ∣}^{3}} + \frac{q_{2} (\to r - {\to r}_{2})}{4 π ε_{0} {| \to r - {\to r}_{2} |}_{2}^{3}} = 0$
or, $q_{1} (\to r - {\to r}_{1}) + q_{2} (\to r - {\to r}_{2}) \cdot \frac{{| \to r - {\to r}_{1} |}_{1}^{3}}{{| \to r - {\to r}_{2} |}_{2}^{3}} = 0$ ...(iv)
Since $E = 0$ at P, $∣ ∣ {\to E}_{1} ∣ ∣ = ∣ ∣ {\to E}_{2} ∣ ∣$
$\Rightarrow \frac{q_{1}}{4 π ε_{0} r_{P_{1}}^{2}} = \frac{q_{2}}{4 π ε_{0} r_{P_{2}}^{2}}$
Where ${\to r}_{P_{1}} = \to r - {\to r}_{1}$ and ${\to r}_{P_{2}} = \to r - {\to r}_{1}$ . Therefore,
$\frac{q_{1}}{{| \to r - {\to r}_{1} |}_{1}^{2}} = \frac{q_{2}}{{| \to r - {\to r}_{2} |}_{2}^{2}}$ or $\frac{| \to r - {\to r}_{1} |}{| \to r - {\to r}_{2} |} = \frac{{\sqrt{q}}_{1}}{{\sqrt{q}}_{2}}$ ...(v)
Substituting the value of $| \to r - {\to r}_{1} | / ∣ \to r - {\to r}_{1}$ from Eq. (v) in Eq. (iv) we get
$q_{1} (\to r - {\to r}_{1}) + q_{2} (\to r - {\to r}_{2}) (\frac{q_{1}}{q_{2}}) = 0$ or $\to r = \frac{{\to r}_{1} \sqrt{q_{2}} + {\to r}_{2} {\sqrt{q}}_{1}}{{\sqrt{q}}_{1} + {\sqrt{q}}_{2}}$ .

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Two point charges $+ 8 q$ and $- 2 q$ are located at $x = 0$ and $x = L$ respectively. The location of a point on the x axis at which the net electric field due to these two point charges is zero is
A8L
B4L
C2 L
DL

CENGAGE PHYSICS-COULOMB LAW AND ELECTRIC FIELD-Single Answer Correct Type

Home
Ask Doubt
Profile