Find the expression for the potential energy of a system of two point charges q1,andq2 glocated at →r1and→r2 respectively in an external electric field →E.
(b) Draw equipotential surfaces due to an isolated point charge (-q) and depict the electric field lines.
(c) Three point charges +1μC,−1μCand+2μC are initially infinite distance apart. Calculate the work done in assembling these charges at the vertices of an equilateral triangle of side 10 cm.
(a) To find the potential energy of a system of two point charges →r1and→r2 situated at points and respectively in an external electric field →E, first we calculate the work done in bringing the charge q1. from infinity to →r1. Work done in this step is
W1=q1V(→r1), where V(→r1) is the electrostatic potential at →r1.
Now we consider the work done in bringing q2 to →r2. In this step work W, is done against the electric field and work W2 is done against the field due to charge q1. Obviously
W2=q2V(→r2), where V(→r2) is the electrostatic potential at →r2
and W12=q1q24πε0.r12where r12=|→r1−→r2| = distance between q1andq2
Total work done in bringing q2 to →r2=W2+W12=q2V(→r2)+q1q24π∈0.r12
Potential energy of the system U.
= The total work done in assembling the configuration
U=W1+W2+W12=q1V(r1)+q2V(r2)+q1q24πε0.r12
(b) Equipotential surfaces due to an isolated point charge-q are drawn here and electric field lines have been depicted. 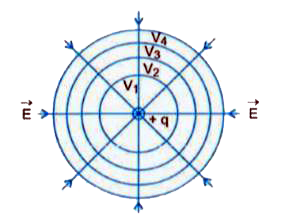
(c) Hence q1=+1μC=+1×10−6C,q2=−1μC=−1×10−6C,q3=+2μC=+2×10−6Candr12=r13=r23=10cm=0.1m
Work done is assembling the charges =Potential energy of charge alignment
W=U=14πε0[q1q2r12+q1q3r13+q2q3r23]
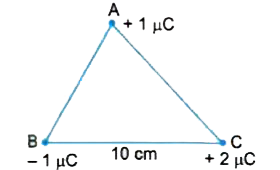
=9×109[(1×10−6)×−1×10−60.1+(1×10−6)×2×10−60.1+(−1×10−6)×2×10−60.1]
=0.09J
Similar Questions
Deduce the expression for the potential energy of a system of two point charges q1 and q2 brought from infinity to the points →r1 and →r2 respectively in the presence of external electric field →E.
View SolutionTwo point charges q1andq2 are located at →r1and→r2 respectively in an external electric field E. Obtain the expression for the total work done in assembling this configuration.
View Solution
Knowledge Check
The positions of two point charges q1 and q2 are →r1 and →r2 , respectively. Find the position of the point where the net field is zero due to thses charges.
A→r1√q1+→r2√q2√q1+√q2B→r1√q2+→r2√q1√q1+√q2C→r1√q2+→r2√q1√q1+q2D→r1√q1+→r2√q2√q1+q2The two point charges 4μC and 1μC are separated by a distance of 2 m in air. Find the point on the line joining the charges at which net electric field of the system is zero.
A43m from 1μCB23m from 1μCC43m from 4μCD23m from 4μC
The positions of two point charges q1 and q2 are →r1 and →r2 , respectively. Find the position of the point where the net field is zero due to thses charges.
View SolutionCalculate the electrostatic potential energy of a system of three point charges q1,q2 and q3 located respectively at →r1,→r2 and →r3 with respect to a common origin O.
View SolutionTwo point charges +4μCand−2μC are separated by a distance of 1 m in air. Calculate at what point on the line joining the two charges is the electric potential zero.
View SolutionTwo point charges q1andq2 are kept r distance apart in a uniform external electric field →E. Find the amount of work done in assembling this system of charges.
View SolutionThe point charges q1=1μC,q2=−2μC and q3=3μC are placed at (1m,0,0),(0,2m,0) and (0,0,3m) respectively. Find the electric potential at origin.
View SolutionTwo point charges +4μCand+1μC are separated by a distance of 2 m in air. Find the point on the line joining the two charges at which the net electric field of the system is zero.
View Solution
