Consider
 .
.
The potential difference VA−VB is.
A
E[C1C4−C2C3(C1+C2)(C3+C4)]
B
E[C1C4+C2C3(C1+C3)(C2+C4)]
C
E[C1C3+C2C4(C1+C2)(C3+C4)]
D
E[C1C3+C2C4(C1+C3)(C2+C4)]
Text Solution
The correct Answer is:A
Let charge be as shown. (Capacitors in series have the same the charge.)
Taking loop containing C1,C2, and E, we get
qC1+qC2−E=0 or q=E[C1C2C1+C2]
Similarly, from loop containing C3,C4, and E, we get
q'C3+q'C4−E=0 or q'=E[C3C4C3+C4]
Now, VA−VB=qC2−q'C
=E[C1C1+C2−C3C3+C4]
=E[C1C4−C3C2(C1+C2)(C3+C4)]
Now VA−VB=E⇒C1C4−C2C3=0
or C1C2=C3V4.
|
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Multile Correct
11 Videos
- ATOMSBOOK - CENGAGE PHYSICSCHAPTER - ATOMSEXERCISE - QUESTION BANK
40 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
Similar Questions
Knowledge Check
Two capacitors C1 and C2 are connected in a circuit as shown in figure. The potential difference (VA−VB) is
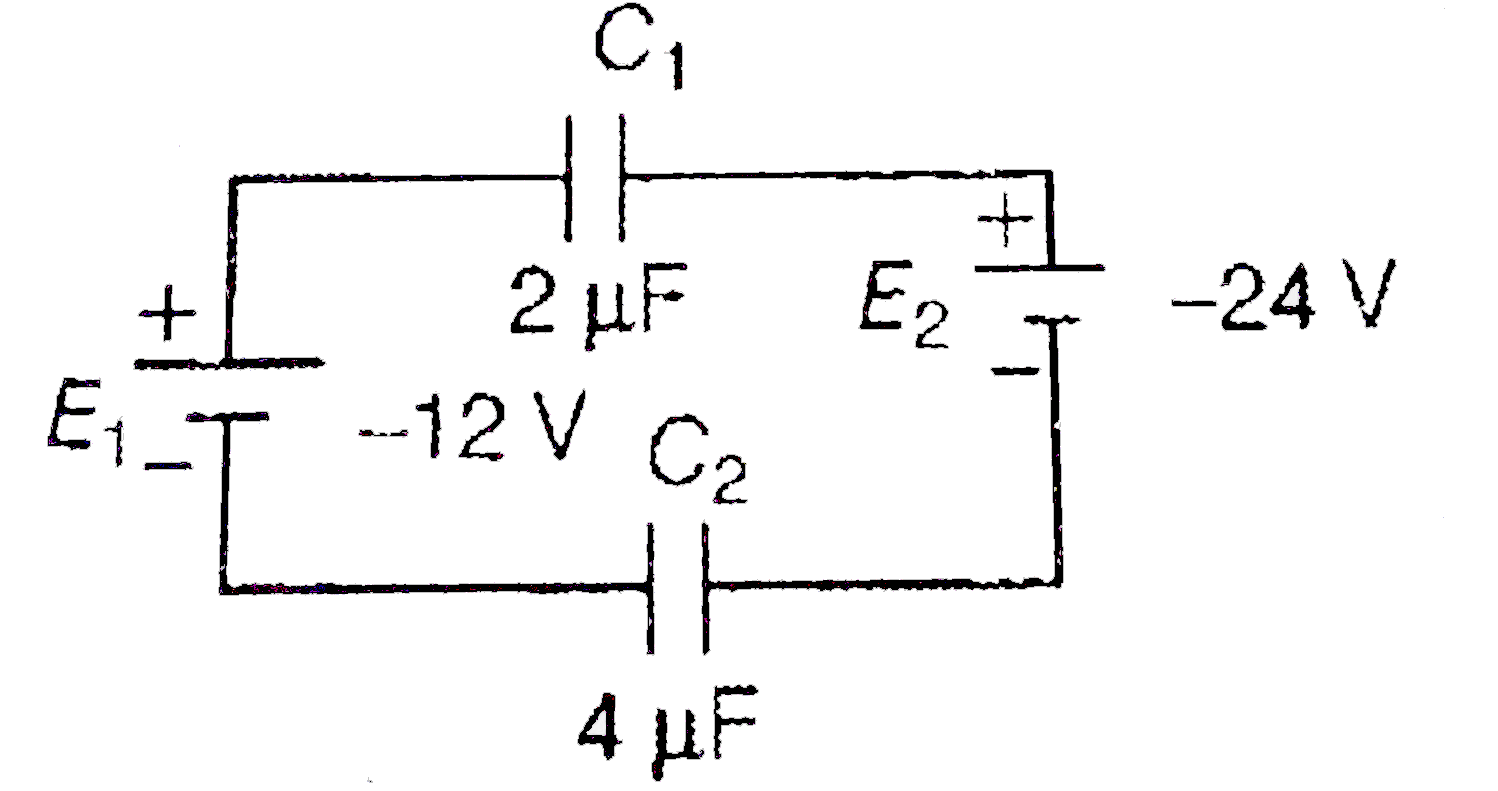 A8VB−8VC12VD12V
A8VB−8VC12VD12VIn figure two points A and B are located in a region of electric field . The potential difference VB−VA is
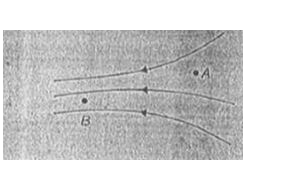 APositiveBNegativeCZeroDNone of these
APositiveBNegativeCZeroDNone of theseA current of 2A flows in a system of conductor as shown. The potential difference (VA−VB)
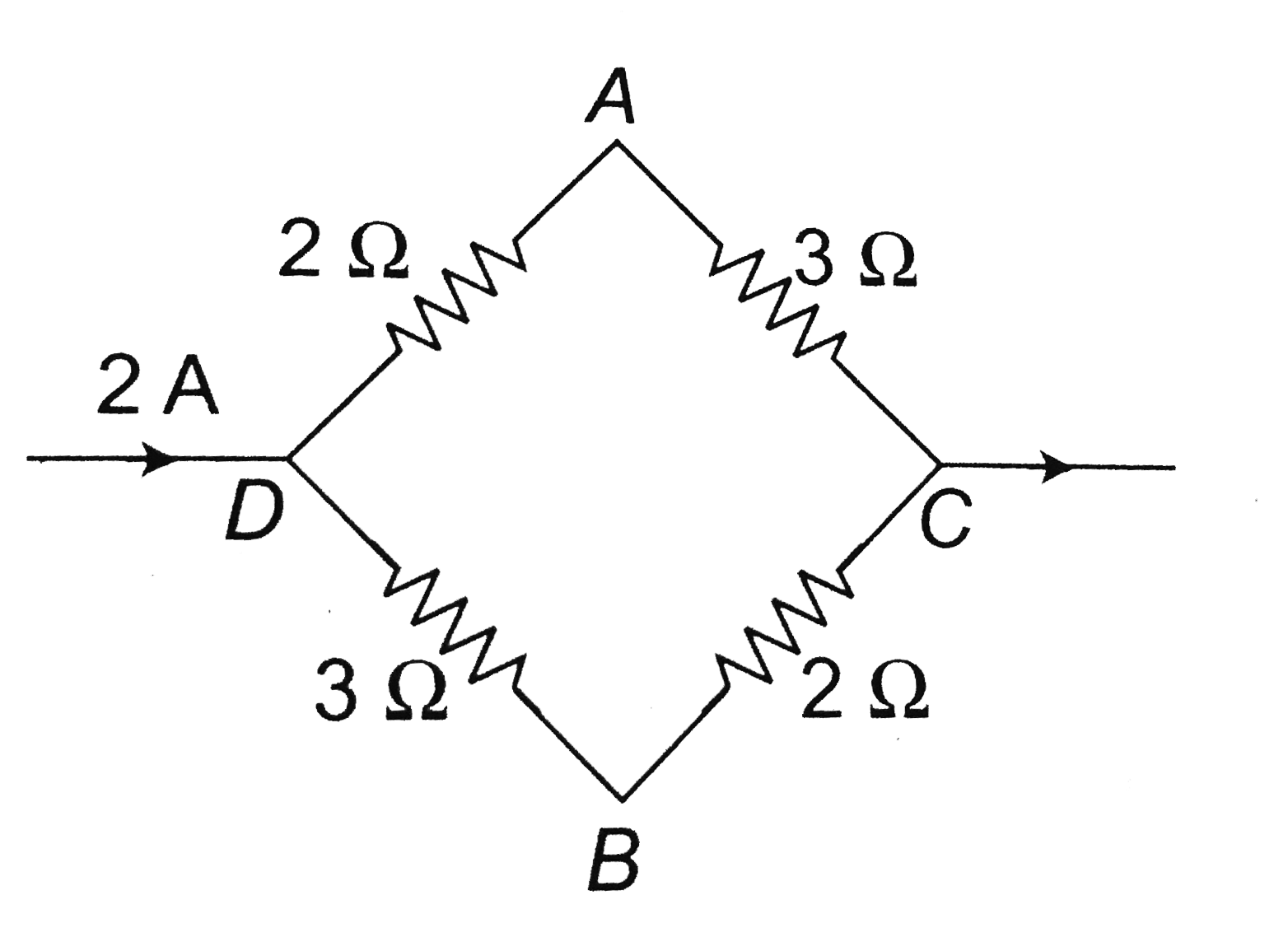 A+2VB+1VC−1VD−2V
A+2VB+1VC−1VD−2V
A current of 2A flows in a system of conductor as shown. The potential difference (VA−VB)

View SolutionA current of 2A flows in a system of conductor as shown. The potential difference (VA−VB)

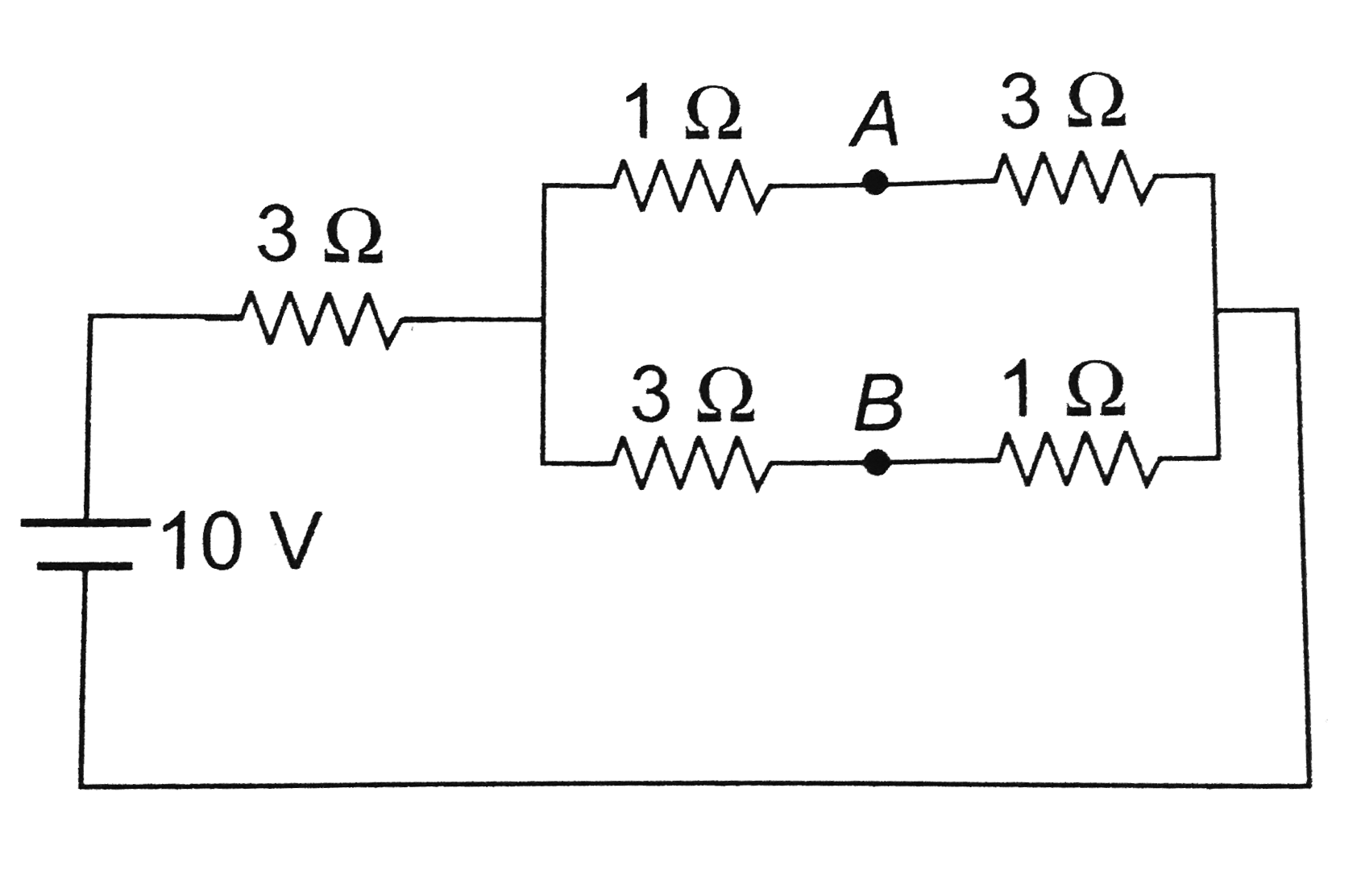
View SolutionA battery of e.m.f. 10V is connected to resistance as shown in figure. The potential difference VA−VB between the point A and B is
View SolutionThe work done in carrying an electron from point A to a point B in an electric is 10 MJ. The potential difference (VB−VA) is then
View SolutionA battery of e.m.f. 10V is connected to resistance as shown in figure. The potential difference VA−VB between the point A and B is

View Solution
