A thick glass slab of refractive index is kept in front of a plane mirror. Find the position of the image (relative to mirror) as seen by an observer through the glass slab when a point object is kept in air at a distance of from the mirror.
To find the position of the image as seen by an observer through a glass slab in front of a plane mirror, we can follow these steps:
Step 1: Understand the setup
We have a plane mirror and a glass slab of thickness 20 cm and refractive index 1.5 placed in front of it. A point object is located in air at a distance of 40 cm from the mirror.
Step 2: Calculate the shift due to the glass slab
The effective shift caused by the glass slab can be calculated using the formula:
Shift=t(1−1μ)
where t is the thickness of the glass slab and μ is the refractive index.
Given:
- t=20 cm
- μ=1.5
Substituting the values:
Shift=20(1−11.5)=20(1−23)=20(13)=203 cm
Step 3: Calculate the apparent position of the object
The object is located 40 cm from the mirror. Due to the shift caused by the glass slab, the effective distance of the object from the mirror will be:
Effective distance=Distance from mirror−Shift
Effective distance=40−203=1203−203=1003 cm
Step 4: Find the position of the image
In a plane mirror, the image is formed at the same distance behind the mirror as the object is in front of it. Therefore, the image position relative to the mirror will be:
Image position=Effective distance=1003 cm
Step 5: Adjust for the shift through the glass slab
Since the observer sees the image through the glass slab, we need to account for the shift again:
Final image position=Effective distance−Shift
Final image position=1003−203=803 cm
Thus, the position of the image as seen by the observer through the glass slab is:
Final image position=803 cm from the mirror
Final Answer
The position of the image relative to the mirror is 803 cm.
---
We have a plane mirror and a glass slab of thickness 20 cm and refractive index 1.5 placed in front of it. A point object is located in air at a distance of 40 cm from the mirror.
The effective shift caused by the glass slab can be calculated using the formula:
Shift=t(1−1μ)
where t is the thickness of the glass slab and μ is the refractive index.
- t=20 cm
- μ=1.5
Shift=20(1−11.5)=20(1−23)=20(13)=203 cm
The object is located 40 cm from the mirror. Due to the shift caused by the glass slab, the effective distance of the object from the mirror will be:
Effective distance=Distance from mirror−Shift
Effective distance=40−203=1203−203=1003 cm
In a plane mirror, the image is formed at the same distance behind the mirror as the object is in front of it. Therefore, the image position relative to the mirror will be:
Image position=Effective distance=1003 cm
Since the observer sees the image through the glass slab, we need to account for the shift again:
Final image position=Effective distance−Shift
Final image position=1003−203=803 cm
Final image position=803 cm from the mirror
The position of the image relative to the mirror is 803 cm.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Solved Examples
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.1
14 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK
1 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
Similar Questions
A point object (A) is kept at a distance (L) from a convex mirror on its principal axis. A glass slab is inserted between the object and the mirror with its refracting surfaces perpendicular to the principal axis of the mirror. The thickness of the slab is 6 cm and its refractive index is 32. The image formed after refraction through the slab, reflection from the mirror followed by refraction through the slab is a virtual image at a distance of 10 cm from the pole of the mirror (on its principal axis). Consider paraxial rays only and calculate the distance (L) of the object from the mirror. Focal length of the mirror is f=20cm.
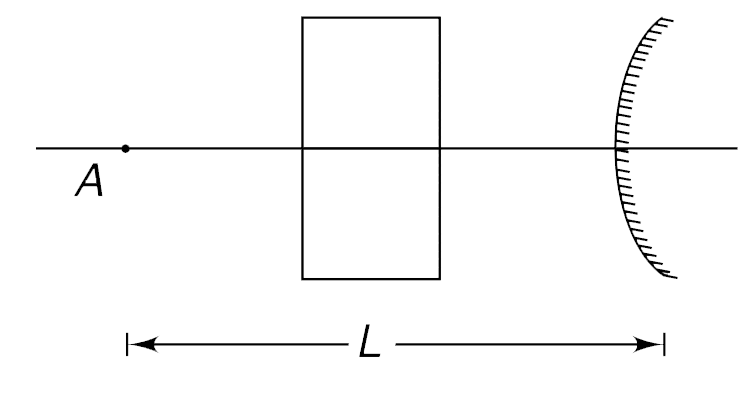
View SolutionA glass slab of thickness 3cm and refractive index 1.5 is placed in front of a concave mirror of focal length 20cm. Where should a point object be placed if it is to image on to itself?
View Solution
Knowledge Check
An object is placed at a distance of 10 cm in front of a plane mirror, then the distance of image from mirror is:
A5 cmB10 cmC20 cmD0A slab of glass, of thickness 6 cm and refractive index μ=1.5 is placed in front of a concave mirror as shown in the figure. If the radius of curvature of the mirror is 40 cm and the reflected image coincides with the object, then the distance of the object from the mirror is
 A30 cmB22 cmC42 cmD38 cm
A30 cmB22 cmC42 cmD38 cmAn object is placed 10 cm in front of a convex mirror of focal length 20 cm . The distance of the image from the mirror is
A10/3 cmB20/3 cmC10 cmD40/3 cm
A flat glass slab of thickness 6cm and index 1.5 is placed in front of a plane mirror . An observer is standing behind the glass slab and looking at the mirror. The actual distance of the observer from the mirror is 50cm. The distance of his image from himself, as seen by the observer is
View SolutionA flat glass slab of thickness 6cm and index 1.5 is placed in front of a plane mirror. An observer is standing behind the glass slab and looking at the mirror. The actual distance of the observer from the mirror is 50cm. The distance of his image from himself, as seen by the observer is
View SolutionA biconvex lens of focal length 15 cm is in front of a plane mirror. The distance between the lens and the mirror is 10 cm. A small object is kept at a distance of 30 cm from the lens. The final image is
View SolutionA biconvex lens of focal length 15 cm is in front of a plane mirror. The distance between the lens and the mirror is 10 cm. A small object is kept at a distance of 30 cm from the lens. The final image is
View SolutionAn object is placed 21 cm in front of a concave mirror of radius of curvature 10cm. A glass slab of thickness 3cm and refractive index 1.5 is then placed close to the error in the space between the object and the mirror. The distance of the near surface of the slab from the mirror is 1cm. The final image from the mirror will be formed at
View Solution
