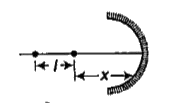
A short linear object is placed at a distance u along the axis of a spherical mirror of focal length f.
a. Obtain an expression for the longitudinal magnification.
b. Also, obtain an expression for th eration of the velocity of image (v) to the velocity of the object (u).

Video Solution

Text Solution

Verified by Experts

a. Mirror formula $: \frac{1}{v} + \frac{1}{u} = \frac{1}{f}$
On differenciating both sides, we get
$- \frac{d v}{v^{2}} - \frac{d u}{u^{2}} = 0 or d v = - d u {(\frac{v}{u})}^{2}$
Since $\frac{v}{u} = \frac{f}{u - f}$ , therefore
$d v = - d u {(\frac{f}{u - f})}^{2}$
Lateral magnification, $m_{2} = \frac{d v}{d u} = - {(\frac{f}{u - f})}^{2}$
The negative sign shows that the image is longitudinally inverted.
b. Since velocity of object, $U = \frac{d u}{d t}$
and velocity of image,
$V = \frac{d v}{d t}$
$∴ V = - U {(\frac{f}{u - f})}^{2}$ or $\frac{V}{U} = - {(\frac{f}{u - f})}^{2}$
The negative sign shows that the object and image always move in opposite directions.
$v = \frac{- x f_{0}}{f_{0} - x}$
v becomes negative (real image) only when $x < f_{0}$ .

Updated on:21/07/2023