A point object O is placed in front of a concave mirror of focal length 10cm. A glass slab of refractive index μ=3/2 and thickness 6cm is inserted between the object and mirror.
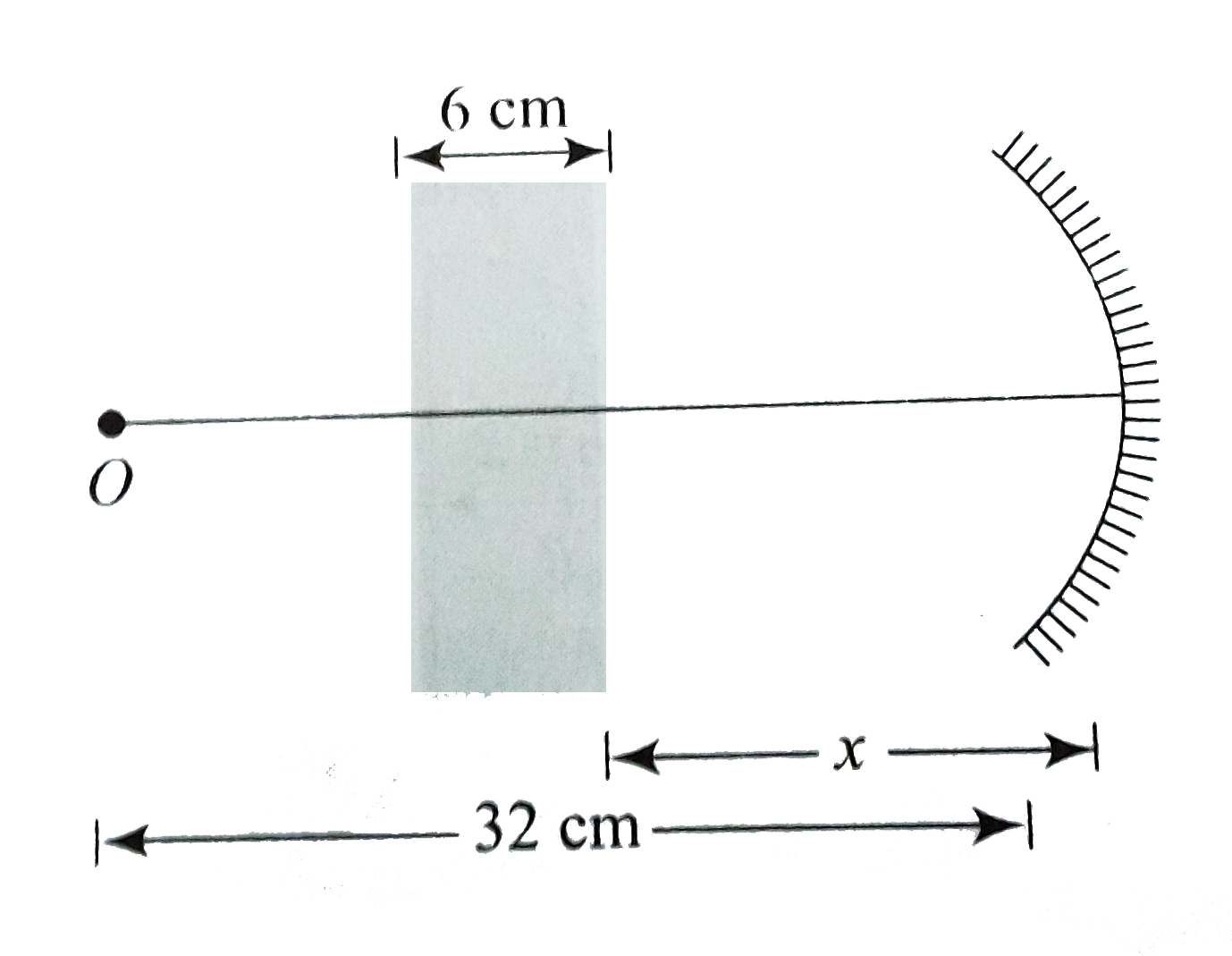
Q. Find the position and nature of the final image when the distance x shown in figure, is 5cm.
11cm, virtual
17cm, real
14cm, real
20cm, virtual
The correct Answer is:b.
The normal shift produced by a gless slab is,
Δx=(1−1μ)t=(1−23)(6)=2cm
i.e., for the mirror, the object is placed at a distanced (32−Δx)=30cm from it.
Applying mirror formula,
1v+1u=1f
1v+130=−110
or, v=−15cm
a. when x=5cm: The light falls on the slab on its return journey as shown. But the slab will again shift it by a distance
Δx=2cm . Hence the final real image is formed at a distance (15+2)=17cm from the mirror.
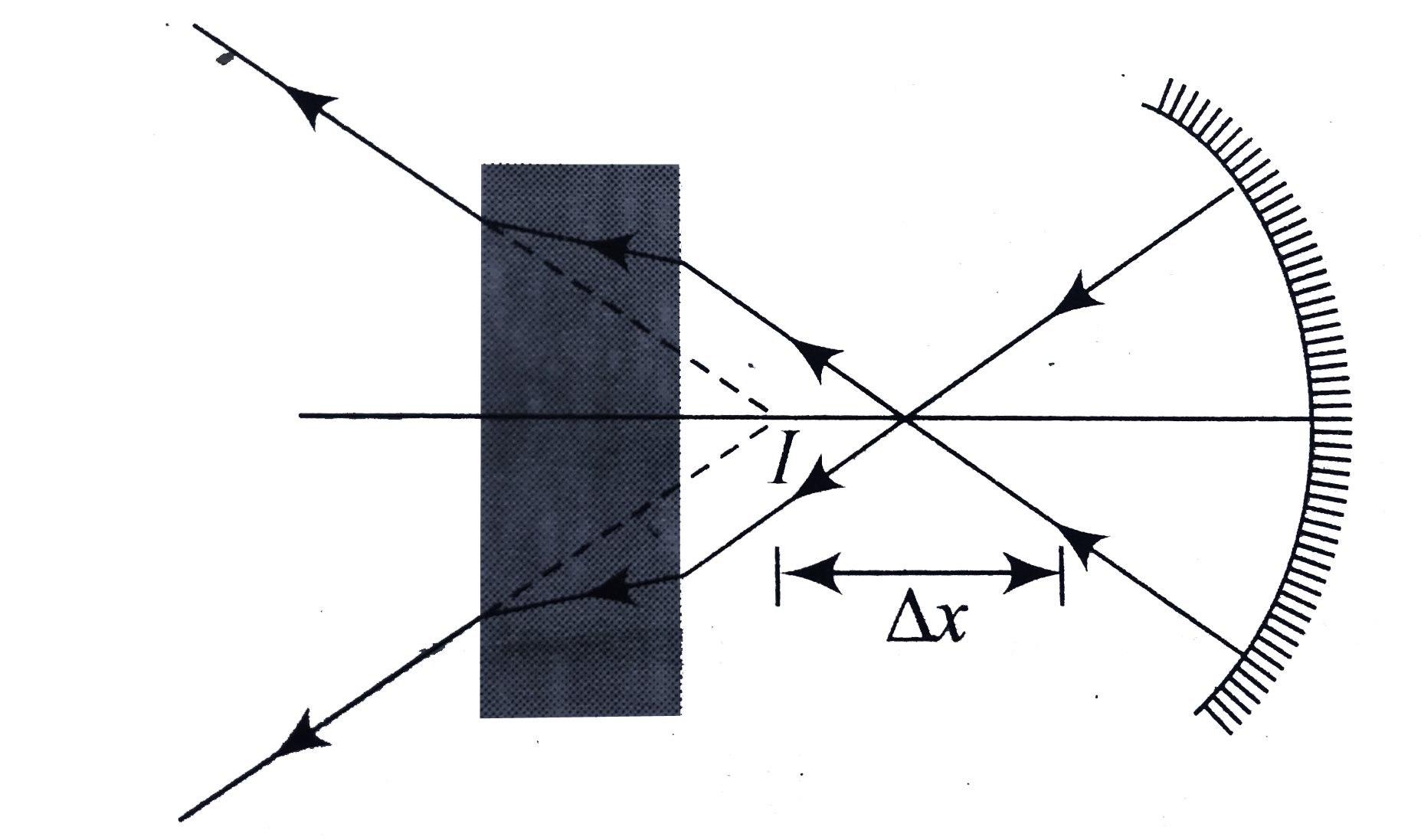
b. When x=20cm: This time also the final image is at a distance of 17cm from the mirror but it is virtual as shown.
BMS_V04_C01_E01_383_S02.png" width="80%">
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Integer
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Archives
7 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICSCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- FRICTIONBOOK - CENGAGE PHYSICSCHAPTER - FRICTIONEXERCISE - QUESTION BANK
1 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - Question Bank
39 Videos
Similar Questions
A point object O is placed in front of a concave mirror of focal length 10cm. A glass slab of refractive index μ=3/2 and thickness 6cm is inserted between the object and mirror.
Find the position of final image when the distance x shown in Fig... is, (a) 5cm and (b) 20 cm.
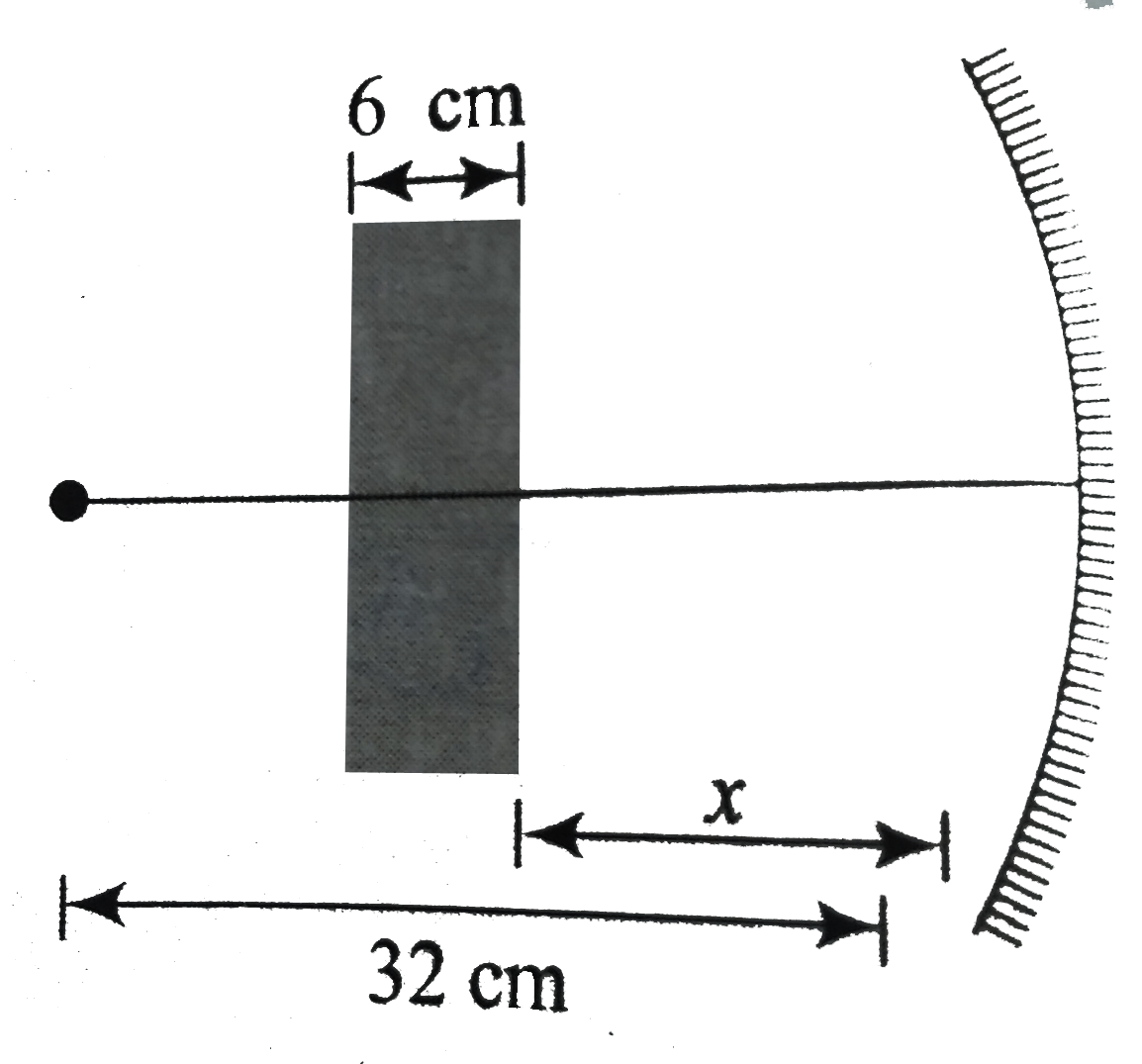
View SolutionA point object O is placed in front of a concave mirror of focal length 10cm. A glass slab of refractive index μ=3/2 and thickness 6cm is inserted between the object and mirror.
Find the position of final image when the distance x shown in Fig... is, (a) 5cm and (b) 20 cm.

View Solution
Knowledge Check
A point object O is placed in front of a concave mirror of focal length 10cm. A glass slab of refractive index μ=3/2 and thickness 6cm is inserted between the object and mirror.
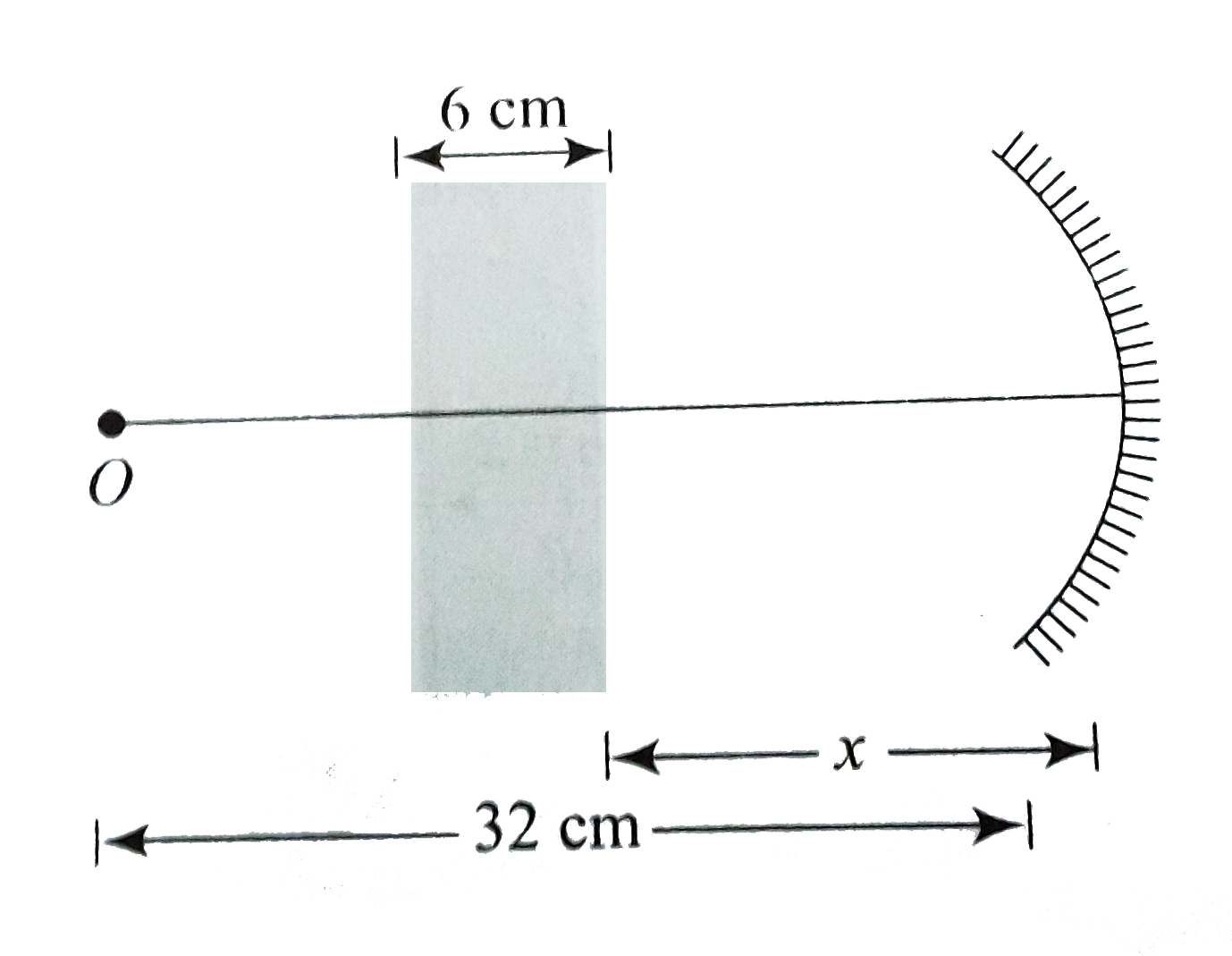
Q. Find the position and nature of the final image when the distnace x shown in figure, is 20cm.A17cm, virtualB17cm, realC12cm, virtualD15cm, virtualAn object is placed 10 cm in front of a convex mirror of focal length 20 cm . The distance of the image from the mirror is
A10/3 cmB20/3 cmC10 cmD40/3 cmAn object is placed at 50 cm in front of a concave mirror of focal length 25 cm. What is the nature of the image produced by the mirror ?
Areal and erect and of the same sizeBvirtual and inverted and bigger in sizeCreal, inverted and of the same sizeDreal, inverted and smaller in size
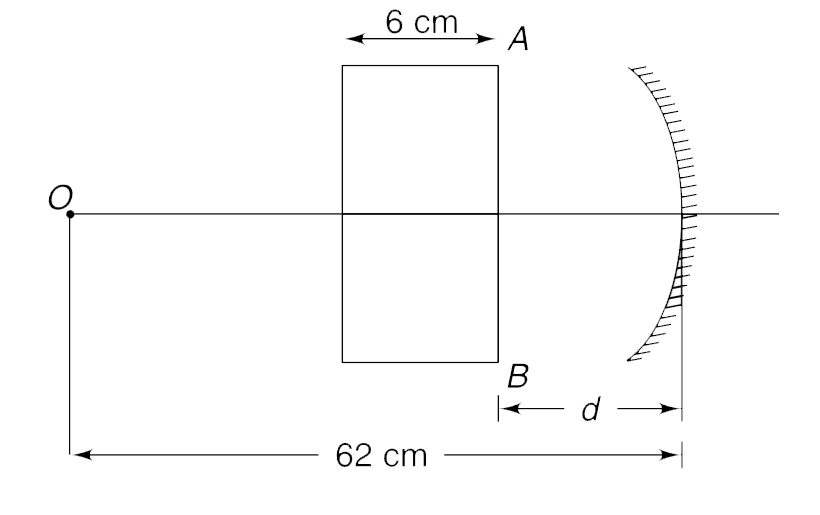
A point object O is placed at a distance of 62 cm in front of a concave mirror of focal lengthf=20cm. A glass slab of refractive index μ=32 and thickness 6 cm is inserted between the object and the mirror. Let’s define final image as image formed after the light ray originating from O passes through the slab, gets reflected from the mirror and then again passes through the slab. At what distance d from the mirror, the face AB of the slab can be placed so that the final image is formed inside the slab itself?
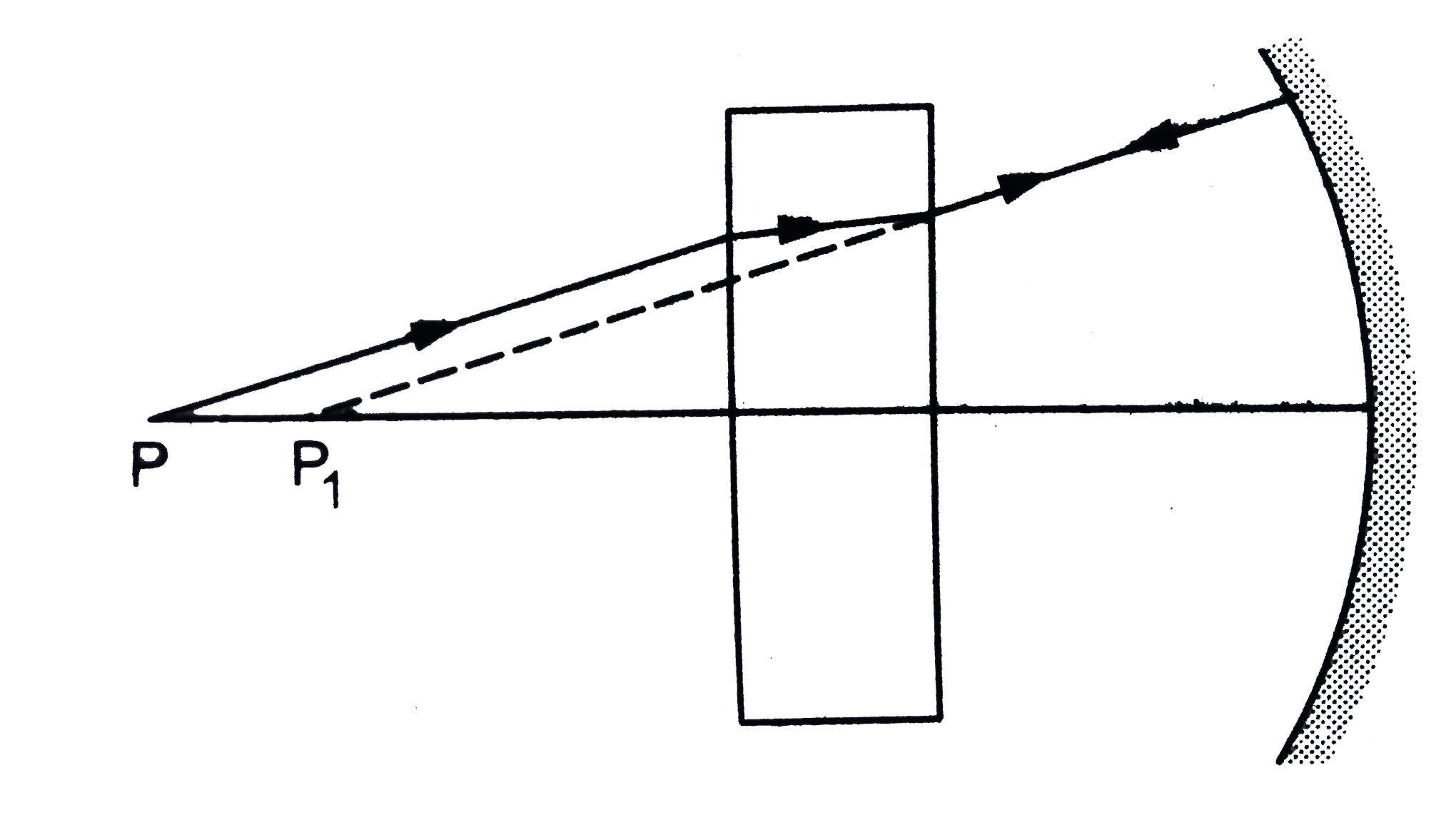
View SolutionAn object is placed 21 cm in fron of a concave mirror of radius of curvature 20 cm.A glass slab of thicknes 3 cm and refractive index 1.5 is palced close to the mirror in the space between the object and the mirror. Find the position of the final image fromed. The distance of the nearer surface of the slab from the mirror is 10 cm.
View SolutionAn object is placed at 6 cm distance in front of a concave mirror of focal length 4 cm.
What will be the nature of the image?
View SolutionAn object is placed at 6 cm distance in front of a concave mirror of focal length 4 cm.
Find the position of the image period.
View SolutionA point object is placed at a distance 30 cm in front of a concave mirror of focal length 20 cm. Find the nature and location of image obtained.
View Solution
