In Young's experiment, light of wavelength 600 nm falls on the double slits separated by 0.1 mm. What is the highest order of maximum intensity in the interference pattern obtained on a screen kept 3 m from the slits? How does the highest order change if the distance of screen from the slits is changed?
As we know, nmax=dλ
Given d=0.1mm=0.1×10−3m
and λ=600nm=600×10−9m.
∴nmax=0.1×10−3600×10−9=166.67⇒nmax=166
Obviously, nmax=d/λ does not depend on the distance of screen form the slits.
Hence, it remains 166 if the distance between the slits and the screen is changed.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Solved Examples10videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.112videos
WAVE OPTICS
- BOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK34videos
THERMODYNAMICS
- BOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK38videos
WAVES
Similar Questions
In Young's experiment performed with light or wavelength 5500 Å what should be the separation between the two slits so that the highest order of maximum intensity in the interference pattern is 2 ? You may assume D>>d.
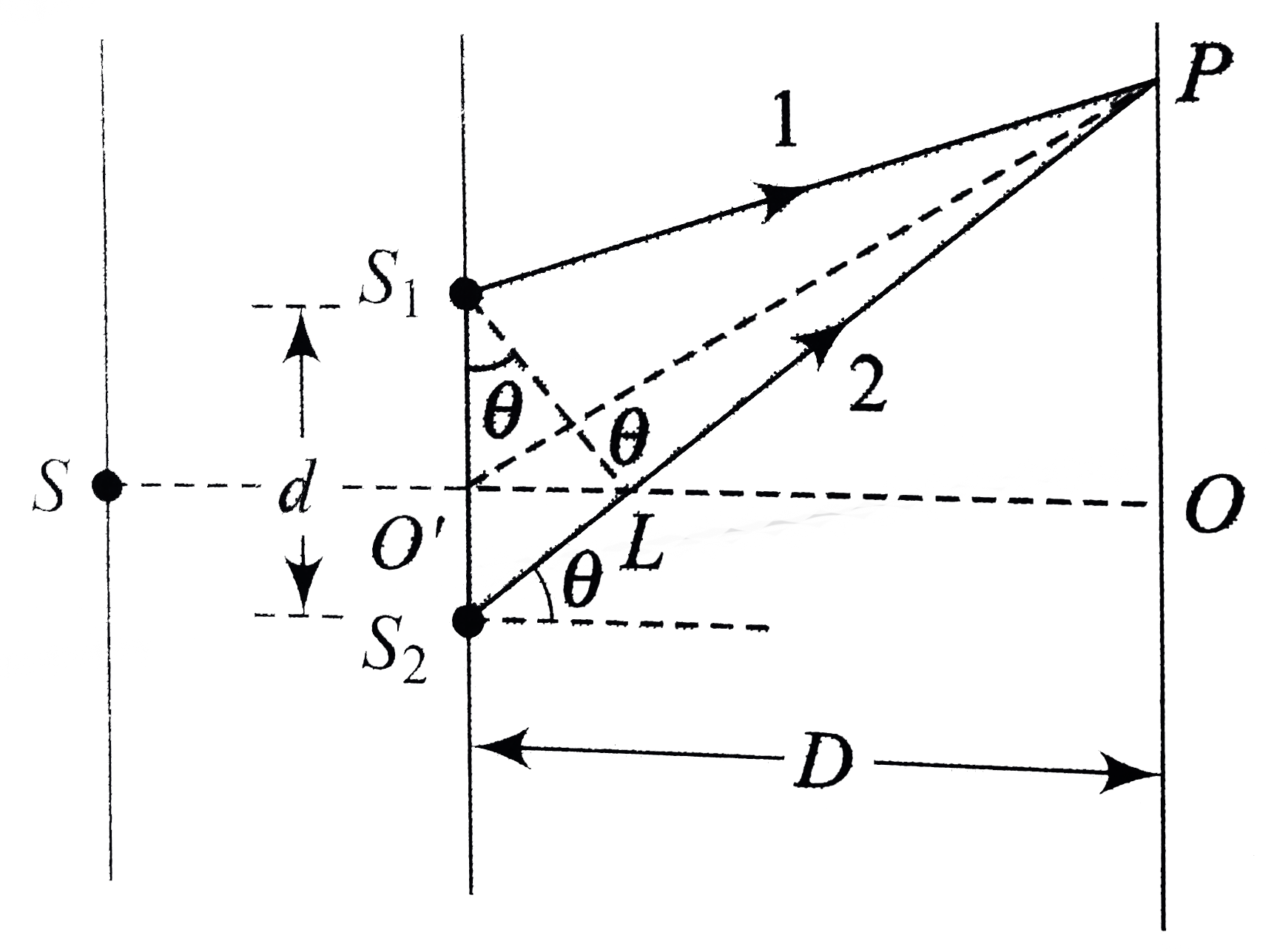 View Solution
View SolutionTwo slits 4.0×10−6 m apart are illuminated by light of wavelength 600nm . What is the highest order fringe in the interference pattern?
View Solution
Knowledge Check
- Question 1 - Select One
The figure shows the interference pattern obtained in a double-slit experiment using light of wavelength 600 nm.
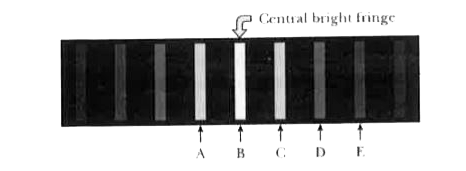
Which fringe is the same distance from both slits?AABCCBDD - Question 2 - Select One
In Young's double slit experiment the two slits are d distance apart. Interference pattern is observed on a screen at a distance D from the slits. A dark fringe is observed on the screen directly opposite to one of the slits. The wavelength of light is
AD22dBd22DCD2dDd2D - Question 3 - Select One
In Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
A25B12C15D20
In Young's experiment the slits, separated by d=0.8mm, are illuminated with light of wavelength 7200 Å, Interference pattern is obtained on a screen D=2m from the slits. Find minimum distance from central maximum at which the a average intensity is 50% if of maximum?
View SolutionIn single slit diffraction pattern, how does the angular separation between firnges change when distance of screen from slit is doubled ?
View SolutionIn Young's double slit experiment , red light of wavelength 620 nm is used and the two slits are 0.3 mm apart . Interference fringes are observed on a screen 1.3 mm apart . Calculate (i) the distance of slits from the screen and (ii) the fringe width if this distance is doubled .
View SolutionA source emitting light of wavelengths 480 nm and 600 nm is used in a double slit interference experiment. The separation between the slits is 0.25 mm and the interference is observed on a screen placed at 150 cm from the slits. Find the linear separation between the first maximum (next to the central maximum) corresponding to the two wavelengths.
View SolutionIn Young's double-slit experiment, light of wavelength 600 nm is used. When the slits are kept at a distance of 1mm, interference pattern is observed at a diatance of 1m. What will be the distance between central maxima and fringe with intensity equal to one-forth of maximum intensity.
View Solution

