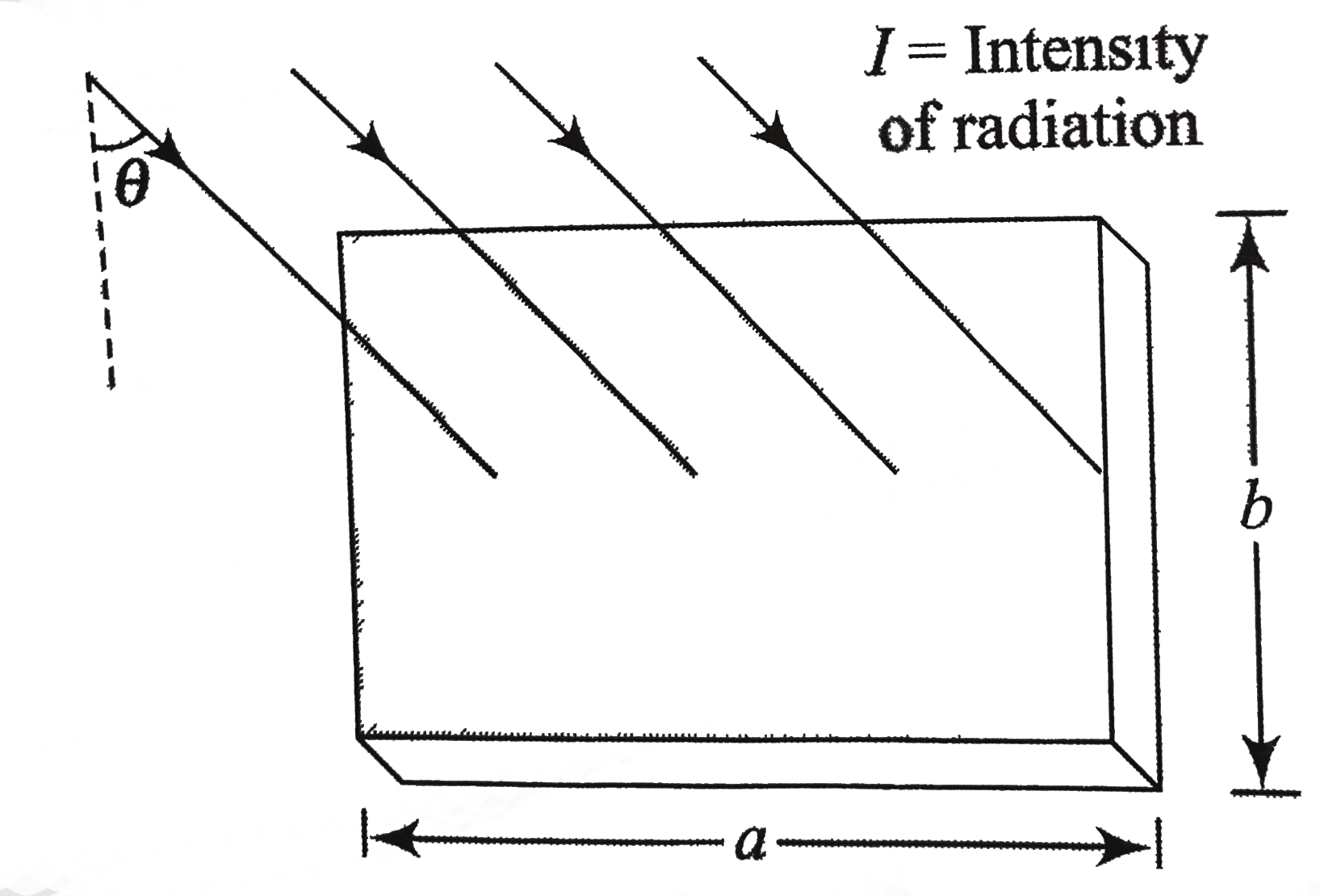
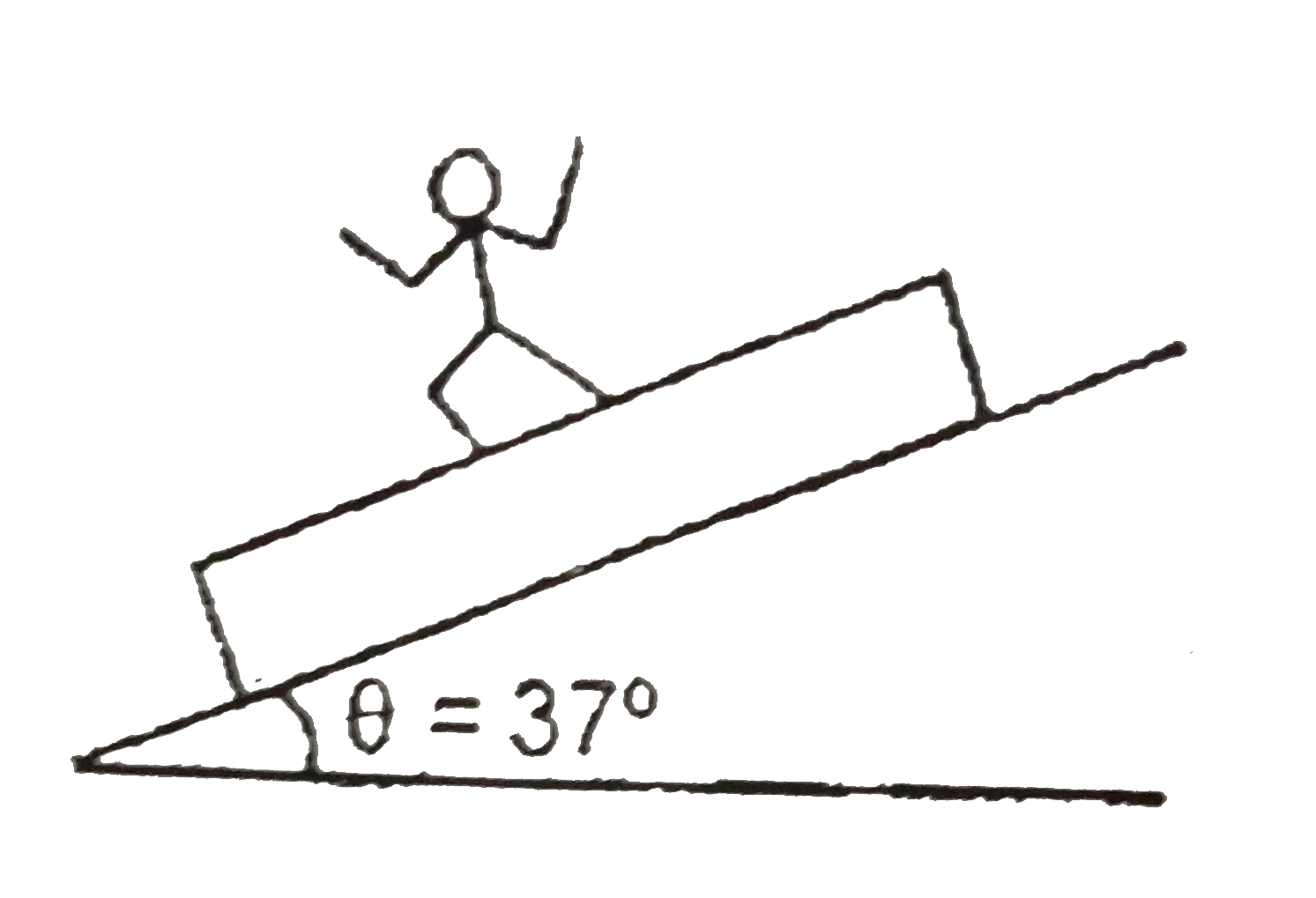
A plank of mass m is lying on a rough suface having coeffient of frinction as μ is situation as shown in fig Find the acceleration of the plank assuming that it slips and the surface of body exposed to radiaton is black body.
E= Energy received by surface per second is
IAcosθ=Iabcosθ
Number of photons reveived by the surface per second ,
N=Ehcλ=Iabcosθλhc
△Pone photon)=hλ
⇒fradian=△Ponephoton N=Iabcosθc
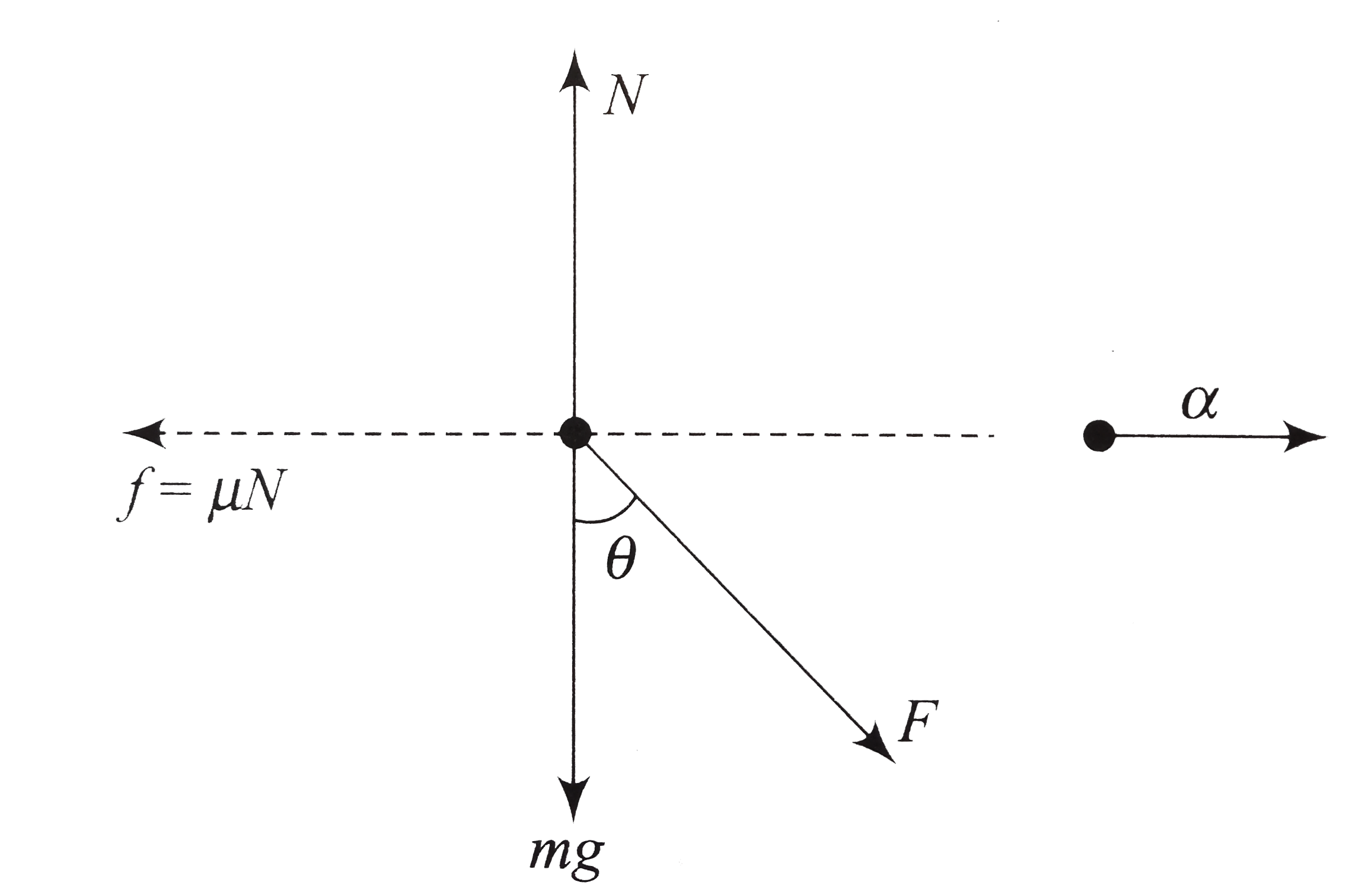
Let us draw the FBD of the plank (see fig.)
Fsinθ−μN=mα...(i)
N=mg+Fcosθ...(ii)
From Eqs. (i) and (ii), we get
α=Fsinθ−μ(mg+Fcosθ)m
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Examples8videos
PHOTOELECTRIC EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Exercise 3.115videos
PHOTOELECTRIC EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - NUCLEIEXERCISE - QUESTION BANK59videos
NUCLEI
- BOOK - CENGAGE PHYSICSCHAPTER - RAY OPTICSEXERCISE - DPP 1.612videos
RAY OPTICS
Similar Questions
A body of mass m slides down a rough plane of inclination α . If μ is the coefficient of friction, then acceleration of the body will be
View SolutionA plank of mass M is placed on a rough horizontal force F is applied on it A man of mass m rens on the plank find the acceleration of the man so that the plank does not move on the surface . The coefficent of friction between the plank and the surface is μ Assume that the man does not slip on the plank
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A body of mass m is placed on a rough surface with coefficient of friction μ inclined at θ. If the mass is in equilibrium , then
Aθ=tan−1μBθ=tan−1(1μ)Cθ=tan−1(m)μDθ=tan−1(μ)m - Question 2 - Select One
A body of mass m slides down a rough plane of inclination α . If μ is the coefficient of friction, then acceleration of the body will be
AgsinαBμcosαCg(sinα−μcosα)Dg(cosα−μsinα) - Question 3 - Select One
A block of mass m lying on a rough horizontal surface of friction coefficient μ is pulled by a force F as shown , the limiting friction between the block and surface will be
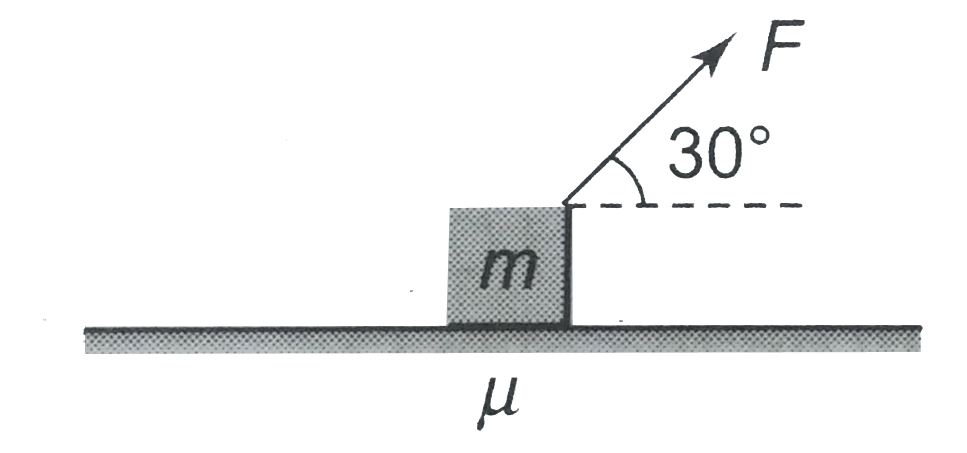 AμmgBμ(mg+F2)Cμ(mg−F2)Dμ(mg−√3F2)
AμmgBμ(mg+F2)Cμ(mg−F2)Dμ(mg−√3F2)
A plank of mass M is placed on a rough horizontal surface and a contant horizontal force F is applied on it . A man of mass m runs on the plank find the range of acceleration of the man that the plank does not move on the surface is μ .Assume that the does not slip on the plank
 View Solution
View Solution
Consider a cylinder of mass M and radius R lying on a rough horizontal plane. It has a plank lying on its top as shown in figure. A force F is applied on the plank such that the plank moves and causes the cylinder to roll the plank always remains horizontal. there is no slipping at any point of contact. Calculate the acceleration of the cylinder and the frictional forces at the two contact.View SolutionIn the Fig. shown, mass 'm' is being pulled on the incline of a wedge of mass M. All the surface are smooth. Find the acceleration of the wedge.
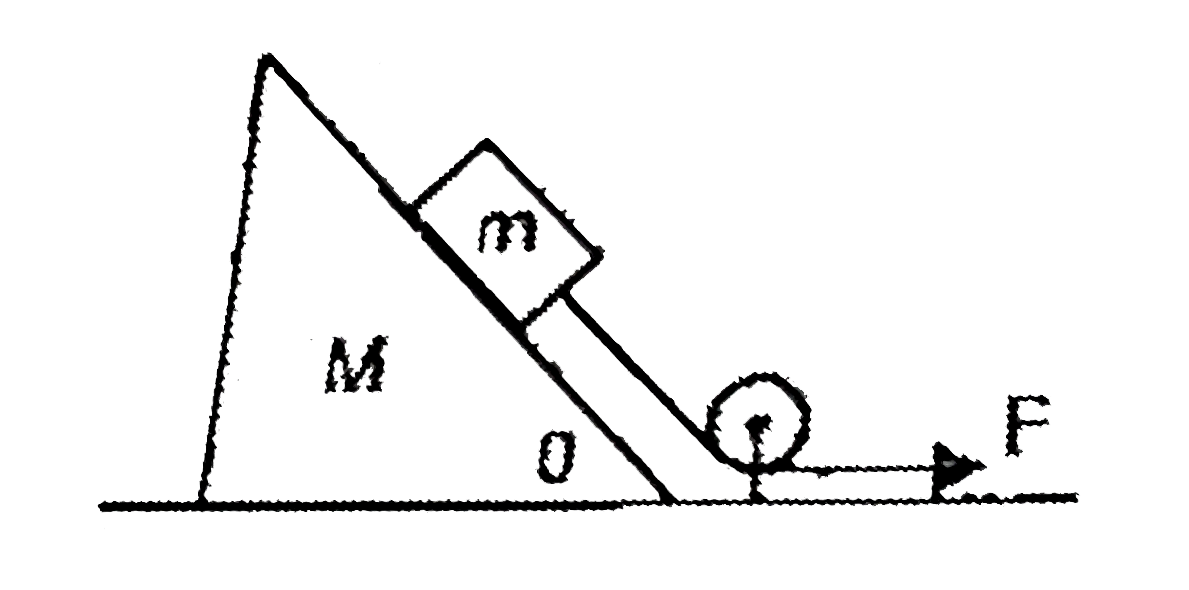 View Solution
View Solutionfind the acceleration of the block of mass M in the situation shown in figure. All the surfaces are frictionless and the pulleys and the string are light.
 View Solution
View SolutionA plank of mass 3m is placed on a rough inclined plane and a man of mass m, the coefficient of friction between the board and inclined plane is μ=0.5, the minimum acceleration of man so that plank does not slide is :
 View Solution
View Solution

