A neutron with an energy of $4.6 M e V$ collides elastically with proton and is retarded. Assuming that upon each collision the neutron is deflected by $45^{\circ}$ , find the number of collisions which will reduce its energy to $0.23 e V$ .

Video Solution

Text Solution

Verified by Experts

Mass of neutron-mass of proton $= m$

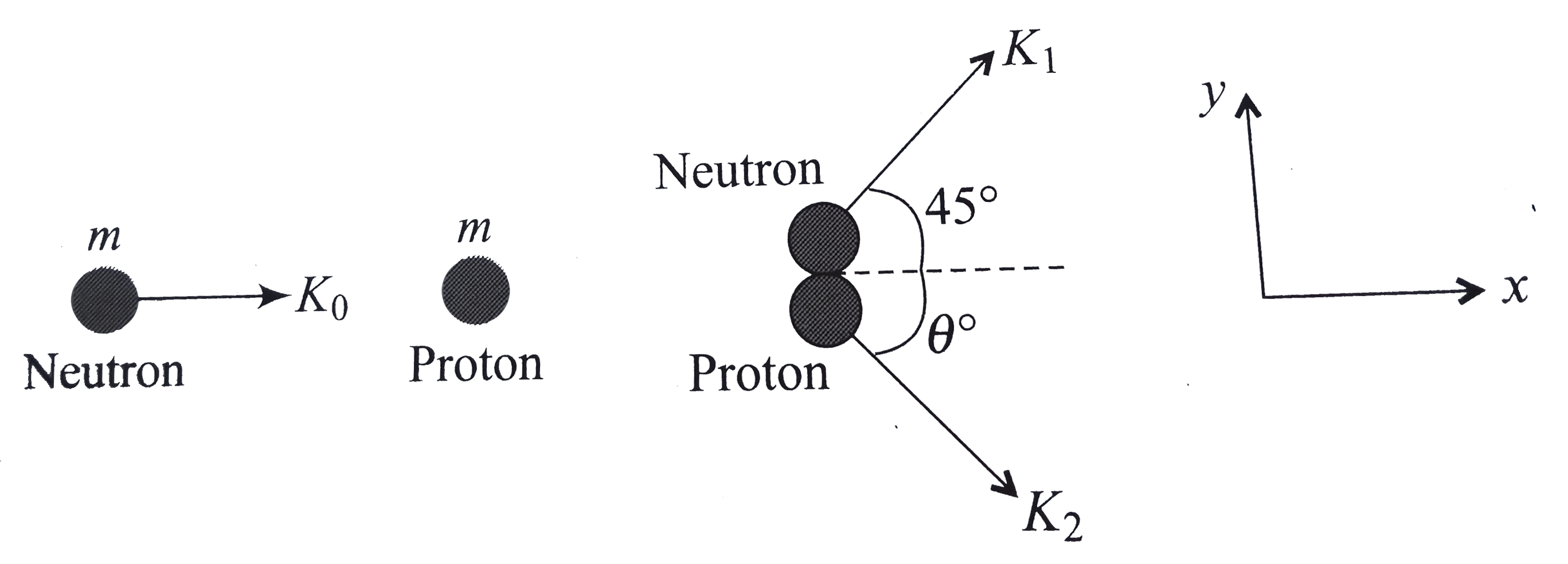
From conseervation of momentum in y-direction,
$\sqrt{2 m K_{1}} {sin 45}^{\circ} = \sqrt{2 m K_{2}} sin θ$ …(i)
In x-direction
$\sqrt{2 m K_{0}} = \sqrt{2 m K_{1}} {cos 45}^{\circ} + \sqrt{2 m K_{2}} cos θ$ …(ii)
Squarring and adding Eqs, (i) and (ii), we have
$K_{2} = K_{1} + K_{0} - \sqrt{2 K_{0} K_{1}}$ ...(iii)
From conseervation of energy ,
$K_{2} = K_{0} + K_{1}$ [as collision is elastic] ...(iv)
Solving Eqs, (iii) and (iv), we get
$K_{1} = \frac{K_{0}}{2}$
i.e. after each collision energy remain half. Therefore , after $n$ collision ,
$K_{n} = K_{0} {(\frac{1}{2})}^{n}$
$∴ 0.23 = (4.6 \times 10^{6}) {(\frac{1}{2})}^{n}$ $2^{n} = \frac{4.6 \times 10^{6}}{0.23}$
Taking log and solving, we get
$n = 24$

Updated on:21/07/2023