A wooden cubical block ABCDEFG of mass m and side a is wrapped by a square wire loop of perimeter 4a, carrying current I. The whole system is placed at frictionless horizontal surface in a uniform magnetic field →B=B0ˆj as shown in fig. In this situation, normal force between horizontal surface and block passes through a point at a distance x from center. Choose correct statement (s).

The block must not topple if I<mgaB0
The block must not topple if I<mg2aB0
x=a4ifI=mg2aB0
x=a4ifI=mg4aB0
The correct Answer is:(b,d)
Torque of magnetic field : τ=MB0=Ia2B0
For rotation equilibrium: τ=mgx⇒Ia2B0=mgx
⇒x=Ia2B0mg
For the block not to topple:
x<a2⇒I<mg2aB0

Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Assertion -reasoning
8 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Linked Comprehension
28 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Single Correct
70 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
- Magnetism and MatterBOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank
50 Videos
Similar Questions
A wooden cubical block ABCDEFG of mass m and side a is wrapped by a square wire loop of perimeter 4a, carying current I. The whole system is placed at frictionless horizontal surface in a uniform magnetic field →B=B0ˆj as shown in fig. In this situation, normal force between horizontal surface and block passes through a point at a distance x from centre. Choose correct statement (s).

View SolutionA wooden cubical block ABCDEFG of mass m and side a is wrapped by a square wire loop of perimeter 4a, carrying current I. The whole system is placed at frictionless horizontal surface in a uniform magnetic field →B=B0ˆj as shown in figure. In this situation, normal force between horizontal surface and clock passes through a point at a distance x from centre. Choose correct statement (s).

View Solution
Knowledge Check
A wooden cubical block ABCDEFG of mass m and side a is wrapped by a square wire loop of perimeter 4a, carrying current I. The whole system is placed at frictionless horizontal surface in a uniform magnetic field →B=B0ˆj as shown in fig. In this situation, normal force between horizontal surface and block passes through a point at a distance x from center. Choose correct statement (s).
 AThe block must not topple if I<mgaB0BThe block must not topple if I<mg2aB0Cx=a4ifI=mg2aB0Dx=a4ifI=mg4aB0
AThe block must not topple if I<mgaB0BThe block must not topple if I<mg2aB0Cx=a4ifI=mg2aB0Dx=a4ifI=mg4aB0Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

If the extension of the spring is x0 at time t, then the displacement of the second block at this instant isAFt22m−x0B12(Ft22m+x0)C12(2F2m−x0)D12(Ft22m−x0)Two blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

Then the displacement of the centre of mass in at time t isAFt22mBFt23mCFt24mDFt2m
A wooden cubical block of mass m and side a is resting on a horizontal surface. A wire carrying current I, is wrapped around it in from of a square of side a. A uniform magnetic field →B=B0→j is switched on in the region. Neglect the mass of the wire.
(a) At what distance from the x axis does normal force applied by the horizontal surface on the wooden cube act?
(b) What is the maximum value of current for which the block will not topple?

View SolutionA circular wire loop of radius R, mass m carrying current I lies on a rough surface (as shown in Fig. 1.86). There is a horizontal magnetic field →B. How large can the current I be before one edge of the loop will lift off the surface?
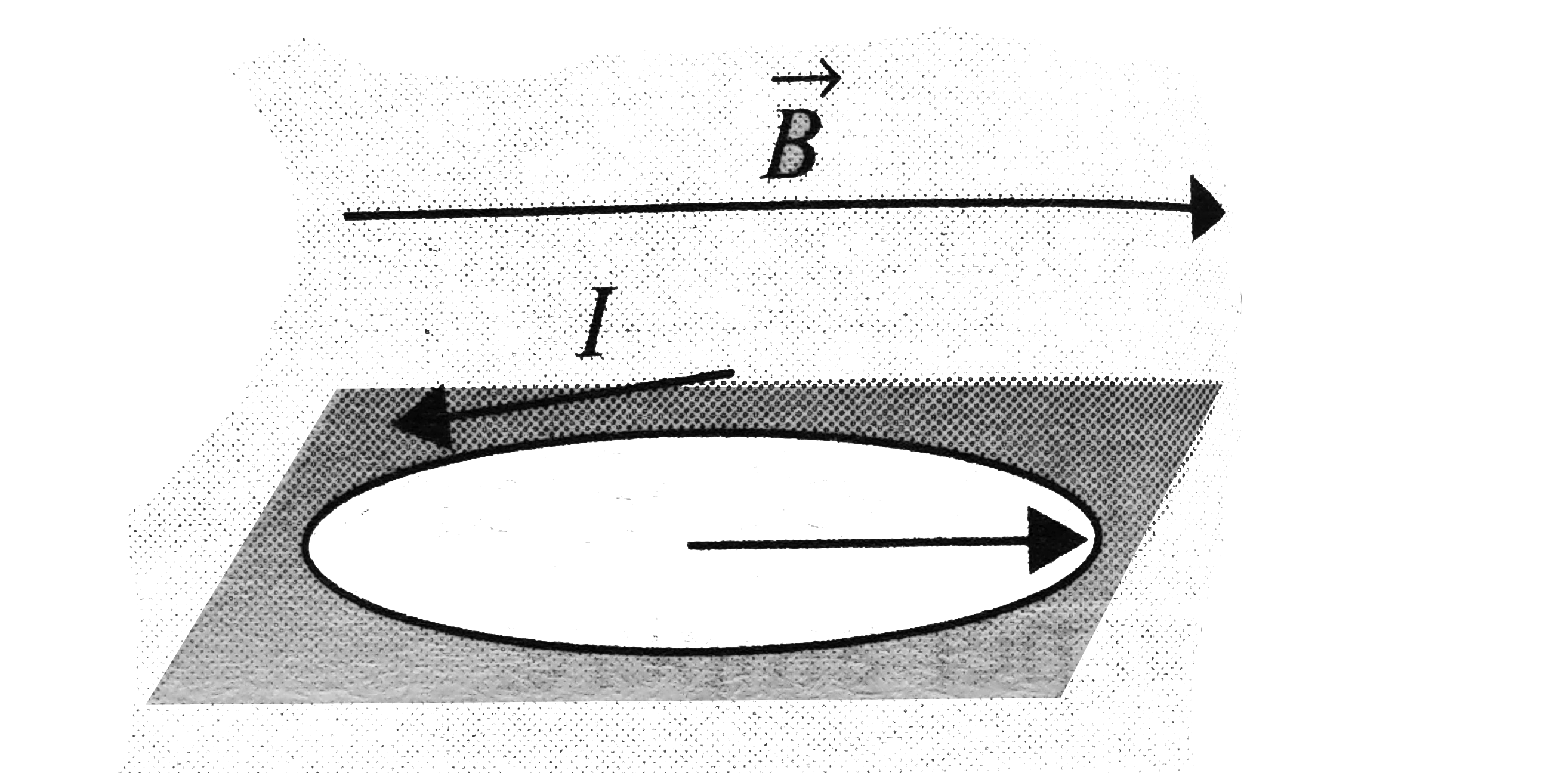
View SolutionA circular wire loop of radius R, mass m carrying current I lies on a rough surface (as shown in Fig. 1.86). There is a horizontal magnetic field →B. How large can the current I be before one edge of the loop will lift off the surface?

View SolutionTwo blocks of equal mass m are connected by an unstretched spring and the system is kept at rest on a frictionless horizontal surface. A constant force F is applied on the first block pulling away from the other as shown in Fig.

Then the displacement of the centre of mass in at time t is
View SolutionTwo blocks of masses m and 2m are placed on a smooth horizontal surface as shown in the figure. Find the acceleration of each block and normal reaction between two blocks if a horizontal force F0 is applied on (a) m and (b) 2m
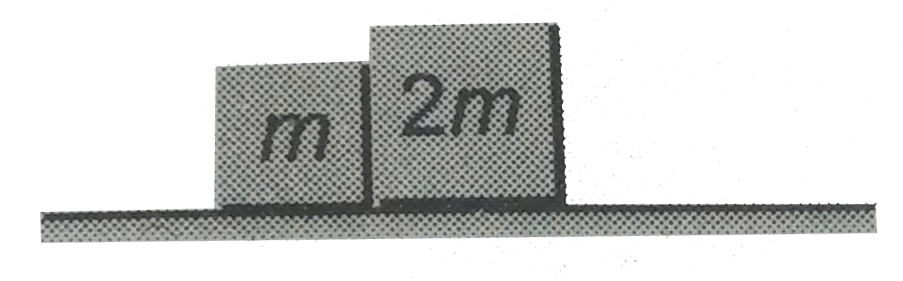
View Solution
