Two particles having identical masses and charges 2Q and Q are moving with velocities v and 2v resp. In uniform magnetic field B. Find ratio of radius of circle described by them
44228
0.084027777777778
44287
0.16736111111111
To solve the problem, we need to find the ratio of the radii of the circular paths described by two charged particles moving in a magnetic field. Let's denote the two particles as Particle A and Particle B.
1. Identify the Given Parameters:
- Charge of Particle A, qA=2Q
- Charge of Particle B, qB=Q
- Velocity of Particle A, vA=v
- Velocity of Particle B, vB=2v
- Mass of both particles, mA=mB=m (identical masses)
2. Use the Formula for the Radius of Circular Motion in a Magnetic Field:
The radius r of the circular path of a charged particle moving in a magnetic field is given by the formula:
r=mvqB
where:
- m is the mass of the particle,
- v is the velocity of the particle,
- q is the charge of the particle,
- B is the magnetic field strength.
3. Calculate the Radius for Each Particle:
- For Particle A:
rA=mvAqAB=mv2QB
- For Particle B:
rB=mvBqBB=m(2v)QB=2mvQB
4. Find the Ratio of the Radii:
To find the ratio rArB:
rArB=mv2QB2mvQB=mv2QB⋅QB2mv
Simplifying this expression:
rArB=14
5. Conclusion:
The ratio of the radius of the circle described by Particle A to the radius of the circle described by Particle B is:
rArB=14
Final Answer:
The ratio of the radius of the circles described by the two particles is 14.
- Charge of Particle A, qA=2Q
- Charge of Particle B, qB=Q
- Velocity of Particle A, vA=v
- Velocity of Particle B, vB=2v
- Mass of both particles, mA=mB=m (identical masses)
The radius r of the circular path of a charged particle moving in a magnetic field is given by the formula:
r=mvqB
where:
- m is the mass of the particle,
- v is the velocity of the particle,
- q is the charge of the particle,
- B is the magnetic field strength.
- For Particle A:
rA=mvAqAB=mv2QB
- For Particle B:
rB=mvBqBB=m(2v)QB=2mvQB
To find the ratio rArB:
rArB=mv2QB2mvQB=mv2QB⋅QB2mv
Simplifying this expression:
rArB=14
The ratio of the radius of the circle described by Particle A to the radius of the circle described by Particle B is:
rArB=14
The ratio of the radius of the circles described by the two particles is 14.
Topper's Solved these Questions
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-A
80 Videos
- JEE MAIN 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2021EXERCISE - SECTION-B
40 Videos
- JEE MAINBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINEXERCISE - All Questions
473 Videos
- JEE MAIN 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAIN 2022EXERCISE - Question
492 Videos
Similar Questions
A change q of mass m is moving with a velocity of V, at right angles to a uniform magnetic fiels B. Deduce the expression for the radius of the circular path it describes.
View SolutionA particle of mass m and positive charge q, moving with a uniform velocity v, enters a magnetic field B as shown in Find The radius of the circular are it describes in the magnetic field. (b) find the angle subtended by the arc at the centre. (c ) How long does the particle stay inside the magnetic field? (d) Solve the three parts of the above problem if the charge q on the particle is negative.
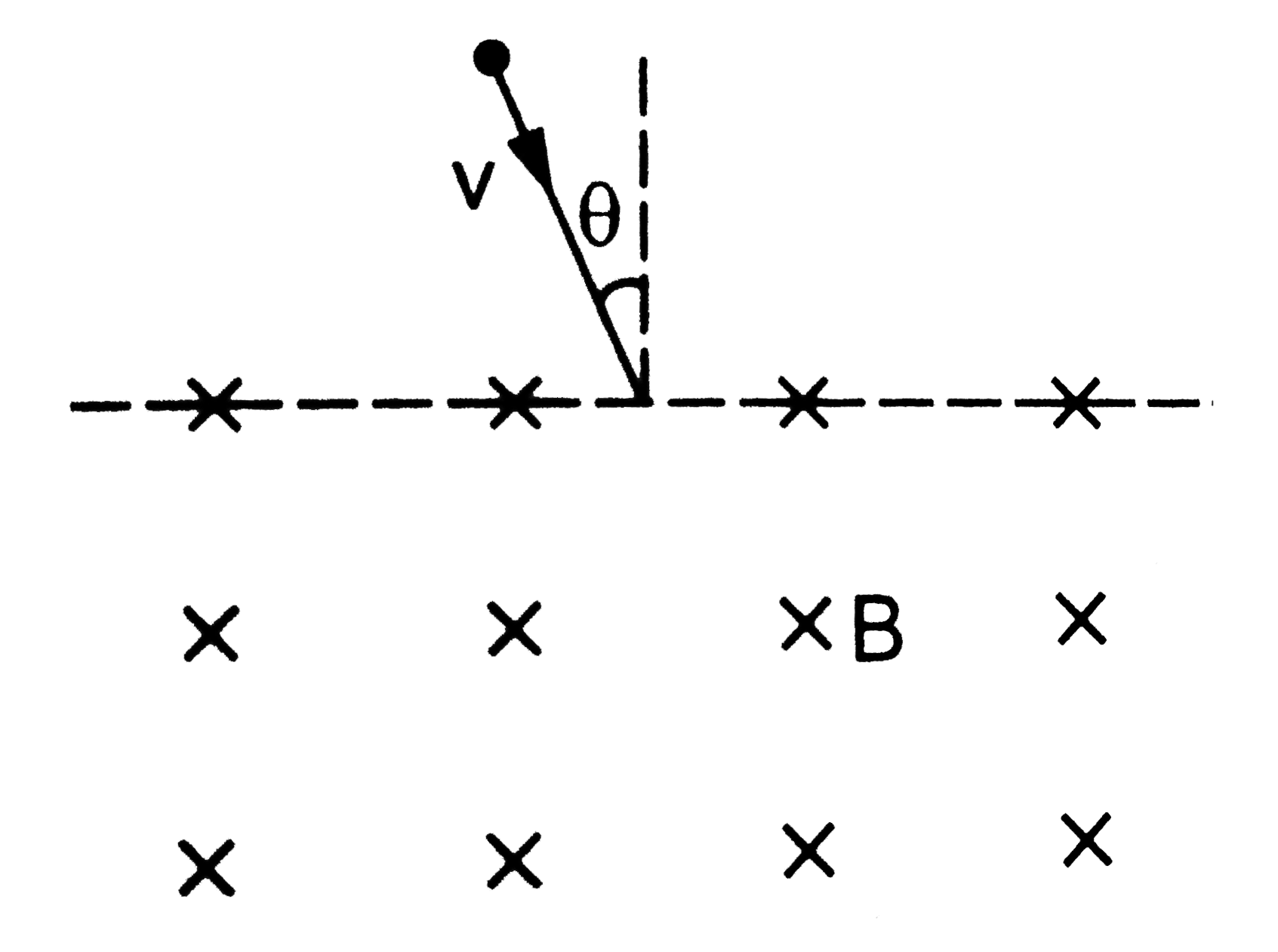
View Solution
Knowledge Check
A charged particle moves with velocity →v in a uniform magnetic field →B. The magnetic force experienced by the particle is
AAlways zeroBNever zeroCZero, if →B and →v are perpendicularDZero, if →B and →vare parallelA charge particle moves with velocity →V in a uniform magnetic field →B . The magnetic force experienced by the particle is
Aalways zeroBzero, if →Band→V are perpendicualrCzero, if →Band→V are parallelDzero, if →Band→V are inclined at 45∘Two identical particles having the same mass m and charges +q and -q separated by a distance d enter a uniform magnetic field B directed perpendicular to paper inwards with speeds v1andv2 as shown in Fig. 1.139. The particles will not collide if
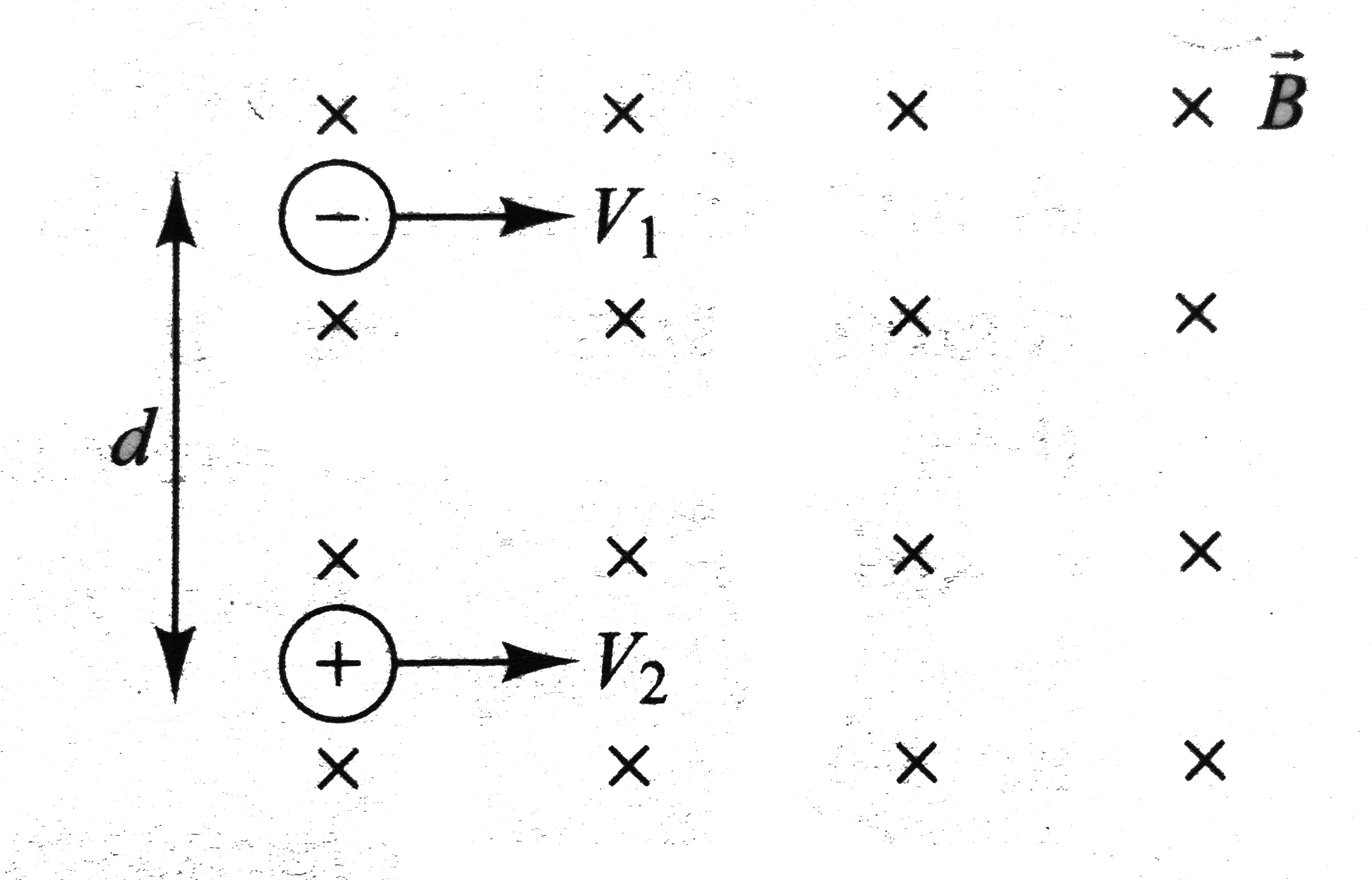 Ad>mBq(v1+v2)Bd<mBq(v1+v2)Cd>2mBq(v1+v2)Dv1=v2
Ad>mBq(v1+v2)Bd<mBq(v1+v2)Cd>2mBq(v1+v2)Dv1=v2
Two objects having same mass 'm' are moving with velocities v and 2v. Find ratio of their kinetic energies.
View SolutionWhat is the direction of the force acting on a charged. Particle q moving with a velocity →v in a uniform magnetic field →B ?
View SolutionWhat is the direction of the force acting on a charged particle q, moving with a velocity →v a uniform magnetic field →B ?
View SolutionA particle of mass m and positive charge q,moving with a uniform velocity v enters a magnetic field B as shown in figure.(a) Find the radius of the circular arc it describes in the magnetic field. (b)Find the angle subtended by the arc at the centre. (c) How long does the particle stay inside the magnetic field? (d)Solve the three parts of the above problem if the charge q on the particle is negative.
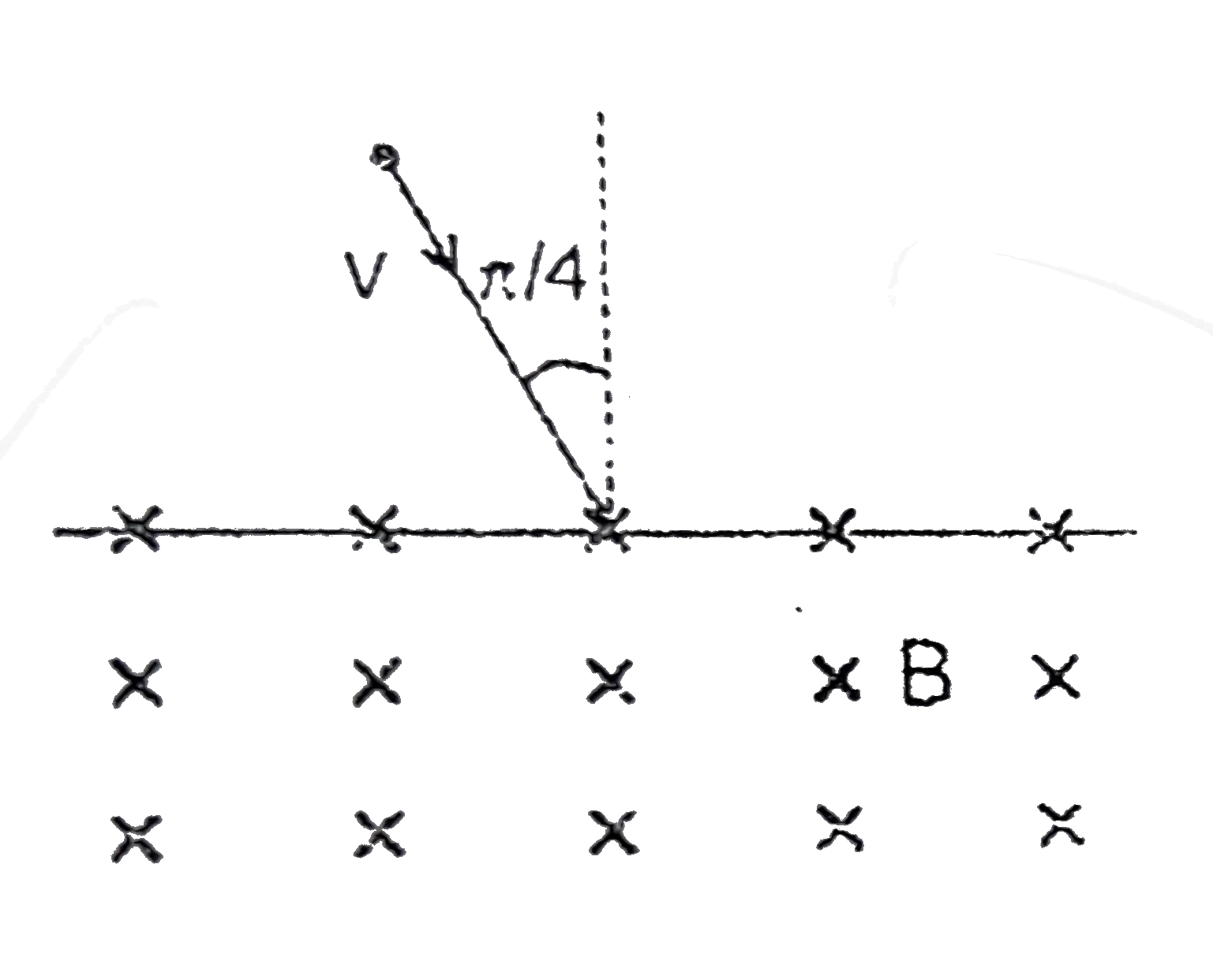
View SolutionA particle of mass m and charge Q moving with a velocity v enters a region on uniform field of induction B Then its path in the region is s
View Solution
