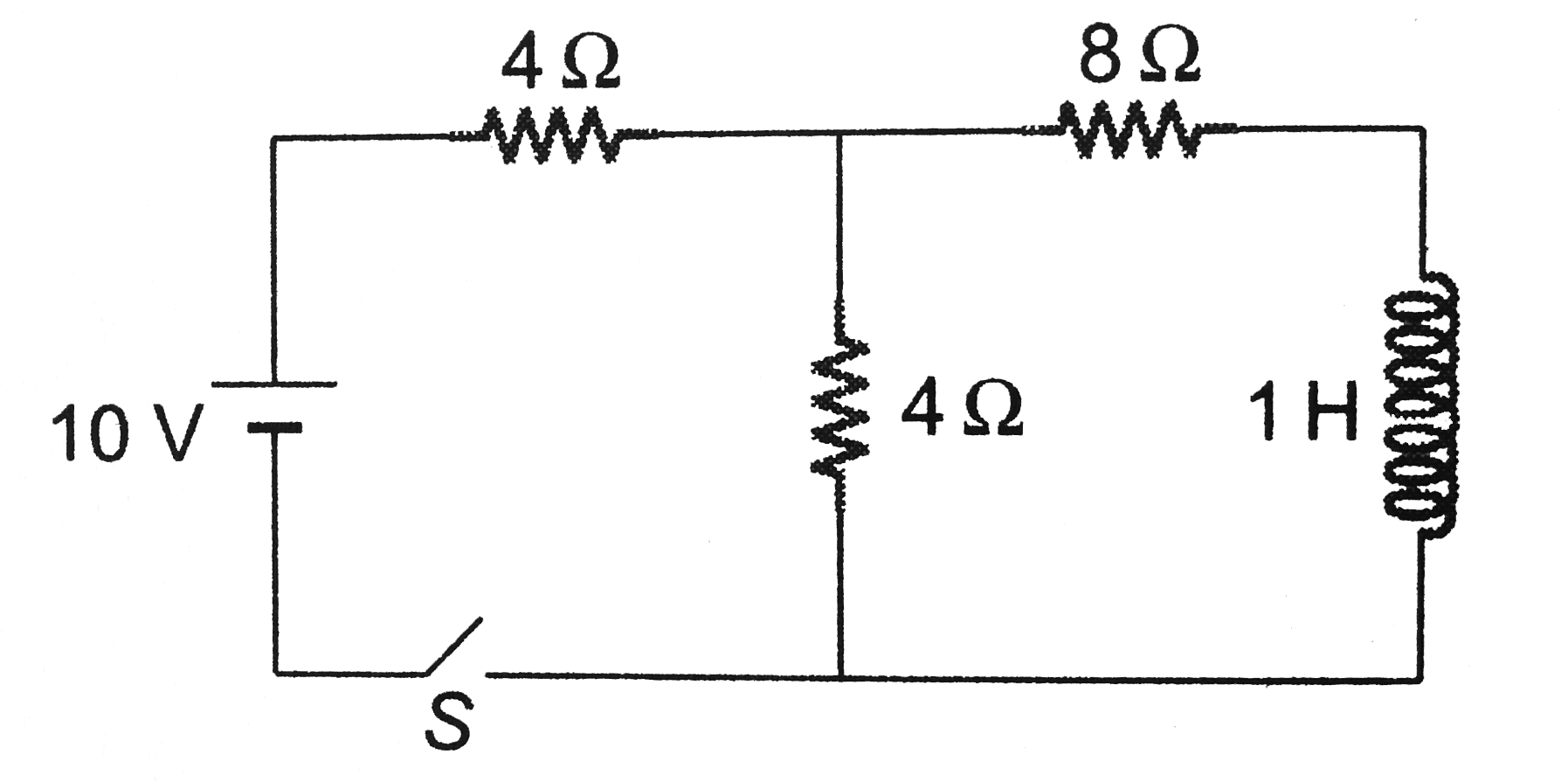
In the following circuit (Fig.)the switch is closed at $t = 0$ . Intially, there is no current in inductor. Find out the equation of current in the inductor coil as s function of time.

Text Solution

Verified by Experts

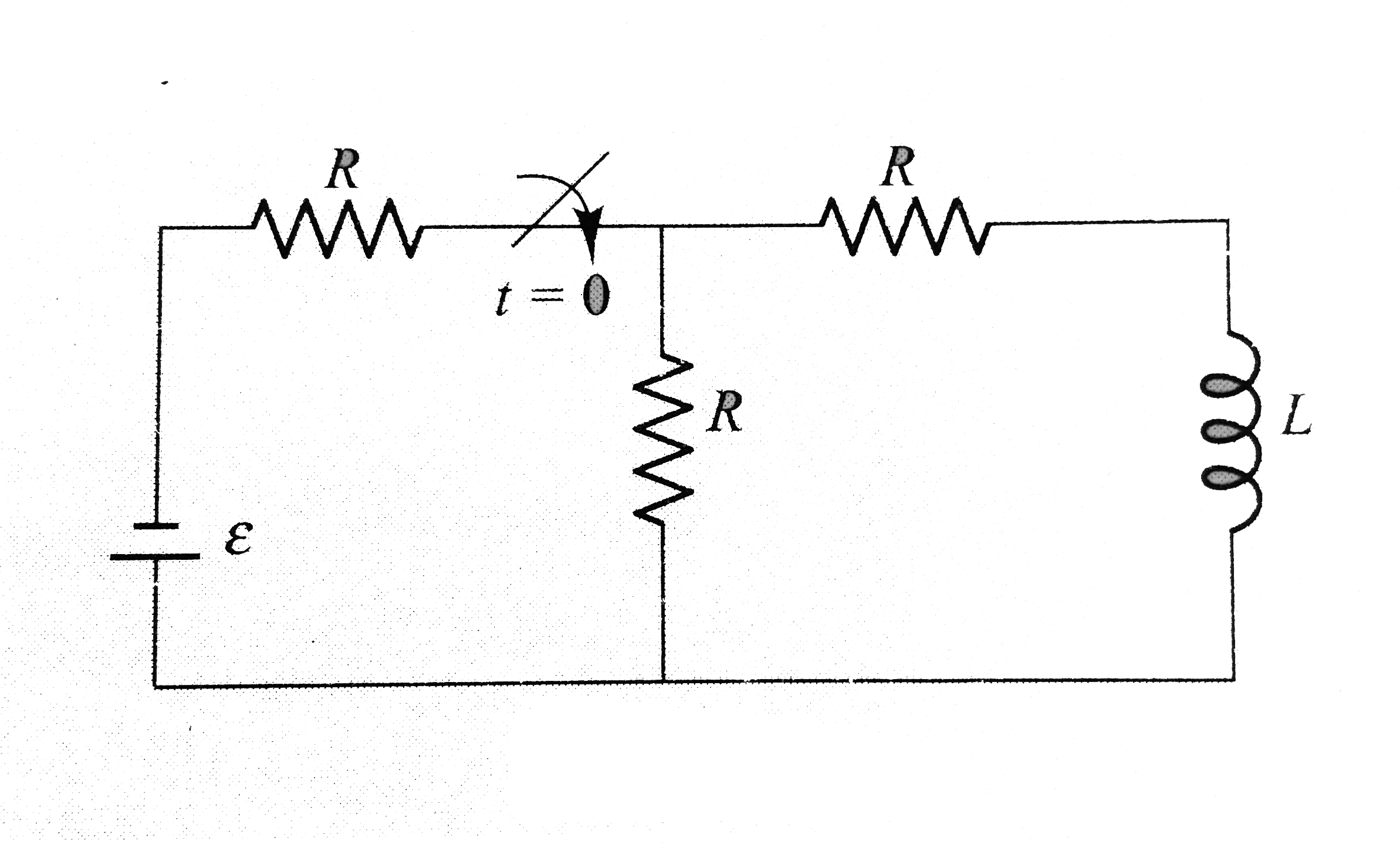
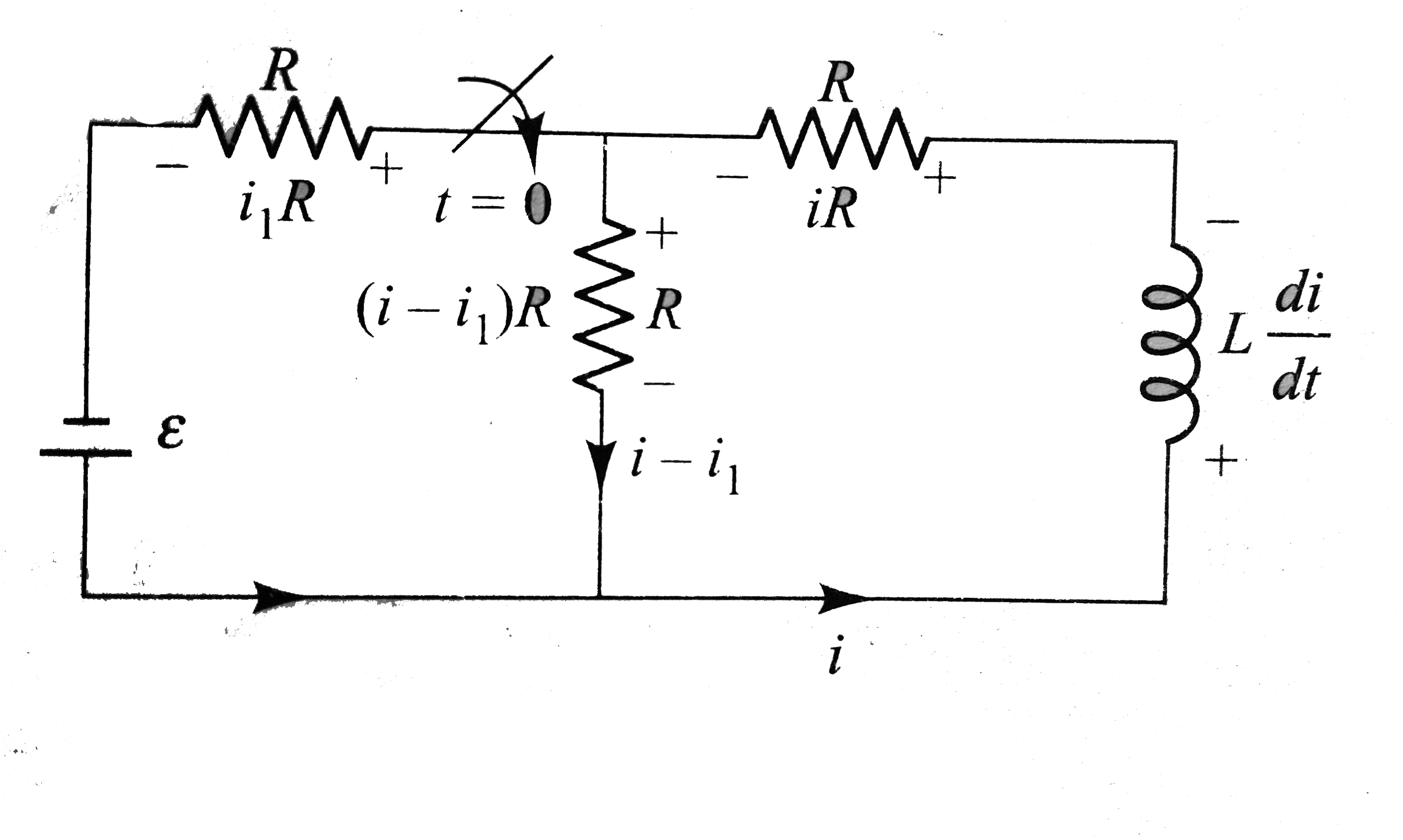
At any time $t, - ε + i_{1} R - (i - i_{1}) R = 0$
$- ε + 2 i_{1} R - i R = 0$
$i_{1} = \frac{i R + ε}{2 R}$

Now
$- ε + i_{1} R + i R + L \frac{d i}{d t} = 0$
$- ε + (\frac{i R + ε}{2}) + i R + L \frac{d i}{d t} = 0$
$\frac{ε}{2} + \frac{3 i R}{2} = - L \frac{d i}{d t}$
$(\frac{ε + 3 i R}{2}) d t = - L d i \Rightarrow - \int_{0}^{t} \frac{d t}{2 L} = \int_{0}^{i} \frac{d i}{- ε + 3 i R}$
$\frac{t}{2 L} = \frac{1}{3 R}$ In $(\frac{- ɛ + 3 i R}{- ɛ}) \Rightarrow - I n (\frac{- ɛ + 3 i R}{- ɛ}) = \frac{3 R t}{2 L}$
$i = + \frac{ɛ}{3 R} (1 - e^{- \frac{3 R t}{2 L}})$

|

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

In adjacent circuit, switch S is closed at t = 0. The time at which current in the circuit becomes half of the steady current is

A $τ ln 2$
B $\frac{ln 2}{τ}$
C $2 τ ln 2$
D $\frac{τ}{2} ln 2$

CENGAGE PHYSICS-INDUCTANCE-Concept Based

Home
Ask Doubt
Profile