Derive an expression for the total magnetic energy stored in two coils with inductances L1 and L2 and mutual inductance M, when the currents in the coils are I1 and I2, respectively.
When the currents are increaseing in the circuit, we have emfs
E1=−L1dI1dt±MdI2dt,E2=−L2dI2dt±MdI1dt
where ± sign appears conistently in both equations and depends on the geometry of the coils and the sense of current. Work done in pushing charges dq1 and dq2 through each circuit. respectively, is
dW=−E1dq−E2dq2
=L1dI1dtdq1¯¯¯¯¯+MdI2dtdq1+L2dI2dtdq2¯¯¯¯¯+MdI1dtdq2
I1=dq1dt,dq1=I1dt,I2=dq2dt,dq2=I2dt
dW=L1I1dI1+L2I2dI2¯¯¯¯¯+(MI1dI2+MI2dI1)
=L1I1dI1+L2I2dI2¯¯¯¯¯+Md(I1I2)
On integating the above expression from 0 to final current, we have
U=∫dW=L1∫I10I1dI1+L2∫I20I2dI2¯¯¯¯¯+M∫(I1I2)0d(I1I2)
=12L1L21+12L2I22¯¯¯¯¯+MI1I2
Topper's Solved these Questions
- INDUCTANCEBOOK - CENGAGE PHYSICSCHAPTER - INDUCTANCEEXERCISE - Solved Examples
3 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICSCHAPTER - INDUCTANCEEXERCISE - Exercise 4.1
24 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICSCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
28 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
Similar Questions
Define the term 'mutual inductance between the two coils. Obtain the expression for mutual inductance of a pair of long coaxial solenoids each of length l and radii r1andr2(r2>r1). Total number of turns in two solenoids are N1andN2 respectively.
View SolutionObtain the expression for the magnetic energy stored in an ideal inductor of self inductance L when a current I passes through it.
Hence obtain the expression for the energy density of magentic field produced in the inductor.
View Solution
Knowledge Check
Mutual inductance of two coils depends on their self inductance L1 and L2 as :
AM12=L1/L2BM12=L2/L1CM12=√L1L2DM12=√L1/L2The mutual inductance M12 of coil 1 with respect to coil 2
Aincreases when they are brought nearerBdepends on the current passing through the coilsCincreases when one of them is rotated about an axisDis the same as M21 coil 2 with respect to coil 1Coefficient of coupling between two coils of self-inductances L1 and L2 is unity. It means
A50% flux of L1 is linked with L2B100% flux of L1 is linked with L2C√L1 time of flux of L1 is linked with L2DNone of the above
Coefficient of coupling between two coils of self-inductances L1 and L2 is unity. It means
View SolutionA system consists of two coaxial current carrying circular loops as shown in Fig. The self inductance of loop 1 is L1 and self inductance of loop 2 is L2. Magnitude of mutual inductance is M. If at any instant current flowing through loop 1 and loop 2 are i1andi2 respectively, then total magnetic energy of the system is
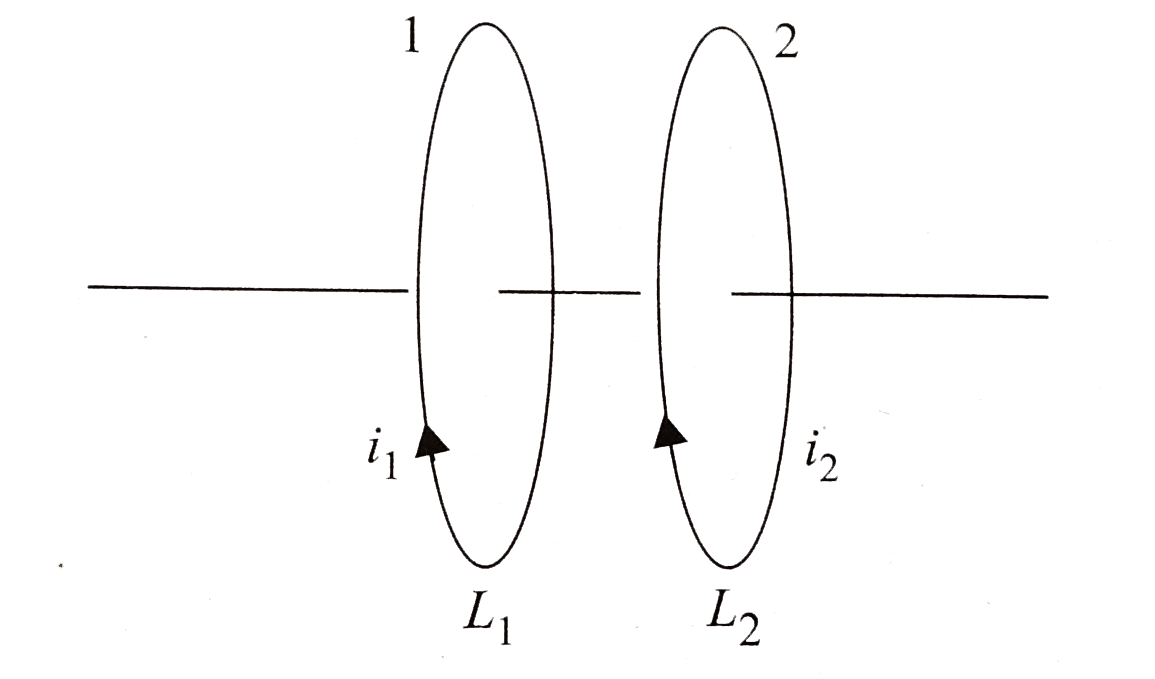
View SolutionTwo different coils have self-inductance L1=8mH,L2=2mH. The current in one coil is increased at a constant rate. The current in the second coil is also increased at the same rate. At a certain instant of time, the power given to the two coils is the same. At that time the current, the induced voltage and the energy stored in the first coil are i1,V1 and W1 respectively. Corresponding values for the second coil at the same instant are i2,V2 and W2 respectively. Then
View SolutionTwo different coils have self-inductances L1=8mHandL2=2mH. The current in one coil is increased at a constant rate. The current in the second coil is also increased at the same constant rate. At a certain instant of time, the power given to the two coil is the same. At that time, the current, the induced voltage and the energy stored in the first coil are i1,V1andW1 respectively. Corresponding values for the second coil at the same instant are i2,V2andW2 respectively. Then:
View SolutionTwo different coils have self inductances L1=9mH and L2=2mH. The current in one coil is increased at a constant rate. The current in the second coil is also increased at the same constant rate. At a certain instant of time, the power given to the two coils is the same. At that time, the current the induced voltage and the energy stored in the first coil are i1,V1 and W1 respectively.
Corresponding values for the second coil at the same instant are i2,V−2 and W2 respectively. Then,
View Solution