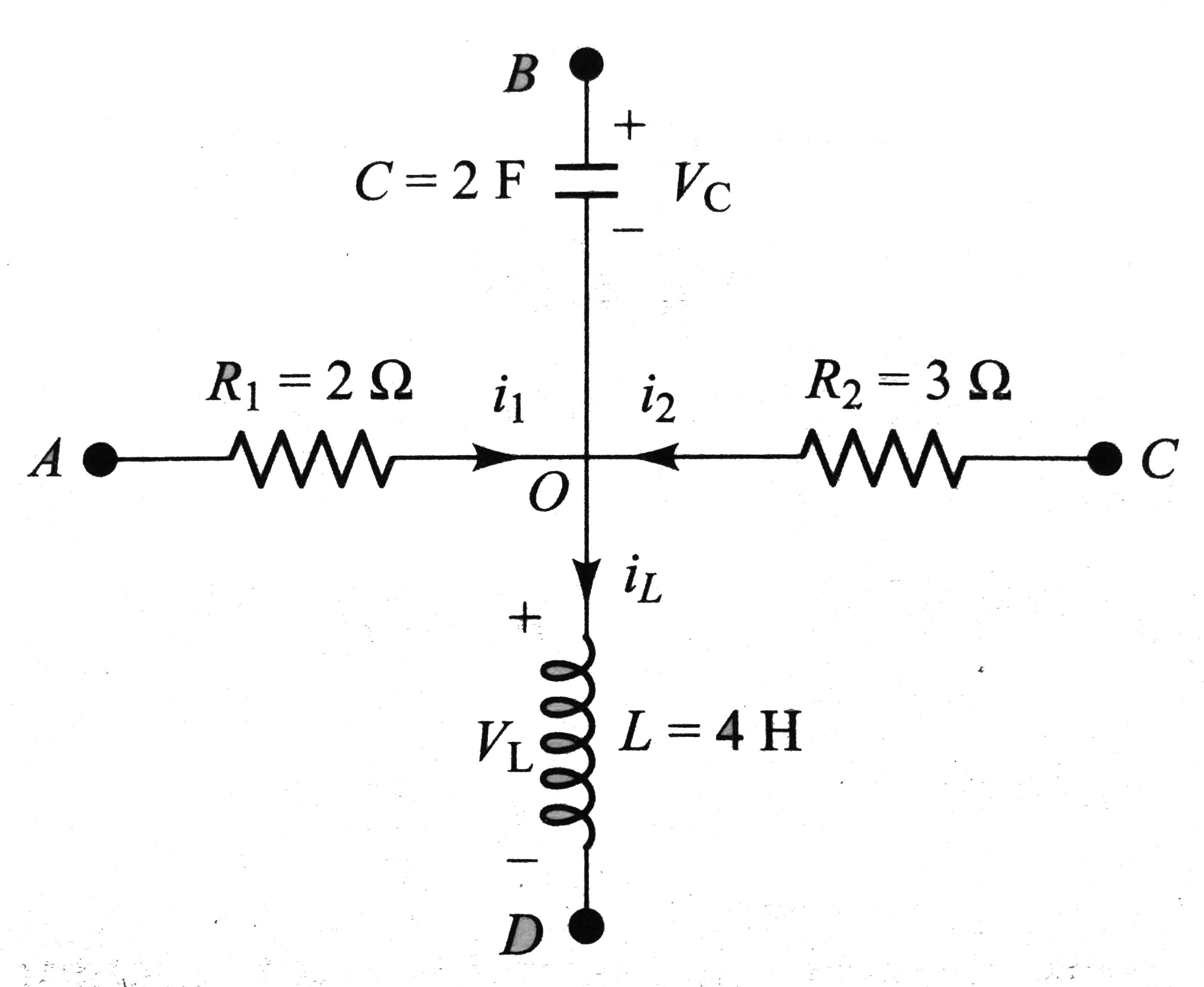
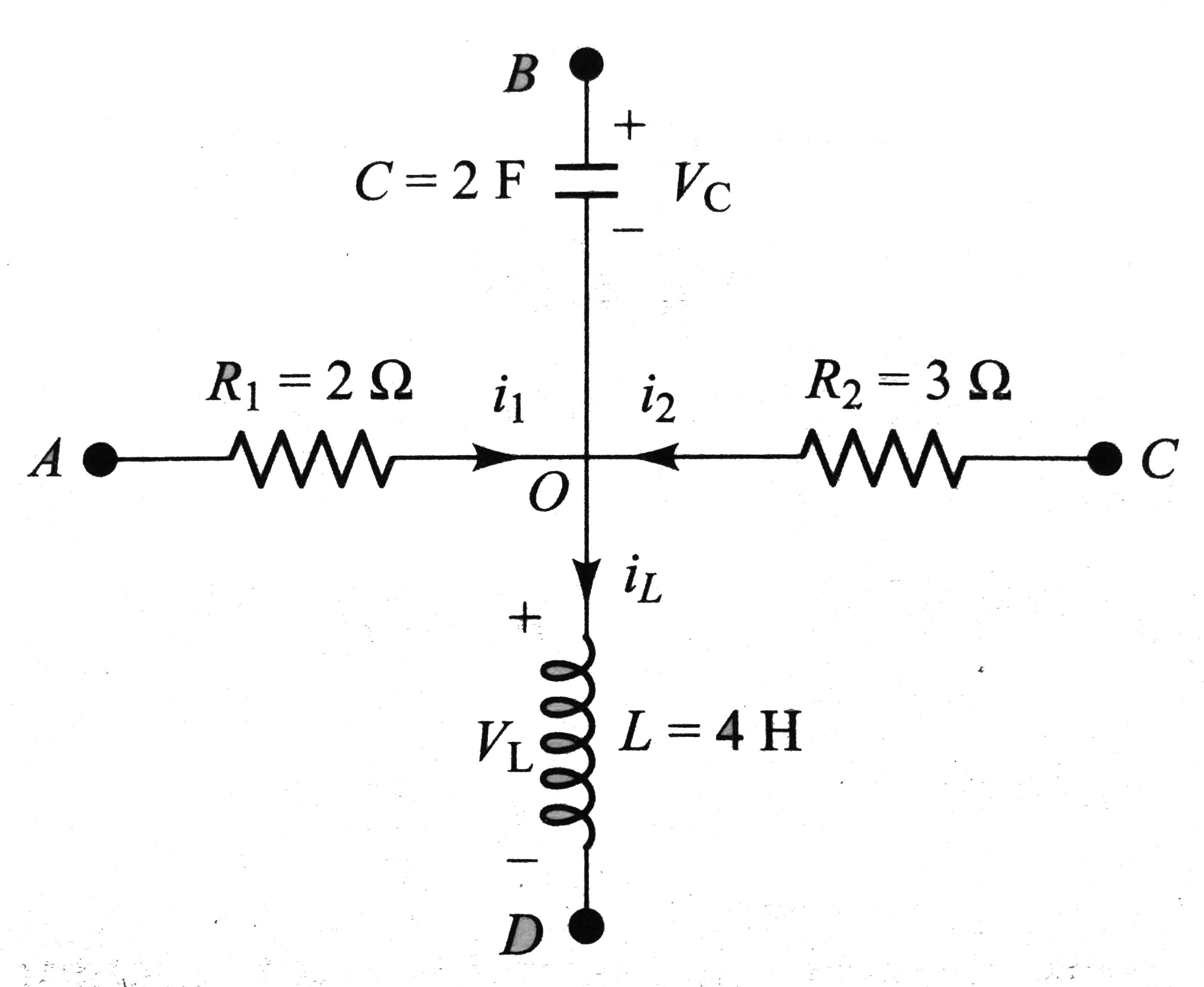
In Fig , , and .
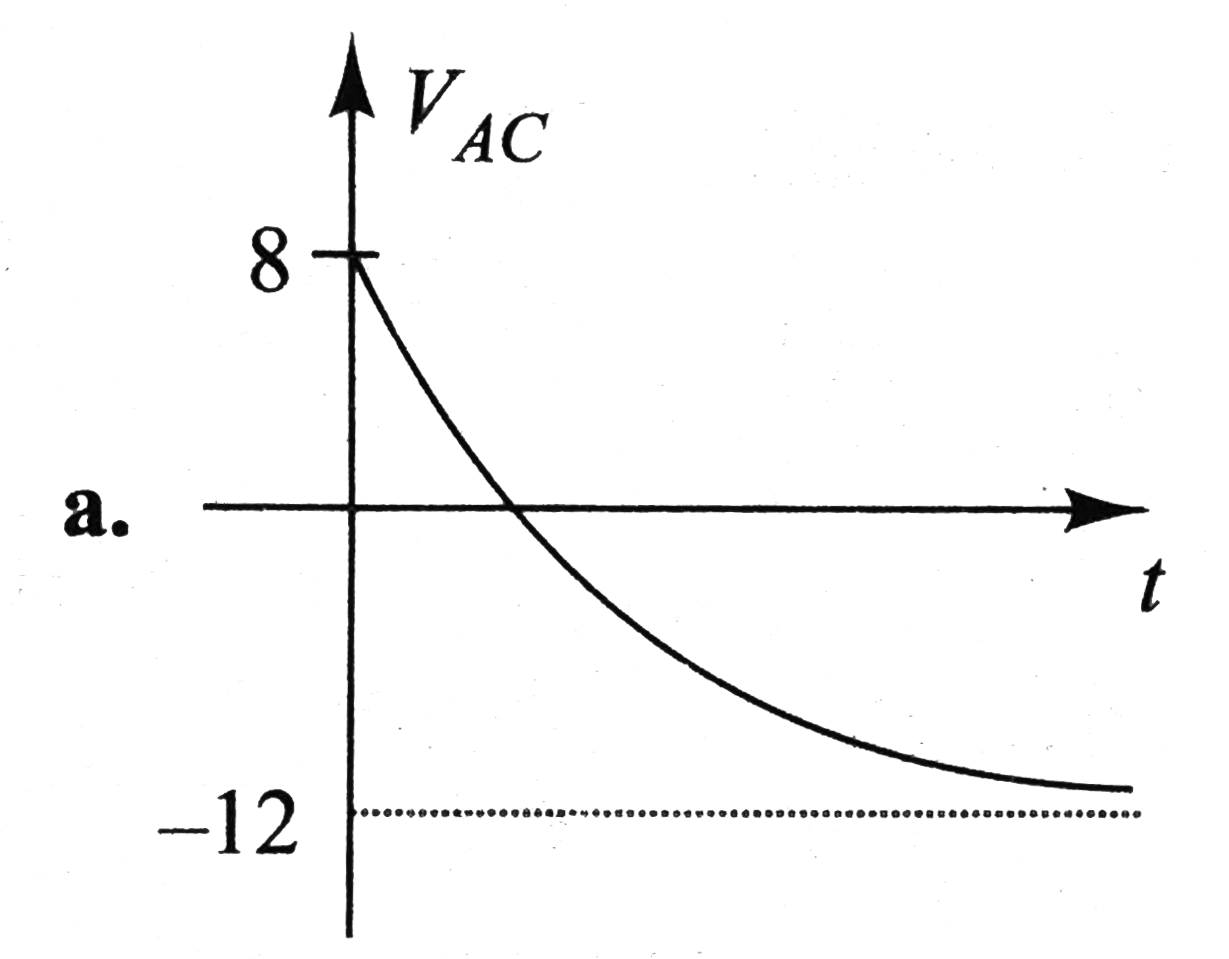
the vartiation of potential difference across and with time can be respresented as
A
B

C

D

Text Solution
The correct Answer is:A
Instanctaneous current in the capacitor,
Current,
This current flows from to .
From , we have
. time graph is as shows in Fig lt
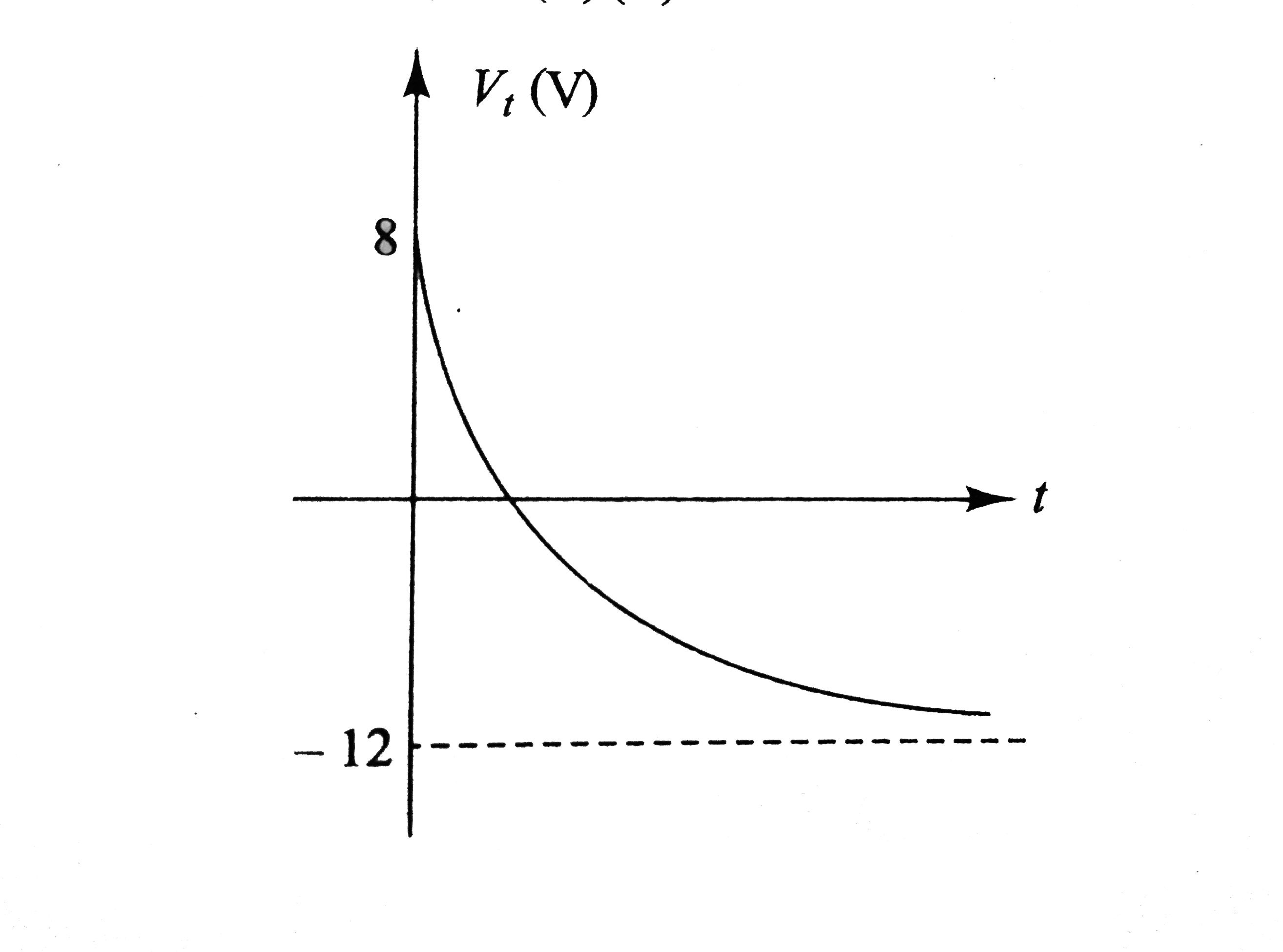
increases from to expontially.

decreases exponentially from to as shows in Fig
To determine , we begin from and at . From ,
we have

Substituting the values, we have
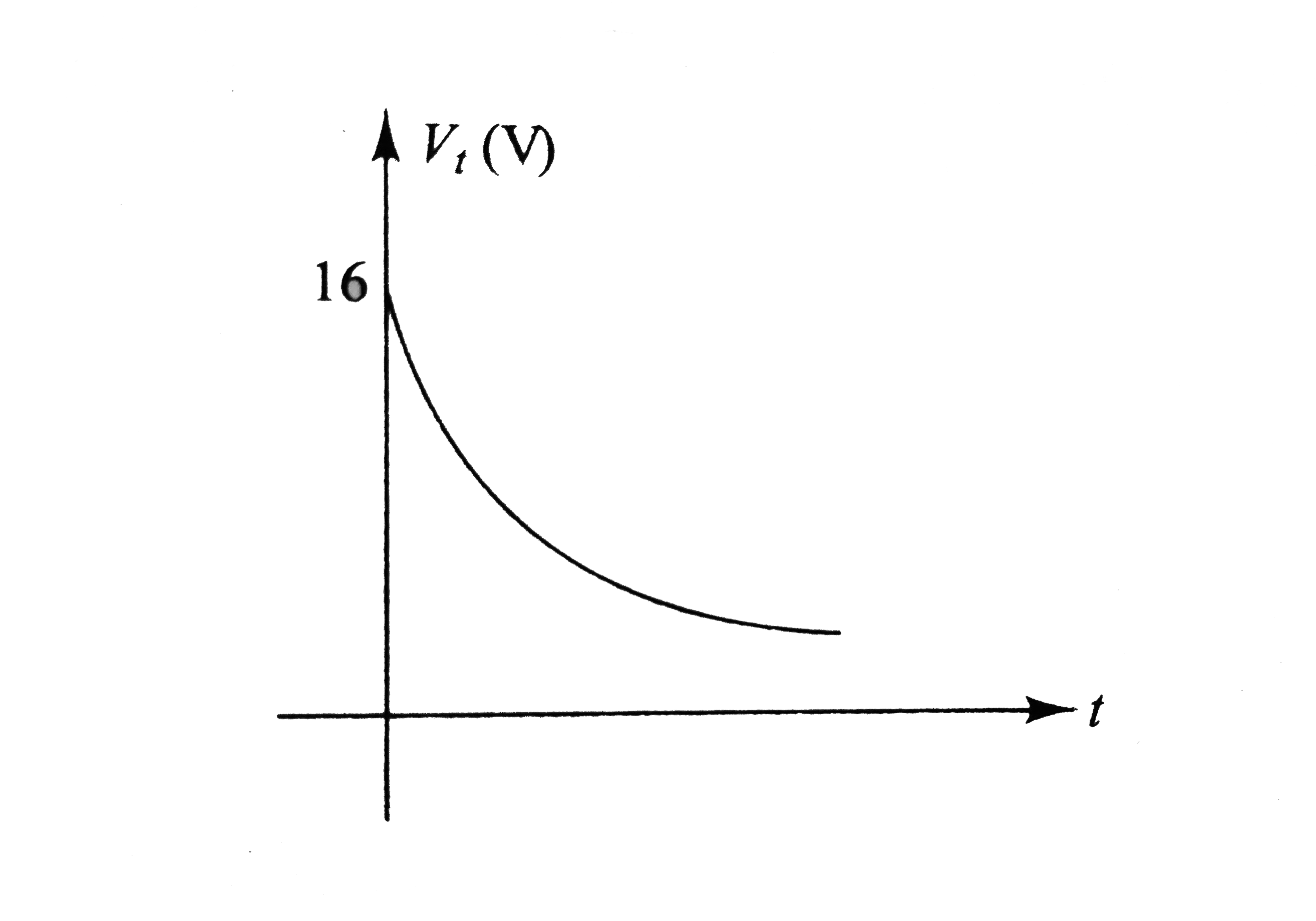
At ,
At ,
Therefore, decrease exponentially from to .
Similarly, we have from to
Substituting the values, we have
Thus, decreases exponentially from to .
As we move from to ,
Substituting the values we have,
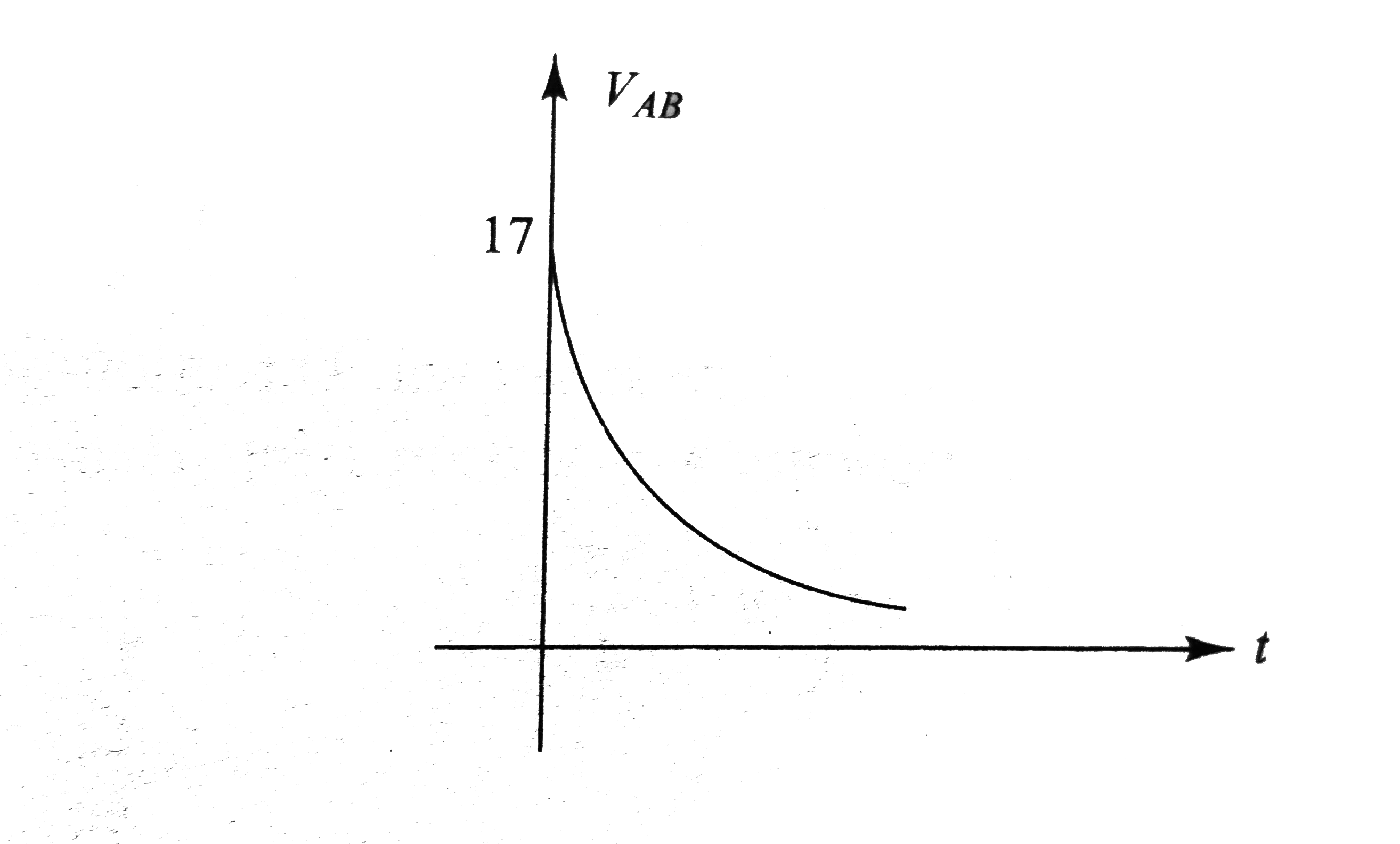
At
and at
i.e., decreases exponentially from to .
|
Topper's Solved these Questions
- INDUCTANCEBOOK - CENGAGE PHYSICSCHAPTER - INDUCTANCEEXERCISE - Exercises (integer)
8 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICSCHAPTER - INDUCTANCEEXERCISE - Archives (fills In The Blanks)
3 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICSCHAPTER - INDUCTANCEEXERCISE - Exercises (assertion-reasoning)
2 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICSCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
28 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
Similar Questions
Knowledge Check
In Fig i1=10e−2tA, i2=4A, and VC=3e−2tV.
The variation of potential difference across C and D(VCD) with time can be expressed asA B
B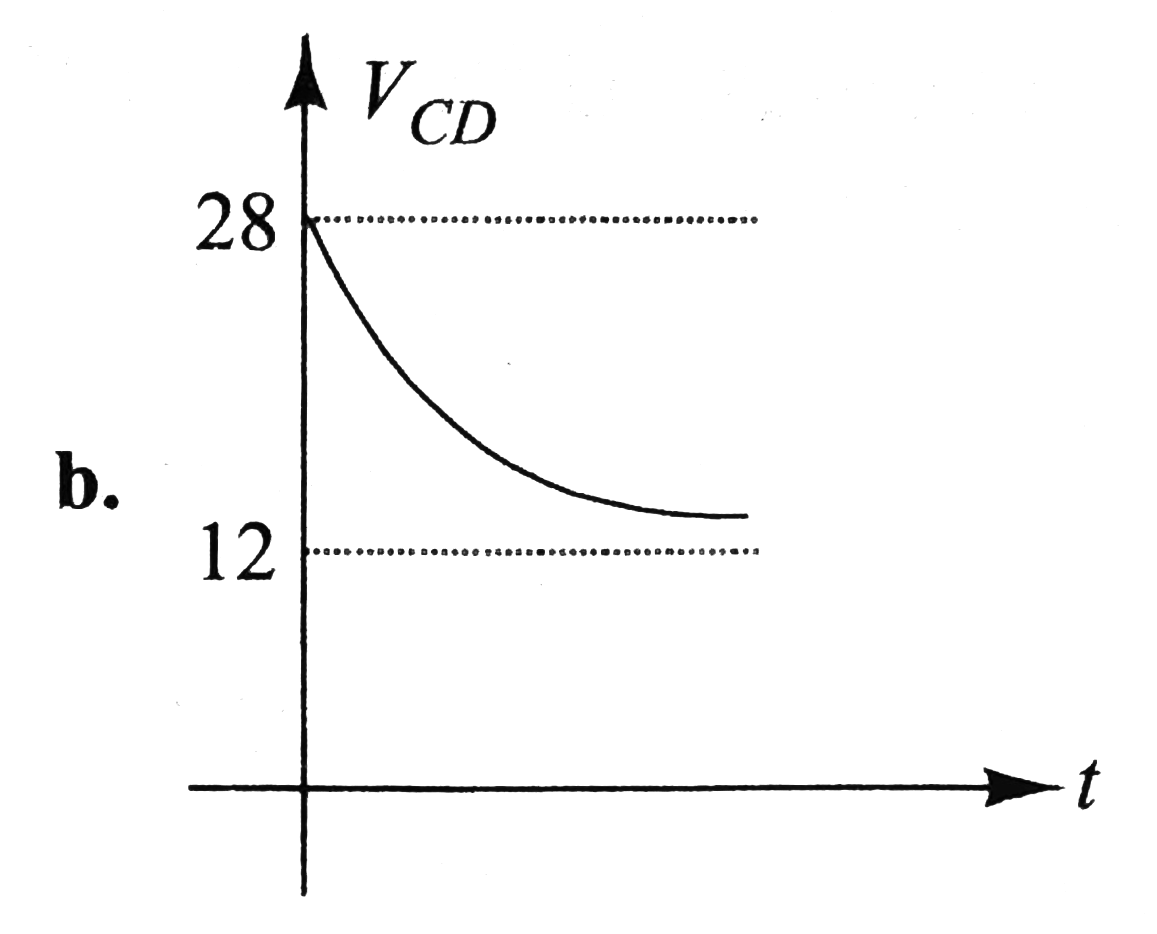 C
C D
D
In figure i1=10e−2tA,i2=4A,vC=3e−2tV
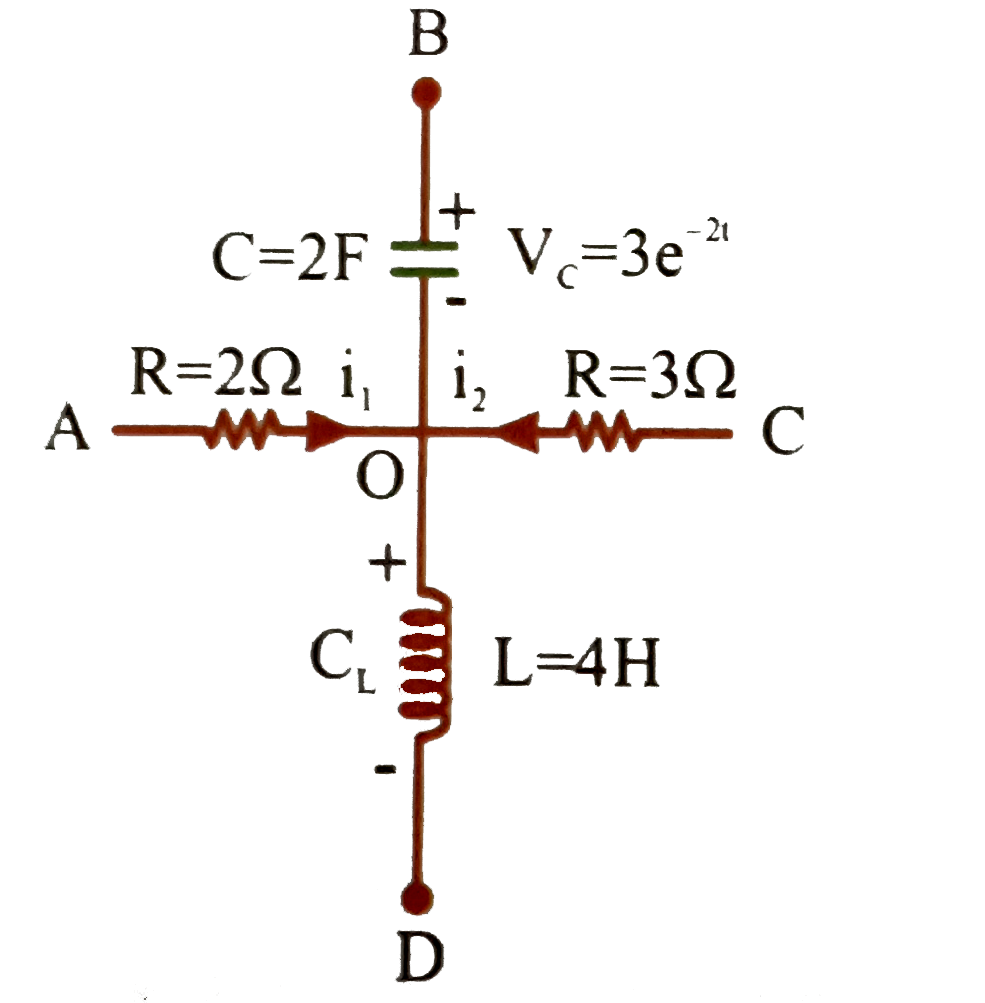
The variation of potential difference acorss A and C with time can be represented asA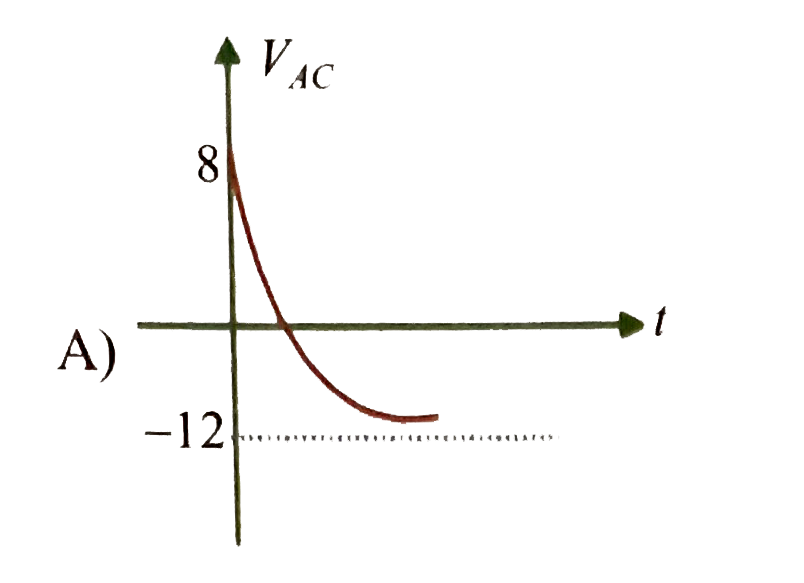 B
B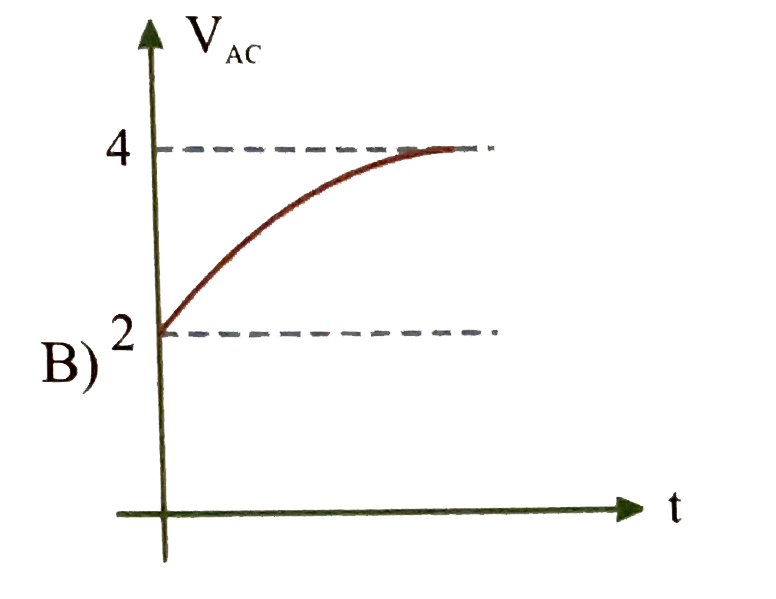 C
C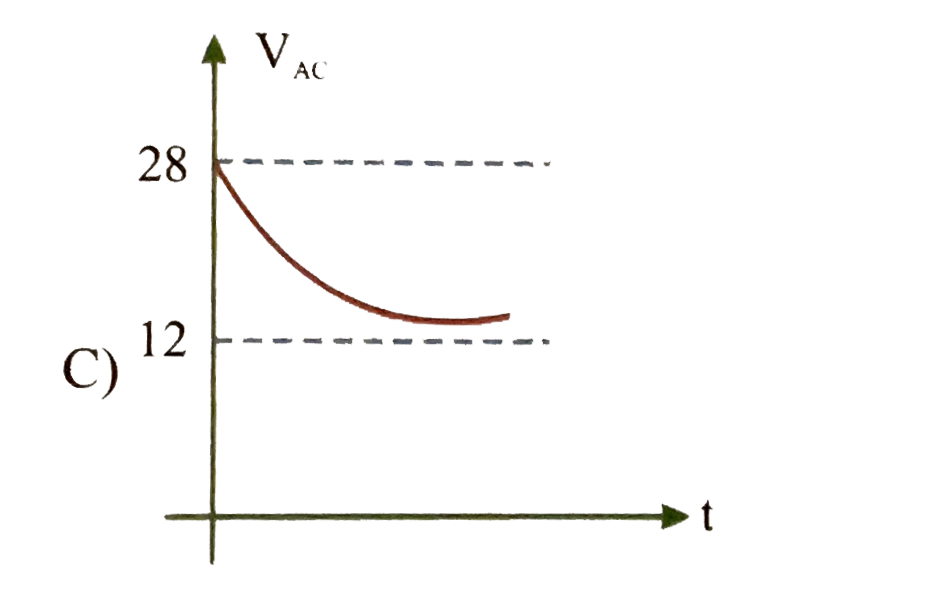 D
D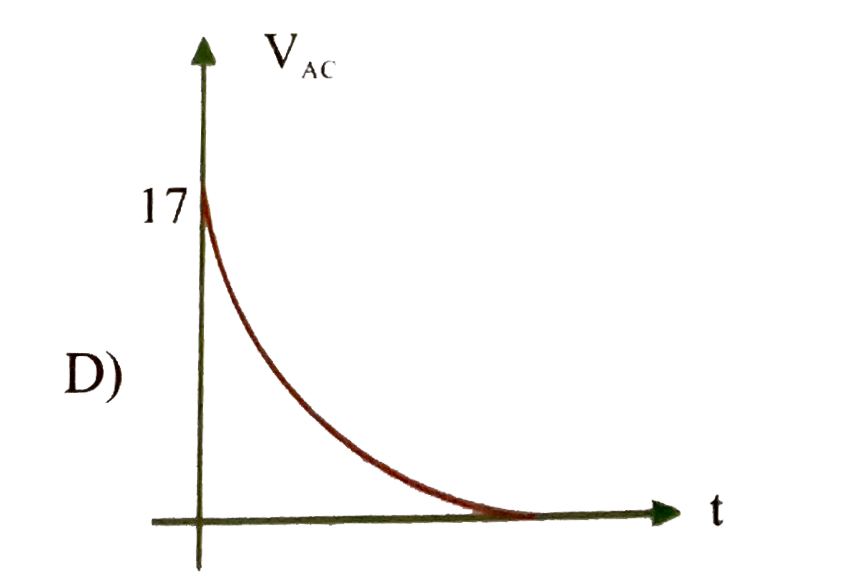
In figure i1=10e−2tA,i2=4A,vC=3e−2tV
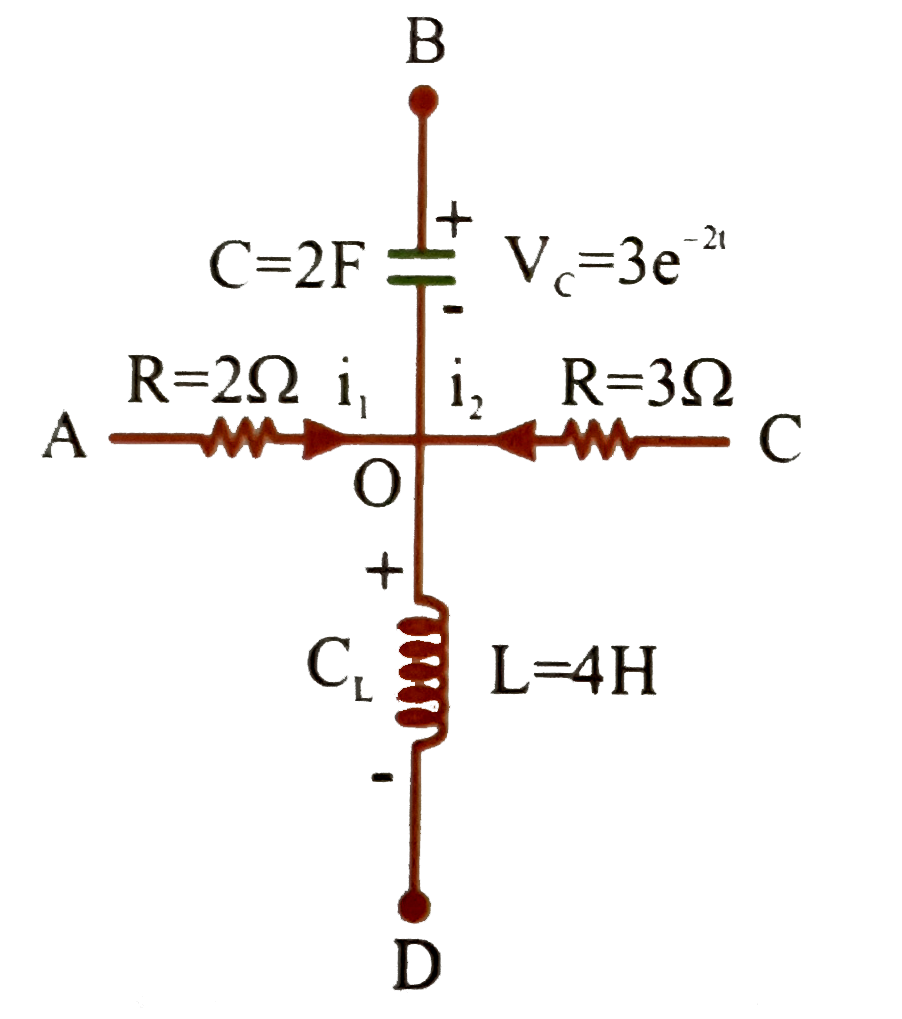
The potential difference across inductor VL is :A8e−2tVB9e−2tVC16e−2tVD18e−2tV
In the figure shown, i1=10e2tA , i2=4A and Vc=3e−2tV Determine
(a) iL and VL
(b) Vac , Vab and Vcd
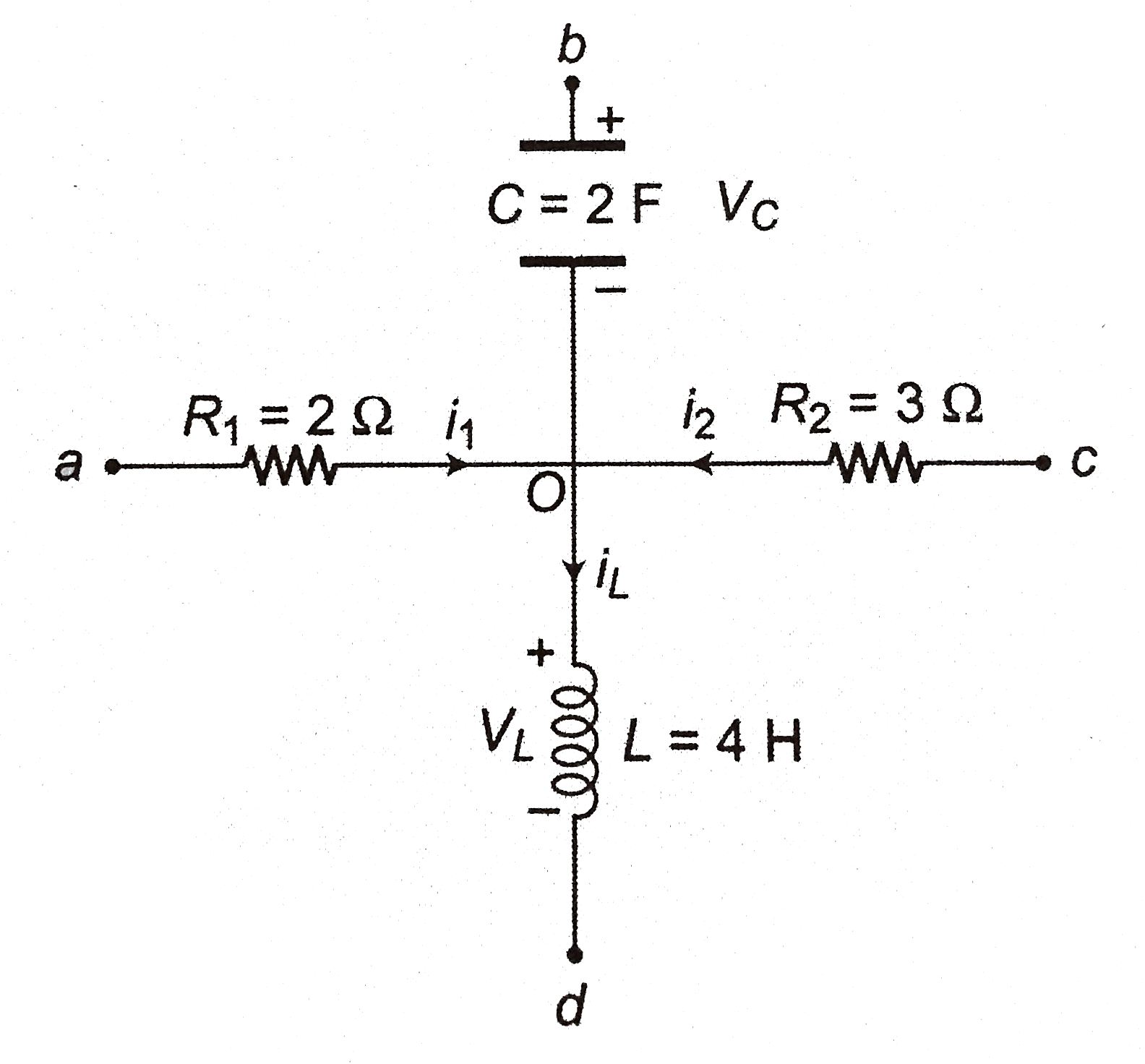
View SolutionIn figure i1=10e−2tA,i2=4A,vC=3e−2tV
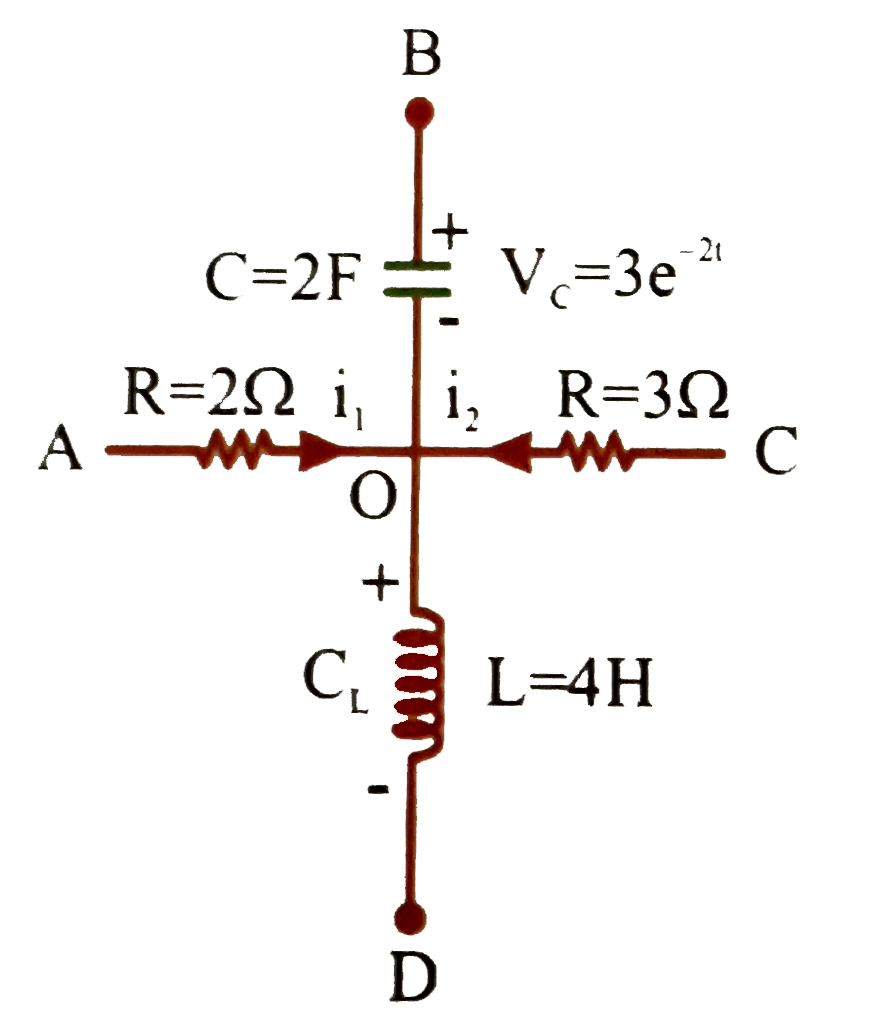
The current iL is
View SolutionIn figure i1=10e−2tA,i2=4A,vC=3e−2tV
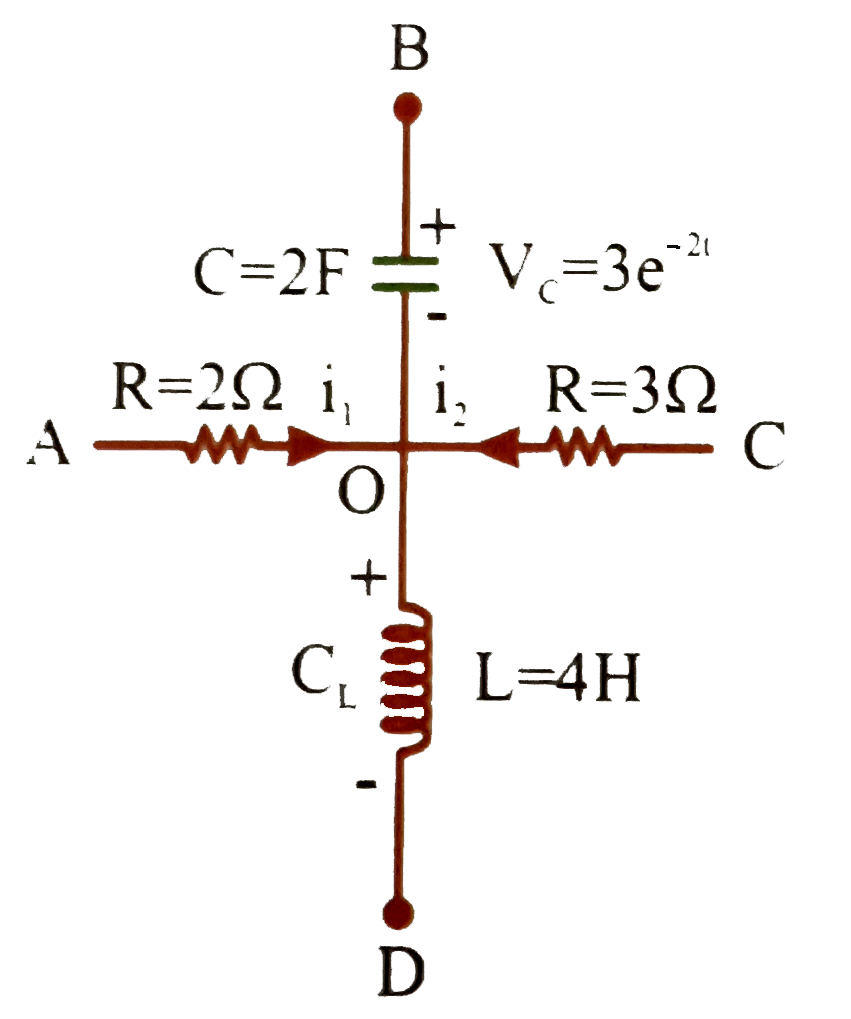
The potential difference across AB(VAB) is:
View SolutionIn Fig i1=10e−2tA, i2=4A, and VC=3e−2tV.
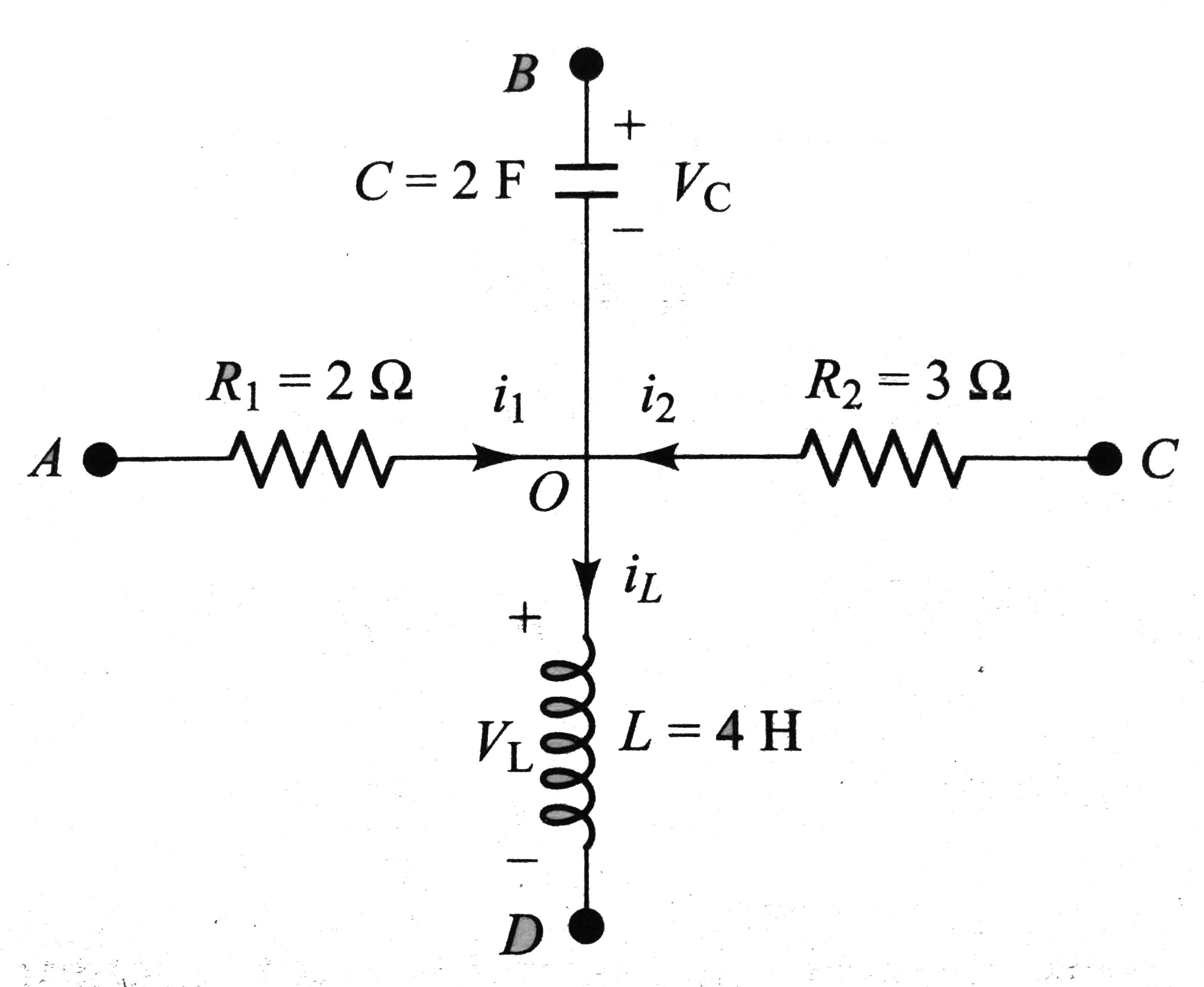
The variation of current in the inductor with time can be represented as
View SolutionIn figure i1=10e−2tA,i2=4A,vC=3e−2tV
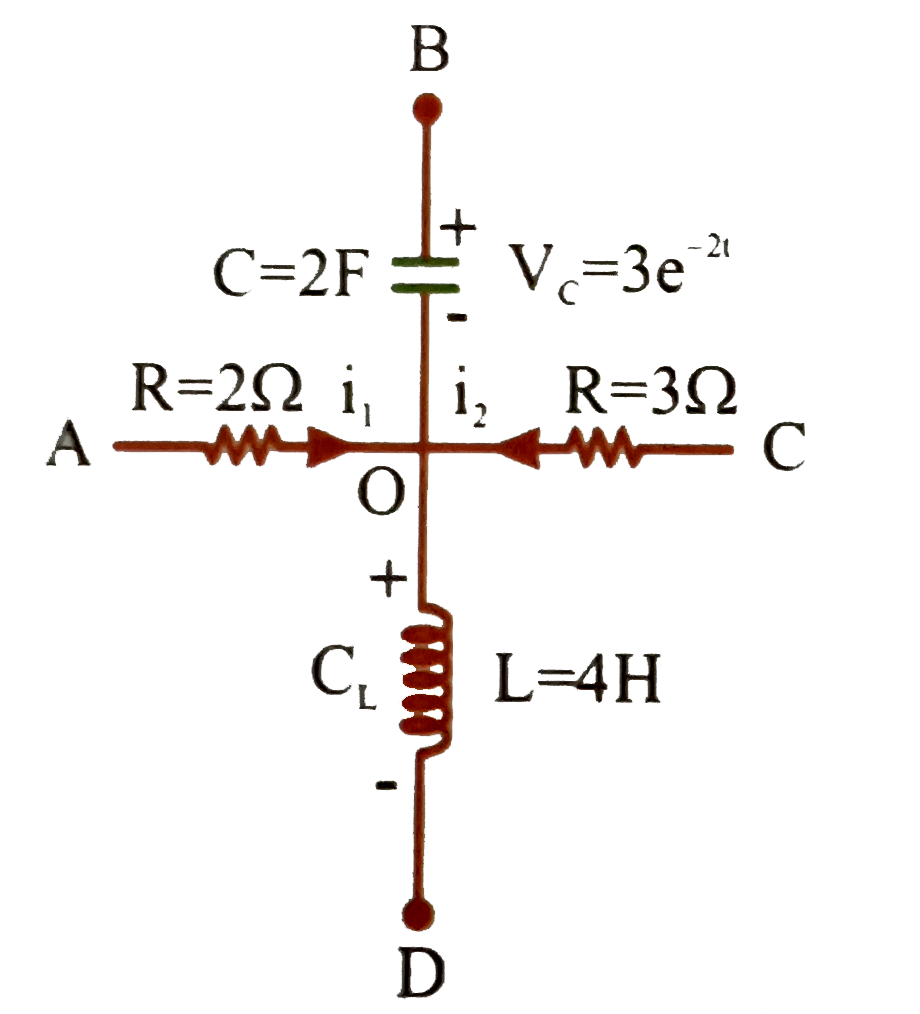
The variaton of current in the inductor with time can be repesented as :
View Solution