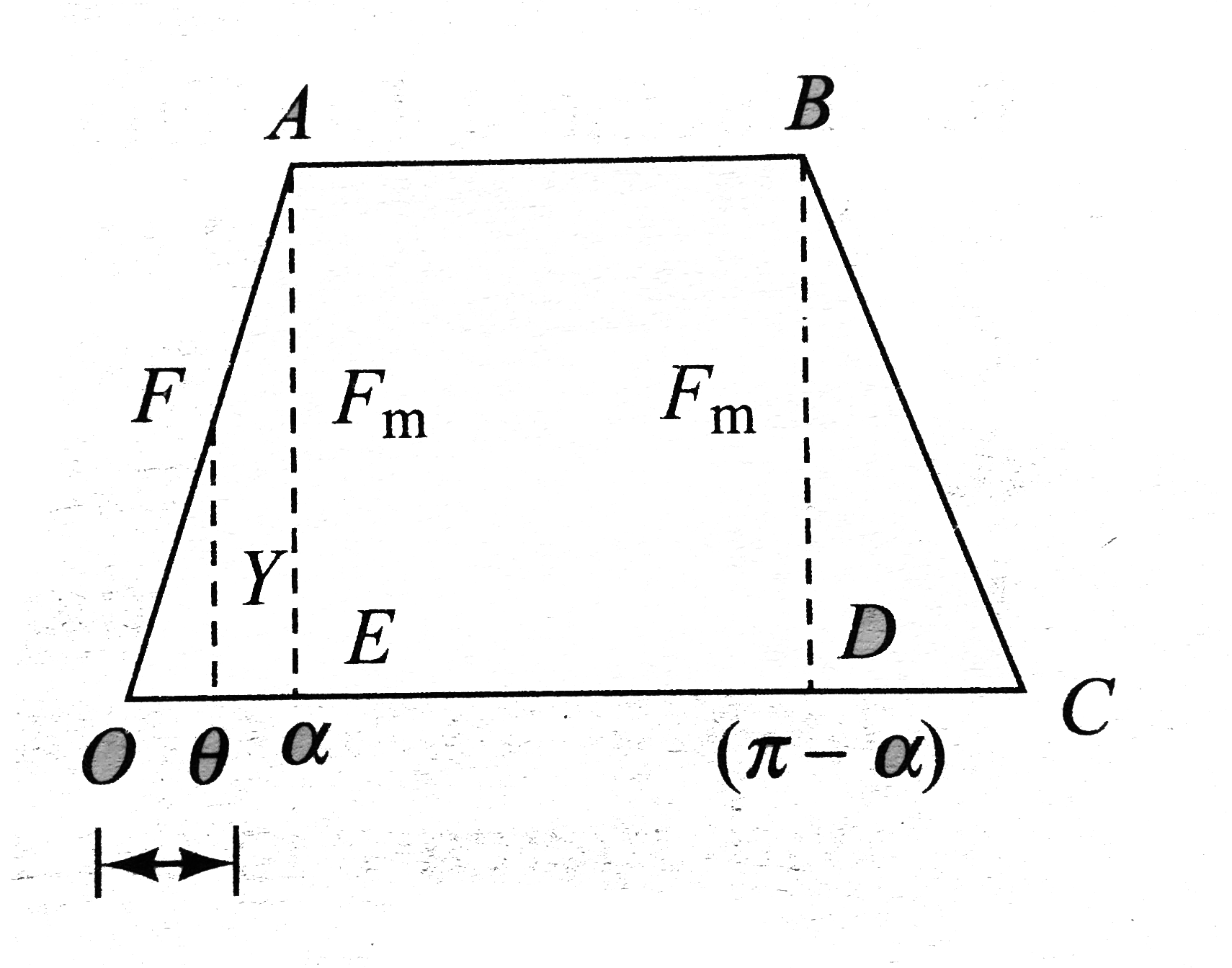
The half cycle of an alternating singnal is shown in fig. It increases uniformly form zero at $0^{\circ} \to F_{m} a t {(α)}^{\circ}$ and decrease uniformly from $(F_{m}) a t 180^{\circ}$

The average value of the singnal is

A

$\frac{(π + α) F_{m}}{π}$

B

$\frac{(π - α) F_{m}}{π}$

C

$(\frac{π + α}{3 π}) F_{m}$

D

$(\frac{2 π + α}{2 π}) F_{m}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

For finding the average value m, we would find the total area of the trapezium and divide it by $(π)$ .
Area $= 2 \times Δ O A E + r e c tan g \leq A B D E$
$= 2 \times (1 / 2) \times F_{m 0 α + (π - 2 α) F_{m 0 = (π - α) F_{m}}}$
Average value $= (π - α) F_{m} / (π)$
rms value: From similar triangles , we get
$\frac{y}{t ˆ a} = \frac{F_{m}}{α}$ or $(y^{2}) = \frac{F_{m}^{2}}{α^{2}} t {ˆ a}^{2}$
This gives the equation of the single over the two triangles OAE and DBC. The single reamins constant over the angle $α$ to $(π - α)$ , i.e., over an angluar distance of $(π - α) - α = (π - 2 α)$ .
Sum of the square
$\frac{2 f_{m}^{2}}{α^{2}} F_{m}^{2} (π - \frac{4 α}{3})$
The mean value of the squares
$= \frac{1}{π} F_{m}^{2} (π - \frac{4 α}{3}) = F_{m}^{2} (1 - \frac{4 α}{3 π})$
rms value $= F_{m} \sqrt{(1 - \frac{4 α}{3 π})}$ .

|

Updated on:21/07/2023

Class 12 PHYSICS ALTERNATING CURRENT

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The half cycle of an alternating singnal is shown in fig. It increases uniformly form zero at $0^{\circ} \to F_{m} a t {(α)}^{\circ}$ and decrease uniformly from $(F_{m}) a t 180^{\circ}$

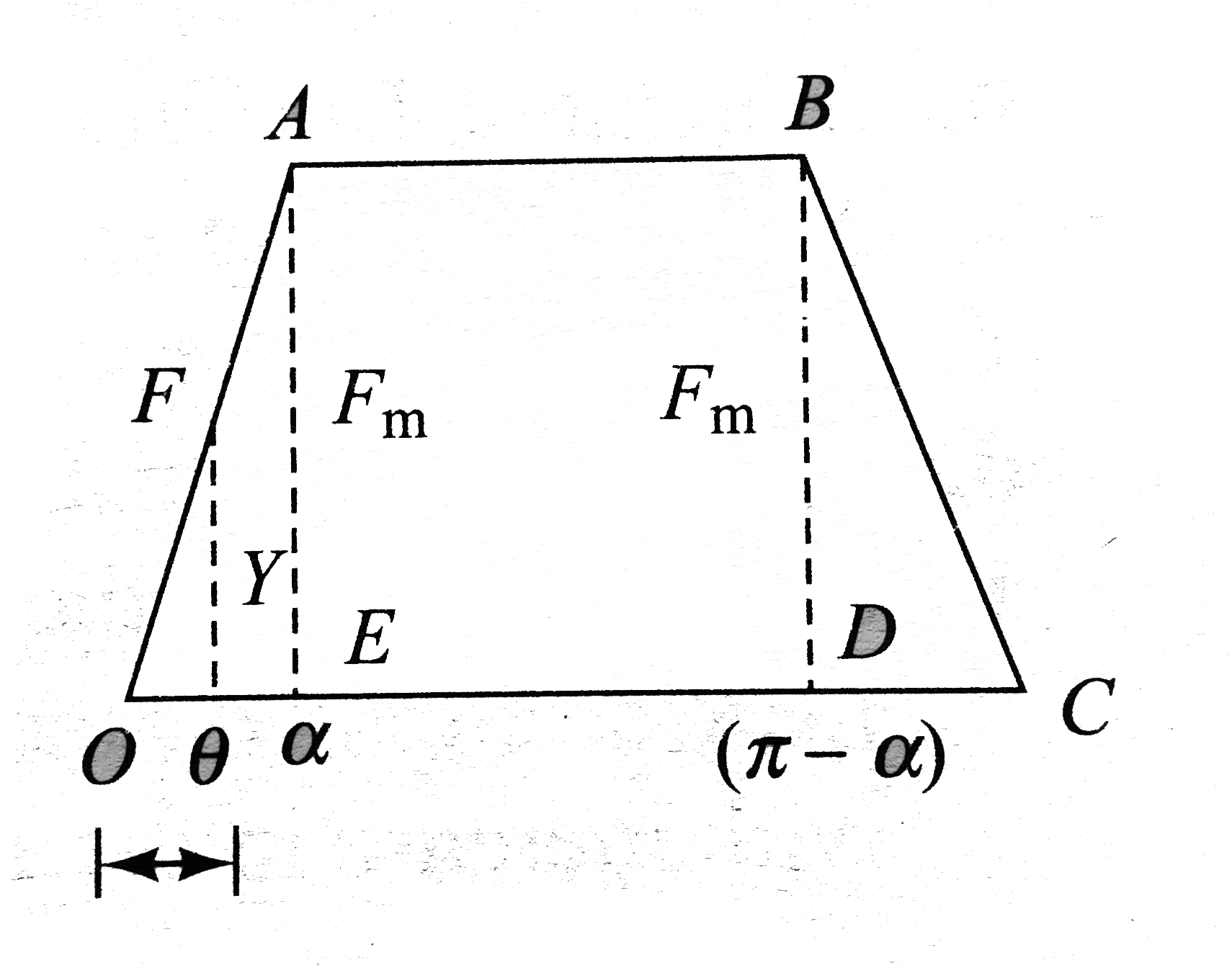
The effective value of the singnal is
A $F_{m} \sqrt{(1 - \frac{4 α}{3 π})}$
B $F_{m} \sqrt{(1 + \frac{4 α}{3 π})}$
C $F_{m} \sqrt{(1 - \frac{3 α}{4 π})}$
D $F_{m} \sqrt{(1 + \frac{3 α}{4 π})}$
Question 2 - Select One
The r.m.s value of alternating e.m.f. is
Atwice peak value
B $\sqrt{2}$ times greater than peak value
Cequal to peak value
Dless than peak value
Question 3 - Select One
The peak value of alternating e.m.f. in a generator is given by $e_{0} =$
ANAB
B $N A B ω$
CNAB v
Dnone of these

CENGAGE PHYSICS-ALTERNATING CURRENT-Exercises Linked Comprehension

Home
Ask Doubt
Profile