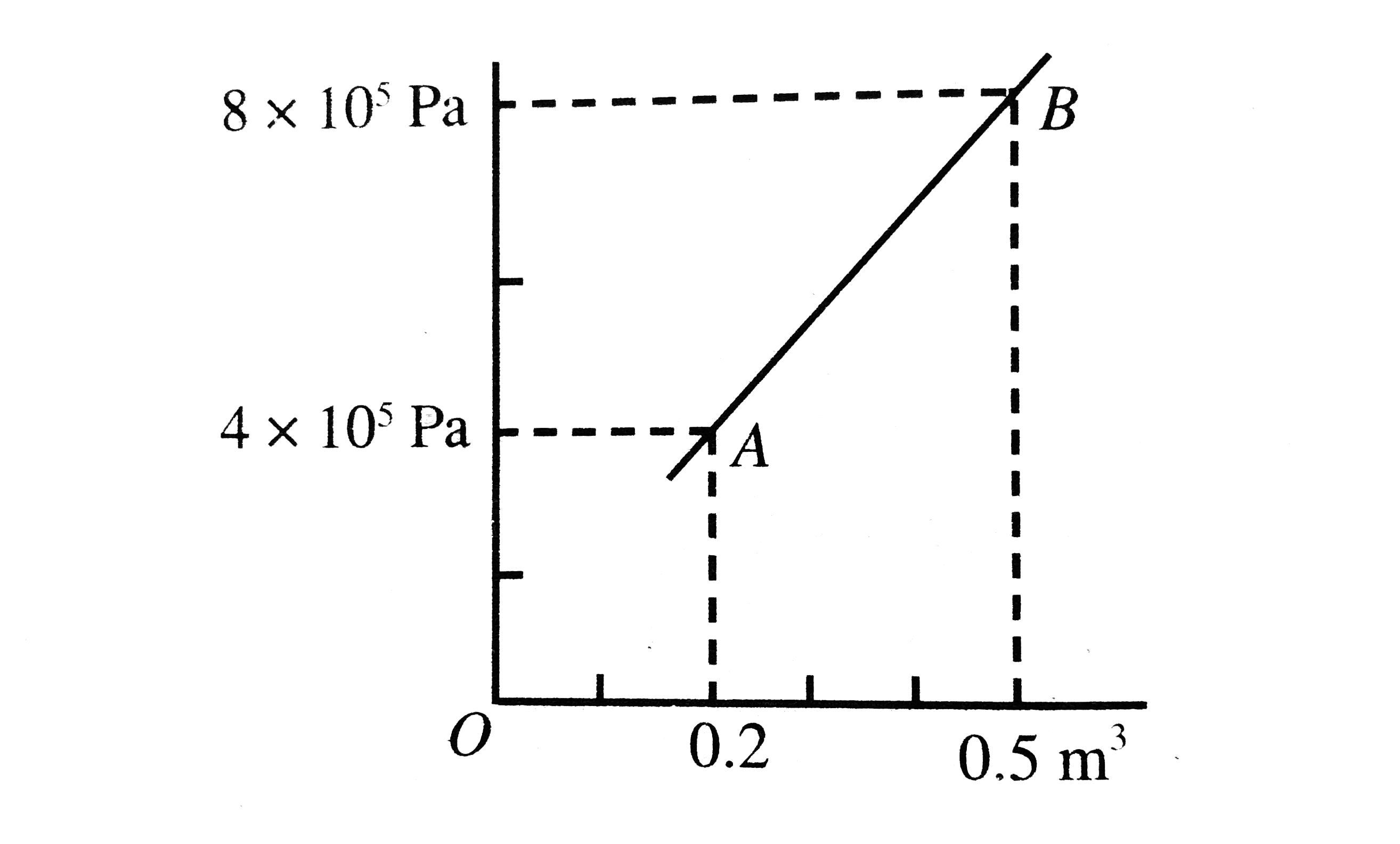
The volume of a monatomic ideal gas increases linearly with pressure, as shown in Fig. Calculate (a) increase in internal energy, (b) work done by the gas and (c ) heat supplied to the gas.

Video Solution

Text Solution

Verified by Experts

We know that for a perfect gas, internal energy is given by
$U = \frac{p V}{γ - 1}$
$\Rightarrow Δ U = \frac{p_{2} V_{2} - p_{1} V_{1}}{γ - 1}$
$∴ Δ U = \frac{8 \times 10^{5} \times 0.5 - 4 \times 10^{5} \times 0.2}{5 / 3 - 1} = 4.8 \times 10^{5} J$
Now, $Δ W = \int_{0.2}^{0.5} P d V$
Here, p varies with V along a straight line.
Therefore, one way say2 $p = k V + V_{0}$
At $p = 4 \times 10^{5}, V = 0.2 m^{2}$ and $p = 8 \times 10^{5}, V = 0.5 m^{3}$
$4 \times 10^{5} = k \times 0.2 + V_{0}$ and $8 \times 10^{5} = k \times 0.5 + V_{0}$
Here, $k = \frac{4}{3} \times 10^{6}$ and $V_{0} = \frac{4}{3} \times 10^{5}$
$p = \frac{4}{3} \times 10^{5} (10 V + 1)$
$Δ W = \int_{0.2}^{0.5} \frac{4}{3} \times 10^{5} {(\frac{V^{2}}{2})}_{0.2}^{0.5} + \frac{4}{3} \times 10^{5} {(V)}_{0.2}^{0.5}$
$\frac{4}{3} \times 10^{6} \times \frac{0.21}{2} + \frac{4}{3} \times 10^{5} \times 0.3 = 1.8 \times 10^{5} J$
By the first law of thermodynamics
$Δ Q = Δ U + Δ W$
$∴ Δ Q = 4.8 10^{5} + 1.8 \times 10^{5} = 6.6 \times 10^{5} J$

Updated on:21/07/2023

The internal energy of an ideal gas increases when it
AExpands
BIs compressed
CIs first expanded and then compressed
DNone of these
The internal energy of the gas increases In
AAdiabatic expansion
BAdiabatic compression
CIsothermal expansion
DIsothermal compression
A monatomic gas expands at constant pressure on heating. The percentage of heat supplied that increases the internal energy of the gas and that is involed in the expansion is
A $75 %, 25 %$
B $25 %, 75 %$
C $60 %, 40 %$
D $40 %, 60 %$