A rod of nickel of length is clamped at its midpoint . The rod is stuck and vibrations are set up in the rod . Find the general expression for the frequency of the longitudinal vibrations of the rod . Young's modulus and density of the rod is , respectively.
The correct Answer is:
To find the general expression for the frequency of the longitudinal vibrations of a nickel rod clamped at its midpoint, we can follow these steps:
Step 1: Understand the Setup
The rod has a length L and is clamped at its midpoint. This means that the rod can vibrate in modes where there are nodes at the midpoint.
Step 2: Determine the Wavelength for the Fundamental Mode
For the fundamental mode of vibration in a rod clamped at its midpoint, the length of the rod L is equal to half the wavelength (λ1) of the fundamental frequency. Thus, we can write:
L=λ12
This implies:
λ1=2L
Step 3: Relate Frequency, Wavelength, and Wave Speed
The speed of longitudinal waves in a rod is given by:
v=√Yρ
where Y is the Young's modulus and ρ is the density of the rod.
The relationship between speed, frequency, and wavelength is given by:
v=fλ
For the fundamental frequency f1:
v=f1λ1
Step 4: Substitute for Wavelength
Substituting λ1=2L into the wave speed equation gives:
v=f1(2L)
Thus, we can express the frequency as:
f1=v2L
Step 5: Substitute the Speed of Sound in the Rod
Now, substituting the expression for v:
f1=12L√Yρ
Step 6: Generalize for Higher Modes
For the n-th mode of vibration, the relationship changes. The general expression for the frequency of the n-th mode can be given by:
fn=(2n−1)2L√Yρ
where n is a positive integer (1, 2, 3, ...).
Final Expression
Thus, the general expression for the frequency of the longitudinal vibrations of the rod is:
fn=(2n−1)2L√Yρ
The rod has a length L and is clamped at its midpoint. This means that the rod can vibrate in modes where there are nodes at the midpoint.
For the fundamental mode of vibration in a rod clamped at its midpoint, the length of the rod L is equal to half the wavelength (λ1) of the fundamental frequency. Thus, we can write:
L=λ12
This implies:
λ1=2L
The speed of longitudinal waves in a rod is given by:
v=√Yρ
where Y is the Young's modulus and ρ is the density of the rod.
v=fλ
For the fundamental frequency f1:
v=f1λ1
Substituting λ1=2L into the wave speed equation gives:
v=f1(2L)
Thus, we can express the frequency as:
f1=v2L
Now, substituting the expression for v:
f1=12L√Yρ
For the n-th mode of vibration, the relationship changes. The general expression for the frequency of the n-th mode can be given by:
fn=(2n−1)2L√Yρ
where n is a positive integer (1, 2, 3, ...).
Thus, the general expression for the frequency of the longitudinal vibrations of the rod is:
fn=(2n−1)2L√Yρ
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Single Correct144videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple26videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Exercise 7.232videos
SUPERPOSITION AND STANDING WAVES
- BOOK - CENGAGE PHYSICSCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer16videos
SOUND WAVES AND DOPPLER EFFECT
- BOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - 241videos
THERMODYNAMICS
Similar Questions
Determine the possible harmonics in the longitudinal vibrations of a rod clampred in the middle.
View SolutionA metal rod of length 1m is clamped at two points as shown in the figure. Distance of the clamp from the two ends are 5 cm and 15 cm, respectively. Find the minimum and next higher frequency of natural longitudinal oscillation of the rod. Given that Young's modulus of elasticity and density of aluminium are Y=1.6×1011Nm−2 and ρ=2500kgm−3 , respectively.
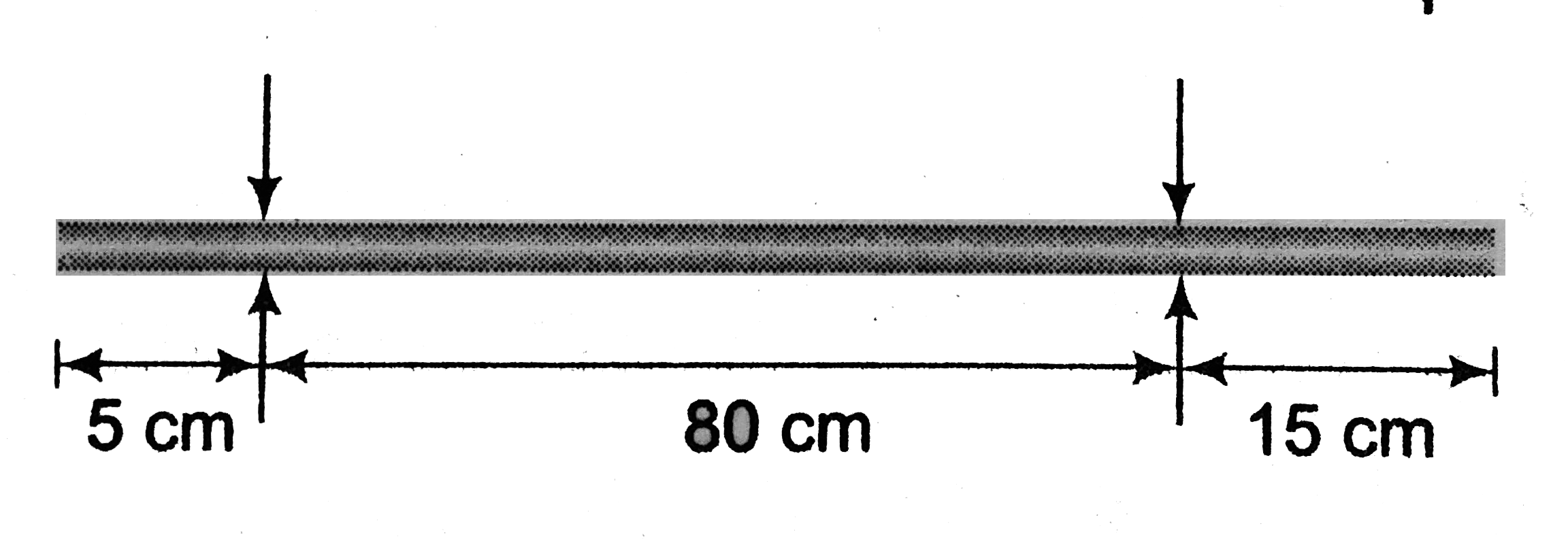 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A steel rod 100 cm long is clamped at its midpoint. The fundamental frequency of longitudinal vibrtions of the rod is 3 kHz. What is the speed of the sound in the rod?
A3×103ms−1B4×103ms−1C5×103ms−1D6×103ms−1 - Question 2 - Select One
A steel rod 100 cm long is clamped at its middle. The fundamental frequency of loungitudinal vibrations of the fundamental frequency of longitudinal vibrations of the rod are given to be 2.53 KHz. What is the speed of soind in steel?
A6.2 km/sB5.06 km/sC7.23 km/sD7.45 km/s - Question 3 - Select One
A steel rod of length 100 cm is clamped at the middle. The frequency of the fundamental mode for the longitudinal vibrations of the rod is
(Speed of sound in steel = 5 km s−1)A1.5 KHzB2 KHzC2.5 KHzD3 KHz
A copper rod of length l=50cm is clamped at its midpoint. Find the number of natural longitudinal oscillations of the rod in the frequency range from 20 to 50kHz. What are those frequencies equal to ?
View SolutionA metallic rod of length 1m is rigidly clamped at its midpoint. Longitudinal stationary waves are set up in the rod in such a way that there are two nodes on either side of the mid - point and those of constituent waves in the rod . (Y=2×1011N/m2andρ=8×103kg/m3)
View SolutionA rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the
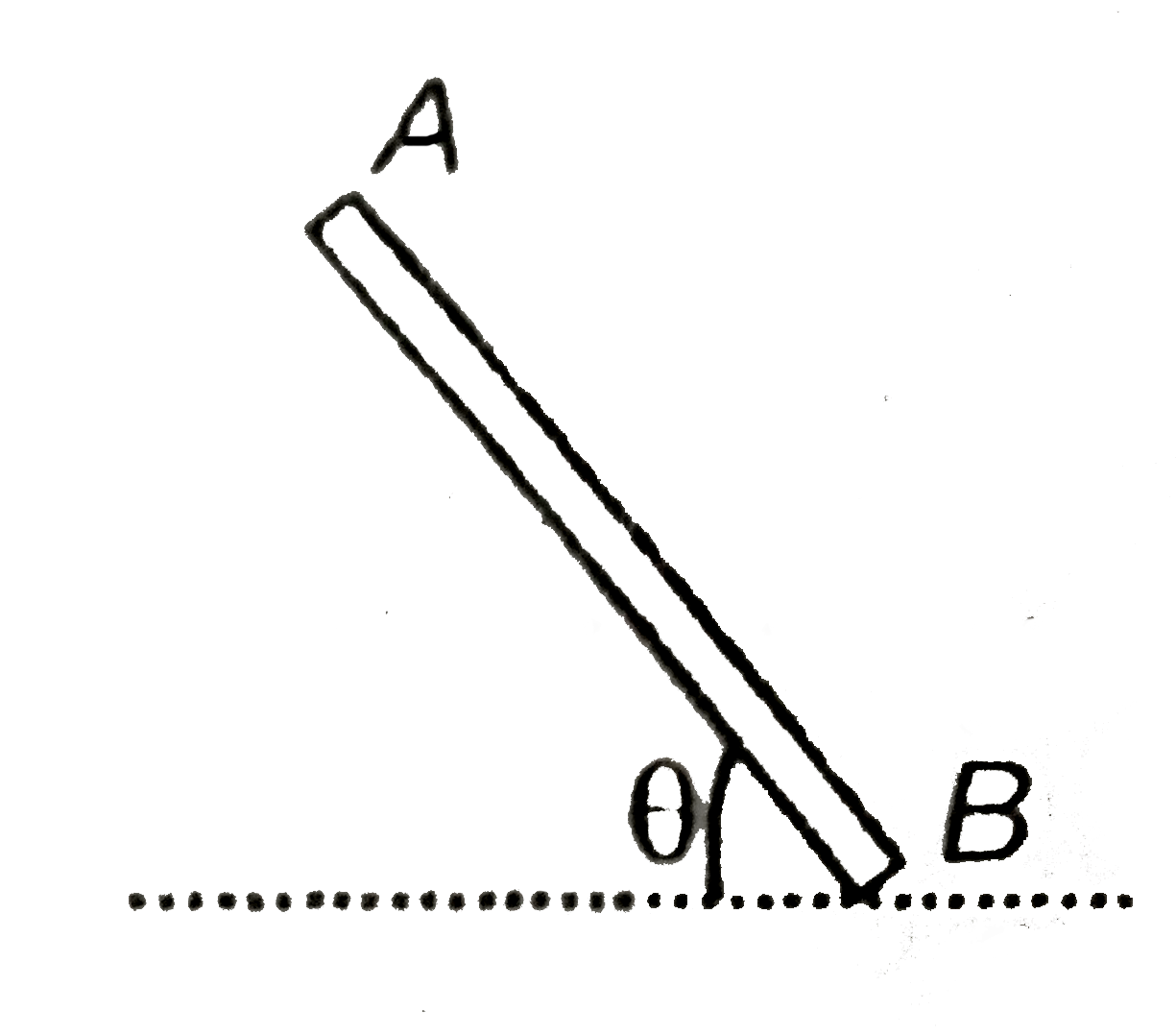
Normal reaction by ground on the rodView SolutionA metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The magnitude of strain at midpoint of the rod at t=1sec isView SolutionA metallic rod of length 1m has one end free and other end rigidly clamped. Longitudinal stationary waves are set up in the rod in such a way that there are total six antinodes present along the rod. The amplitude of an antinode is 4×10−6m. young's modulus and density of the rod are 6.4×1010N/m2 and 4×103Kg/m3 respectively. Consider the free end to be at origin and at t=0 particles at free end are at positive extreme.
The equation describing stress developed in the rod isView Solution

